MRSPTU

UNIT 3

Projections of Points

QUESTION BANKS

1. Explain Projections of Points

A point may be situated, in space, in any one of the four quadrants formed by the two principal planes of projection or may lie in any one or both. Its projections are obtained by extending projectors perpendicular to the planes.

One of the planes is then rotated so that the first and third quadrants are opened out. The projections are shown on a flat surface in their respective positions either above or below or in xy.

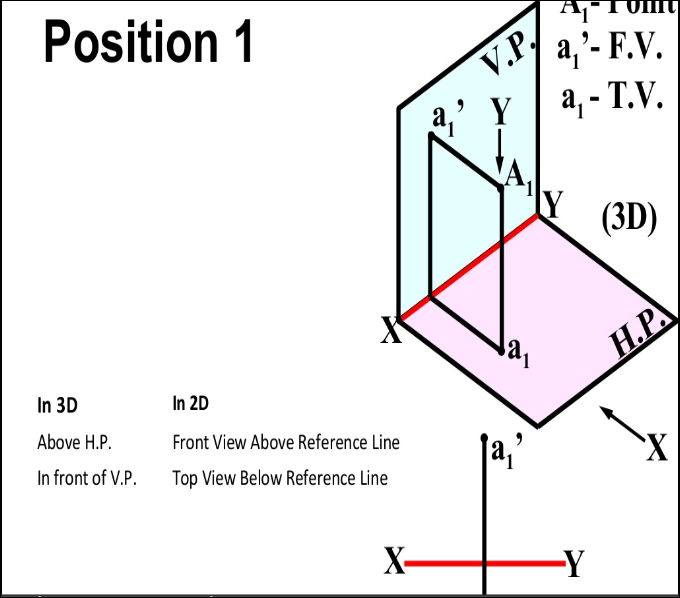
2. Draw if a point is situated in the first quadrant?

The pictorial view shows a point A situated above the H.P. And in front of the V.P., i.e. in the first quadrant. a' is its front view and the top view. After rotation of the plane

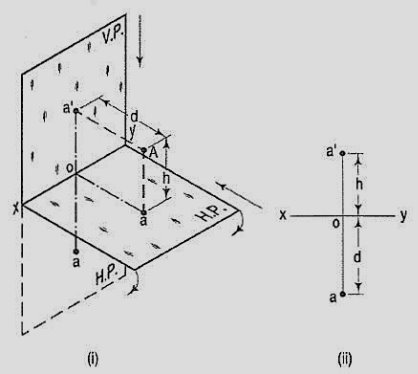
The front view a' is above xy and the top view a below it. The line joining a' and a (which also is called a projector), intersects xy at right angles at a point o. It is quite evident from the pictorial view that a'o = Aa, i.e. the distance of the front view from xy = the distance of A from the H.P. Viz. h. Similarly, ao = Aa',i.e. the distance of the top view from xy = the distance of A from the V.P. Viz. d.

3. Draw if a point is situated in the second quadrant?

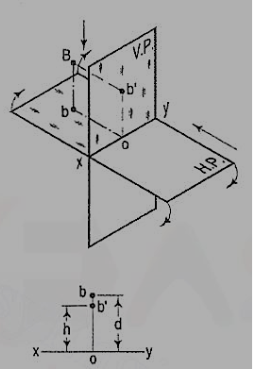
If a point is situated in second quadrant,

A point B is above the H.P. And behind the V.P., i.e. in the second quadrant. b' is the front view and b the top view. When the planes are rotated, both the views are seen above xy. Note that b'o = Bb and bo = Bb'.

4. Draw if a point is situated in the third quadrant?

If a point is situated in third quadrant,

A point C is below the H.P. And behind the Y.P., i.e. in the third quadrant. Its front view c' is below xy and the top view c above xy. Also, c'o = Cc and co = Cc'.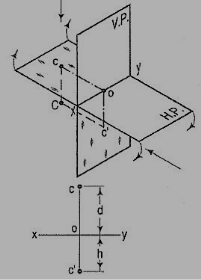

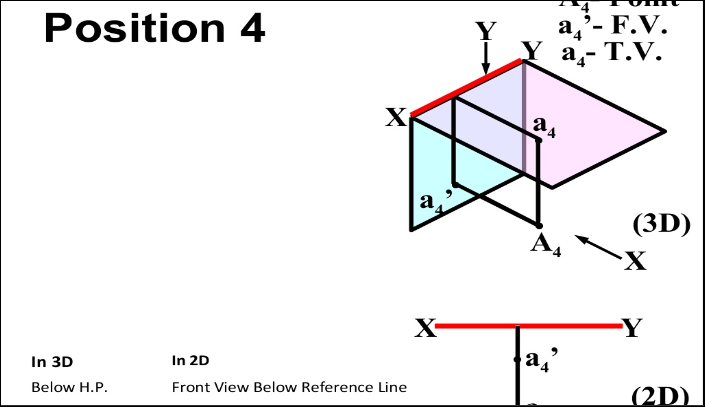
5. Draw if a point is situated in the forth quadrant?

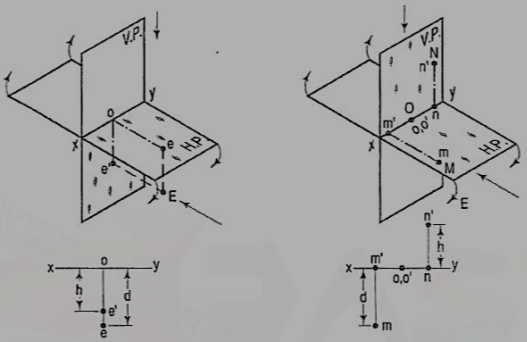
If a point is situated in 4th quadrant,

A point f is below the H.P. And in front of the V.P., i.e. in the fourth quadrant. Both its projections are below xy, and e'o = Ee and eo = Ee'.

6 . A point P is in the first quadrant. Its shortest distance from the intersection point of H.P., V.P. And Auxiliary vertical plane, perpendicular to the H.P. And V.P. Is 70 mm and it is equidistant from principal planes (H.P. And V.P.). Draw the projections of the point and determine its distance from the H.P. And V.P.

Note: ‘O’ represents intersection of H.P., V.P. And A.V.P.

Drawing steps:

1. Draw xy and x1 y1 perpendicular reference lines.

2. ‘O’ represents intersection of H.P., V.P. And A.V.P.

3. Draw from O a line inclined at 45° of 70 mm length.

4. Project from P" on xy line and x1 y1. The projections are n and m respectively as shown in figure. From O draw arc intersecting x1 y1.

5. Draw a parallel line at convenient distance from x1 y1. Extend P"m to intersect

a parallel line at p' and p as shown.

6. Measure distance from xy line, which is nearly 49.4974 mm say 49.5 mm.

7. What are the types of Projection of Points ?

There are basically nine type of projections of point is space :

1. In FIRST Quadrant (Above H.P. , In front of V.P.)
2. In SECOND Quadrant (Above H.P. , Behind V.P.)
3. In THIRD Quadrant (Below H.P. , Behind V.P.)
4. In FOURTH Quadrant (Below H.P. , In front of V.P.)
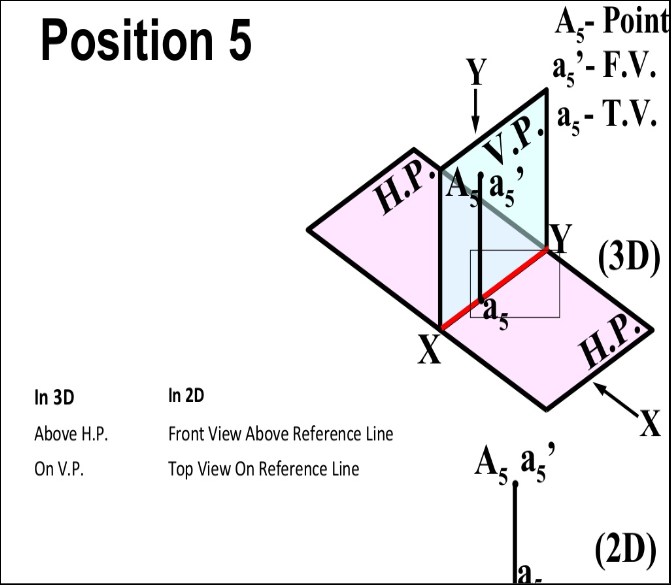
5. In PLANE (On V.P. , Above H.P.)
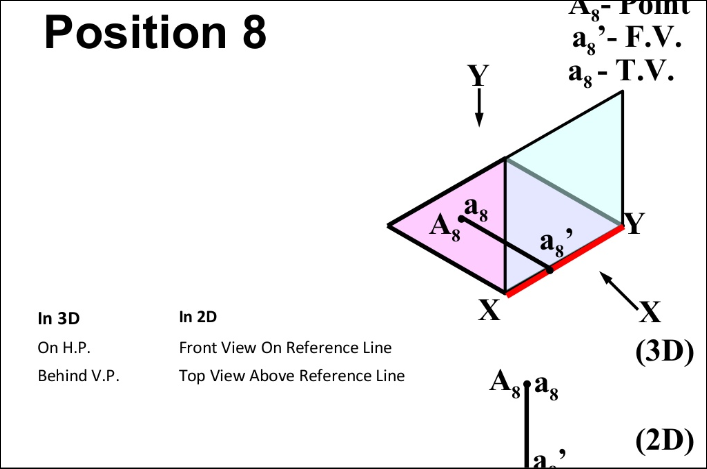
6. In PLANE (On H.P. , Behind V.P.)
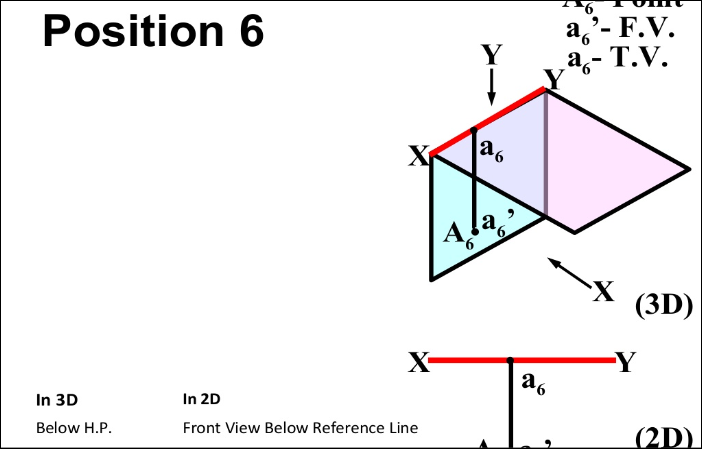
7. In PLANE (On V.P. , Below H.P.)
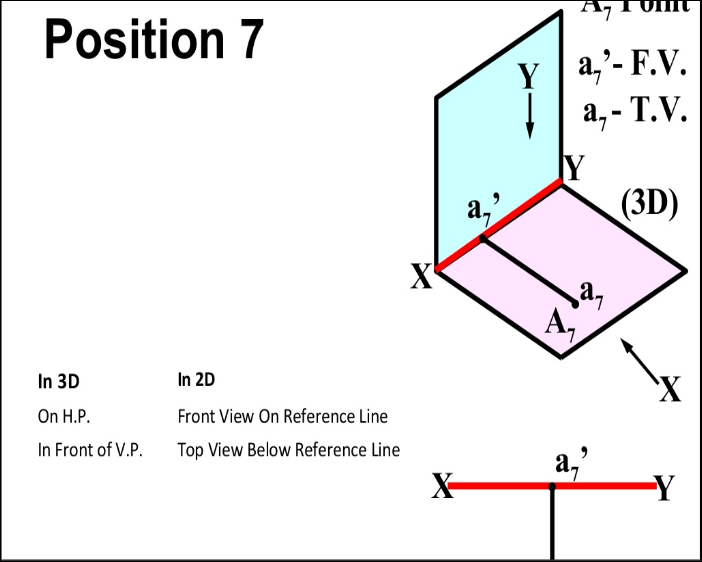
8. In PLANE (On H.P. In front of V.P.)
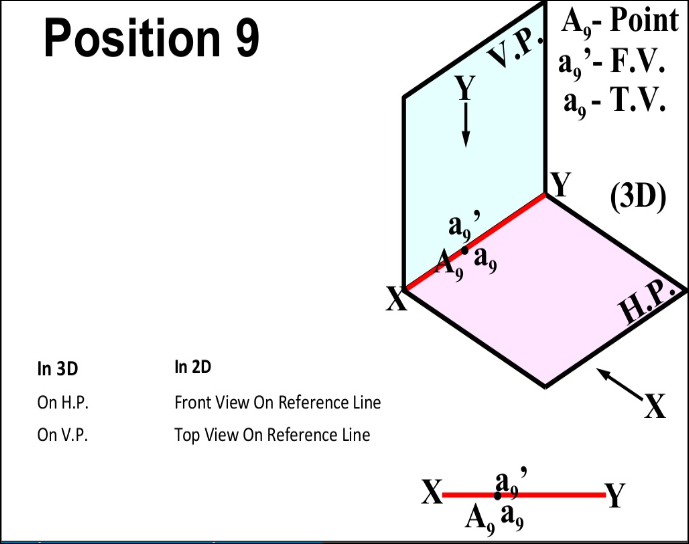
9. In PLANE (On both H.P. & V.P.)