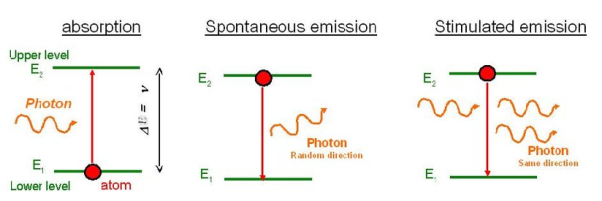
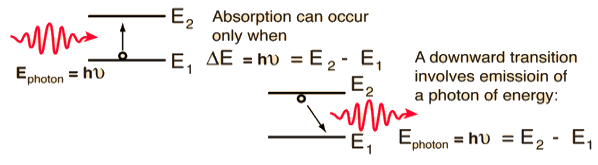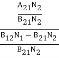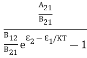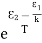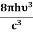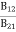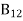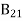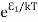Ruby laser is a threelevel solidstate laser and was constructed by Maiman in 1960. Ruby laser is one of the few solidstate lasers that produce visible light. It emits deep red light of wavelength 694.3 nm.

PHY

UNIT-3LASERS AND APPLICATIONSQ1) Differentiate between spontaneous and stimulated emissions.A1)

Spontaneous emission	Stimulated emission
1.The spontaneous emission was postulated by Bohr	1.The stimulated emission was postulated by Einstein
2. Additional photons are not required in spontaneous emission	2. Additional photons are required in stimulated emission
3.One photon is emitted in spontaneous emission	3.Two photons are emitted in stimulated emission
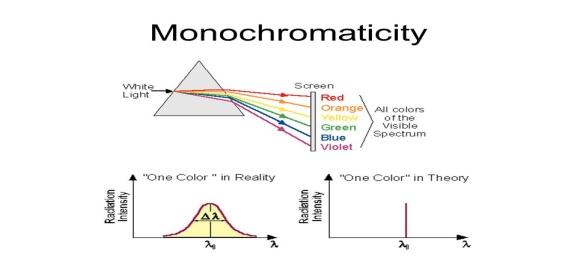
4.The emitted radiation is poly-monochromatic	4.The emitted radiation is monochromatic
5. The emitted radiation is Incoherent	5. The emitted radiation is Coherent
6. The emitted radiation is less intense	6. The emitted radiation is high intense
7.The emitted radiation has less directionality	7.The emitted radiation has high directionality
8. Example: light from sodium or mercury lamp	8. Example: light from the laser source.

Q 2) What is LASER? Discuss basic phenomena involved in producing laser light?A2)LASER stands for “Light Amplification by Stimulated Emission of Radiation”.L = LightA = Amplification (by)S = StimulatedE = Emission (of)R = RadiationTheodore H. Maiman of the Hughes Research Laboratory, California, was the first scientist who experimentally demonstrated laser by flashing light through a ruby crystal in 1960. But the basic idea behind the development of laser was given by the great scientist “Albert Einstein” in 1917. Let us discuss Einstein’s theory of the interaction of electromagnetic radiation with matter. He proposed that electromagnetic radiation interacts with matter in the following three steps.

Stimulated Absorption

Spontaneous Emission

Stimulated Emission

Stimulated Absorption:Let E1 and E2 be the energies of the ground and excited states of an atom. Suppose, if a photon of energy hν= E1− E2 interacts with an atom present in the ground state, the atom gets excitation from ground state E1 to excited state E2. This process is called stimulated absorption. Stimulated absorption rate depends upon the number of atoms available in the lowest energy state as well as the energy density photons.

Stimulated absorption rate ∝ Number of atoms in the ground state

∝ The density of photons Spontaneous emission

Spontaneous Emission: Let E1 and E2 be the energies of the ground and excited states of an atom. Suppose, if a photon of energy hν= E1− E2 interacts with an atom present in the ground state, the atom gets excitation from ground stateE1 to excited state E2. The excited atom does not stay a long time in the excited state. The excited atom gets de-excitation after its lifetime by emitting a photon of energy hν= E1− E2. This process is called spontaneous emission. Also Spontaneous means by its own. Here excited atom comes to the ground state on its own so it is named as spontaneous emission.The spontaneous emission rate depends upon the number of atoms present in the excited state.Spontaneous emission ∝ rate number of atoms in the excited state Stimulated Emission: This phenomenon is responsible for producing laser light. Let E1and E2 be the energies of the ground and excited states of an atom. Suppose, if a photon of energy hν= E1− E2 interacts with an atom present in the ground state, the atom gets excitation from ground stateE1 to excited state E2. Let, a photon of energy hν= E1− E2 interacts with the excited atom within their lifetime; the atom gets de-excitation to the ground state by emitting another photon. These photons have the same phase and it follows coherence. This phenomenon is called stimulated emission. Stimulated emission rate depends upon the number of atoms available in the excited state as well as the energy density of photons.

Stimulated emission rate ∝ number of atoms in the excited state

∝ Density of photons

Q3) Discuss Properties of Laser? A3)PROPERTIES OF LASERThe laser light exhibits some peculiar properties compare with the conventional light. Those are 1. Highly monochromatic2. Highly coherence 3. Highly directionality 4. Highly intense5. Laser Speckles 1. Highly monochromaticMonochromatic light means a light containing a single colour or wavelength. The photons emitted from ordinary light sources have different energies, frequencies, wavelengths, or colours. Ordinary light is a mixture of waves having different frequencies or wavelengths. The light waves of laser have a single wavelength or colour.

Figure 5: MonochromaticTherefore, laser light covers a very narrow range of frequencies or wavelengths.Hence The laser light is more monochromatic than that of a conventional light source. This may be due to the stimulated characteristic of laser light. The bandwidth of the conventional monochromatic light source is 1000 Å. But the bandwidth of an ordinary light source is 10 Å. For high sensitive laser source is 10-8 Å.

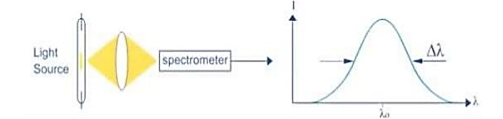
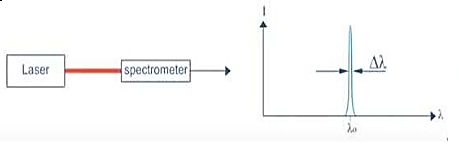
Figure 6: Intensity- wavelength graph for light and laser

2. Highly coherence Definition: A predictable correlation of the amplitude and phase at any one point with another point is called coherence.

Figure 7: Incoherence and coherenceTwo waves are said to be coherent, the waves must have In the case of conventional light, the property of coherence exhibits between a source and its virtual source whereas in the case of laser the property coherence exists between any two or more light waves. There are two types of coherencei) Temporal coherence ii) Spatial coherence Temporal coherence (or longitudinal coherence):-The predictable correlation of amplitude and phase at one point on the wave train w. r. t another point on the same wave train, then the wave is said to be temporal coherence To understand this, let us consider two points P1 and P2 on the same wave train, which is continuous as shown in the figure.

Figure 8: Wave trainSuppose the phase and amplitude at any one point is known, then we can easily calculate the amplitude and phase for any other point on the same wave train by using the wave equation

y= a sin ( (ct-x))

Where ‘a’ is the amplitude of the wave and ‘x’ is the displacement of the wave at any instant of time‘t’.Spatial coherence (or transverse coherence) The predictable correlation of amplitude and phase at one point on the wave train w. r. t another point on a second wave, then the waves are said to be spatial coherence (or transverse coherence)

Figure 9: Spatial coherence3. Highly directionalityThe light ray coming from an ordinary light source travels in all directions, but laser light travels in a single direction. For example, the light emitted from torchlight spreads 1km distance it spreads 1 km distance. But the laser light spreads a few centimetres distance even it travels lacks kilometre distance.The directionality of the laser beam is expressed in terms of divergence ∆θ =

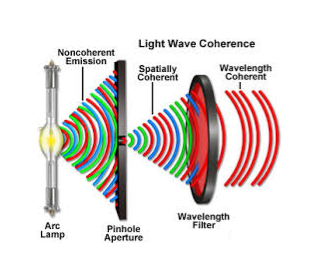
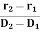Where r1 and r2 are the radii of laser beam spots at distances of D1 and D2 respectively from the laser source. 4. Highly Intense or Brightness We know that the intensity of a wave is the energy per unit time flowing through a unit's normal area. Laser light is highly intense than conventional light. A one mill watt He-Ne laser is highly intense than the sun intensity. This is because of the coherence and directionality of the laser. Suppose when two photons each of amplitude a are in phase with other, then young’s principle of superposition, the resultant amplitude of two photons is 2a and the intensity is 4a2. Since in laser many numbers of photons are in phase with each other, the amplitude of the resulting wave becomes na and hence the intensity of the laser is proportional to n2a2. So 1mW He-Ne laser is highly intense than the sun.In an ordinary light source, the light spreads out uniformly in all directions. If you look at a 100 Watt lamp filament from a distance of 30 cm, the power entering your eye is less than 1/1000 of a watt. If you look at laser beam X(caution: don’t do it at home, direct laser light can damage your eyes)X, then all the power in the laser would enter your eye. Thus, even a 1 Watt laser would appear many thousand times more intense than a 100 Watt ordinary lamp. 5. Laser Speckles The term speckle refers to a random granular pattern that can be observed when a highly coherent light beam is diffusely reflected at a surface with a complicated structure. This phenomenon results from the interference of different reflected portions of the incident beam with random relative optical phases.

Figure 10: Laser SpecklesEven minor changes of the conditions, such as of the illuminated spot or the direction of the incident laser beam, can change the detailed shape of a speckle pattern.When laser light that has been scattered off a rough surface falls on another surface, it forms an "objective speckle pattern". If a photographic plate or another 2-D optical sensor is located within the scattered light field without a lens, a speckle pattern is obtained whose characteristics depend on the geometry of the system and the wavelength of the laser. Q4) Derive Einstein’s coefficient A and B? A4) The distribution of atoms in the two energy levels will change by absorption or emission of radiation. Einstein introduced three empirical coefficients to quantify the change of population of the two levels. Let N1 be the number of atoms per unit volume with energy E1 and N2 be the number of atoms per unit volume with energy E2. Let ‘n’ be the number of photons per unit volume at frequency ‘υ’ such that hυ= E1− E2. Then, the energy density of photons ρ(υ) = nhυ

Description: http://hyperphysics.phy-astr.gsu.edu/hbase/imgmod/qpro1.gif

When these photons interact with atoms, both upward (absorption) and downward (emission) transition occurs. At the equilibrium, the upward transitions must be equal to downward transitions.Upward Transition Stimulated absorption rate depends upon the number of atoms available in the lowest energy state as well as the energy density photons.We have seen above that

Stimulated absorption rate ∝ N1 i.e. Number of atoms in the ground state

∝ ρ(υ) i.e. Density of photons spontaneous emission

Stimulated absorption rate = B12N1ρ(υ) ………(1)

Where B12 is the Einstein coefficient of stimulated absorption.

Downward transition

The spontaneous emission rate depends upon the number of atoms present in the excited state.

Spontaneous emission rate ∝ N2 i.e. number of atoms in the excited state

Spontaneous emission rate = A21N2 ………(2)

Where A21 is the Einstein coefficient of spontaneous emission.

Stimulated emission rate depends upon the number of atoms available in the excited state as well as the energy density of photons.

Stimulated emission rate ∝ N2 i.e. number of atoms in the excited state

∝ ρ(υ) i.e. Density of photons

Stimulated emission rate = B21N2ρ(υ) ………(3)

If the system is in equilibrium the upward transitions must be equal to downward transitions.

upward transitions = downward transitions

B12N1ρ(υ) = A21N2 + B21N2ρ(υ) ………(4)

B12N1ρ(υ) - B21N2ρ(υ) = A21N2

(B12N1- B21N2) ρ(υ) = A21N2

ρ(υ) = ………(5)

Divide with B21N2 in numerator and denominator in the right side of the above equation,

ρ(υ) = = ………(6)

ρ(υ) = = = ………(7)

We know from Maxwell Boltzmann distribution law

= ………(8)

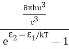
And also from Planck’s law, the radiation density

ρ(υ) = ………(9)

Comparing the two equations (7) and (9)

= and =1 ………(10)

The above relations are referred to as Einstein relations.

From the above equation for non-degenerate energy levels, the stimulated emission rate is equal to the stimulated absorption rate at the equilibrium condition.

= ………(11)

Q5) What is the relationship between B21 and B12?
a) B12 > B21
b) B12 < B21
c) B12 = B21
d) No specific relationA5)C is the correct answer.
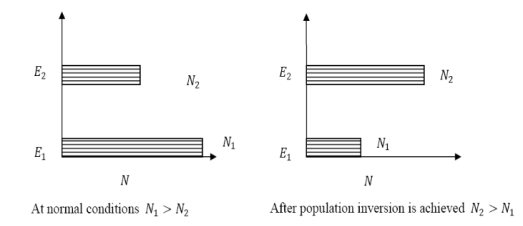
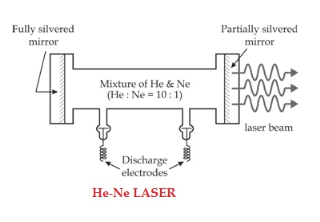
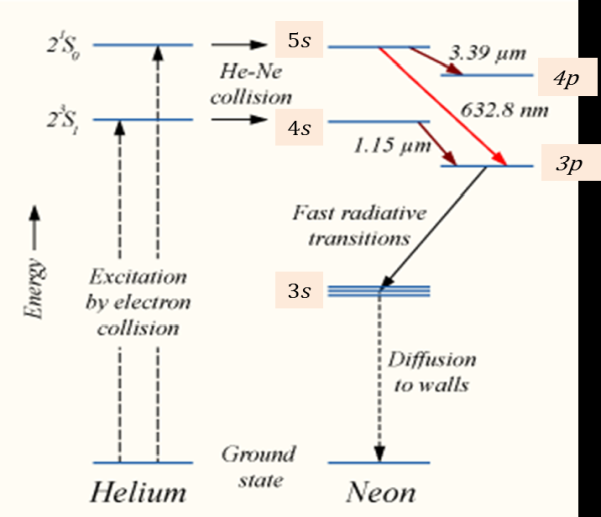
B21 is the coefficient for the stimulated emission while B12 is the coefficient for stimulated absorption. Both the processes are mutually reverse processes and their probabilities are equal. Therefore, B12 = B21. Q6) How laser light get amplify by population inversion?A6)Population inversionDefinitionThe number of atoms present in the excited state or higher energy state is greater than the number of atoms present in the ground state or lower energy state is called population inversion.Population inversion as the name suggests that this is an inverted phenomenon. In general, the lower energy level is more populated which means it has more number atoms in the lower energy level as compared to a higher energy level. But by pumping we will obtain a state when the number of atoms present in the higher energy state is greater than the number of atoms present in the lower energy state.Let us consider a two-level energy system of energies E1 and E2 as shown in the figure. Let N1 and N2 be the populations that mean several atoms per unit volume of energy levels E1 and E2 respectively.According to Boltzmann’s distribution the population of an energy level E, at temperature T is given by

Ni=N0

Where N0 is the population of the lower level or ground state and k is Boltzmann’s constant. From the above relation, the population of energy levels E1 and E2 are

N1=N0

N2=N0

At ordinary conditions N1 >N2 i.e., the population in the ground or lower state is always greater than the population in the excited or higher states. The stage of making, the population of higher energy level is greater than the population of lower energy level is called population inversion i.e. N1 < N2

When the population inversion method is used to enforce more and more atoms to give up photons. This initiates a chain reaction and releasing a massive amount of energy.This results in a rapid build-up of the energy of emitting one particular wavelength traveling coherently in a fixed direction. This process is called amplification by stimulated emission using population inversion.This population inversion situation is essential for laser action. For any stimulated emission, the upper energy level or metastable state must have a long lifetime, i.e., the atoms should pause at the metastable state for more time than at the lower level. Q7) What is Pumping? Discuss the various type of pumping mechanisms? A7)For laser action, the pumping mechanism (exciting with external source) maintain a higher population of atoms in the upper energy level relative to that in the lower level.A system in which population inversion is achieved is called an active system. The method of raising the particles from a lower energy state to a higher energy state is called pumping. The process of achieving population inversion is called pumping. This can be done in several ways. The most commonly used pumping methods are

Optical pumping

Electrical discharge pumping

Chemical pumping

Thermal Pumping

Injection current pumping

Inelastic Atom-Atom Collisions

Optical pumping As the name suggests, in this method, light is used to supply energy to the laser medium. Optical pumping is used in solid laser. Xenon flash tubes are used for optical pumping. Since these materials have very broadband absorption, a sufficient amount of energy is absorbed from the emission band of the flash lamp and population inversion is created. So xenon flash lamp is used to produce more electrons in the higher energy level of the laser medium. Examples of optically pumped lasers are ruby, Nd: YAG Laser (Neodymium: Yttrium Aluminum Garnet).Electrical discharge pumping is used in gas lasers. Since gas lasers have a very narrow absorption band pumping then any flash lamp is not possible. Electric discharge refers to the flow of electrons or electric current through a gas, liquid, or solid. In this method of pumping, electric discharge acts as the pump source or energy source. A high voltage electric discharge (flow of electrons, electric charge, or electric current) is passed through the laser medium or gas. The intense electric field accelerates the electrons to high speeds and they collide with neutral atoms in the gas. As a result, the electrons in the lower energy state gains sufficient energy from external electrons and jumps into the higher energy state Examples of Electrical discharge pumped lasers are He-Ne laser, CO2 laser, argon-ion laser, etc. Chemical pumping Chemical reaction may also result in excitation and hence the creation of population inversion in a few systems. If an atom or a molecule is produced through some chemical reaction and remains excited at the time of production, then it can be used for pumping. The hydrogen fluoride molecule is produced in an excited state when hydrogen and fluorine gas chemically combine. The number of produced excited atoms or molecules is greater than the number of normal state atoms or molecules. Thus, population inversion is achieved. Examples H2 + F2 → 2HF, in this chemical reaction, hydrogen (H2) and fluorine (F2) molecules are chemically combined to produce hydrogen fluoride molecule (2HF) in an excited state.Thermal Pumping: Sometimes we can achieve population inversion by heating the laser medium. In thermal pumping, heat acts as the pump source or energy source. In this method, population inversion is achieved by supplying heat into the laser medium.When heat energy is supplied to the laser medium, the lower energy state electrons gain sufficient energy and jumps into the higher energy level. The process of achieving population inversion in thermal pumping is almost similar to the optical pumping or electric discharge method, except that in this method heat is used as a pump source instead of light or electric discharge.Injection current pumping In semiconductors, injection of current through the junction results in creates of population inversion among the minority charge carriers.Examples of such systems are InP and GaAs.Inelastic Atom-Atom Collisions: Like the electric discharge method, here also a high voltage electric discharge acts as a pump source. However, in this method, a combination of two types of gases, say X and Y are used. The excited state of gas X is represented as X+ whereas gas Y is represented as Y+. Both X and Y gases have the same excited states (X+ and Y+).When high voltage electric discharge passes through a laser medium having two types of gases X and Y, the lower energy state electrons in gas X will move to the exciting state X+ similarly the lower energy state electrons in gas Y moves to the excited state Y+. Initially, during electric discharge, the lower energy state electrons in gas X or atom X gets excited to X+ due to continuous collision with electrons. The excited state electrons in gas X+ now collide with the lower energy state electrons in gas Y. As a result, the lower energy state electrons in gas Y gains sufficient energy and jump into the exciting state Y+. This method is used in the Helium-Neon (He-Ne) laser. Q8) Discuss the construction, working, and application of gas Laser? OrDiscuss the construction, working, and application of He-Ne Laser?OrDiscuss four-level laser?A8)He-Ne Laser The first He-Ne gas laser was fabricated in 1961 by Ali Javan, Bennett, and Herriott at Bell Telephone Laboratories. others. Helium-Neon laser is a type of gas laser in which a mixture of helium and neon gas is used as a gain medium. Helium-Neon laser is also known as He-Ne laser. The helium-neon laser was the first continuous-wave laser ever constructed. The helium-neon laser operates at a wavelength of 632.8 nanometres (nm), in the red portion of the visible spectrum.Ruby laser is a pulse laser, even it has high intense output. For continuous laser beams, gas lasers are used. Using gas lasers, we can achieve high coherence, high directionality, and high monochromaticity beam. The output power of the gas laser is generally in few milliwatts. CONSTRUCTIONThe helium-neon laser consists of three essential components:

Pump source (high voltage power supply)

Gain medium (laser glass tube or discharge glass tube)

Resonating cavity

Pump source The gain medium of a helium-neon laser is made up of a mixture of helium and neon gas contained in a glass tube at low pressure. In the He-Ne gas laser, the He and Ne gases are taken in the ratio 10:1 in the discharge tube. Gain medium In He-Ne laser 80cm length and 1cm diameter discharge are generally used. The out power of these lasers depends on the length of the discharge tube and the pressure of the gas mixture. Therefore, to achieve population inversion, we need to pump electrons from the lower energy state of the helium. In He-Ne laser, neon atoms are the active centres and have energy levels suitable for laser transitions while helium atoms help in exciting neon atoms.

Resonating cavityTwo reflecting mirrors are fixed on either end of the discharge tube, in that, one is partially reflecting and the other is fully reflecting. The fully silvered mirror will completely reflect the light whereas the partially silvered mirror will reflect most of the light but allows some part of the light to produce the laser beam. WORKING When the electric discharge is passing through the gas mixture, the electrons accelerated towards the positive electrode. During their passage, they collide with He atoms and excite them into higher levels. 23s1 and 21s0 form the ground state of the He atom. In higher levels, 23s1 and 21s0, the lifetime of He atoms are more. So there is a maximum possibility of energy transfer between He and Ne atoms through atomic collisions. When He atoms present in the levels 23s1 and 21s0 collide with the Ne atom's present ground state, the Ne atoms get excitation into higher levels 4s and 5s. Due to the continuous excitation of Ne atoms, we can achieve the population inversion between the higher levels 4s and 5s and lower levels 3p and 4p. The various transitions 5s to 4p, 4s to 3p, and 5s to 3p leads to the emission of wavelengths 3.93μm, 1.51μm, and 6328 Å or 632.8μm.

The first two correspondings to the infrared region while the last wavelength is corresponding to the visible region. The Ne atoms present in the 4s level are de-excited into 3s level, by spontaneously emitting a photon of around wavelength 6000 Å. When a narrow discharge tube is used, the Ne atoms present in the level 3s collide with the walls of the tube and get de-excited to the ground state energy level. ADVANTAGES OF HELIUM-NEON LASER

The helium-neon laser emits laser light in the visible portion of the spectrum.

High stability

Low cost

Operates without damage at higher temperatures

DISADVANTAGES OF HELIUM-NEON LASER

Low efficiency

Low gain

Helium-neon lasers are limited to low power tasks

APPLICATIONS OF HELIUM-NEON LASERS

Helium-neon lasers are used in industries.

Helium-neon lasers are used in scientific instruments.

Helium-neon lasers are used in the college laboratories

Q9) Discuss Applications of Lasers In various fields?A9)Applications of lasers because of the unique property of laser beams such as coherence, monochromaticity, directionality, and high intensity, they are widely used in various fields like 1. Communication 2. Computers 3. Chemistry 4. Photography 5. Industry 6. Medicine 7. Military 8. Scientific Research 1. Communication In the case of optical communication semiconductors, laser diodes are used as optical sources and their bandwidth is (1014Hz) is very high compared to radio and microwave communications. More channels can be sent simultaneously Signal cannot be tapped as the bandwidth is large, more data can be sent. A laser is highly directional and less divergence, hence it has greater potential use in space crafts and submarines. It is used in optical fiber communications to send information over large distances with low loss. Laser light is used in underwater communication networks. Lasers are used in space communication, radars, and satellites. 2. Computers In LAN (local area network), data can be transferred from memory storage of one computer to another computer using laser for short time. Lasers are used in CD-ROMS during recording and reading the data. Lasers are used in computer printers. 3. Chemistry Lasers are used in molecular structure identification Lasers are also used to accelerate some chemical reactions. Using lasers, new chemical compounds can be created by breaking bonds between atoms are molecules. 4. Photography Lasers can be used to get a 3-D lens with less photography. Lasers are also used in the construction of holograms. 5. Industry Lasers can be used to blast holes in diamonds and hard steel. Lasers are also used as a source of intense heat Carbon dioxide laser is used for cutting drilling of metals and non-metals, such as ceramics plastics glass, etc. High power lasers are used to weld or melt any material. Lasers are also used to cut teeth in saws and test the quality of the fabric. It is used to cut glass and quartz, used in electronic industries for trimming the components of Integrated Circuits (ICs). Lasers are used for heat treatment in the automotive industry. Laser light is used to collect information about the prefixed prices of various products in shops and business establishments from the bar code printed on the product. Ultraviolet lasers are used in the semiconductor industries for photolithography. Photolithography is the method used for manufacturing printed circuit board (PCB) and microprocessor by using ultraviolet light. It is also used to drill aerosol nozzles and control orifices within the required precision. 6. Medicine Pulsed neodymium laser is employed in the treatment of liver cancer. Argon and carbon dioxide lasers are used in the treat men of the liver and lungs. Lasers are used in the treatment of Glaucoma. Lasers are used in endoscopy to scan the inner parts of the stomach. Lasers are used in the elimination of moles and tumors which are developing in the skin tissue and hair removal. It is also used for bloodless surgery.Lasers are used to destroy kidney stones, in cancer diagnosis and therapy also used for eye lens curvature corrections. Lasers are used to study the internal structure of microorganisms and cells. It is used to create plasma. Lasers are used to remove caries or decayed portion of the teeth. 7. Military Lasers can be used as a war weapon. High energy lasers are used to destroy the enemy air-crofts and missiles. Lasers can be used in the detection and ranging likes RADAR. Laser range finders are used to determine the distance to an object. The ring laser gyroscope is used for sensing and measuring a very small angle of rotation of the moving objects.Lasers can be used as a secretive illuminator for reconnaissance during the night with high precision. 8. Scientific research Lasers are used in the field of 3D-photography Lasers are used in Recording and reconstruction of the hologram. Lasers are employed to create plasma. Lasers are used in Raman spectroscopy to identify the structure of the molecule and to count the number of atoms in a substance. Lasers are used in the Michelson- Morley experiment. A laser beam is used to confirm Doppler shifts in frequency for moving objects. A laser helps in studying the Brownian motion of particles. With the help of a helium-neon laser, it was proved that the velocity of light is the same in all directions. Lasers are used to measure the pollutant gases and other contaminants of the atmosphere. Lasers help in determining the rate of rotation of the earth accurately. Lasers are used for detecting earthquakes and underwater nuclear blasts. A gallium arsenide diode laser can be used to set up an invisible fence to protect an area. Q10) Discuss the construction, working, and application of Solid-State Laser? Or Discuss the construction, working, and application of Ruby Laser?Or Discuss three-level laser? A10)Ruby laser

Ruby laser is a three-level solid-state laser and was constructed by Maiman in 1960. Ruby laser is one of the few solid-state lasers that produce visible light. It emits deep red light of wavelength 694.3 nm.Construction A ruby laser consists of three important elements: laser medium, the pump source, and the optical resonator. Laser MediumRuby (Al2O3+Cr2O3) is a crystal of Aluminium oxide, in which 0.05% of Al+3 ions are replaced by the Cr+3 ions. The colour of the rod is pink. The active medium or laser medium in the ruby rod is Cr+3 ions. In ruby laser, 4cm length and 5mm diameter rod is generally used. The ruby has good thermal properties.

Figure 13: Ruby laser The pump source

The ruby rod is surrounded by a xenon flash tube, which provides the pumping light to excite the chromium ions into upper energy levels. The ruby rod was surrounded by a helical xenon flash lamp.We know that population inversion is required to achieve laser emission. Population inversion is the process of achieving a greater population of a higher energy state than the lower energy state. To achieve population inversion, we need to supply energy to the laser medium i.e. to ruby crystal. Xenon flash tube emits thousands of joules of energy in few milliseconds, but only a part of that energy is utilized by the chromium ions while the rest energy heats the apparatus. A cooling arrangement is provided to keep the experimental set up at normal temperatures.Optical resonator

Both the ends of the rods are highly polished and made strictly parallel. The ends are silvered in such a way, one becomes partially reflected the laser beam was emitted through that end and the other end fully reflected to reflect all the rays of light striking it. Working of ruby laser:Consider a ruby laser medium consisting of three energy levels E1, E2, E3 with N number of electrons. We assume that the energy levels will be E1 < E2 < E3. The energy level E1 is known as the ground state or lower energy state, the energy level E2 is known as the metastable state, and the energy level E3 is known as the pump state.Let us assume that initially most of the electrons are in the lower energy state (E1) and only a tiny number of electrons are in the excited states (E2 and E3).The energy level diagram of chromium ions is shown in the figure. The chromium ions get excitation into higher energy levels by absorbing 5500Å of wavelength radiation. The excited chromium ions stay in the level E3 for a short interval of time (10-8 to 10-9 Sec). After their life, most of the chromium ions are de-excited from E3 to E1 and a few chromium ions are de-excited from E3 to E2.

Figure 14: Energy Level diagram of ruby laser

The transition between E3 and E2 is non-radioactive i.e. the chromium ions give their energy to the lattice in the form of heat. In the Metastable state, the lifetime of chromium ions is 10-3 sec. The lifetime of chromium ions in the Metastable state is 105 times greater than the lifetime of chromium ions in a higher state. Due to the continuous working of the flash lamp, the chromium ions are excited to a higher state E3 and returned to the E2 level. After a few milliseconds, the level E2 is more populated than the level E1 and hence the desired population inversion is achieved. The state of population inversion is not a stable one. The process of spontaneous transition is very high. When the excited chromium ion passes spontaneously from E3 to E2it emits one photon of wavelength 6943 Å. The photon reflects back and forth by the silver ends and until it stimulates an excited chromium ion in the E2 state and it to emit a fresh photon in phase with the earlier photon. The process is repeated again and again until the laser beam intensity is reached a sufficient value. When the photon beam becomes sufficiently intense, it emerges through the partially silvered end of the rod. The wavelength 6943 Å is in the red region of the visible spectrum.

Drawbacks of the ruby laser

The laser requires high pumping power

The efficiency of a ruby laser is very small

It is a pulse laser

Application of ruby laser

Ruby lasers are in optical photography

Ruby lasers can be used for the measurement of plasma properties such as electron density and temperature.

Ruby lasers are used to remove the melanin from the skin.

Ruby laser can be used for the recording of holograms.

Q11) Discuss the construction, working, and application of CO2 Laser?A11)PRINCIPLE: The active medium is a gas mixture of CO2, N2 and He. The laser transition takes place between the vibrational states of CO2 molecules.CONSTRUCTION:It consists of a quartz tube 5 m long and 2.5 cm in the diameter. This discharge tube is filled with gaseous mixture of CO2 (active medium), helium and nitrogen with suitable partial pressures.

Figure 11: Construction of CO2The terminals of the discharge tubes are connected to a D.C power supply. The ends of the discharge tube are fitted with NaCl Brewster windows so that the laser light generated will be polarized.WORKING:

Figure 12: energy levels of nitrogen and carbon dioxide molecules

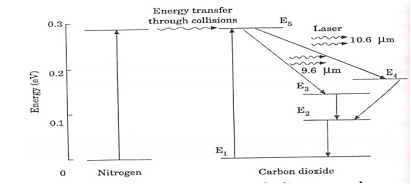
When an electric discharge occurs in the gas, the electrons collide with nitrogen molecules and they are raised to excited states. This process is represented by the equation

N2 + e* = N2* + e

N2 = Nitrogen molecule in ground state

e* = electron with kinetic energy

N2* = nitrogen molecule in excited state

e= same electron with lesser energy

Now N2 molecules in the excited state collide with CO2 atoms in ground state and excite to higher electronic, vibrational and rotational levels.

This process is represented by the equation

N2* + CO2 = CO2* + N2

N2* = Nitrogen molecule in excited state.

CO2 = Carbon dioxide atoms in ground state

CO2* = Carbon dioxide atoms in excited state

N2 = Nitrogen molecule in ground state.

Since the excited level of nitrogen is very close to the E5 level of CO2 atom, population in E5 level increases.

As soon as population inversion is reached, any of the spontaneously emitted photon will trigger laser action in the tube. There are two types of laser transition possible. 1. Transition E5 to E4 This will produce a laser beam of wavelength 10.6μm2. Transition E5 to E3This transition will produce a laser beam of wavelength 9.6μm. Normally 10.6μm transition is more intense than 9.6μm transition. The power output from this laser is 10kW. Characteristics: 1. Type: It is a molecular gas laser. 2. Active medium: A mixture of CO2 , N2 and helium or water vapour is used as active medium3. Pumping method: Electrical discharge method is used for Pumping action 4. Optical resonator: Two concave mirrors form a resonant cavity 5. Power output: The power output from this laser is about 10kW. 6. Nature of output: The nature of output may be continuous wave or pulsed wave. 7. Wavelength of output: The wavelength of output is 0.6μm and 10.6μm.ADVANTAGES: 1. The construction of CO2 laser is simple 2. The output of this laser is continuous. 3. It has high efficiency 4. It has very high output power. 5. The output power can be increased by extending the length of the gas tube.DISADVANTAGES: 1. The contamination of oxygen by carbon monoxide will have some effect on laser action 2. The operating temperature plays an important role in determining the output power of laser. 3. The corrosion may occur at the reflecting plates. 4. Accidental exposure may damage our eyes, since it is invisible (infra-red region) to our eyes.APPLICATIONS: 1. High power CO2 laser finds applications in material processing, welding, drilling, cutting soldering etc.2. The low atmospheric attenuation (10.6μm makes CO2 laser suitable for open air communication.3. It is used for remote sensing 4. It is used for treatment of liver and lung diseases. 5. It is mostly used in neuro surgery and general surgery. 6. It is used to perform microsurgery and bloodless operations. Q12) Discuss Symmetric stretching mode, Bending mode and Asymmetric stretching mode?A12)In a molecular gas laser, laser action is achieved by transitions between vibrational and rotational levels of molecules. Its construction is simple and the output of this laser is continuous. In CO2 molecular gas laser, transition takes place between the vibrational states of Carbon dioxide molecules. CO2 Molecular gas laser It was the first molecular gas laser developed by Indian born American scientist Prof.C.K.N.Pillai. It is a four level laser and it operates at 10.6 μm in the far IR region. It is a very efficient laser. Energy states of CO2 molecules.A carbon dioxide molecule has a carbon atom at the center with two oxygen atoms attached, one at both sides. Such a molecule exhibits three independent modes of vibrations. They are a) Symmetric stretching mode. b) Bending mode c) Asymmetric stretching mode.a. Symmetric stretching modeIn this mode of vibration, carbon atoms are at rest and both oxygen atoms vibrate simultaneously along the axis of the molecule departing or approaching the fixed carbon atoms.

Figure 8: Symmetric stretching mode b. Bending mode:In this mode of vibration, oxygen atoms and carbon atoms vibrate perpendicular to molecular axis.

Figure 9: Bending mode c. Asymmetric stretching mode:

Figure 10: Asymmetric stretching modeIn this mode of vibration, oxygen atoms and carbon atoms vibrate asymmetrically, i.e., oxygen atoms move in one direction while carbon atoms in the other direction.

Sign Up