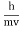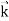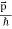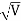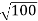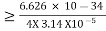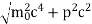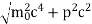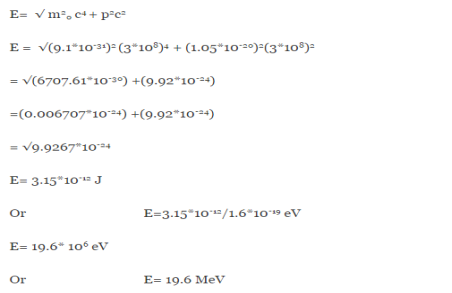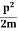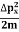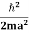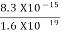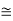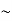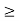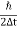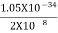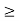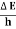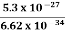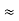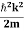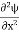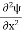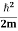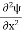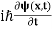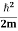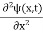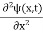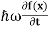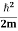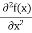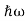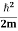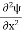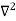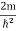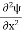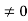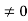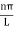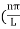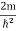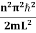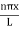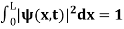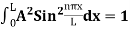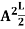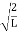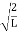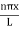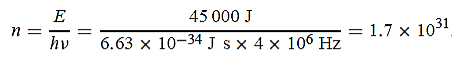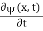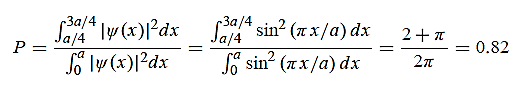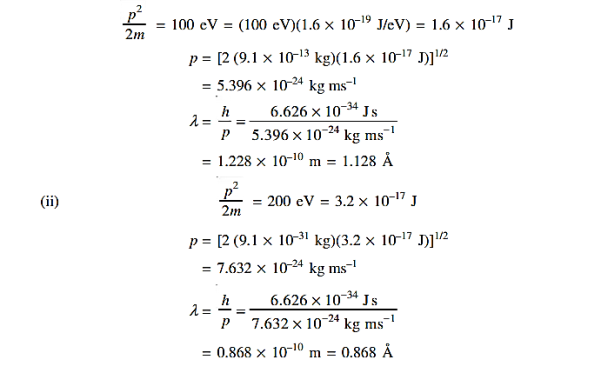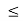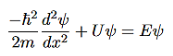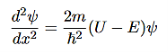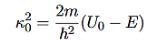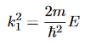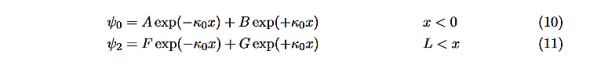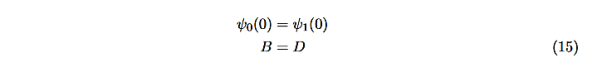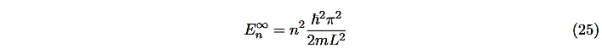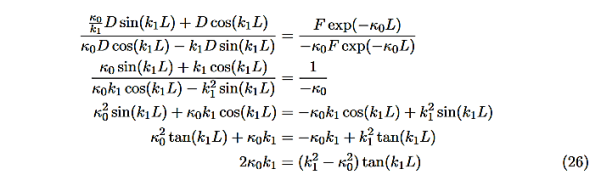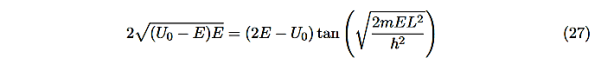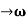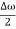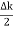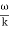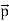 behaves as a group of waves (matter waves) whose wavelength λ and wave vector
behaves as a group of waves (matter waves) whose wavelength λ and wave vector 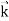 are governed by the speed and mass of the particle. De Broglie proposed that the wave length λ associated with a particle of momentum p is given as where m is the mass of the particle and v its speed.
are governed by the speed and mass of the particle. De Broglie proposed that the wave length λ associated with a particle of momentum p is given as where m is the mass of the particle and v its speed.λ = |
 =
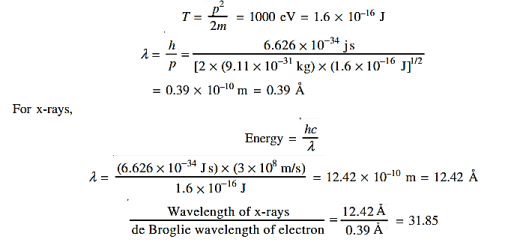
=  = λ Q2) What is the de Broglie wavelength associated with (a) an electron moving with a speed of 5.4×106 m/s, and (b) a ball of mass 150 g travelling at 30.0 m/s?A2)
= λ Q2) What is the de Broglie wavelength associated with (a) an electron moving with a speed of 5.4×106 m/s, and (b) a ball of mass 150 g travelling at 30.0 m/s?A2) (a)For the electron: Mass m = 9.11×10–31 kg, speed v = 5.4×106 m/s. Then, momentum p = m v = 9.11×10–31 kg × 5.4 × 106 (m/s) p = 4.92 × 10–24 kg m/s de Broglie wavelength, λ = h/p = 6.63 x 10-34Js/ 4.92 × 10–24 kg m/s λ= 0.135 nm (b)For the ball: Mass m’ = 0.150 kg, Speed v ’= 30.0 m/s. Then momentum p’ = m’ v’= 0.150 (kg) × 30.0 (m/s) p’= 4.50 kg m/s de Broglie wavelength λ’ = h/p’ =6.63 x 10-34Js/ 4.50 kg m/s =1.47 ×10–34 m The de Broglie wavelength of electron is comparable with X-ray wavelengths. However, for the ball it is about 10–19 times the size of the proton, quite beyond experimental measurement. |
Accelerating potential V = 100 V. The de Broglie wavelength λ is λ= h /p = 1 227/ λ.1 227/ The de Broglie wavelength associated with an electron in this case is of the order of x ray wavelengths. |
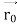 , velocity
, velocity 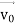 , and all the forces acting on the particle are known, the position
, and all the forces acting on the particle are known, the position 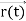 , and velocity
, and velocity 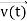 are uniquely determined by means of Newton’s second law. So by Classical physics it can be easily derived.Does this hold for the microphysical world? Since a particle is represented within the context of quantum mechanics by means of a wave function corresponding to the particle’s wave, and since wave functions cannot be localized, then a microscopic particle is somewhat spread over space and, unlike classical particles, cannot be localized in space. In addition, we have seen in the double-slit experiment that it is impossible to determine the slit that the electron went through without disturbing it. The classical concepts of exact position, exact momentum, and unique path of a particle therefore make no sense at the microscopic scale. This is the essence of Heisenberg’s uncertainty principle.In its original form, Heisenberg’s uncertainty principle states that: If the x-component of the momentum of a particle is measured with an uncertainty ∆px, then its x-position cannot, at the same time, be measured more accurately than ∆x = ℏ/(2∆px). The three-dimensional form of the uncertainty relations for position and momentum can be written as follows:
are uniquely determined by means of Newton’s second law. So by Classical physics it can be easily derived.Does this hold for the microphysical world? Since a particle is represented within the context of quantum mechanics by means of a wave function corresponding to the particle’s wave, and since wave functions cannot be localized, then a microscopic particle is somewhat spread over space and, unlike classical particles, cannot be localized in space. In addition, we have seen in the double-slit experiment that it is impossible to determine the slit that the electron went through without disturbing it. The classical concepts of exact position, exact momentum, and unique path of a particle therefore make no sense at the microscopic scale. This is the essence of Heisenberg’s uncertainty principle.In its original form, Heisenberg’s uncertainty principle states that: If the x-component of the momentum of a particle is measured with an uncertainty ∆px, then its x-position cannot, at the same time, be measured more accurately than ∆x = ℏ/(2∆px). The three-dimensional form of the uncertainty relations for position and momentum can be written as follows:
|
Given m = 0.5kg, h = 6.626 × 10-34 m2 kg / s Δp =p×1×10−6 As we know that, Δp = 10-5 Heisenberg Uncertainty principle formula is given as, ∆x∆p ∆x ∆x ∆x =0.527 x 10-29 m |
∆x ∆p =h/2π Thus ∆p=h/2π∆x Or ∆p=6.62 x10-34/2 x 3.14 x 10-14 Or ∆p=1.05 x 10-20 kg m/ sec |
E =
|
∆x ∆p =h/2π Thus ∆p=h/2π∆x Or ∆p=6.62 x10-34/2 x 3.14 x 10-14 Or ∆p=1.05 x 10-20 kg m/ sec If this is p the uncertainty in the momentum of electron then the momentum of electron should be at least of this order that is p=1.05*10-20 kg m/sec. An electron having this much high momentum must have a velocity comparable to the velocity of light. Thus, its energy should be calculated by the following relativistic formula E =
|
∆x = a/2 (half the diameter). According to uncertainty principle ∆x∆px = ℏ/2 or Thus ∆px= ℏ /2∆x ∆px= ℏ /2a/2 = ℏ /a Uncertainty in momentum of particle along x-axis is ∆px= ℏ /a Assuming the momentum of particle to be at least equal to uncertainty in it, the lowest possible value of K.E. of particle is given by K.E. = |
E =
|
∆ x ∆ px =
Similarly ∆ y ∆ py =
And ∆ z ∆ pz =
Multiplying these three equations, we get ∆ x ∆ y ∆ z ∆ px ∆ py ∆ pz = ( The above product is called the volume of elementary cell in phase space. So, volume of an elementary cell in phase space |
∆ E Or ∆ E Or ∆ E Frequency of light is uncertain by ∆ ν = |
E= K+V = By de Broglie’s relation we know that all particles can be represented as waves with frequency ω and wave number k, and that E= ℏω and p= ℏk. Using this equation (1) for the energy will become ℏω = A wave with frequency ω and wave number k can be written as usual as ψ(x, t) =Aei(kx−ωt) ……….. (3) the above equation is for one dimensional and for three dimensional we can write it as ψ(r, t) =Aei(k·r−ωt) ……….. (4) But here we will stick to one dimension only.
If we multiply the energy equation in Eq. (2) by ψ, and using(5) and (6) , we obtain ℏ(ωψ) = This is the time-dependent Schrodinger equation. If we put the x and t in above equation then equation (7) takes the form as given below
In 3-D, the x dependence turns into dependence on all three coordinates (x, y, z) and the The term |ψ(x)|2 gives the probability of finding the particle at position x. Let us again take it as simply a mathematical equation, then it’s just another wave equation. However We already know the solution as we used this function ψ(x, t) =Aei(kx−ωt) to produce Equations (5), (6) and (7) But let’s pretend that we don’t know this, and let’s solve the Schrodinger equation as if we were given to us. As always, we will guess an exponential solution by looking at exponential behaviour in the time coordinate, our guess is ψ(x, t) =e−iωtf(x) putting this into Equation (7) and cancelling the e−iωt yields We already know that E= Eψ = -
|
Given m= 9×10–31 ΔV= 5.7×105 m/sec Δx=? h= 6.6×10–34 Joule-Sec. Δx.Δv ≥h/4πm Δx≥h/4πmΔv ≥6.6×10–34/9×3.14×9.1×10–31×5.7×105 ≥ 0.010×10–8 ≥ 1×10–10m |
Given m=0.15 kg. h=6.6×10-34 Joule-Sec. Δx = 10 –10 m Δv=? Δx.Δv ≥h/4πm Δv≥h/4πmΔx ≥6.6×10-34/4×3.14×0.15×10–10 ≥ 3.50×10–24m |
m=10 gm. h=6.6×10–27 erg-sec Δv= 5.25×10–26 cm Δx=? Δx.Δv ≥h/4πm Δx≥h/4πmΔv ≥6.6×10-34/4×3.14×10×5.25×10–26 ≥ 0.10×10–2m ≥ 1×10–3 cm |
|
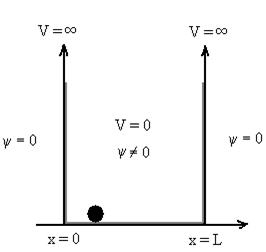
 1031, the quantum nature of this radiation is unimportant. As a result, this radiation can be treated fairly accurately by the classical theory of electromagnetism. Q14) Consider a one-dimensional particle which is confined within the region 0
1031, the quantum nature of this radiation is unimportant. As a result, this radiation can be treated fairly accurately by the classical theory of electromagnetism. Q14) Consider a one-dimensional particle which is confined within the region 0  x
x a and whose wave function is (x, t) =sin(πx/a) exp-iωt.(a) Find the potential V(x).(b) Calculate the probability of finding the particle in the interval a/4
a and whose wave function is (x, t) =sin(πx/a) exp-iωt.(a) Find the potential V(x).(b) Calculate the probability of finding the particle in the interval a/4  x
x  3a/4.A14) Since the first time derivative and the second x derivative of (x, t), are given by
3a/4.A14) Since the first time derivative and the second x derivative of (x, t), are given by
Hence V(x,t) is time independent and given by
(b) The probability of finding the particle in the interval a/4
|
|
|
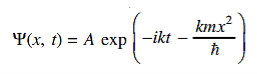 where A and k are constants. Find the explicit form of the potential V (x).A17)
where A and k are constants. Find the explicit form of the potential V (x).A17)
Substituting these values in the time dependendent Schrödinger equation, we have
|
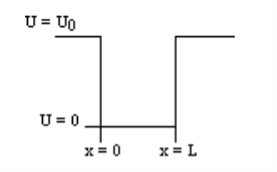
U=U0 x <0 (1) U= 0 0 U=U0 L < x. (3) |
Figure 8: Finite Square Well Potential Energy The time-invariant, non-relativistic Schroedinger’s equation is
that can be rearranged to give
It is convenient to define two new variables (both positive), one for regions 0 and 2, and one for region 1—they are wavenumbers:
and Schrödinger’s equation becomes
In regions 0 and 2 the general solution is a linear combination of exponentials with the same form, but with different constants, namely
In region 1 we have the same general solution that we had for the infinite square well,
Equations (10) to (12) have 7 unknowns—A,B,C,D,F,G and the energy E that is im-plicitly contained in the variables κ0,k1. Therefore we need to get 7 equations to be ableto solve for the unknowns. We will first use the requirement that the wavefunction remain finite everywhere. Consider ψ2 as x→∞. For this to remain finite we must require G= 0. Similarly, as x→−∞, we require A= 0. Our solutions become
The next step is to require that the wavefunction and its first derivative be continuous everywhere, and in our case we look at the boundaries, x= 0 and x=L.
Hence ψ0=D exp(+κ0x).Take derivatives of the wave functions,
There remain 3 unknowns, D,F, and E. Finding them is a bit messier! Consider the boundary conditions at x=L,
We are not going much farther, but if we divided Equation (22) by Equation (23), we can see that the constants D and F cancel leaving us with one rather difficult equation to solve for energy E (remember this is implicitly included in the values of k1and κ0.) There is one remaining condition, normalization, that for this problem is
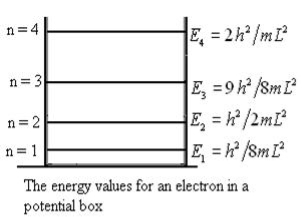
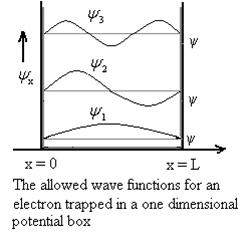
Even without solving the entire problem we can make some conclusions about the wave-function and the allowed energy levels. Recall that for an infinite square well potential of width L the allowed energies are quantized and
Here is Equation(22)/Equation(23)
Now put in the values ofκ0andk1from Equations (6) and (7) and do some algebra to get
This equation is a single equation in a single unknown, E < U0. Once we have the details of our particle (its mass) and the potential energy (depth U0 and width L), we can solve it. There is no analytic solution, only a numerical one. From equation (25) With n being any positive integer. Outside the well the wavefunction is 0. We are certain that the particle is somewhere inside the box, so ∆x ∞ =L. With the finite well, the wavefunction is not zero outside the well,
Figure 9: Wave function in Finite well So ∆x finite> L, hence from the uncertainty principle, ∆pfinite x<∆p∞x. This suggests that the average value of momentum is less for the finite well, and therefore that the kinetic energy inside the well is less for the finite well than for the infinite well. Indeed this is borne out with detailed analysis. In addition, the number of allowed energy levels is finite, and there is a possibility that a well may be sufficiently narrow or sufficiently shallow that no energy levels are allowed. Also note that the non-zero wave functions in regions 0 and 2 mean that there is a non-zero probability of finding the particle in a region that is classically forbidden, a region where the total energy is less than the potential energy so that the kinetic energy is negative. |
Such a wave packet moves with its own velocity vg called the group velocity.The individual wave forming the wave packet possesses an average velocity vp called the phase velocity.
|
u (x, t) =A sin(ωt - kx) …….(1) u’(x, t) =A sin[(ω+∆ω)t-(k+∆k)x] …….(2) Where ω and (ω+Δω) are angular frequencies and k and (k +Δk) are propagation constants The superposition of two waves is of the form ψ(x,t) = u +u’ = A sin(ωt - kx)sin[(ω+∆ω)t - (k+∆k)x] …….(3) As Δ ω and Δk are small therefore (ω+Δω) ψ(x,t) = 2Acos[ This equation represents a vibration of amplitude 2Acos[ The phase of the resultant wave moves with the velocity known as phase velocity vp= And the amplitude moves with the velocity known as group velocity vg = vg =
|