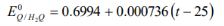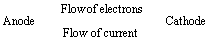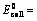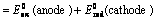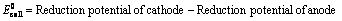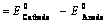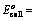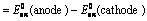undefined | unit 1 electrochemistry and battery chemistry

CHEM

UNIT-1Electrochemistry and Battery Chemistry Q1) What is an Electrochemical cell?A1) Electrochemical cells are that can generate electrical energy, from the chemical reactions that occur in them, or it can use the electrical energy given from an externa source to assist a chemical reaction. Hence, they are able to convert electrical energy into chemical energy or vice versa. A common example of an electrochemical cell is a standard 1.5-volt cell which is used to power many electrical appliances such as TV remotes and clocks.Therefore, cells that are able to generate electric current from chemical reactions occurring in them are called voltaic cells or Galvanic cells, on the other hand Electrolytic cells are those cells that generate chemical reaction when electric current is passed through them.

Fig 1 : Electrochemical cell Q2) Define cell reaction and cell potential? A2) Cell reaction and cell potentialCell reaction is best explained with an example

The Cu2+/Cu redox couple (corresponding to the reaction Cu2+(aq) + 2e– → Cu (s) ) is represented by

Cu (s) | Cu2+(aq)

The Fe3+/Fe2+ redox couple (corresponding to the reaction Fe3+(aq) + e– → Fe2+(aq) ) is represented:

Pt | Fe2+(aq) , Fe3+(aq)

In an Electrochemical, they are combined with the copper system as the left-hand electrode, the overall representation is:

Cu (s) | Cu2+(aq) || Fe3+(aq) , Fe2+(aq) | Pt

The cell reaction can be concluded as the overall reaction that takes place in the cell, assuming that the right-hand Electrode is the Cathode, with the assumption that the spontaneous reaction, that includes reduction occurs in the right hand.(In simple terms the Standard potentials of the two electrodes predict if the cathode is in the right side if the reaction is spontaneous, or if cathode is on the left side them the reverse of the cell reaction is spontaneous. The cell reaction for any reaction cell diagram is that the right-hand half of the reaction is a reduction reaction and the left-hand half reaction is oxidation. (This is exactly the same as subtracting the left-hand equation written as a reduction, which is the formally correct procedure.)Thus, the cell reaction for the Cu2+/Cu and Fe3+/Fe2+ cell described above is as follows:

2Fe3+(aq) + Cu (s) → 2Fe2+(aq) + Cu2+(aq)

An important thing to be noted is that without having a knowledge of the two electrodes Standard potential, it’s difficult to figure out if the reaction is spontaneous or notThe driving force of the electron flow from anode to cathode shows a potential drop in the energy of the electrons moving into the wire. The difference in potential energy between the anode and cathode is known as the cell potential in a voltaic cell.The standard cell potential (EocellEOcell) is the difference of the two electrodes, which forms the voltage of that cell. To find the difference of the two half cells, the following equation is used:

EoCell=EoRed,Cathode−EoRed,Anode

with

EoCellECello is the standard cell potential (under 1M, 1 Barr and 298 K).

EoRed,CathodeEORed,Cathode is the standard reduction potential for the reduction half reaction occurring at the cathode

EoRed,AnodeEORed,Anode is the standard reduction potential for the oxidation half reaction occurring at the anode

The units of the potentials are typically measured in volts (V). Note that this equation can also be written as a sum rather than a difference
Q3)Explain Calomel Quinhydrone Electrode? A3) Calomel electrodes is used in voltmeter and also pH meters, it’s a kind of reference electrode, and is based on reactions between mercury(I) chloride (calomel) and elemental mercury.A calomel electrolyte should be a non-polarizable one and robust, they are commonly used two-electrode systems, where the supporting electrolyte is a non-reactive chloride salt. The structure of the electrode includes of an outer glass with a frit at the bottom, which allows electric contact with a solution outside the electrode. An inner tube is present inside an outer tube. The bottom of the inner tube has glass wool to enable electric contact between the contents of both tubes.On the innermost tube, is the Mercury paste, with mercurous chloride being dispersed in a saturated potassium chloride solution.

Fig 2: calomel electrode.It’s a type of Half-cell, where the electrode is mercury that is coated with calomel (Hg2Cl2) and a solution of potassium chloride and saturated calomel forms the electrolyte. This can be represented as:Hg|Hg2Cl2KCl (xM) saturatedThe electrode reaction is:Hg2Cl2 + 2e Hg2Cl2 == 2Hg + 2Cl Q4)Derive the Nernst Equation?A4) Nernst EquationAny change in the Gibbs free energy G directly correspond to changes in free energy for processes at constant temperature and pressure, change is the maximum non-expansion work obtainable under these conditions in a closed system; ΔG is negative for spontaneous process, positive for nonspontaneous process, and zero for processes at equilibrium.It takes into consideration the values of the standard electrode potentials, temperature, activity and the reaction quotient for the calculation of cell potential. For any cell reaction, that occurs Gibbs free energy can be related to standard electrode potential as:

ΔG =-nFE

Where, n = number of electrons transferred in the reaction, ΔG= Gibbs free energy, E= cell potential F = Faradays constant (96,500 C/mol) and. Under standard conditions, the above equation can be written as,

ΔGo =-nFEo

According to the theory of thermodynamics, Gibbs free energy under general conditions can be related to Gibbs free energy under standard condition and the reaction quotient as:

ΔG=ΔGo + RT lnQ

Where, Q= reaction quotient, R= universal gas constant and T= temperature in Kelvin. Incorporating the value of ΔGo and ΔG, from the first two equations, we get the equation:

-nFE = -nFE0 + RT lnQ

E = E0 – (RT/nF) lnQ

By conversion of Natural log to log10, the above equation is called as the Nernst equation. Here, it shows the relation of the reaction quotient and the cell potential. Special cases of Nernst equation:

E = Eo − (2.303RT/nF) log10Q

At standard temperature, T= 298K:

E = Eo − (0.0592V/n) log10Q

At standard temperature T = 298 K, the 2.303RTF, term equals 0.0592 V.

Under Equilibrium ConditionAs the redox reaction in the cell progresses, the concentration of reactants decreases while the concentration of products increases. This process goes on until equilibrium is achieved. At equilibrium, ΔG = 0. Hence, cell potential, E = 0. Thus, the Nernst equation can be modified to:

E0 – (2.303RT/nF) log10Keq = 0

E0 = (2.303RT/nF) log10Keq

Where, Keq = equilibrium constant and F= faradays constant. Therefore, the above equation gives us a relation between standard electrode potential of the cell where the reaction takes place and the equilibrium constant.Q5) Explain the EMF of a cell?A5)Electromotive force, or, as it is often written, e.m.f., is described as that source of energy which enables electrons movement around an electric circuit.For any object to move from rest, there has to be some energy change. To ensure electrons movement round an electrical circuit, they should receive energy from a source of e.m.f. which usually is a battery or a generator.For every coulomb of electricity to move completely around an electrical circuit, a certain amount of electrical energy is needed, which depends on the particular circuit. The e.m.f. is expressed in volts and is numerically the number of joules of energy given by the source of e.m.f. to each coulomb to enable movement around the circuit. The symbol for volt is the capital letter V.ThusJoules colombs=volts.It follows that:joules=volts × coulombs = volts × ampers × seconds.A 12-volt (12-V) battery is able to give 12 joules (12 J) of energy to each coulomb to enable movement around an electrical circuit.The symbol for e.m.f. is the capital letter E. Example:Calculate the energy supplied by a 12v battery when a current of 4 A flows for 10 minutes.Energy supplied = Volts x amperes x seconds = 12 x 4 x (10 x 60) Joules = 28,800 J

Cell Potential

(1) “The difference in potentials of the two half – cells of a cell known as electromotive force (emf) of the cell or cell potential.”The potential difference of the two half – cells of a cell arises because of the flow of electrons from anode to cathode and flow of current from cathode to anode.

(2) The emf of the cell or cell potential can be calculated from the values of electrode potentials of two half – cells constituting the cell. The following three methods are in use (i) When oxidation potential of anode and reduction potential of cathode are taken into consideration

Oxidation potential of anode + Reduction potential of cathode

(ii) When reduction potentials of both electrodes are taken into consideration

(iii) When oxidation potentials of both electrodes are taken into consideration

Oxidation potential of anode – Oxidation potential of cathode

Q6) Differentiate between the primary and secondary Batteries?A6)Primary BatteriesZn-Carbon BatteriesThe battery is mainly a dry cell primary battery, that enables direct electric current from electrochemical reactions that occur between zinc and manganese dioxide. The cell shows a voltage of 1.5 volts between the zinc anode, that is known to be the container for the battery, and a rod made of carbon that has positive polarity, namely the cathode, that collects the current from the manganese dioxide, hence its name. The General-purpose batteries use electrolyte as an aqueous paste of ammonium chloride that may be mixed with zinc chloride solution. The heavy-duty batteries use a paste primarily of zinc chloride.Zinc–carbon batteries were the first commercial dry batteries, developed from the technology of the wet Leclanche cell. Since these batteries worked in orientation, they were used in making flashlights and other portable devices. They are still used in remote controls clocks flashlights, transistor radios, Zinc–carbon dry cells are single-use primary cells.Secondary BatteriesPb-acid Batteries The battery converts chemical energy into electrical energy, as it uses sponge lead and lead peroxide for the conversion. such type of battery is called a lead acid battery. They are seen commonly in power stations and substations due to its high cell voltage and low costThe lead-acid battery is commonly used as storage batteries in the field of automobiles. The battery is made up of several cells, each of which consists of lead plates immersed in an electrolyte of dilute sulfuric acid. The voltage per cell is typically 2 V to 2.2 V. For a 6 V battery, three cells are connected in series, and for a 12 V battery, six cells are connected in a series.Li-ion Batteries A lithium-ion battery or Li-ion battery, are batteries that are rechargeable. They are popular in aerospace and in military, they are also commonly used in portable electronics and electric vehicles.In the batteries, the lithium ions, during discharge move from the negative electrode to the positive electrode through an electrode. And back while charging. At the positive electrode the intercalated lithium compound is present, and graphite at the negative electrode, the battery shows high energy density, low discharge and no memory loss. They can however be a safety hazard since they contain flammable electrolytes, and if damaged or incorrectly charged can lead to explosions and fires. Q7) Define Cell notations?A7)It is the symbolic representation of the two halves of the galvanic cells by using abbreviations and symbols of the elements. Guidelines of cell notation are as follow:

The two halves are represented by using symbols of the elements and chemical formulas of the compounds.

The anode half is written first and the cathode half is written later. First, the reactants are mentioned within each half and then the products are mentioned.

Reactions of both the halves are separated by using two vertical parallel lines in between. This double vertical line indicates the salt bridge of the galvanic cell.

The phased of each of the element and compound is mentioned in parentheses as s, g and aq for solid, gas and aqueous respectively.

The cell notation of the above-mentioned galvanic cell is:Cu(s)│1MCu(NO3)2(aq)║1MAgNO3(aq)│Ag(s)Q8) What are flow batteries?A8) cell consists of anodic and cathodic compartments. Both the compartments contain platinum electrode. At the Anode compartment, Methanol containing H2SO4 is passed. Oxygen is passed through cathodic compartment. Electrolyte consists of sulphuric acid. A membrane is provided which prevents the diffusion of Methanol into the cathode.

Fig 3: Fuel cellReactions:At anode: CH3OH + H2O → CO2 + 6H+ + 6e-At cathode: 3/2 O2 + 6H+ + 6e- → 3H2OAdvantages:Methanol shows low carbon contentThe OH group in methanol is easily oxidisableMethanol is highly soluble in water.Uses: in military applications. Q9) Differentiate between Anode and Cathode?A9)Electrochemical cells generally consist of a cathode and an anode. The key features of the cathode and the anode are tabulated below.

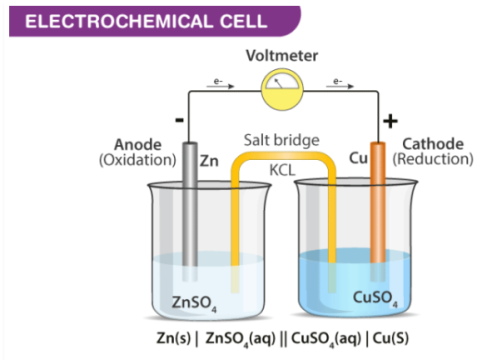
Cathode	Anode
Cathode has a positive sign, as electrons are consumed	Anode have a negative sign as electrons are liberated here
In an electrochemical cell, at the cathode a reduction reaction occurs.	At the Anode an oxidation reaction occurs
Electrons move into the cathode	Electrons move out of the anode

Q10) Determine the pH a solution using Quinhydrone electrode?A10)Determination of pH using the Quinhydrone Electrode The quinhydrone electrode shows a redox type of a reaction, and is used to determine the hydrogen ion concentration of solutions in chemical experiments. They form the alternative for glass electrodes. Redox reaction, involves the transfer of electrons of from one species to another, the transfer may also include the transfer of other atoms or ions. Eventually a redox reaction involves the difference of two half reactions that are conceptual reactions showing gain of electrons. The redox electrode consists of an inert material like platinum or gold dipped in a solution containing a chemical species in two different oxidation states., the transfer of electrons between these two species takes place through inert material. The quinhydrone electrode cannot be used in solutions that would react with quinone or hydroquinone as they are weak acids, the electrode cannot be used above pH = 8.5 when the dissociation of hydroquinone becomes appreciable. Another drawback is that quinone is oxidized by air in strongly alkaline medium. In spite of all this, the quinhydrone electrode is frequently used.

The electrodes are taken off from the storage solution

The Electrodes are rinsed with distilled water

The beakers are filled with measured amount of solution

The approximate pH values are written on the flask.

Note down the temperature of solution (t)

A bit of quinhydrone is taken using wood spatula, and added to the solution and carefully mixed using a glass stick, to obtain a saturated solution with quinhydrone.

The electrodes are immersed in the solutions of the beaker

Th quinhydrone forms the positive electrode and the calomel electrode the negative terminal.

The electromotive force (E in mV) reading is taken from the potentiometer after it shows a stable value.

The E value is noted and the standard potential of Quinhydrone electrode at lab temperature is calculated using the formulae

Sign Up