Que. No | Question Statement | Que. No | Question Statement | |
Law of Parallelogram | ||||
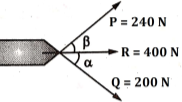
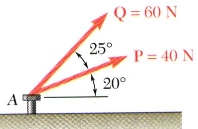
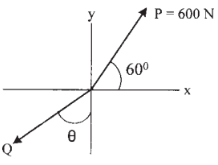
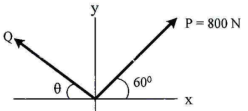
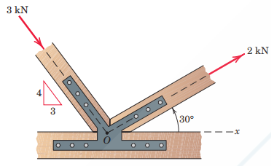
1 |
Ans: θ = 49.46° β = 22.33° α = 27.13°
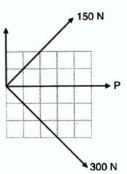
| 2 |
Ans:
| |
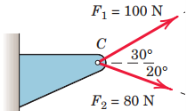
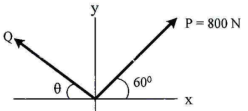
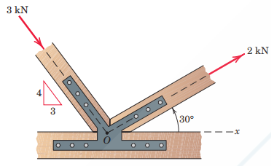
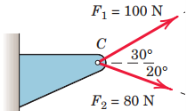
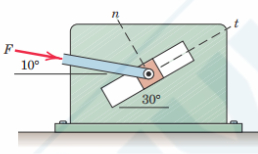
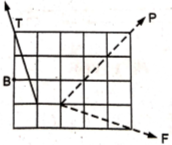
3 | Forces Fl and F2 act on the bracket as shown. Determine the resultant R.
| 4 |
Ans:
| |
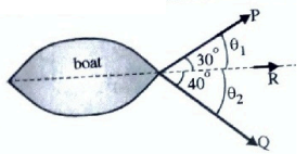
5 | To move the boat uniformly along the river at a given Speed a resultant force R = 520 N is required. Two men are pulling with force P and Q by means of ropes do this. The ropes make an angle of 30° and 40° respectively with the sides of the river as shown in Fig (i ) Determine the force P and Q (ii) if θ1 = 30°, find the value of θ2 such that the force in the rope Q is minimum. What is the minimum force Q. Ans: P = 355.7 N, Q = 276.69 N θ2 = 60°, Qmin = 260 N |
| ||
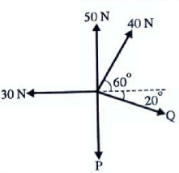
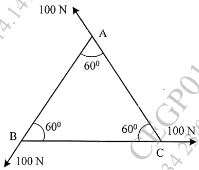
6 | The sum of two concurrent forces P and Q is 270 N and their resultant is of 180 N. If resultant is perpendicular to P. Find P and Q. | 6 | For two forces P and Q acting at a point, maximum resultant is 2000 N and minimum magnitude of resultant is 800 N. Find values of P and Q. | |
7 | The angle between the two concurrent forces is 90° and their resultant is 2500 N. The resultant makes an angle of 46° with one of the force, determine the magnitude of each force. | 8 | The resultant of two forces is 180 N. It makes an angle of 40° with each force. Find magnitude of two forces. | |
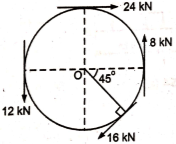
Resolution and composition – Resultant of Concurrent Forces | ||||
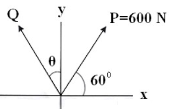
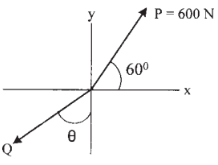
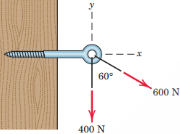
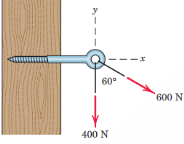
1 | The resultant of two forces P and Q is 1200 N vertical. Determine the force Q and the corresponding angle θ for the system of forces as shown in Figure.
Q = 743.59 N | 2 |
Ans: Q = 1587.45 N θ = 70.89°,
| |
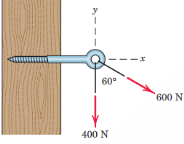
3 | The angle between the two concurrent forces is 90° and their resultant is 2500 N. The resultant makes an angle of 46° with one of the force; determine the magnitude of each force. Ans: P = 1737 N Q = 1798 N | 4 |
Ans: P = -318.2 N = 318.2 N← R = 106.07 N↓
| |
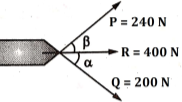
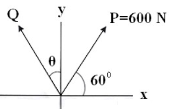
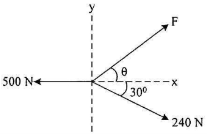
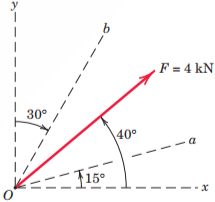
5 |
Ans: α = 40.91° R = 523.94 N
Direction of resultant = 48.58° in 4th Quadrant | 6 | The force system shown in Fig. have a resultant of 200 N along positive Y-axis, determine the magnitude and position θ of force F.
θ = 47.6° R = 433.31 N | |
7 |
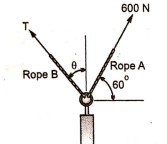
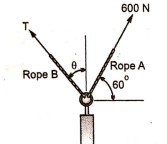
| 8 | The post is to be pulled out of the ground using two ropes A and B. Rope A is subjected to a force of
Ans: θ = 23.79° R = 743.7 N | |
9 |
Ans: | 10 | Determine the resultant R of the two forces shown by summing scalar components.
| |
11 |
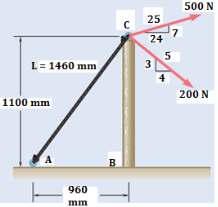
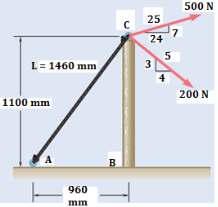
| 12 | Knowing that the tension in rope AC is 365 N, determine the resultant of the three forces exerted at point C of post BC.
| |
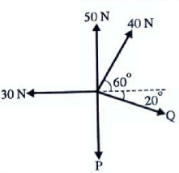
13 | The following forces act at a point: i) 20 N inclined at 30° towards North of East ii) 25 N towards North iii)30 N towards North West iv) 35 N inclined at 40° towards South of West. Find the magnitude and direction of the resultant force. | Ans: | ||
14 |
Ans:
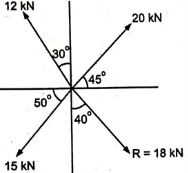
| 15 | R = 18 kN is the resultant of four concurrent forces out of which only three are known. Find the fourth force in magnitude and direction.
Ans: F = 29.83 kN, θ= 64.04° (4th quadrant)
| |
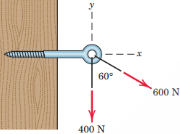
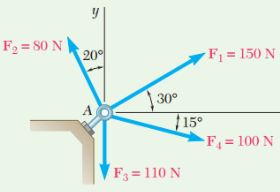
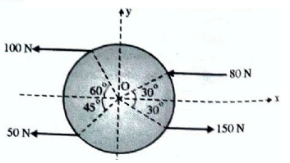
16 |
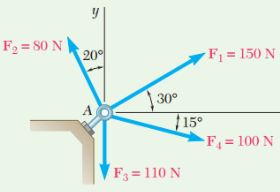
Ans: | 17 | Four forces act on bolt A as shown. Determine the resultant of the forces on the bolt. Ans:
| |
18 |
Ans:
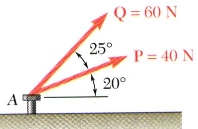
| 19 |
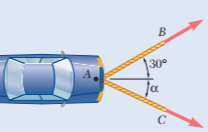
Ans: P = 81 N Q = 10.64 N | |
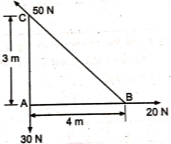
Resultant of Non Concurrent Forces, Moment, Varignon’s Theorem | ||||
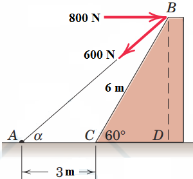
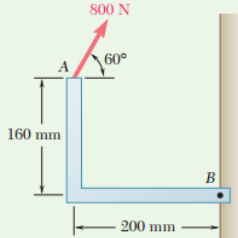
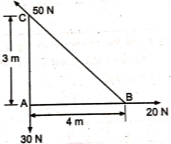
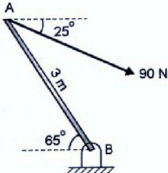
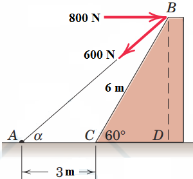
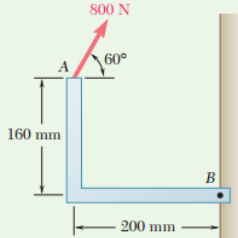
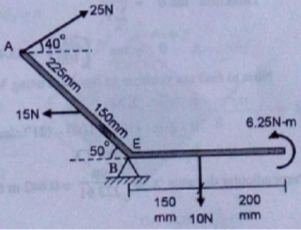
1 | A force of 800 N acts on a bracket as shown. Determine the moment of the force about B.
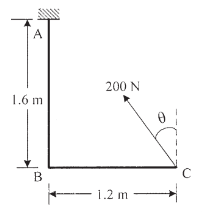
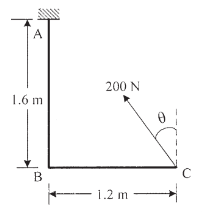
| 2 | The lever ABC fixed at A shown in Figure is subjected to a 200 N force at C at θ = 30°. Find the moment of this force about A. Also find the value of θ for which the moment about A is Zero.
| |
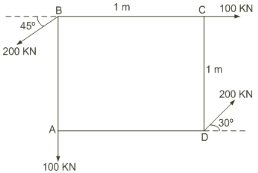
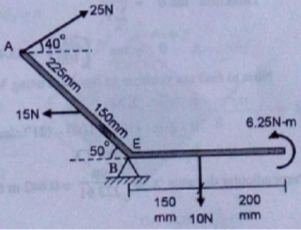
3 | Find resultant moment of two couples for the loading as shown in Fig. [Ans: Resultant moment = 130 N.m (clockwise)]
| 4 |
Ans: R = 19.88 N, α = 50.35° x = 1.51 m from O. | |
5 |
| 6 | If the resultant moment about point A is 4800 Nm clockwise, determine the magnitude of F3 if F1=300 N and F2 = 400 N.
F3 = 1592.7 N
| |
7 |
Ans: R = 20 N ← x = 6 m from B | 8 |
Ans:
| |
9 |
Ans: F = -581.716 N T = -929.282 N | 10 |
Ans: MB=173.53 N.m | |
11 |
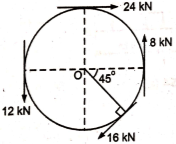
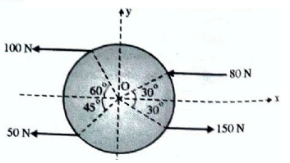
Ans: R = 7.35 N, α = 55.64° x = 394.75 mm | 12 | For the given force system, find the resultant in magnitude and direction. Also locate point of application of resultant w.r.t. ‘O’.
| |
Que. No | Question Statement | Que. No | Question Statement | |
Law of Parallelogram | ||||
1 |
Ans: θ = 49.46° β = 22.33° α = 27.13°
| 2 |
Ans:
| |
3 | Forces Fl and F2 act on the bracket as shown. Determine the resultant R.
| 4 |
Ans:
| |
5 | To move the boat uniformly along the river at a given Speed a resultant force R = 520 N is required. Two men are pulling with force P and Q by means of ropes do this. The ropes make an angle of 30° and 40° respectively with the sides of the river as shown in Fig (i ) Determine the force P and Q (ii) if θ1 = 30°, find the value of θ2 such that the force in the rope Q is minimum. What is the minimum force Q. Ans: P = 355.7 N, Q = 276.69 N θ2 = 60°, Qmin = 260 N |
| ||
6 | The sum of two concurrent forces P and Q is 270 N and their resultant is of 180 N. If resultant is perpendicular to P. Find P and Q. | 6 | For two forces P and Q acting at a point, maximum resultant is 2000 N and minimum magnitude of resultant is 800 N. Find values of P and Q. | |
7 | The angle between the two concurrent forces is 90° and their resultant is 2500 N. The resultant makes an angle of 46° with one of the force, determine the magnitude of each force. | 8 | The resultant of two forces is 180 N. It makes an angle of 40° with each force. Find magnitude of two forces. | |
Resolution and composition – Resultant of Concurrent Forces | ||||
1 | The resultant of two forces P and Q is 1200 N vertical. Determine the force Q and the corresponding angle θ for the system of forces as shown in Figure.
Q = 743.59 N | 2 |
Ans: Q = 1587.45 N θ = 70.89°,
| |
3 | The angle between the two concurrent forces is 90° and their resultant is 2500 N. The resultant makes an angle of 46° with one of the force; determine the magnitude of each force. Ans: P = 1737 N Q = 1798 N | 4 |
Ans: P = -318.2 N = 318.2 N← R = 106.07 N↓
| |
5 |
Ans: α = 40.91° R = 523.94 N
Direction of resultant = 48.58° in 4th Quadrant | 6 | The force system shown in Fig. have a resultant of 200 N along positive Y-axis, determine the magnitude and position θ of force F.
θ = 47.6° R = 433.31 N | |
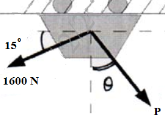
7 |
| 8 | The post is to be pulled out of the ground using two ropes A and B. Rope A is subjected to a force of
Ans: θ = 23.79° R = 743.7 N | |
9 |
Ans: | 10 | Determine the resultant R of the two forces shown by summing scalar components.
| |
11 |
| 12 | Knowing that the tension in rope AC is 365 N, determine the resultant of the three forces exerted at point C of post BC.
| |
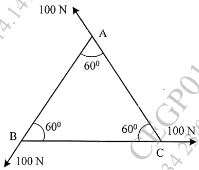
13 | The following forces act at a point: i) 20 N inclined at 30° towards North of East ii) 25 N towards North iii)30 N towards North West iv) 35 N inclined at 40° towards South of West. Find the magnitude and direction of the resultant force. | Ans: | ||
14 |
Ans:
| 15 | R = 18 kN is the resultant of four concurrent forces out of which only three are known. Find the fourth force in magnitude and direction.
Ans: F = 29.83 kN, θ= 64.04° (4th quadrant)
| |
16 |
Ans: | 17 | Four forces act on bolt A as shown. Determine the resultant of the forces on the bolt. Ans:
| |
18 |
Ans:
| 19 |
Ans: P = 81 N Q = 10.64 N | |
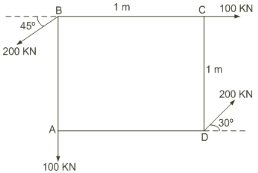
Resultant of Non Concurrent Forces, Moment, Varignon’s Theorem | ||||
1 | A force of 800 N acts on a bracket as shown. Determine the moment of the force about B.
| 2 | The lever ABC fixed at A shown in Figure is subjected to a 200 N force at C at θ = 30°. Find the moment of this force about A. Also find the value of θ for which the moment about A is Zero.
| |
3 | Find resultant moment of two couples for the loading as shown in Fig. [Ans: Resultant moment = 130 N.m (clockwise)]
| 4 |
Ans: R = 19.88 N, α = 50.35° x = 1.51 m from O. | |
5 |
| 6 | If the resultant moment about point A is 4800 Nm clockwise, determine the magnitude of F3 if F1=300 N and F2 = 400 N.
F3 = 1592.7 N
| |
7 |
Ans: R = 20 N ← x = 6 m from B | 8 |
Ans:
| |
9 |
Ans: F = -581.716 N T = -929.282 N | 10 |
Ans: MB=173.53 N.m | |
11 |
Ans: R = 7.35 N, α = 55.64° x = 394.75 mm | 12 | For the given force system, find the resultant in magnitude and direction. Also locate point of application of resultant w.r.t. ‘O’.
| |