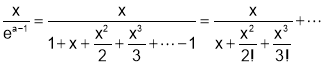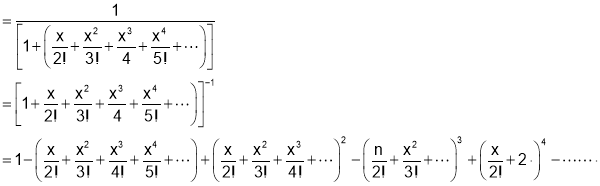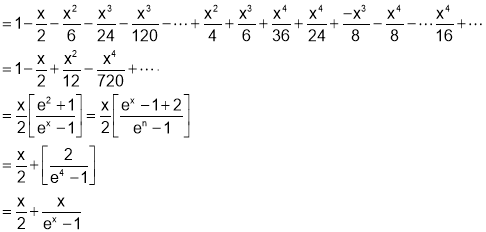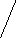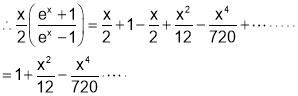Module 2

Limits, homogenous, Maxima Minima

Q1.   If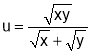 then verify Euler’s theorem for u.

Sol 1.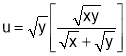               

By symmetry,

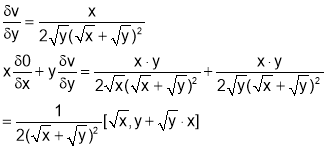
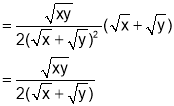
b)    Put x=xt, y=yt we get

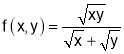
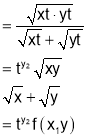
Thus u is homogenous function of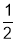

Hence, by Euler’s Theorem

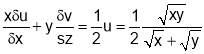
Q2.   If 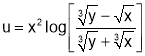prove that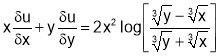

Sol 2.       Putting x=xt, y=yt we get

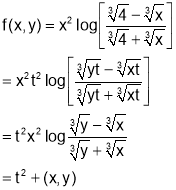
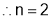
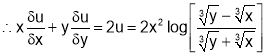
Q3.   If 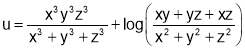find value of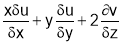

Sol 3.       Let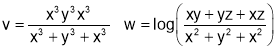

Let u=v+w

Putting x=xt, y=yt, z=zt

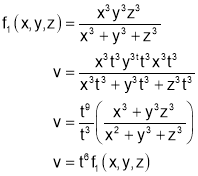
Thus v is homogenous function of degree 6.

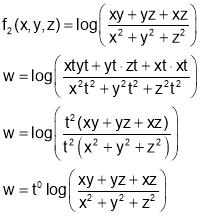
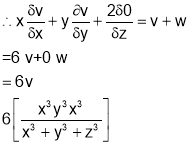
Q4. Find all stationary values of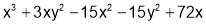

Sol 4: We have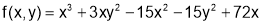

Step I: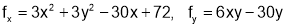

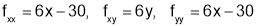
Step II: We now solve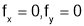

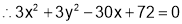  i.e.

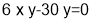
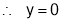And     or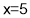

(i)        When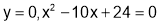

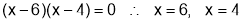
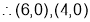are stationary points.

(ii)      When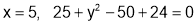

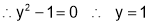or .

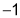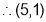and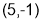 are stationary points.

Step III: (i) When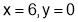

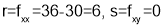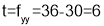
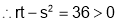and

and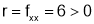

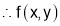is minimum at.

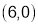The minimum value.

(ii)      When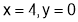

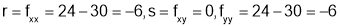
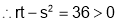and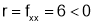

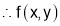is maximum at.

The maximum value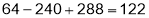

(iii)   When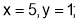 

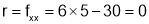
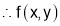is neither maximum nor minimum.

(iv)    When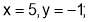  

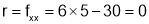
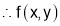is neither maximum nor minimum

Q5. Find the minimum distance from the point 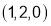to the cone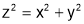

Sol 5: If 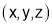  is any point on the cone, its distance from the point 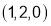is

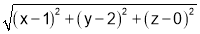
When the distance is minimum, its square is also minimum.

Step I : We have to find the stationary value of

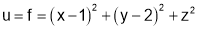                             ………………(1)

With the condition that 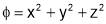                           ………………….(2)

Consider the Lagrange’s function

  gives

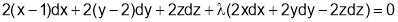
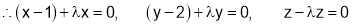     ………………….(3)

Step II : We have to eliminate x, y, z from (1), (2) and (3).

From (3),

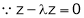
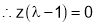
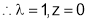
Hence,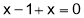     

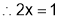
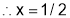
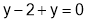
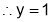
Hence, the required point is .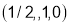

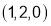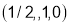The distance between and is

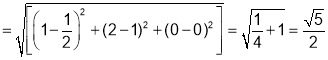
Q6. If 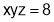, find the values of x, y, z for which 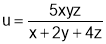is maximum.

Sol6 : We have to maximize 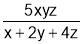with the condition that .

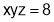This means we have to maximize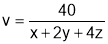

i.e. we have to minimiz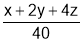ei.e.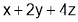

Step I : We have to minimize 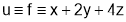 ………………(1)

With the condition that 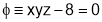   ……………….(2)

Consider the Lagrange’s function,

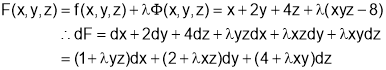
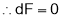gives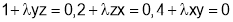

But

and

Q7. Prove that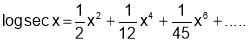

SOL7: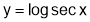   

Diff wrt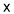

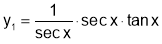
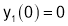
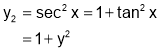
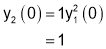
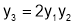
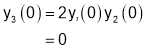
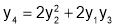
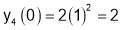
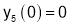
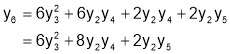
Hence by Maclaurin’s Series

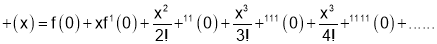we get

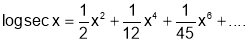
Q8. Expand 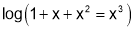upto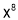

Sol 8:We have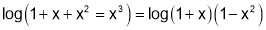

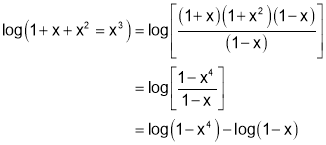
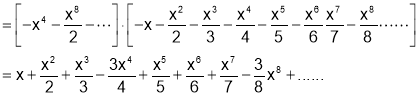
Q9. Prove that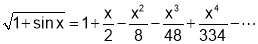

Sol 9. We have

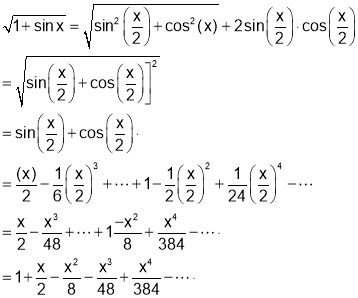
Q10. Expand in Power of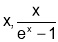

Hence Prove that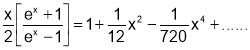

Sol 10.