Unit 3
Maxwell’s equations
Q1) Explain Maxwell’s Equation?
A1) According to Ampere’s circuit law the line integral of magnetic field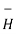 around a closed path is equal to the current enclosed by the path.
around a closed path is equal to the current enclosed by the path.
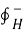 .
. 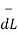 = I enclosed ---------------------------------------------------------(1)
= I enclosed ---------------------------------------------------------(1)
Replacing current by the surface integral of conduction current density 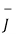 over an area bounded by the path of integration of
over an area bounded by the path of integration of 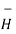 we get
we get
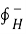 .
. 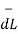 =
= 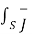 .dS ---------------------------------------------------------------(2)
.dS ---------------------------------------------------------------(2)
Above expression can be made further general by adding displacement current density to conduction current density as follows
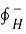 .
. 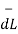 =
= 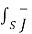 +
+ 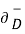 /
/ 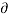 t . d
t . d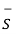 -------------------------------------------------(3)
-------------------------------------------------(3)
Eq(3) is called the Maxwell equation.
Q2) Explain Equation for Continuity for time varying field?
A2) Statement: Equation of continuity represents the law of conservation of charge. That is the charge flowing out that is current through a closed surface in some volume is equal to the rate of decrease of charge within the volume :
I = -dq/dt ------------------------(1)
Where I is current flowing out through a closed surface in a volume and
-dq/dt is the rate of decrease of charge within the volume.
As I = 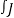 .ds and q =
.ds and q = 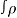 dv
dv
Where J is the Conduction current density and ρ is the Volume charge density
Substitute the value of I and q in equation (1), it will become
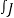 .ds = -
.ds = -  /dt dv ----------------------------------- (2)
/dt dv ----------------------------------- (2)
Apply Gauss’s Divergence Theorem to L.H.S. Of above equation to change
Surface integral to volume integral,
 divergence (J)dV = -
divergence (J)dV = -  (dρ/dt) dv
(dρ/dt) dv
As two volume integrals are equal only if their integrands are equal
Divergence (J) = – dρ/dt
This is equation of continuity for time varying fields.
Q3) Explain Inconsistency of Ampere’s Circuit law?
A3) From Ampere circuital law the line integral of magnetic field B around any closed path is equal to times total current l enclosed by that closed path.
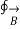 .
. 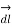 =
= 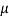 o I
o I
Where l is the steady current.
But this equation is logically inconsistent. Consider the charging capacitor, as the charging continues current flows through the connecting wires which changes with time.
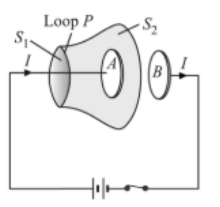
Figure1. Two surfaces
Consider two surfaces S1 and S2 bounded in the same loop P. When Ampere’s circuital law is applied to the two surfaces S1 and S2 bounded by the same loop P.
For surface S1
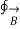 .
. 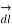 =
= 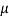 o I
o I
For surface S2
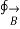 .
.  =
= 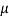 o (0)=0
o (0)=0
Now the two equations are inconsistent. Although surfaces S1 and S2 belong to the same loop, Ampere law works for surface S1 but not for surface S2. Therefore, Ampere law is ambiguous as it does not provide continuity to current path. This ambiguity has arisen because Ampere’s law is valid for steady currents only. As the capacitor charges the current is changing continuously.
Q4) Explain Maxwell’s equation in integral and differential form?
A4) Faraday’s law stated as:
e.m. f d/dt ɸ----------[V] -------------------------------------------- (1)
This equation implies a closed path.
If the closed path taken by N turns conductor, then,
Emf = -N d/dt ɸ -----------------------------------------------------------(2)
Emf = 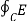 . Dl ------------------------------------------------------------------------(3)
. Dl ------------------------------------------------------------------------(3)
Also, magnetic flux ɸ = 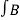 . DS
. DS
Then
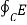 . Dl = -
. Dl = - 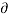 /
/ 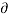 t
t 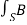 . DS ---------------------------------------------------------(4)
. DS ---------------------------------------------------------(4)
This equation is the integral form of Maxwells equation.
Apply Stokes theorem
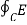 . Dl =
. Dl =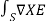 . DS
. DS
Eq(4) becomes 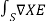 . DS = -
. DS = - 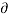 /
/ 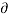 t
t 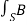 . DS
. DS
That is
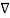 X E = -
X E = - 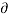 /
/ 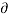 t B ---------------------------------------------------------------(5)
t B ---------------------------------------------------------------(5)
This is Maxwells equation in differential form
Which means that a time- changing magnetic field B(t) produces an electric field, E, it has a property of circulation, its line integral about a general closed path isn’t zero.
Q5) Explain solution of wave equation in free space?
A5) The propagation of electromagnetic radiation as waves is a consequence of the form of Maxwells equations. In free space
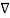 . E =0 ---------------------------------------(1)
. E =0 ---------------------------------------(1)
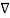 . B =0 ---------------------------------------------(2)
. B =0 ---------------------------------------------(2)
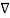 xE = -
xE = - 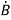 ---------------------------------------------(3)
---------------------------------------------(3)
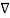 xB =
xB = 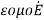 ----------------------------------(4)
----------------------------------(4)
In these expressions E and B are dielectric and magnetic field vectors of the wave 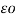 and
and 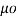 which is called electric permittivity and magnetic permeability of free space.
which is called electric permittivity and magnetic permeability of free space.
It is easily confirmed that the plane wave
Ex = Eo cos(wt-kz) -----------------------------------------------(5)
Ey=0
Ez=0
Bx=0
By = Eo /c cos (wt -kz)--------------------------------------------(6)
Bz=0
From the above equations the wave speed
c=w/k = 1/ √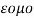
The constant c is the speed of light for electromagnetic waves in free space.
Q6) Explain uniform plain wave propagation?
A6) Waves with constant phase fronts (plane waves) and whose amplitude (E0 ) is uniform
Recall 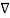 x
x  = -jwμoH
= -jwμoH
Where E field of a uniform plane wave is given by
 =
=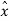 Eo -jkz
Eo -jkz
The magnetic field is then
 =
=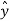 Eo -jkz / ղo
Eo -jkz / ղo
E field is in 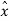 direction
direction
H field is in 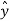 direction
direction
Wave propagating in + 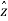 direction
direction
In time domain
E(z,t) = Re{ E ejwt } 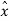 =
= 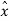 Eo cos(wt -kz)
Eo cos(wt -kz)
H(z,t) = Re{ E ejwt / ղo} 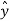 =
= 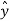 Eo/ ղo cos (wt -kz)
Eo/ ղo cos (wt -kz)
Where ղo is the intrinsic impedance of free space.
Ղo = √ μo/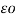 = 120 π = 377 Ω
= 120 π = 377 Ω

Figure. Uniform Wave for Propagation
Q7) Explain the wave equation for conducting medium?
A7) Let us assume that medium is linear, homogeneous and isotropic characterized by permittivity 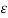 , permeability μ, and conductivity
, permeability μ, and conductivity 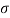 but not any charge or any current other than that determined by Ohms law. Then,
but not any charge or any current other than that determined by Ohms law. Then,
D = 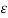 E,B = μH,J =
E,B = μH,J = 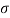 E ,
E , 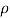 =0.
=0.
Thus Maxwells equation
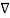 E =
E = 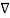 H =0
H =0 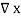 E = -μ
E = -μ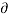 H/
H/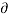 t
t
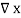 H=
H= 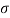 E +
E + 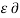 E/
E/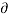 t
t
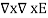 = -μ
= -μ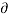 /
/ 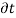 (
( )
)
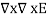 = -μ
= -μ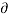 /
/  (
(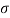 E +
E + 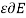 /
/ 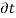 )
)
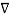 (
( 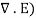 -
- 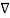 2 E =
2 E = 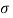 μ
μ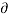 /
/ 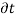 – μ.
– μ.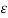 .
.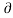 2 E /
2 E / 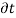 2
2
From the first equation ( 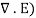 = 0
= 0
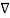 2 E -
2 E - 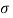 μ
μ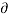 /
/ 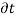 - – μ.
- – μ.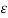 .
.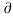 2 E /
2 E /  2 =0
2 =0
This is the equation that governs the conducting medium.
Q8) Explain the wave propagation in lossless and conducting medium?
A8) Lossless medium:
In source free losless medium J = 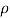 =
= 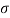 = 0
= 0
Maxwells equations :
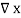 E = -μ
E = -μ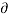 H/
H/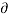 t
t
 H =
H = 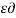 E/
E/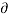 t
t
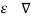 . E = 0
. E = 0
μ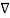 . H =0
. H =0
J = currnt density
 = charge density
= charge density
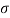 = conductivity
= conductivity
Take the curl of the first equation
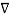 2E -μ
2E -μ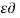 2 /
2 / 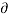 t 2 E =0
t 2 E =0
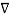 2 H- μ
2 H- μ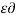 2 /
2 / 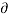 t 2 E=0
t 2 E=0
In free space
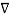 2E -μo
2E -μo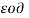 2 /
2 /  t 2 E =0
t 2 E =0
μo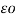 = 1/c 2
= 1/c 2
c = speed of light in free space.
Conductive medium
Consider the Ampère-Maxwell equation,
 x B = μo (jt +
x B = μo (jt + 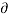 D/
D/ 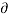 t) ----------------------------------------------(1)
t) ----------------------------------------------(1)
Here, jt is the true current: that is, the current carried by free, as opposed to bound, charges.
Jt = 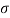 E
E
Here,  is the conductivity.
is the conductivity.
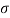 = fo N e 2 / m(
= fo N e 2 / m(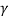 o – iw) .
o – iw) .
Q9) Explain conductors and dielectrics?
A9) Conductor is one in which the outer electrons of an atom is easily detachable and migrate with application of weak Electric field.
A dielectric is one in which the electrons are rigidly bounded to their nucleus, so the ordinary electric field will not be able to detach them away. The dielectric placed in electrostatic field will be subjected to electrostatic induction. The electric field will be twisted and strain the molecules to orient the positive charges in the direction of electric field and negative charges oppositely. If the electric field strength is too high the dielectric will break down cease to beam insulator.
Q10) Write a short note on polarisation?
A10) The polarisation of a plane wave can be defined as the orientation of the electric field vector as a function of time at a fixed point in space. For an electromagnetic wave, the specification of the orientation of the electric field is sufficient as the magnetic field components are related to electric field vector by the Maxwell's equations.
Let us consider a plane wave travelling in the +z direction. The wave has both Ex and Ey components.
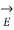 = (
= (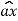 Eex +
Eex + 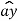 Eey) e -j
Eey) e -j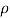 z -----------------------------------------------------(1)
z -----------------------------------------------------(1)
The corresponding magnetic fields are given by
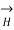 = 1/
= 1/ 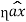 x
x 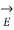
= 1/ 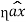 x (
x (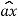 Eox +
Eox + 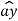 Eoy) e -j
Eoy) e -j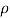 z
z
= 1/ 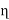 (-
(-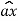 Eoy +
Eoy + 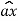 Eox) e -j
Eox) e -j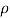 z
z
Depending on the values of Eox and Eoy there are several possibilities:
- If Eoy=0 then the wave is linearly polarised in x-direction
- If Eoy=0 then the wave is linearly polarised in y-direction
- If Eox and Eoy are both real we get linearly polarised wave with the axis of polarisation inclined at an angle tan-1 Eoy/Eoxwith respect to x-axis.
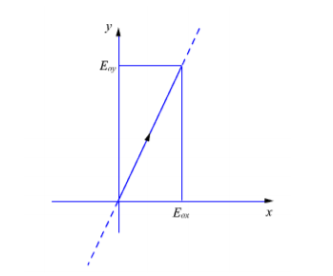
Figure . Linear polarisation
If Eox and Eoy are complex with different phase angles, will not point to a single spatial direction.
Let Eox = |Eox| e iz
Eoy = |Eoy| ejb
Then
Ex(z.t) = Re[ |Eox| e jz e -jβz e jwt] = |Eox| |cos(wt – βz + a)
Ey(z,t) = Re[ |Eoy| e jb e -jβz e jwt] = |Eoy| |cos(wt – βz + b)---------------------(2)
To keep the things simple, let us consider a =0 and b=∏/2 . Further, let us study the nature of the electric field on the z =0 plain.
Ex(o,t) = |Eox| coswt
Ey(o.t) = |Eoy| cos(wt+π/2) = |Eoy|(-sinwt)
(Ex(0,t)/|Eox|) 2 + [Ey(o,t)/|Eoy|) 2 = cos 2 wt + sin 2 wt =1 ----------------------------------(3)
And the electric field vector at z=0 can be written as
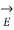 (0,t) = |Eox| cos(wt)
(0,t) = |Eox| cos(wt)  - |Eoy| sin(wt) )
- |Eoy| sin(wt) ) 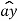 ----------------------------------------------------(4)
----------------------------------------------------(4)
Assuming |Eox| > |Eoy| plot  (o,t) for various values
(o,t) for various values
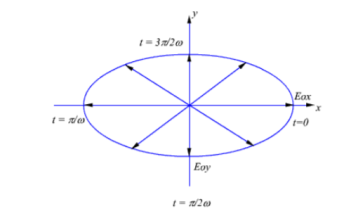
Figure . Plot E(o,t)
We observe that the tip of the arrow representing electric field vector traces qn ellipse and the field is said to be elliptically polarised.
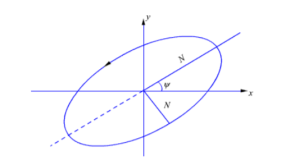
Figure. Polarisation ellipse
If |Eox| = |Eoy| then the tip of the arrow representing electric field vector traces out a circle. Such a case is referred to as Circular Polarisation.