Unit – 1
Fluids
Q1) Define fluid and its type.
A1) A fluid is a substance which is capable of flowing under the action of shear force (however small force may be).
Example- Liquid, gases, vapour.
For a static fluid, shear force is zero.
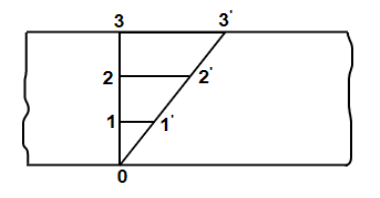
Figure: Force at static fluid
Solid regains their original shape if the shear force within elastic limit when the force is removed. But fluid never regains original shape after the removal of force.
There are two types of fluid:
- Newtonian fluid
- Non-Newtonian fluid
Q2) Explain Newton’s law of viscosity.
A2) According to Newton’s law of viscosity the shear stress is directly proportional to rate of shear strain or rate of angular deformation or velocity gradient. The fluid which follows this is law is called Newtonian fluid.

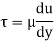
Newton’s law of viscosity is similar to Hooke’s law for solid.
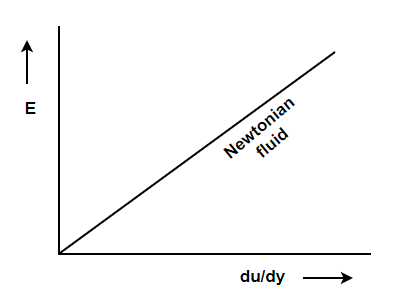
Figure: Diagram for Newton’s law of viscosity
Q3) Define the following terms:
Mass Density
Specific Volume
Specific Gravity
A3) Mass Density:
It is defined as the ratio of mass of the fluid to its volume. Its unit is kg/m3 and its dimensional formula is ML-3.
Density depends on temperature and pressure.
Specific Volume:
Specific volume is defined as the ratio of volume of fluid to the mass of fluid.
It is denoted by Vs.
Mathematically it is denoted as
Vs=V/m=1/
Specific Gravity:
It is defined as the ratio of density of fluid to the density of standard fluid.
Or
- It can be defined as the ratio of specific weight of fluid to the specific weight of standard fluid.
- It is unit less or dimensionless.
- For liquid, the standard fluid is water and for gases the standard fluid is either H2
- Or air at given temperature and pressure.
- The specific gravity of water is 1.
- If the specific gravity of liquid is less than 1 that means it is lighter than water and if the specific gravity of liquid is greater than 1, it means the liquid is heavier than water.
Q4) Explain Viscosity and derive its formula.
A4) Viscosity is a measure of a fluid's resistance to flow. It describes the internal friction of a moving fluid. A fluid with large viscosity resists motion because its molecular makeup gives it a lot of internal friction. It is the internal resistance offered by one layer fluid to the other layer.

Figure: Viscosity diagram
V=d/t
D=v.t
d =du.dt
Tandθ= (du. Dt)/dy
As the tandθ is very less, then
Tandθ=dθ
Then, dθ= (du. Dt)/dy
dθ/dt=du/dy
We know that, shear stress= shear force/shear area
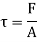
As the molecules are taken into same area:
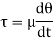
Q5) A flat plate of area 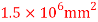 is pulled with a speed of 0.4m/s relative to another plate located at a distance of 0.15mm from it. Find the force and power required to maintain this speed. If the fluid separating them is having viscosity as 1 poise.
is pulled with a speed of 0.4m/s relative to another plate located at a distance of 0.15mm from it. Find the force and power required to maintain this speed. If the fluid separating them is having viscosity as 1 poise.
A5) Area of plate 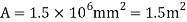
Speed of plate relative to another plate,
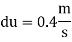
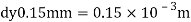
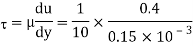
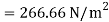
(i) Shear force
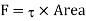
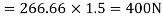
(ii)
Power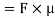
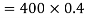
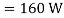
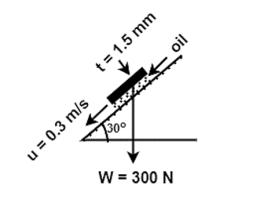
Q6) Calculate the dynamic viscosity of an oil, which is used for lubrication between a square plate of size 0.8m×0.8m and an inclined plane with angle of inclination 30o as shown in figure. The weight of square plate is 200N and it slides down the inclined plane with a uniform velocity of 0.3 m/s. The thickness of oil film is 1.5mm.
A6) A=0.8m×0.8m
𝛉 =30o
W=300N
U=0.3 m/s
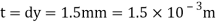
Component of weight W, along the plane
=W cos 60o
=300 cos 60o
F=150 N
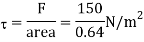
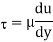
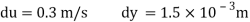
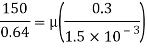
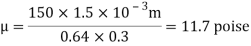
Q7) Find the kinematic viscosity of an oil having density 981kg/m3. The shear stress at a point in oil is 0.2452 N/m2 and velocity gradient at that point is 0.2 per second.
A7) 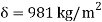
=0.2452 N/m2


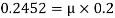
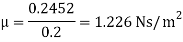
Kinematic viscosity 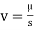
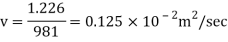
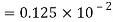 ×
×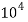 c
c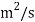
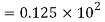 c
c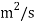

Q8) Define Surface tension.
A8) “Surface tension is the tension of the surface film of a liquid caused by the attraction of the particles in the surface layer by the bulk of the liquid, which tends to minimise surface area”.
Surface tension is the tendency of liquid surfaces to shrink into the minimum surface area possible. Surface tension allows insects (e.g., water striders), to float and slide on a water surface without becoming even partly submerged.
At liquid–air interfaces, surface tension results from the greater attraction of liquid molecules to each other (due to cohesion) than to the molecules in the air (due to adhesion).
There are two primary mechanisms in play. One is an inward force on the surface molecules causing the liquid to contract. Second is a tangential force parallel to the surface of the liquid. The net effect is the liquid behaves as if its surface were covered with a stretched elastic membrane.
Because of the relatively high attraction of water molecules to each other through a web of hydrogen bonds, water has a higher surface tension (72.8 milli newtons (mN) per meter at 20 °C) than most other liquids. Surface tension is an important factor in the phenomenon of capillarity.
Surface tension has the dimension of force per unit length, or of energy per unit area. The two are equivalent, but when referring to energy per unit of area, it is common to use the term surface energy, which is a more general term in the sense that it applies also to solids.
In materials science, surface tension is used for either surface stress or surface energy.
Formula for surface tension
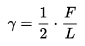
Where, 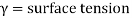
L=Length
F= force
S.I. Unit of Surface tension is N/m
The dimensional formula of surface tension is MT-2.
Q9) Explain capillarity.
A9) Capillarity:
It is the rise or depression of a liquid in a small passage such as a tube of small cross-sectional area, like the spaces between the fibres of a towel or the openings in a porous material.
Capillary action occurs when the adhesion to the walls is stronger than the cohesive forces between the liquid molecules. The height to which capillary action will take water in a uniform circular tube (picture to right) is limited by surface tension and, of course, gravity.
A tube of very fine bore is called capillary. The phenomenon of rise or fall of a liquid in a capillary tube is called capillarity.
If we consider meniscus to be hemispherical in shape, the weight of the liquid rise (the total upward force) = (Volume of liquid in cylinder up to h + volume of liquid in cylinder of radius r and height r – volume of hemisphere of radius r) ρg.
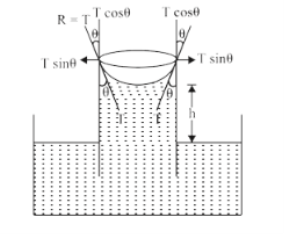
Figure: Capillarity phenomenon
2πr×Tcosθ=[πr2×h+πr2×r−23πr3] ρg2πr×Tcosθ=[πr2×h+πr2×r-23πr3] ρg
T=r(h+r3) ρg2cosθT=r(h+r3) ρg2cosθ.
For capillary r3r3 can be neglected h=2Tcosθrρgh= 2Tcosθrρg.
This is called Ascent formula.
Q10) Explain Compressibility and Bulk Modulus.
A10) It is reciprocal of Bulk modulus (K)
ß=1/K
Bulk Modulus is defined as the ratio of compressive stress to volumetric strain.
K= 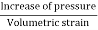
K=(dp)/(-dv/V)
-ve sign means the volume decrease with increase in pressure.
We know that, 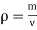
Since, R= Characteristics gas constant and T is constant (isothermal process).
Differentiating the above equation (dp/d )= RT
)= RT
Now the isothermal bulk modulus
KT=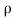 .(dp/d
.(dp/d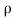 )=
)= 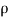 . RT=P
. RT=P
KT=P
Q11) Calculate the modulus of liquid elasticity that reduced 0.035 percent of its volume by applying a pressure of 5[Bar] in a s slow process.
A11) K=(dp)/(-dv/V)
=5/0.00035
= 14285.714 Bar
Q12) Calculate the pressure needed to apply on water to reduce its volume by 1 percent. Assume the temperature to be 20±C.
A12) Bulk modulus is given by
K=(dp)/(-dv/V)
=2.15 10 9 X 0.01
= 2.15 X10 7 [N/m2]
=215 Bar
Q13) Calculate the maximum force necessary to lift a thin wire ring of 0.04[m] diameter from a water surface at 20±C. Neglect the weight of the ring.
A13) F = 2(2 π r 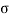 ) cos β
) cos β
The actual force is unknown since the contact angle is unknown. However, the maximum
Force is obtained when β = 0 and thus cos β = 1.
Therefore,
F = 4 π r 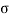
=4 × π r × 0.04 × 0.0728
= 0366[N]
In this value the gravity is not accounted for.
Q14) A small liquid drop is surrounded with the air and has a diameter of 0.001 [m]. The pressure difference between the inside and outside droplet is 1[kPa]. Estimate the surface tension?
A14) The surface tension for selected materials at temperature 20±C when not mentioned
For ex. Chemical
Component SurfaceTension T correction
(mN/m) (mN/mK )
Acetic Acid 27.6 20 n/a
Acetone 25.20 - - 0.1120
Aniline 43.4 22 -0.1085
Benzene 28.88 - -0.1291
Benzyl alcohol 39.00 - -0.0920
Water 72.80 - -0.1514
Q15) The space between two square flat parallel pates is filled with oil. Each of the plate is 720 mm. The thickness of the oil film is 15 mm. The Upper plate, which moves at 3m/s requires a force of 120 N to maintain the speed. Determine
(1) The dynamic viscosity of the oil.
(2) The kinematic viscosity of oil if the specific gravity of oil is 0.95
A15) L =720 mm=0.72 m
t =15mm =15 X10-3 mm
V= 3m/s, F =120 N
To find µ, ν?
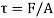
120/0.722 =231.48 N/m2
Newton’s law of viscosity
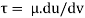
 48 = µ X 3/15 X10-3 mm
48 = µ X 3/15 X10-3 mm
µ =1.16 N.s/m2
Kinematic viscosity
ν = µ/ƍ
= 1.16/0.95 X1000
ν =1.218 X10-3 m2 /sec
Q16) A Newtonian fluid of kinematic viscosity 3 stokes flows over a flat horizontal plate of surface area 0.8 m2. Velocity at y meters from plate is given as u =2y-2y3 in m/s. If the shear force on plate is 0.352 N. Find density of the liquid.
A16) Given: kinematic viscosity ν = 3 stokes =3 X10-4 m2/s
Area, A =0.8 m2, Shear force, F =0.352 N
ν = µ/ƍ
Dynamic viscosity µ = ν. ƍ
= 3X10-4 X ƍ
Velocity, u = 2y-2y3
Differentiate w.r.t. y
Du/dy =2-6y2
At plate surface, y=0, du/dy =2
Shear force F =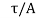
= (µ. Du/dy) X A
0.352 = 3X10-4 X ƍ X2 X0.8
ƍ =733.33 kg/m3
Q17) If the bulk modulus of elasticity of water 2.07 X106 kN/m2.
(i) What is the pressure required to reduce its volume by 25
(ii) What will be the changes in mass density
A17) Given: K =2.07 X106kn /m
DV/V=2 %
ƍ for water =1000 kg/m3
To find: dp, dƍ
(i) Pressure required to reduced its volume by 2%
K = dp /(-dV/V)
2.07 X106 = dp / (2/100)
=41.4 X103 kN/m2
(ii) Change in mass density
K = ƍ dp/dƍ
dƍ / ƍ = dp /k
= 41.4 X 10 3/2.07 X106
dƍ / ƍ = 20 kg/m3
Q18) A glass tube of internal diameter 2 mm is partially dipped in glycerine with its lower end 3omm deep below surface. Air is blown in the tube so as to form an air bubbles at its bottom end of the tube. If specific weight and surface tension of glycerine are 12.356 kN/m2 and0.0637 N/m, find the pressure of air blown.
A18) Given: 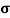 glycerine =0.0637 N/m, d =2 mm =2 X10-3 m
glycerine =0.0637 N/m, d =2 mm =2 X10-3 m
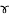 g = 12.356 kN/m2
g = 12.356 kN/m2
Pressure due to 30 mm deep of glycerine
P1 = 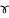 h =12356 X30 X10-3
h =12356 X30 X10-3
= 370.68 N/m2
An air bubble has only one surface,
P2 =4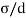
= 4 X0.0637 / (2 X10-3)
= 127.4 N/m2
Pressure of air blow,
P = P1+ P2
= 370.68 +127.4
P = 498.08 N/m2
Q19) Determine the diameter of a droplet of water in mm if the pressure inside is to be greater than that outside by 130 N/m2.
Take  =7.26X10-2 N/m
=7.26X10-2 N/m
A19) Given:
P =130N/m2, =7.26 X10-2 N/m
=7.26 X10-2 N/m
For droplet P =4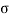 /d
/d
D =4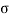 /d =4 X7.26 X10-2 /130
/d =4 X7.26 X10-2 /130
= 2.33 X10-3 m
d = 2.33 mm
The diameter of a droplet of water is 2.33 mm
Q20) A square block weighing 1.0 [kN] with a side surfaces area of 0.1 [m2] slides down an incline surface with an angle of 200. The surface is covered with oil film. The oil creates a distance between the block and the inclined surface of 1 × 10−6[m]. What is the speed of the block at steady state? Assuming a linear velocity profile in the oil and that the whole oil is under steady state. The viscosity of the oil is 3 × 10−5[m2/sec].
A20) The shear stress at the surface is estimated for steady state by
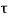 = µdu/dx
= µdu/dx
= 3 × 10-5X U/(1X10-6)
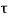 =30 U
=30 U
The total fiction force is then
f = 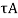
= 0.1 × 30U
= 3U
The gravity force that acting against the friction is equal to the friction hence
Fg = f = 3U = U
U = mg sin 200/3
Or the solution is
U =1 X 9.8 X sin 200/3