Unit - 2

Heat Convection

Q1) Given:  Nitrogen gas at 0°C is flowing over a 1.2 m long, 2 m wide plate maintained at 80°C with a velocity of 2.5 m/s. For nitrogen, r = 1.142 kg/m3, cp= 1.04 kJ/kgK, n = 15.63 x 10–6 m2/s and k = 0.0262 W/mK.

To find: (a) The average heat transfer coefficient and (b) the total heat transfer from the plate.

A1)

The critical Reynolds number for flow over a flat plate is 500,000, so that

Rexc= uxc/v = 500,000

 xc = (500,000  15.63  10-6)/2.5 = 3.126 m = 312.6 cm

Since the plate length is 120 cm in flow direction, laminar flow persists in the entire length of the plate, for which

Num= 0.664 Rel1/2Pr1/3

Where  ReL = uL/v = (2.5  1.2)/(15.63  10-6) = 191, 938.6

And (ReL)1/2 = 438.1

Pr = cpμ/k =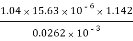

= 0.708

Pr1/3 = (0.708)1/3 = 0.89

 Num = 0.664  438.1  0.89 = 258.9 = hmL/k

 hm = (258.1  0.0262)/1.2 =5.653 W/m2K Ans. (a)

Total rate of heat transfer from the plate

Q = hm(Lb)(T - T) = 5.653  1.2  2  (80 – 0)

= 1085.4 W = 1.085 kW Ans. (b)

Q2) Air at 20°C and at atmospheric pressure flows at a velocity of 4.5 m/s past a flat plate with a sharp leading edge. The entire plate surface is maintained at a temperature of 60°C. Assuming that the transition occurs at a critical Reynolds number of 5 x 105, find the distance from the leading edge at which the boundary layer changes from laminar to turbulent. At the location, calculate the following:

(i)                Thickness of hydrodynamic boundary layer,

(ii)              Thickness of thermal boundary layer,

(iii)           Local and average convective heat transfer coefficients,

(iv)            Heat transfer rate from both sides per unit width of plate,

Assume cubic velocity profile and approximate method. Thermo-physical properties of air at mean film temperature of 40°C are: = 1.128 kg/m3, = 16.96 x 10–6 m3/s, k = 0.02755 W/mK and Pr = 0.7.

A2)

At the transition point,

Rexc = u xc/v

 xc =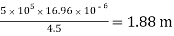

(i) Thickness of hydroddynamic boundary layer

δ = 4.64x/Rexc = (4.64  1.88)/ 5105

= 0.01234 m or 12.34 mm Ans. (i)

(ii)  Thickness of thermal boundary layer

δth = 0.975δ/(Pr)1/3 = (0.975  0.01234)/(0.7)1/3

δth = 0.01355 m or 13.55 mm  Ans. (ii)

(iii) Nuxc = 0.332 (Rexc)1/2 (Pr)1/3

= 0.332(5  105)1/2(0.7)1/3 = 208.34

hc = Nuc  k/xc = (208.34  0.02755)/1.88

= 3.05 W/m2K Ans. (iii)

Average heat transfer coefficient

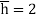hc = 2 3.05 = 6.1 W/m2K Ans. (iii)

(iv)  Heat transfer from both sides per unit width of the plate

Q = 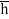(2As)T = 6.1  2  (1.88  1)  (60 – 20)

= 917.4 W Ans.(iv)

Q3) Air at 20°C and 1 atm flows over a flat plate at 40 m/s. The plate is 80 cm long and is maintained at 60°C. Assuming unit depth in z-direction, calculate the heat transfer rate from the plate. Properties of air at 40°C are: Pr = 0.7, k = 0.02723 W/m K, cp= 1.007 kJ/kg K and 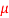= 1.906 x 10–5 kg/ms.

A3)

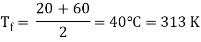
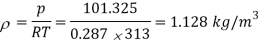
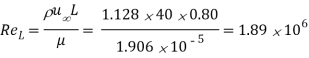
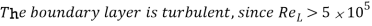
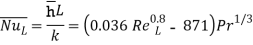
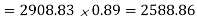
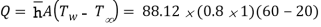
= 2820 W = 2.82 kW       Ans.

Q4) Given: Water at 10°C flows over a flat plate (at 90°C) measuring 1 m x 1 m, with a velocity of 2 m/s. Properties of water at 50°C are = 988.1kg/m3, = 0.556 x 10–6 m2/s, Pr = 3.54 and k = 0.648 W/mK. To find: (a) The length of plate over which the flow is laminar, (b) the rate of heat transfer from the entire plate.

A4)

Up to Rec = 500,000, the flow is laminar and beyond this value, the flow is turbulent after a transition section. Therefore,

Rec = 500,000 = uxc/v

xc = (500,000  0.556  10-6)/2 = 0.139 m

The length of plate up to which the flow is laminar is 0.139 m. Ans. (a)

Laminar Part:

Nuc = 0.664 (Rec)1/2(Pr)1/3

= 0.664 (500,000)1/2(3.54)1/3

= 715.08 = hmxc/k

hm = (715.08  0.648)/0.139 = 3333.6 W/m2K

Qlaminar = hmA(T - T)

= 3333.6  0.139  1  (90 – 10)

= 37069.6 W = 37.07 kW

Turbulent Part

hm(L – xc)/k = 0.036 [(ReL)0.8 – (ReC)0.8] Pr1/3

Where ReL = UL/v = (2  1  106)/0.556 = 3.597  106

And (ReL)0.8 = 175, 692

(Rec)0.8 = (500,000)0.8 = 36239

Pr1/3 = (3.54)1/3 = 1.523

 hm(1- 0.139)/0.648 = 0.036 (175692 – 36,239)  1.523

= 7645.93

 hm = 5754.4 W/m2K

Qturbulent  = 5754.4  0.861  1  (90 – 10)

= 396365 W = 396.365 kW

 Qtotal = Qlam. + Qturb

= 37.07 + 396.37

= 433.33 kW Ans. (b)

Q5) Water is heated while flowing through a 1.5 cm x 3.5 cm rectangular cross-section tube at a velocity of 1.2 m/s. The entering temperature of the water is 40°C, and the tube wall is maintained at 85°C. Determine the heat transfer coefficient. Properties of water at the mean bulk temperature of 55°C are: = 985.5 kg/m3; cp= 4.18 kJ/kg K, = 0.517 x 10–6 m2/s, k = 0.654 W/m K and Pr = 3.26.

A5)

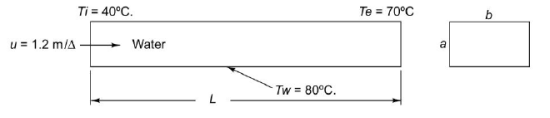
De 4A/P = 4ab/2(a+b) = 2ab/(a+b) = (2  1.5  3.5 )/(1.5+ 3.5) = 2.1 cm

Red = umDe/v = (1.2  2.1  10-2)/(0.517  10-6) = 48,740

(Red)0.8 (40, 740)0.8 = 5627.4

(Pr)0.4 = (3.26)0.4 = 1.604

Using Dittus- Boelter equation, with water being heated,

Nud = 0.023 Red0.8 Pr0.4 = 0.023  5627.4  1.604

= 207.6 = hcDc/k

hc=( 207.6  0.654)/(2.1  10-2) = 6465 W/m2 K = 6.465 kW/m2K

Q6) Air at 20°C and a pressure of 1 bar is flowing over a flat plate at a velocity of 3 m/s. If the plate is 280 mm wide and at 56°C, estimate the following quantities at x = 280 mm, given that the properties of air at the bulk mean temperature of 38°C are: = 1.1374 kg/m3, k = 0.02732 W/mK, cp= 1.005 kJ/kgK, and = 16.768 x 10–6 m2/s:

(i)boundary layer thickness, (ii) local friction coefficient, (iii) average friction coefficient, (iv) shearing stress due to friction, (v) thickness of thermal boundary layer, (vi) local convective heat transfer coefficient, (vii) average convective heat transfer coefficient, (viii) rate of heat transfer by convection.

A6)

u = 3 m/s, x = 280 mm = 0.28 m,  = 1.1374 kg/m3

k = 0.02732 W/mK, cp = 1.005 kJ/kgK, v = 16.768  10-6 m2/s.

Pr = cpμ/k = (1.005  16.768  10-6  1.1374  103)/0.02732 = 0.7

We are to confirm first whether the flow is laminar or turbulent. At x = 0.28 m,

Rex = ux/v = (30.28)/(16.768  10-6) = 5  104

Since Rex< 5  105, the flow is laminar throughout.

(i) Boundary layer thickness

δ = 5x/Rex = 50.28/5104 = 0.00626 m

= 6.26 mm Ans.

(ii) Local friction coefficient

cfx = 0.664/Rex = 0.664/5104

= 0.002969 Ans.

(iii) Average friction coefficient

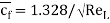= 1.328/ 5 104 = 0.005938 Ans.

(iv) Shearing stress due to friction

τw = cfx u2/2 = 0.002960  1.1374 32/2

= 0.01519 N/m2 Ans.

(v) Thickness of thermal boundary layer

δth = 8/Pr1/3 = (0.00626)/(0.7)1/3 = 0.00705 m = 7.05 mm Ans.

(vi) Local convective heat transfer coefficient

hx = 0.332 k/x  (Rex)1/2(Pr)1/3

= 0.332  0.02732/0.28  (5  104)1/2 (0.7)1/3

= 6.43 W/m2 K Ans.

(vii) Average convective heat transfer coefficient

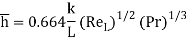
= 0.664  0.02732/0.28  (5  104)1/2 (0.7)1/3

= 12.86 W/m2 K Ans.

(vii) Rate of heat transfer

Q =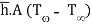

= 12.86  0.28  0.28  (56 – 20)

= 36.29 W Ans.

Q7) A metal plate 0.609 m in height forms the vertical wall of an oven and is at a temperature of 171 °C. Within the oven is air at a temperature of 93.4 °C and atmospheric pressure. Assuming that natural convection conditions hold near the plate, and that for this case Nu = 0.548 (Gr Pr)1/4

Find the mean heat transfer coefficient and the heat taken up by air per second per metre width. For air at 132.2°C, take k = 33.2 x 10–6 kW/m K, 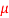= 0.232 x 10–4 kg/ms, cp= 1.005 kJ/kg K. Assume air as an ideal gas and R = 0.287 kJ/kg K.

A7)

Prandtl number Pr = μcp/k = (0.232  10-4  1.005  103)/(32.2  10-6  103)

= 0.7241

Tmean = (171 + 93.4)/2 = 132.2oC = 405.2 K

β = 1/Tmean = 2.47  10-3 K-1

θ = Tw - T = 171 – 93.4 = 77.6oC = 77.6 K

 = p/RT = 101.325/(0.287  405.2) = 0.8713 kg/m3

v = μ/ = (0.232  10-4)/0.8713 = 2.663  10-5 m2/s

Gr = gβθL3/v2 = (9.81  2.47  10-3  77.6  (0.609)3)/(2.663  10-5)2

= 5.985  108

Nu = 0.548(5.985  108  0.7241)1/4 = 79.07

= (mL)/k

hm = (79.07  32.2  10-6)/0.609 = 4.181  10-3 kW/m2 K

= 4.181 W/m2 K Ans.

Q = hm (Lb)θ

Q/b = 4.181  0.609  77.6 = 197.57 W/m Ans.

Q8) Given: A 0.15 m outer diameter of steel pipe lies 2 m vertically and 8 m horizontally in a large room with an ambient temperature of 30°C. The pipe surface is at 250°C and has an emissivity of 0.60. To estimate: The total rate of heat loss from the pipe to the atmosphere.

A8)

Heat is lost by the pipe to the atmosphere both by natural convection and radiation.

Natural convection β = 1/Tf = 1/(140 + 273) = 1/413 K-1

(a) Vertical Part: Grashof number, Gr = gβθL3/v2

Or,  Gr = (9.81  (1/413)(250 – 30) 23)/(27.8  10-6)2

= 0.0541  1012

Gr Pr = 0.0541  1012  0.684

= 3.7  1010

Since Gr.Pr > 109, the flow is turbulent over the pipe.

Nu = 0.13 (Gr.Pr)1/3

= 0.13(3.7  1010)1/3

= 432.7 = hvL/k

hv = (432.7  0.035)/2 = 7.572 W/m2K.

(b) Horizontal part:

Grd = gβθD3/v2

= (9.81  1/413  220  (0.15)2)/(27.8  10-6)2

= 2.282  107

GrdPr = 2.282  107  0.684

= 1.56  107

Since Grd. Pr < 2 x 107, the flow is laminar.

Nud = 0.53 (Grd .Pr)1/4

= 0.53 (1.56  107)1/4

= 33.3 = hHD/k

hH = (33.3 0.035)/0.15 = 7.77 W/m2K

Total heat loss by natural convection

QC = QH + QV

= (hHAH+ hVAV) (T - T)

= [ 7.572  π 0.15  2 + 7.77π 0.15  8] (250 – 30)

= 6444 W = 6.444 kW

Radiation: Heat lost by radiation

Qr = σA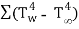

= 5.67  π  0.15  10  0.6 (5.234 – 3.034)

= 10643 W = 10.643 kW

Total heat loss = Qc + Qr

= 6.444 + 10.643

= 18.657 kW Ans.

Q9) A nuclear reactor with its core constructed of parallel vertical plates 2.2 m high and 1.45 m wide has been designed on free convection heating of liquid bismuth. The maximum temperature of the plate surfaces is limited to 960°C, while the lowest allowable temperature of bismuth is 340°C. Calculate the maximum possible heat dissipation from both sides of each plate. For the convection coefficient the appropriate correlation is

Nu = 0.13 (Gr.Pr)1/3

Where the properties evaluated at the mean film temperature of 650°C for bismuth are: = 104 kg/m3, 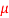= 3.12 kg/m-h, cp= 150.7 J/kgK, k = 13.02 W/mK.

A9)

β = 1/Tf = 1/(650 + 273) = 1.08  10-3 K-1

Where Tf = (960+340)/2 = 650oC

Pr = μcP/k =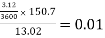

Gr = gβθL32/μ2 = (9.81  1.08  10-3  (960 – 340)(2.2)3 (104)2)/(3.12/3600)2

= 9.312  1015

Gr.Pr = 9.312  1015  0.01 = 93.12  1012

For the given correlation

Nu = hL/k = 0.13 (93.12  1012)0.333

= 0.13(93.12)0.333 104 = 0.5883  104

h = 13.02/2.2  0.5883  104 = 34820 W/m2K

 Heat dissipation from both sides of each plate

Q = 2h AT

= 2  34, 820  (2.2  1.45)  (960 – 340)

= 137734 W = 137.734 kW Ans.

Q10) The maximum allowable surface temperature of an electrically heated vertical plate 15 cm high and 10 cm wide is 140°C. Estimate the maximum rate of heat dissipation from both sides of the plate in an atmosphere at 20°C. The radiation heat transfer coefficient is 8.72 W/m2 K. For air at 80°C, take n = 21.09 x 10–6 m2/s, Pr = 0.692 and k = 0.03 W/m K.

A10)

= (9.81  (1/353)  120  (0.15)3)/(21.09  10-6)2  0.692

= 17,510,650(< 109)

Nu = 0.59 (Ra)1/4 = 0.59  (17,510,650)1/4

= 38.166 = hCL/k

hc = (38.166  0.03)/0.15 = 7.6332 W/m2K

As heat transfer takes place from both the sides

QC = 2hcA(Tw - T)

= 2 7.6332  0.15  0.1  120 = 27.48 W

Qr = 2hrA(Tw - T) = 2 8.72  0.15  0.1  120

= 31.392 W

Qtotal = Qc +Qr = 58.872 W Ans.

Q11) Differentiate between forced and natural convection.

A11)