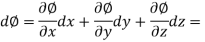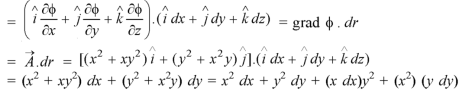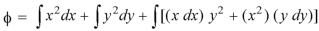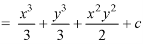Unit-6

Vector Differential Calculus

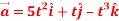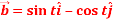Q1) If and then find-

1.

2.

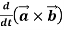
Sol. 1. We know that-

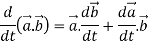
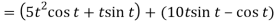
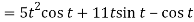
2.

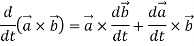
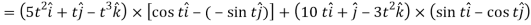
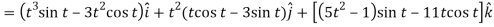
Q2) A object move in the path 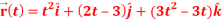where t is the time in seconds and distance is measured in feets. Then find and as functions of t.

Sol.

We know that-

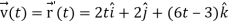
And

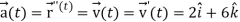
Now we will use-

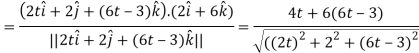
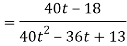
And now-

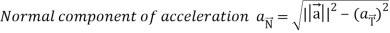
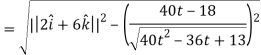
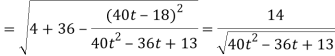
Q3) If 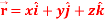, then show that

1.

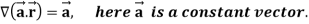
2.

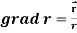
Sol.

Suppose 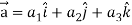  and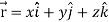

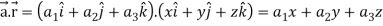
Now taking L.H.S,

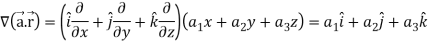
Which is

                                                                                               Hence proved.

2.

So that

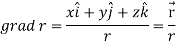
Q4) If 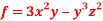then find grad f at the point (1,-2,-1).

Sol.

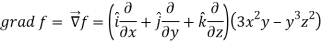
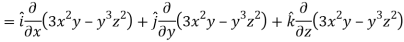
Now grad f at (1 , -2, -1) will be-       

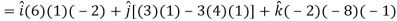
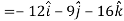
Q5) If 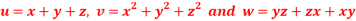then prove that grad u , grad v and grad w are coplanar.

Sol.

Here-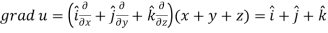                     

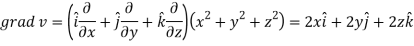
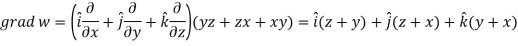
Now-

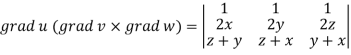
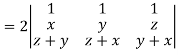
Apply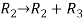

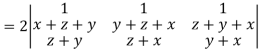
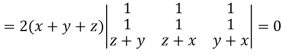
Which becomes zero.

So that we can say that grad u, grad v and grad w are coplanar vectors.

Q6) then find the divergence and curl of .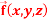

Sol.  we know that-

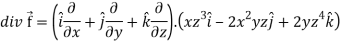
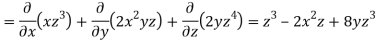
Now-

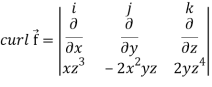
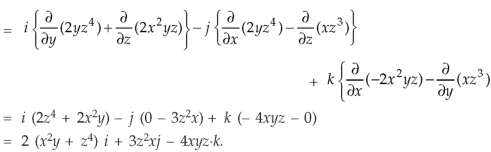
Q7) Find the directional derivative of 1/r in the direction where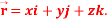

Sol. Here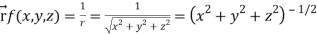

Now,

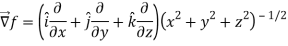
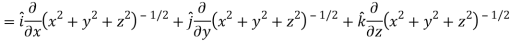
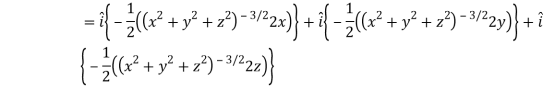
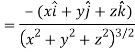
And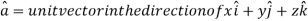

We know that-

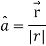
So that-

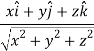
Now,

   Directional derivative-

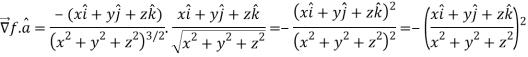
Q8) Find the directional derivative of At the points (3, 1, 2) in the direction of the vector .

Sol. Here it is given that-

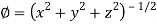
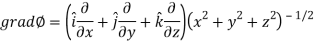
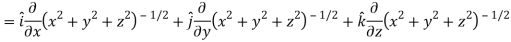
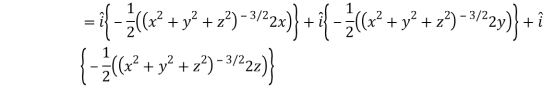
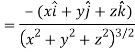
Now at the point (3, 1, 2)-

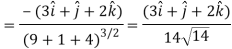
Let 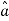be the unit vector in the given direction, then

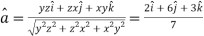at (3, 1, 2)

Now,

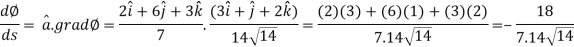
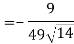
Q9) Prove that the vector field is irrotational and find its scalar potential.

Sol. As we know that if 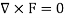then field is irrotational.

So that-

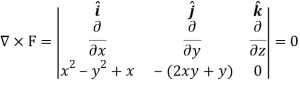
So that the field is irrotational and the vector F can be expressed as the gradient of a scalar potential,

That means-

Now-

   ………………… (1)

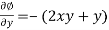  ……………………. (2)

Integrating (1) with respect to x, keep ‘y’ as constant-

We get-

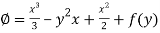      …………….. (3)

Integrating (1) with respect to y, keep ‘x’ as constant-

We get-

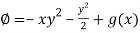      …………….. (4)

Equating (3) and (4)-

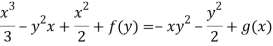
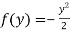and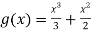

So that-

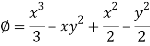
Q10) Show that the vector field 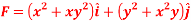is irrotational and find the scalar potential function.

Sol. Now for irrotational field we need prove-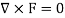 

So that-

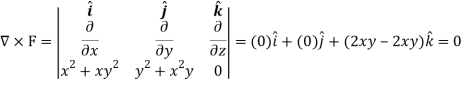
So that the vector field is irrotational.

Now in order to find the scalar potential function-

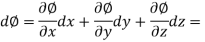
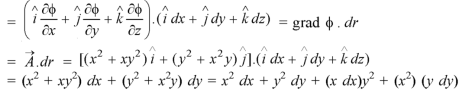
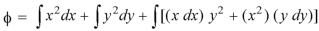
Unit-6

Vector Differential Calculus

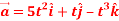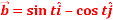Q1) If and then find-

1.

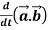
2.

Sol. 1. We know that-

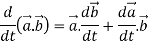
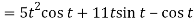
2.

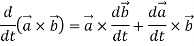
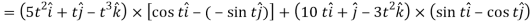
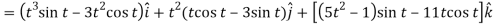
Q2) A object move in the path 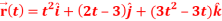where t is the time in seconds and distance is measured in feets. Then find and as functions of t.

Sol.

We know that-

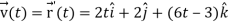
And

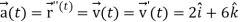
Now we will use-

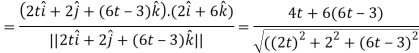
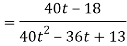
And now-

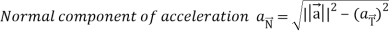
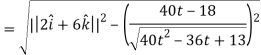
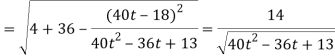
Q3) If 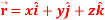, then show that

1.

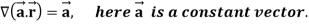
2.

Sol.

Suppose 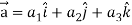  and

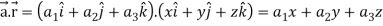
Now taking L.H.S,

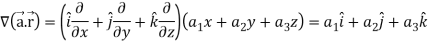
Which is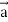

                                                                                               Hence proved.

2.

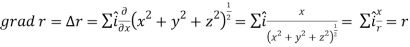
So that

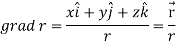
Q4) If then find grad f at the point (1,-2,-1).

Sol.

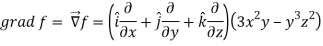
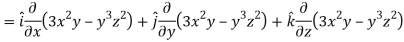
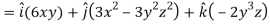
Now grad f at (1 , -2, -1) will be-       

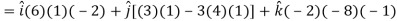
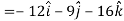
Q5) If 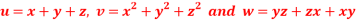then prove that grad u , grad v and grad w are coplanar.

Sol.

Here-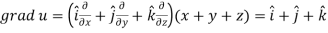                     

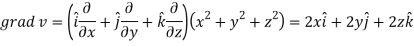
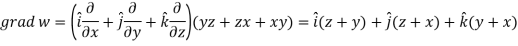
Now-

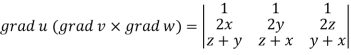
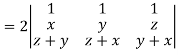
Apply

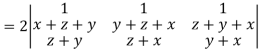
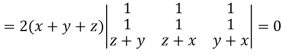
Which becomes zero.

So that we can say that grad u, grad v and grad w are coplanar vectors.

Q6) then find the divergence and curl of .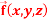

Sol.  we know that-

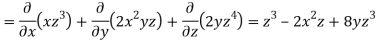
Now-

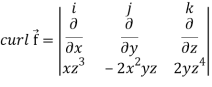
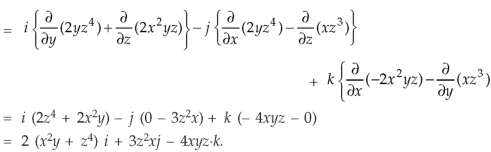
Q7) Find the directional derivative of 1/r in the direction where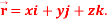

Sol. Here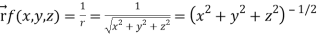

Now,

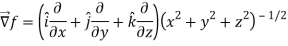
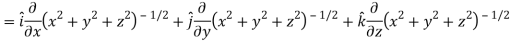
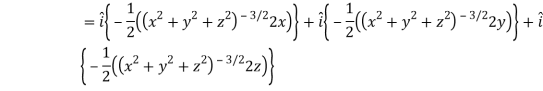
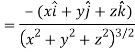
And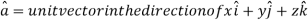

We know that-

So that-

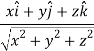
Now,

   Directional derivative-

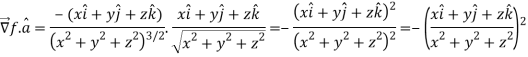
Q8) Find the directional derivative of 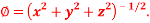At the points (3, 1, 2) in the direction of the vector .

Sol. Here it is given that-

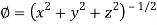
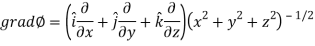
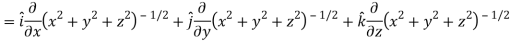
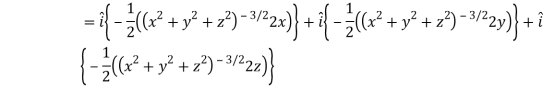
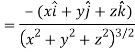
Now at the point (3, 1, 2)-

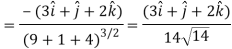
Let 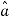be the unit vector in the given direction, then

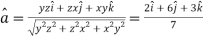at (3, 1, 2)

Now,

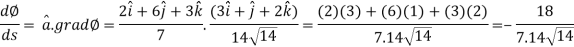
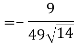
Q9) Prove that the vector field 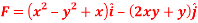is irrotational and find its scalar potential.

Sol. As we know that if 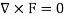then field is irrotational.

So that-

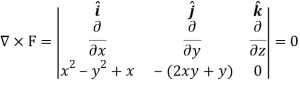
So that the field is irrotational and the vector F can be expressed as the gradient of a scalar potential,

That means-

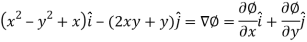
Now-

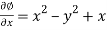   ………………… (1)

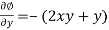  ……………………. (2)

Integrating (1) with respect to x, keep ‘y’ as constant-

We get-

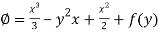      …………….. (3)

Integrating (1) with respect to y, keep ‘x’ as constant-

We get-

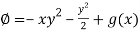      …………….. (4)

Equating (3) and (4)-

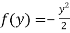and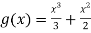

So that-

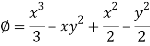
Q10) Show that the vector field 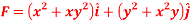is irrotational and find the scalar potential function.

Sol. Now for irrotational field we need prove-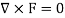 

So that-

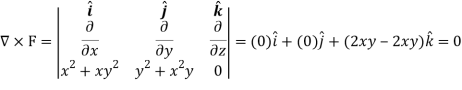
So that the vector field is irrotational.

Now in order to find the scalar potential function-