Unit-4
Center of Gravity and Moment of Inertia
Question 1) Determine moment of Inertia for  section about x & y axis as shown in figure
section about x & y axis as shown in figure
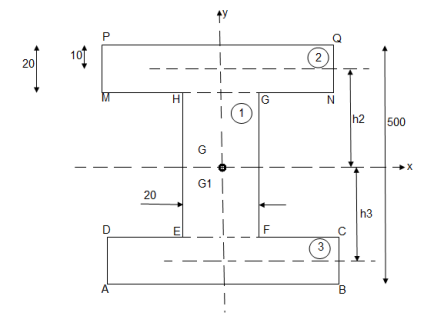
M.I. If this rectangle about xx and yy axis will be,  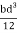 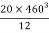 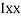 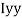 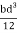 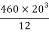 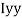 | 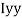 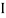 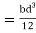 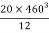 = 106666666.7 mm4 Consider rectangle ABCD area ③  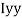 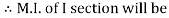 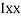 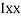 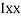 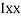 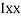  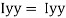 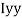 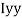 = 213.64 
|
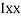 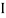 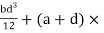 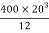 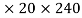  |
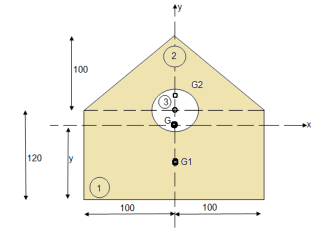 Question 2): Determine the moment of inertia of shaded area as shown in figure about its centroid as axis.
Question 2): Determine the moment of inertia of shaded area as shown in figure about its centroid as axis.
Answer 2)
As this figure is symmetrical about
Y axis
X = 100 mm
Y = 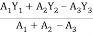
There
Area ① = rectangle
Area ② = triangle
Area ③ = circle
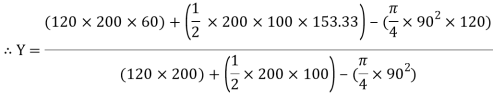
Y = 79.95 mm
 to find M.I. Of shaded portion , let G is the centroid of shaded area which is at y = 79.95 mm from base.
to find M.I. Of shaded portion , let G is the centroid of shaded area which is at y = 79.95 mm from base.
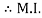 of shaded portion @ x-x axis passing through its centroid G will be,
of shaded portion @ x-x axis passing through its centroid G will be,
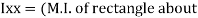 x-x) +
x-x) +
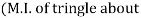 x-x) –
x-x) –
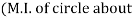 x-x axis)
x-x axis)
 1 +
1 +  2
2 3
3
= (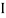 G1 + A1h12) +
G1 + A1h12) + 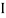 G2 + A2h22) -
G2 + A2h22) - 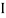 G3 + A3h32)
G3 + A3h32)
=  +
+ 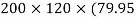 -60
-60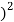 ] +
] +
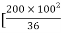 +
+ 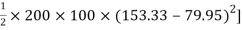 –
–
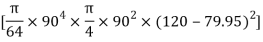
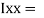 84329013.21 mm4
84329013.21 mm4
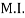 of shaded portion about y-y axis passing through its centroid G will be,
of shaded portion about y-y axis passing through its centroid G will be,
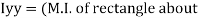 y - y) +
y - y) +
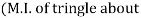 y-y axis) –
y-y axis) –
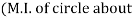 y - y axis)
y - y axis)
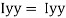 1 +
1 + 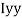 2
2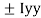 3
3
= 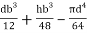
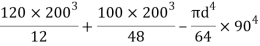
= 80000000 + 16666666.67 – 3220623.34
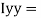 93446043.33 mm4
93446043.33 mm4
Question 3) Find the support reactions of given beam for loading as shown below.
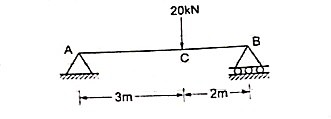
Answer 3)
 Draw FBD of given beam & applying conditions of equilibrium
Draw FBD of given beam & applying conditions of equilibrium
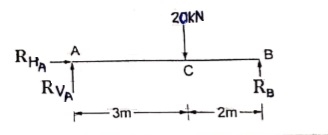
∑fx = 0
RHA = 0
∑fxy = 0
RVA – 20 + RB = 0
RVA + RB = 20 -----(1)
Taking moments at A
∑MA = 0
(20 × 3) – (RB × 5) = 0
60 – 5RB
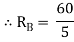
RB = 12 kN  put in equation (1)
put in equation (1)
 RVA + 12 = 20
RVA + 12 = 20
 RVA = 20-12
RVA = 20-12
RVA = 8 kN 
Question 4) Find support reaction for given beam
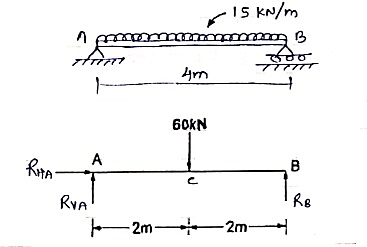
Answer 4)  Resolving forces horizontally
Resolving forces horizontally
∑fx = 0
RHA = 0
Resolving forces vertically
∑fy = 0
RVA – 60 + RB = 0
RVA + RB = 60 ------- (1)
Taking moment at A
∑MA = 0
(60 × 2) – (RB × 4) = 0
 120 – 4RB = 0
120 – 4RB = 0
 RB =
RB = 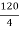
RB = 30 kN 
Question 5) Find the support reactions of a given loading for Beam.
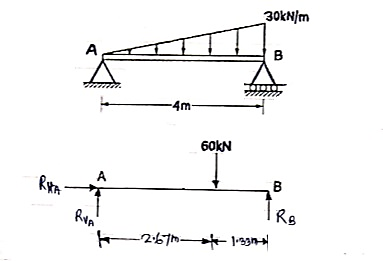
Answer 5)
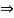 Resolving forces horizontally
Resolving forces horizontally
∑fX = 0
RHA = 0
Resolving forces vertically
∑fY = 0
RVA – 60 + RB = 0
RVA + RB = 60 ----------------- (1)
Taking moments at A
∑ MA = 0
(RVA × 0) + (60 × 2.67) – 4RB = 0
160.2 – 4RB = 0
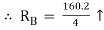 Put in equation (1)
Put in equation (1)
We get
RVA + 40 = 60
RVA = 60 – 40
RVA = 20 kN 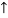
Question 6) Find the support reactions for given beam.
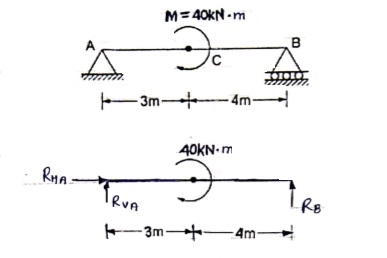
Answer 6)
 Resolving force horizontally
Resolving force horizontally
∑fX = 0
RHA = 0
Resolving forces vertically
∑fy = 0
RVA + RB = 0 ------- (1)
Taking moments at A
 40 – 7RB = 0
40 – 7RB = 0
 RB =
RB = 
RB = 5.71 kN 
Put above value in equation (1)
RVA + RB = 0
RVA + 5.71 = 0
RVA = - 5.71 kN
RVA = 5.71 kN 
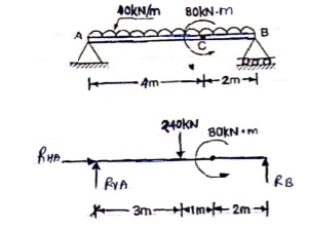 Question 7)
Question 7)
Answer 7)
Resolving forces horizontally
∑fX = 0
RHA = 0
Resolving forces vertically
∑fy = 0
RVA – 240 + RB = 0
RVA + RB = 240 ----------- (1)
Taking moment at A
∑ MA = 0
(240 × 3) – 80 – 6RB = 0
640 – 6RB = 0
RB = 106.67 kN 
Put this value in equation (1)
RVA + RB = 240
RVA = 240 – 106.67
RVA = 133.33 kN 
Question 8) Find support reactions for the loading as shown below:
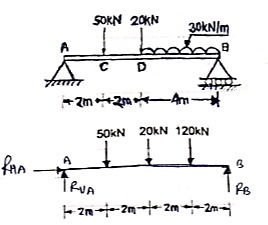
Answer 8)
 Resolving forces vertically
Resolving forces vertically
∑fy = 0
RVA – 50 – 20 – 120 + RB = 0
RVA + RB = 190 ---- (1)
Resolving forces horizontally
∑fX = 0
RHA = 0
Taking moments at A
∑ MA = 0
(50 × 2) + (20 × 4) + (120 × 6) – 8RB = 0
100 + 80 + 720 – 8RB = 0
900 – 8RB = 0
RB – 112.5 kN 
Put above value in equation (1)
RVA + 112.50 = 190
RVA = 77.5 kN 
Question 9) Find the reactions at support A & B.
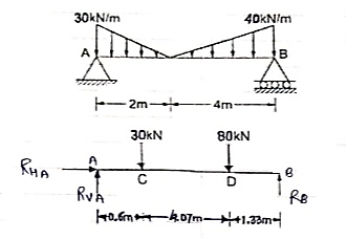
Answer 9)
 Resolving forces vertically
Resolving forces vertically
∑fy = 0
RVA – 30 – 80 + RB = 0
RVA + RB = 110 ------ (1)
Resolving forces horizontally
∑fX = 0
RHA = 0
Taking moments at A
∑ MA = 0
(30 × 0.6) + [80 × (4.07 + 0.6)]- (6 × RB) = 0
18 + 373.6 – 6RB = 0
6RB = 391.6
RB= 65.267 kN
Put above value in equation (1)
RVA + RB = 110
RVA + 65.267 = 110
RVA = 110 – 65.267
RVA = 44.733 KN
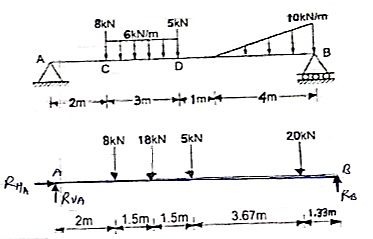
Question 10) Find support reactions at A & B
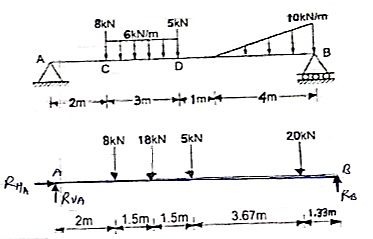
Answer 10)
 Resolving forces vertically
Resolving forces vertically
∑fy = 0
RVA – 8 – 18 – 5 – 20 + RB = 0
RVA + RB = 51 ------ (1)
Resolving forces horizontally
∑fX = 0
RHA = 0
Taking moments at A
∑ MA = 0
(8 × 2) + (18 × 3.5) + (5 × 5 ) + (20 × 8.67) – 10RB = 0
16+ 63 + 25 + 173.4 – 10RB = 0
277.4 – 10RB = 0
RB = 27.74 kN
Put above value in equation (1)
RVA = 51 – 27.74
RVA = 23.26 kN
Question 11) Explain the difference between centre of gravity and centroid?
Answer 11)
Difference Between Center of Gravity and Centroid | |
Center of Gravity | Centroid |
The point where the total weight of the body focuses upon | It is referred to the geometrical center of a body |
It is the point where the gravitational force (weight) acts on the body | It is referred to the center of gravity of uniform density objects |
It is denoted by g | It is denoted by c |
Center of Gravity in a uniform gravitational field is the average of all points, weighted by local density or specific weight | The centroid is a point in a plane area in such a way that the moment of area about any axis throughout that point is 0 |
It is a physical behaviour of the object, a point where all the weight of an object is acting | It is a geometrical behaviour. It is the center of measure of the amount of geometry. |
Question 12: Find the centroid of the triangle whose vertices are A(2, 6), B(4, 9), and C(6,15).
Answer 12) Solution:
Given:
A(x1, y1) = A (2, 6)
B(x2, y2) = B (4, 9)
C(x3, y3) = C (6, 15)
We know that the formula to find the centroid of a triangle is = ((x1+x2+x3)/3, (y1+y2+y3)/3)
Now, substitute the given values in the formula
Centroid of a triangle = ((2+4+6)/3, (6+9+15)/3)
= (12/3, 30/3)
= (4, 10)
Therefore, the centroid of the triangle for the given vertices A(2, 6), B(4,9), and C(6,15) is (4, 10).
Unit-4
Unit-4
Unit-4
Center of Gravity and Moment of Inertia
Question 1) Determine moment of Inertia for  section about x & y axis as shown in figure
section about x & y axis as shown in figure

M.I. If this rectangle about xx and yy axis will be, 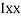 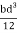 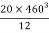 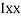 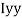  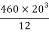 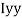 | 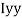 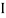 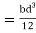 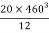 = 106666666.7 mm4 Consider rectangle ABCD area ③ 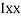 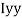 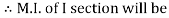 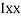      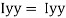 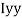 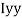 = 213.64 
|
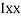  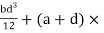 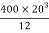 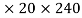 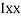 |
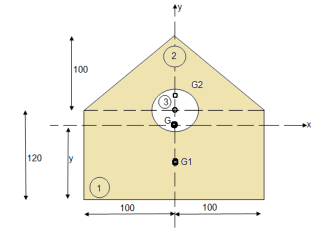 Question 2): Determine the moment of inertia of shaded area as shown in figure about its centroid as axis.
Question 2): Determine the moment of inertia of shaded area as shown in figure about its centroid as axis.
Answer 2)
As this figure is symmetrical about
Y axis
X = 100 mm
Y = 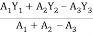
There
Area ① = rectangle
Area ② = triangle
Area ③ = circle
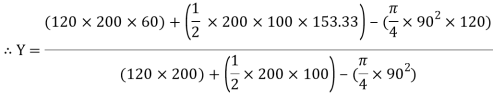
Y = 79.95 mm
 to find M.I. Of shaded portion , let G is the centroid of shaded area which is at y = 79.95 mm from base.
to find M.I. Of shaded portion , let G is the centroid of shaded area which is at y = 79.95 mm from base.
 of shaded portion @ x-x axis passing through its centroid G will be,
of shaded portion @ x-x axis passing through its centroid G will be,
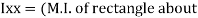 x-x) +
x-x) +
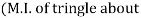 x-x) –
x-x) –
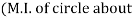 x-x axis)
x-x axis)
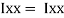 1 +
1 + 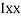 2
2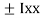 3
3
= (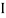 G1 + A1h12) +
G1 + A1h12) + 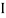 G2 + A2h22) -
G2 + A2h22) - 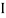 G3 + A3h32)
G3 + A3h32)
= 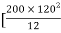 +
+ 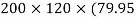 -60
-60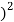 ] +
] +
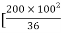 +
+ 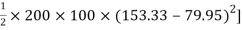 –
–
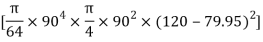
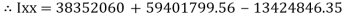
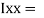 84329013.21 mm4
84329013.21 mm4
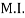 of shaded portion about y-y axis passing through its centroid G will be,
of shaded portion about y-y axis passing through its centroid G will be,
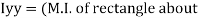 y - y) +
y - y) +
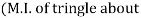 y-y axis) –
y-y axis) –
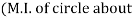 y - y axis)
y - y axis)
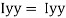 1 +
1 + 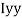 2
2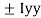 3
3
= 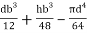
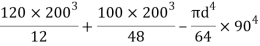
= 80000000 + 16666666.67 – 3220623.34
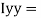 93446043.33 mm4
93446043.33 mm4
Question 3) Find the support reactions of given beam for loading as shown below.
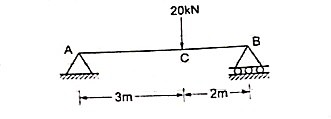
Answer 3)
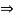 Draw FBD of given beam & applying conditions of equilibrium
Draw FBD of given beam & applying conditions of equilibrium
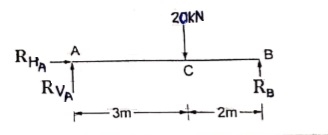
∑fx = 0
RHA = 0
∑fxy = 0
RVA – 20 + RB = 0
RVA + RB = 20 -----(1)
Taking moments at A
∑MA = 0
(20 × 3) – (RB × 5) = 0
60 – 5RB
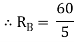
RB = 12 kN 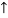 put in equation (1)
put in equation (1)
 RVA + 12 = 20
RVA + 12 = 20
 RVA = 20-12
RVA = 20-12
RVA = 8 kN 
Question 4) Find support reaction for given beam
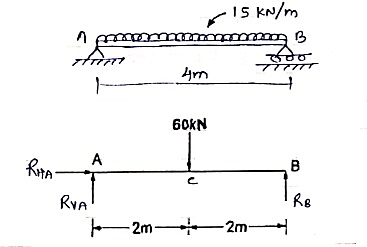
Answer 4)  Resolving forces horizontally
Resolving forces horizontally
∑fx = 0
RHA = 0
Resolving forces vertically
∑fy = 0
RVA – 60 + RB = 0
RVA + RB = 60 ------- (1)
Taking moment at A
∑MA = 0
(60 × 2) – (RB × 4) = 0
 120 – 4RB = 0
120 – 4RB = 0
 RB =
RB = 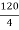
RB = 30 kN 
Question 5) Find the support reactions of a given loading for Beam.
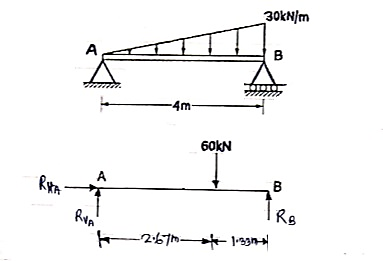
Answer 5)
 Resolving forces horizontally
Resolving forces horizontally
∑fX = 0
RHA = 0
Resolving forces vertically
∑fY = 0
RVA – 60 + RB = 0
RVA + RB = 60 ----------------- (1)
Taking moments at A
∑ MA = 0
(RVA × 0) + (60 × 2.67) – 4RB = 0
160.2 – 4RB = 0
 Put in equation (1)
Put in equation (1)
We get
RVA + 40 = 60
RVA = 60 – 40
RVA = 20 kN 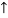
Question 6) Find the support reactions for given beam.
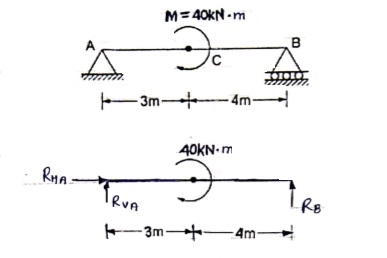
Answer 6)
 Resolving force horizontally
Resolving force horizontally
∑fX = 0
RHA = 0
Resolving forces vertically
∑fy = 0
RVA + RB = 0 ------- (1)
Taking moments at A
 40 – 7RB = 0
40 – 7RB = 0
 RB =
RB = 
RB = 5.71 kN 
Put above value in equation (1)
RVA + RB = 0
RVA + 5.71 = 0
RVA = - 5.71 kN
RVA = 5.71 kN 
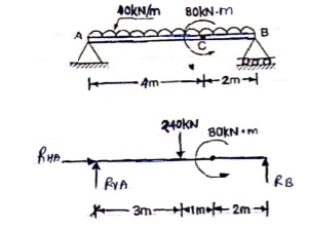 Question 7)
Question 7)
Answer 7)
Resolving forces horizontally
∑fX = 0
RHA = 0
Resolving forces vertically
∑fy = 0
RVA – 240 + RB = 0
RVA + RB = 240 ----------- (1)
Taking moment at A
∑ MA = 0
(240 × 3) – 80 – 6RB = 0
640 – 6RB = 0
RB = 106.67 kN 
Put this value in equation (1)
RVA + RB = 240
RVA = 240 – 106.67
RVA = 133.33 kN 
Question 8) Find support reactions for the loading as shown below:
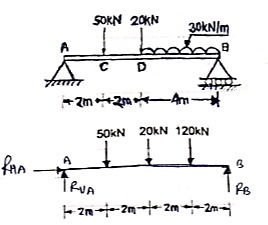
Answer 8)
 Resolving forces vertically
Resolving forces vertically
∑fy = 0
RVA – 50 – 20 – 120 + RB = 0
RVA + RB = 190 ---- (1)
Resolving forces horizontally
∑fX = 0
RHA = 0
Taking moments at A
∑ MA = 0
(50 × 2) + (20 × 4) + (120 × 6) – 8RB = 0
100 + 80 + 720 – 8RB = 0
900 – 8RB = 0
RB – 112.5 kN 
Put above value in equation (1)
RVA + 112.50 = 190
RVA = 77.5 kN 
Question 9) Find the reactions at support A & B.
Answer 9)
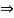 Resolving forces vertically
Resolving forces vertically
∑fy = 0
RVA – 30 – 80 + RB = 0
RVA + RB = 110 ------ (1)
Resolving forces horizontally
∑fX = 0
RHA = 0
Taking moments at A
∑ MA = 0
(30 × 0.6) + [80 × (4.07 + 0.6)]- (6 × RB) = 0
18 + 373.6 – 6RB = 0
6RB = 391.6
RB= 65.267 kN
Put above value in equation (1)
RVA + RB = 110
RVA + 65.267 = 110
RVA = 110 – 65.267
RVA = 44.733 KN
Question 10) Find support reactions at A & B
Answer 10)
 Resolving forces vertically
Resolving forces vertically
∑fy = 0
RVA – 8 – 18 – 5 – 20 + RB = 0
RVA + RB = 51 ------ (1)
Resolving forces horizontally
∑fX = 0
RHA = 0
Taking moments at A
∑ MA = 0
(8 × 2) + (18 × 3.5) + (5 × 5 ) + (20 × 8.67) – 10RB = 0
16+ 63 + 25 + 173.4 – 10RB = 0
277.4 – 10RB = 0
RB = 27.74 kN
Put above value in equation (1)
RVA = 51 – 27.74
RVA = 23.26 kN
Question 11) Explain the difference between centre of gravity and centroid?
Answer 11)
Difference Between Center of Gravity and Centroid | |
Center of Gravity | Centroid |
The point where the total weight of the body focuses upon | It is referred to the geometrical center of a body |
It is the point where the gravitational force (weight) acts on the body | It is referred to the center of gravity of uniform density objects |
It is denoted by g | It is denoted by c |
Center of Gravity in a uniform gravitational field is the average of all points, weighted by local density or specific weight | The centroid is a point in a plane area in such a way that the moment of area about any axis throughout that point is 0 |
It is a physical behaviour of the object, a point where all the weight of an object is acting | It is a geometrical behaviour. It is the center of measure of the amount of geometry. |
Question 12: Find the centroid of the triangle whose vertices are A(2, 6), B(4, 9), and C(6,15).
Answer 12) Solution:
Given:
A(x1, y1) = A (2, 6)
B(x2, y2) = B (4, 9)
C(x3, y3) = C (6, 15)
We know that the formula to find the centroid of a triangle is = ((x1+x2+x3)/3, (y1+y2+y3)/3)
Now, substitute the given values in the formula
Centroid of a triangle = ((2+4+6)/3, (6+9+15)/3)
= (12/3, 30/3)
= (4, 10)
Therefore, the centroid of the triangle for the given vertices A(2, 6), B(4,9), and C(6,15) is (4, 10).