UNIT-5

WAVE OPTICS

Q 1) Discuss Rayleigh criterion of resolution?

A1)

Limit of Resolution:

The minimum distance between two points on the object so that their images (as produced by the optical instrument) are just seen as separate from each other is called limit of resolution of the optical instrument.

Statement: Two sources are resolvable by an optical instrument when the central maximum of one diffraction pattern falls over the first minimum of the other diffraction pattern and vice versa.

Let us consider the resolution of two wavelengths by a grating. When the difference in wavelengths is smaller and such that the central maximum of the wavelength coincides with the first minimum of the other as shown in figure, then the resultant intensity curve is as shown by the thick curve. The curve shows a distinct dip in the middle of two central maxima. Thus the two wavelengths can be distinguished from one another and according to Rayleigh they are said to be “Just Resolved”.

If the difference in wavelengths is such that their principal maxima are separately visible, then there is a distinct point of zero intensity in between the two wavelengths. Hence according to Rayleigh they are said to be “Resolved”.

When the difference in wavelengths is so small that the central maxima corresponding to two wavelengths come still closer as shown in figure, then the resultant intensity curve is quite smooth without any dip. This curve is as if there is only one wavelength somewhat bigger and stronger.

Hence according to Rayleigh the two wavelengths are “Not Resolved”.

Thus the two spectral lines can be resolved only up to a certain limit expressed by Rayleigh Criterion.

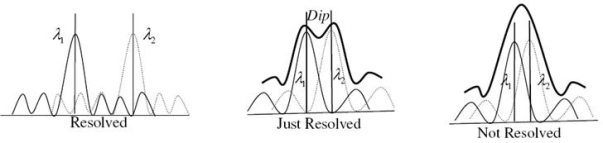
Figure 4: Rayleigh Criterion

Q 2)  Derive expression for resolving power of grating?

A 2)

It is defined as the capacity of a grating to form separate diffraction maxima of two wavelengths which are very close to each other

It is measured by λ/dλ where dλ is the smallest difference in two wavelengths which are just resolvable by grating and λ  is the wavelength of either of them or mean wavelength.

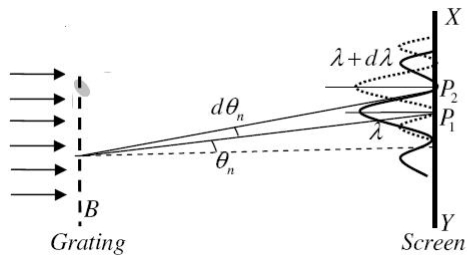
Figure 6: Resolving Power of Diffraction Grating

Let AB represent the surface of a plane transmission grating having grating element (e+d) and N total number of slits.

Let a beam of light having two wavelengths by λ and dλ is normally incident on the grating. Let P1 is nth primary maximum of a spectral line of wavelength λ  at an angle of diffraction  and P2 is the nth primary maximum of wavelength λ+dλ at diffracting angle θ+dθ.

According to Rayleigh criterion, the two wavelengths will be resolved if the principal maximum λ+dλ of nth order in a direction θ+dθ falls over the first minimum of nth order in the same direction θ+dθ.

Let us consider the first minimum of l of nth order in the direction θ+dθ as below.

The principal maximum of   in the θ direction  is given by

(e+d)sinθ =nλ          ………………(1)

The equation of minima is

N(e+d)sinθ = mλ      ………………(2)

Where m takes all integers except 0, N, 2N, …, nN, because for these values of m, the condition for maxima is satisfied.

Thus first minimum adjacent to nth principal maximum in the direction θ+dθ can be obtained by substituting the value of ‘m’ as (nN+1).

Therefore, the first minimum in the direction of θ+dθ is given by

N(e+d)sin(θ+dθ) = (nN+1)λ 

(e+d)sin(θ+dθ) = (n+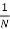)λ       ………………(3)

The principal maximum of  λ+dλ in direction θ+dθ is given by

(e+d)sin(θ+dθ) = n(λ+dλ)        ………………(4)

Dividing eqn(3) by equation (4), we get

(n+)λ =  n(λ+dλ) 

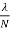nλ + = nλ + ndλ

= ndλ

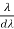  =  nN                                                                                                           

Resolving Power =    =  nN      ………………(5)

Thus the resolving power is directly proportional to

(i) The order of the spectrum ‘n’

(ii) The total number of lines on the grating ‘N’.

Q 3) Write a note on diffraction grating?

A3)

A set of large number of parallel slits of same width and separated by opaque spaces is known as diffraction grating.

Diffraction gratings are much more effective than prisms for dispersing light of different wavelengths so they are used almost exclusively in instruments designed to detect and identify characteristic spectral lines. There is nothing mysterious about these devices.

Fraunhofer used the first grating consisting of a large number of parallel wires placed side by side very closely at regular separation. Now the gratings are constructed by ruling the equidistance parallel lines on a transparent material such as glass with fine diamond point. The ruled lines are opaque to light while the space between the two lines is transparent to light and act as a slit.

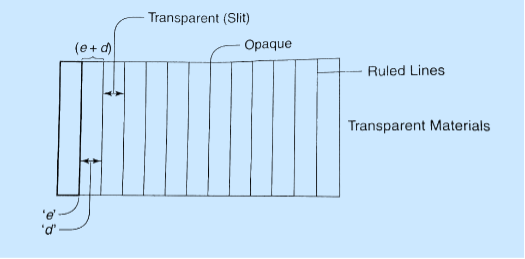
Let ‘e’ be the width of line and ‘d’ be the width of the slit.

Then (e + d) is known as grating element.

If N is the number of lines per inch on the grating then

(𝑒+𝑑)=1𝑖𝑐ℎ=2.54𝑐𝑚

(𝑒+𝑑)=2.54𝑐𝑚/𝑁

Commercial gratings are produced by taking the cost of actual grating on a transparent film like that of cellulose acetate. Solution of cellulose acetate is poured on a ruled surface and allowed to dry to form a thin film, detachable from the surface. This film of grating is kept between the two glass plates.

Q 4) A parallel beam of monochromatic light of wavelength 500nm is inclined normally on a plane diffraction grating 4000 per centimetre. Calculate the angle of diffraction for first-order principal maximum.

A 4)

Given: number of lines per cm =4000

Grating element e+b = 1/4000 cm = 2.5 x 10-6m

λ=500 nm

Order n =1

(e+b) sinθ =nλ

Sinθ = nλ / (e+b)

Sinθ = 1 x 500 x 10-9 / 2.5 x 10-6

Sinθ =0.2

θ =sin-1 0.2

θ = 11.5o

Q 5) A grating containing 4000 slits per centimetre is illuminated with a monochromatic light and produces the second-order bright line at a 30° angle. What is the wavelength of the light used? (1 Å = 10-10 m)

A 5)

The distance between slits (d) = 1 / (4000 slits / cm) = 0.00025 cm = 2.5 x 10-4 cm = 2.5 x 10-6 meters

Order (n) = 2

Sin 30o = 0.5

1 Å = 10-10 m

Wavelength of the light (λ) =?

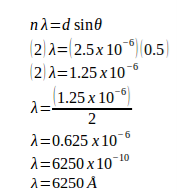
Q 6) A monochromatic light with a wavelength of 2.5x10-7 m strikes a grating containing 10,000 slits/cm. Determine the angular positions of the second-order bright line.

Solution:

Given

The distance between slits (d) = 1 / (10,000 slits / cm) = 0.0001 cm = 1 x 10-4 cm = 1 x 10-6 m

Order (n) = 2

Wavelength (λ) = 2.5 x 10-7 m

Angle (θ)

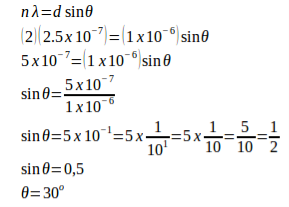
Q 7) A monochromatic light with a wavelength of 500 nm (1 nm = 10-9 m) strikes a grating and produces the second-order bright line at a 30° angle. Determine the number of slits per centimetre.

A 7)

Wavelength (λ) = 500.10-9 m = 5.10-7 m

θ = 30o

n = 2

Distance between slits:

d sin θ = n λ

d (sin 30o) = (2)(5.10-7)

d (0.5) = 10.10-7

d = (10.10-7) / 0.5

d = 20.10-7

d = 2.10-6 m

Number of slits per centimetre:

x = 1 / d

x = 1 / 2.10-6 m

x = 0.5.106 / 1 m

x = 0.5.106 / 102 cm

x = 0.5.104 cm

x = 5.103 /cm

x = 5000 /cm

Q 8) A monochromatic light with a wavelength of 500 nm (1 nano = 10-9) strikes a grating and produces the fourth-order bright line at a 30° angle. Determine the number of slits per centimetre.

A 8)

Wavelength (λ) = 500.10-9 m = 5.10-7 m

θ = 30o

n = 4

Distance between slits:

d sin θ = n λ

d (sin 30o) = (4)(5.10-7)

d (0.5) = 20.10-7

d = (20.10-7) / 0.5

d = 40.10-7

d = 4.10-6 m

Number of slits per centimetre:

x = 1 / 4.10-6 m

x = 0.25.106 / m

x = 0.25.106 / 102 cm

x = 0.25.104 / cm

x = 25.102 per  cm

x = 2500 per cm

Q 9) A grating has 1100 lines ruled on it. What is the difference between the two wavelengths that just appear resolved in the first-order spectrum in the region of wavelength λ = 660 nm?

A 9)

N = 1100 nm

λ = 660 x 10-9 m

n = 1

R = λ /dλ =Nn

dλ = λ /Nn

dλ = 660 x 10-9 / 1100 x 10-9 x 1

dλ = 0.6 x 10-9 m

Q 10) Explain the term optical activity and specific rotation?

A 10)

Optical Activity

The optical activity was first observed in quartz crystals in 1811 by a French physicist, François Arago. Louis Pasteur was the first to recognize that optical activity arises from the dissymmetric arrangement of atoms in the crystalline structures or in individual molecules of certain compounds.

Optical activity, the ability of a substance to rotate the plane of polarization of a beam of light that is passed through it. (In plane-polarized light, the vibrations of the electric field are confined to a single plane.

The intensity of optical activity is expressed in terms of a quantity, called specific rotation, defined by an equation that relates the angle through which the plane is rotated, the length of the light path through the sample, and the density of the sample (or its concentration if it is present in a solution). Because the specific rotation depends upon the temperature and upon the wavelength of the light, these quantities also must be specified. The rotation is assigned a positive value if it is clockwise with respect to an observer facing the light source, negative if counterclockwise. A substance with a positive specific rotation is described as dextrorotatory and denoted by the prefix d or (+); one with a negative specific rotation is levorotatory, designated by the prefix l or (-).

Optical activity is the ability of a chiral molecule to rotate the plane of plane-polarised light, measured using a polarimeter.  A simple polarimeter consists of a light source, polarising lens, sample tube, and analyzing lens.

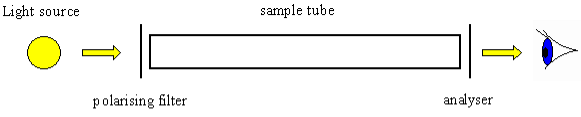
Figure 31

When light passes through a sample that can rotate plane polarised light, the light appears to dim because it no longer passes straight through the polarising filters.  The amount of rotation is quantified as the number of degrees that the analyzing lens must be rotated by so that it appears as if no dimming of the light has occurred.
 

Measuring Optical Activity

When rotation is quantified using a polarimeter it is known as an observed rotation, because rotation is affected by path length (l, the time the light travels through a sample) and concentration (c, how much of the sample is present that will rotate the light).  When these effects are eliminated a standard for comparison of all molecules is obtained, the specific rotation, [a].

[a] = 100a / cl    when concentration is expressed as g sample /100ml solution

Enantiomers will rotate the plane of polarisation in exactly equal amounts (same magnitude) but in opposite directions.

As already discussed  Dextrorotary designated as d or (+), clockwise rotation (to the right) Levorotary designated as l or (-), anti-clockwise rotation (to the left).

If only one enantiomer is present a sample is considered to be optically pure.  When a sample consists of a mixture of enantiomers, the effect of each enantiomer cancels out, molecule for molecule.

For example, a 50:50 mixture of two enantiomers of a racemic mixture will not rotate plane polarised light and is optically inactive.  A mixture that contains one enantiomer excess, however, will display a net plane of polarisation in the direction characteristic of the enantiomer that is in excess.

Q 11) What is the difference between Fresnel’s diffraction and Fraunhofer's diffraction?

A 11)

Q 12) What is dispersive power for grating?

A 12)

The dispersive power of a grating is defined as the ratio of the difference in the angle of diffraction of any two neighbouring spectral lines to the difference in the wavelength between the two spectral lines.

Or

It can also be defined as the diffraction in the angle of diffraction per unit change in wavelength.

The diffraction of the nth order principal maximum for a wavelength λ is given by the equation,

(a + b) sin θ = nλ                          (1)

Differentiating this equation with respect to θ and λ

a + b is constant and n is constant in a given order.

(a + b) cos θ dθ = n dλ

     (2)

In equation (2) dθ/dλ is the dispersive power,

n is the order of the spectrum,

N’ is the number of lines per cm of the grating surface and

θ is the angle of diffraction for the nth order principal maximum of wavelength λ.

From equation (2), it is clear, that the dispersive power of the grating is directly proportional to the number of lines per cm and inversely proportional to cos θ.

Thus, the angular spacing of any two spectral lines is double in the second-order spectrum in comparison to the first order. Secondly, the angular dispersion of the lines is more with a grating having a larger number of lines per cm. Thirdly, the angular dispersion is minimum when θ = 0. If the value of θ is not large the value of cosθ can be taken as unity approximately and the influence of the factor cosθ in the equation (2) can be neglected.

Neglecting the influence of cosθ, it is clear that the angular dispersion of any two spectral lines (in a particular order) is directly proportional to the difference in wavelength between the two spectral lines. A spectrum of this type is called a normal spectrum.

If the linear spacing of two spectral lines of wavelength λ and λ + dλ is dx in the focal plane of the telescope objective or the photographic plate, then,

Dx = fdθ

Where ƒ is the focal length of the objective. The linear dispersion

The linear dispersion is useful in studying the photographs of a spectrum.

Q 13)  Discuss construction and working Laurent’s half shade Polarimeter?

A 12)

Laurent’s half shade Polarimeter

Polarimeter – it is an optical instrument used to measure the angle of rotation of plane-polarized light when it is passed through an optically active substance.

By measuring the angle, the specific rotation of an optically active substance can be determined.

Two types of polarimeters are generally used in the laboratory nowadays Laurent’s Half Shade Polarimeter and Biquartz  Polarimeter.  We will discuss Laurent’s Half Shade Polarimeter.

Construction

• S is the source of monochromatic light placed at the focus of convex
• Just after the convex lens, there is a Nicol Prism P is placed. This prism acts as a polariser.
• H is a half shade device that divides the field of polarised light emerging out of the Nicol P into two halves generally of unequal brightness.
• T is a glass tube in which an optically active solution is filled.
• The light after passing through T is allowed to fall on the analyzing Nicol A which can be rotated about the axis of the tube. (Don’t get confused here, we already discussed in the previous section that Nicol prism acts as a polariser as well as analyser).
• The rotation of analyser can be measured with the help of a scale.

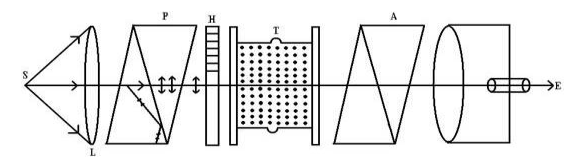
Figure 32

Laurent’s half shade polarimeter is shown in Figure 32

Working

To understand the working of this device we have to know the need for a Laurent half shade device. For this, we assume that the half shade device is not present.

In this basic setup (without the half-shade A) you are looking for the maximum and minimum brightness, which then tells you that the analyzer A is precisely aligned with the output rotation.

When the tube is empty placed the analyzer in such a position that the view of the field is completely dark. Note the position of the analyzer with the help of a circular scale.

Now filled the tube with an optically active solution and it is set in its proper position. The optically active solution rotates the plane of polarization of the light emerging out of the polariser P by some angle. So the light is transmitted by analyzer A and the field of view of the telescope becomes bright.

Now the analyzer is rotated by a finite angle so that the field of view of the telescope again becomes dark. This will happen only when the analyzer is rotated by the same angle by which the plane of polarization of light is rotated by the optically active solution.

The position of the analyzer is again noted. The difference between the two readings will give you the angle of rotation of the plane of polarization.

But here the difficulty associated with this procedure that when analyzer is rotated for the total darkness, then it is attained gradually and hence it is difficult to find the exact position correctly for which complete darkness is obtained.

Figure 33

Laboratory Set-Up of Polarimeter

To overcome the above difficulty half shade device is introduced between polariser P and glass tube T.

Half Shade Device

• It consists of two semi-circular plates ACB and ADC.
• One-half ACB is made of glass while the other half is made of quartz.
• Both the halves are joined together.
• The quartz is cut parallel to the optic axis.
• The thickness of the quartz is selected in such a way that it introduces a path difference of  ‘A/2 between ordinary and extraordinary rays.
• The thickness of the glass is selected in such a way that it absorbs the same amount of light as is absorbed by quartz half.

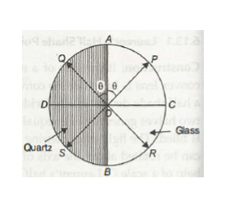
Figure 34

• Let us consider that the vibration of polarisation is along with OP. On passing through the glass half the vibrations remain along with OP. But on passing through quartz half of these vibrations will split into o  and e-components.
• The e-components are parallel to the optic axis while the o-component is perpendicular to the optic axis.
• The o-component travels faster in quartz and hence an emergence 0-component will be along with OD instead of along OC.
• Thus components OA and OD will combine to form a resultant vibration along OQ which makes the same angle with the optic axis as OP.

Now if the Principal plane of the analyzing Nicol is parallel to OP then the light will pass through a glass half passable. Hence glass half will be brighter than the quartz half or we can say that the glass half will be bright and the quartz half will be dark. Similarly if the principal plane of analysing. Nicol is parallel to OQ then quartz half will be bright and glass half will be dark.

When the principal plane of analyzer is along AOB then both halves will be equally bright. On the other hand, if the principal plane of analyzer is along with DOC. Then both the halves will be equally dark.

Thus it is clear that if the analysing Nicol is slightly disturbed from DOC then one half becomes brighter than the other. Hence by using a half shade device, one can measure the angle of rotation more accurately.

Mathematically

The specific rotation is given by S=θ/lC where l is the length of the tube and C is concentration.
 

Q 13) Write the point of Differences between Positive Crystal and Negative Crystal

A 13)

Difference between Positive Crystal and Negative Crystal

Q 14) State and explain Malus Law?

A 14)

Plane polarized light is obtained by passing unpolarized light through a polarizer. When plane polarized light from the polarizer is passed through the analyser, the intensity of polarised light transmitted through the analyser varies as the square of the cosine of the angle between the plane of transmission of the analyser and the plane of the polarizer. This is known as Malus law.

It states that "The intensity of the polarized light transmitted through the analyser is proportional to cosine square of the angle between the plane of transmission of the analyser and plane of transmission of the polarizer."

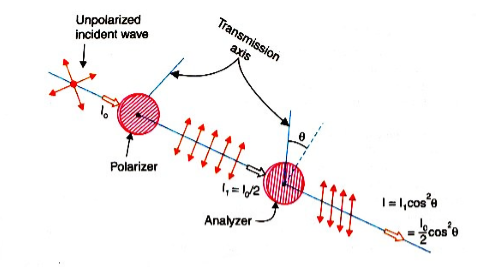
Figure 15: Malus law

Let I0 is the intensity of unpolarized light. The intensity of polarized light from the polarizer is I0/2.

Take I1=I0/2. This plane polarized light then passes through the analyser. Let E is the aptitude of vibration and θ  is the angle between this vibration and transmission axis of an analyser.

E resolves into two components

(i)                Ey parallel to the plane of transmission of the plane of analyser and

(ii)              Ex perpendicular to the plane of analyser.

Now, Ey component is only transmitted through the analyser.

Ey = Ecosθ

Intensity of light for this component:

I = E2cos2θ = I1 cos2θ = I0/2 cos2θ

If, (i) θ = 0°, then axis are parallel I= I1

(ii) θ = 90°, then axis are perpendicular I= 0

(iii) θ = 180°, then axis are parallel I= I1

(iv) θ= 270°, then axis are perpendicular I= 0

Thus, there are two positions of maximum intensity and two positions of zero intensity when we rotate the axis of the analyser with respect to that of the polarizer.

Q 15) Explain Production of Polarized Light by Reflection?

A 15)

Brewster’s law can be used to polarise the light by reflection. When unpolarized light is incident on the boundary between two transparent media, the reflected light is polarised with its electric vector perpendicular to the plane of incidence when the refracted and reflected rays make a right angle with each other.

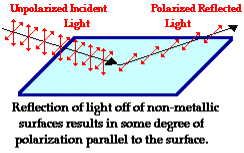
Thus we have seen that when the reflected wave is perpendicular to the refracted wave, the reflected wave is totally polarised. The electric field vectors of all unpolarized light may be resolved into two components as follows;

1. Tangential component- in the plane of incidence.
2. Normal components – in the plane perpendicular to the plane of incidence.

The angle of incidence, in this case, is called Brewster’s angle

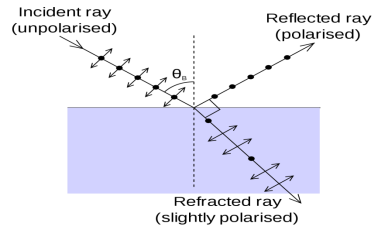
Figure 17

If the angle of incidence θi is varied, the reflected coefficient ‘r’ for the em waves corresponding to the tangential component will vary as shown in the figure. As θi increases r will decrease.

At θi = Brewster angle (about 57° for glass) r will be zero and no reflection of the tangential component will take place.

The reflected beam will contain light waves having only the normal component for the electric field as shown in the figure that is the reflected light will be plane-polarized with the oscillations of electric field vector normal to the plane of incidence.

Cause of Polarization by Reflection

The em waves are generated because of the oscillation of electric charge. The electric field vectors of the transmitted ray act on the electrons in the dielectric.

Since the intensity of em wave emitted by the oscillating charge is maximum in a direction normal to the plane of oscillation, and zero along the line of oscillations for the plane-polarized light having electric field vector in the plane of incidence, there will be no emission of em waves in a direction normal to the reflected ray. In the figure, the direction along which no emission should occur is called a zero intensity line. Let the angle between the reflected ray and the zero intensity line be 𝛿  the angle between the reflected and transmitted ray is  π/2 + 𝛿  suppose we arrange the angle of incidence such that

n=sinθi / sinθr

n=sinθi / sin(π/2 – θi)

n=sinθi / cosθi

n=tan θi

That is possible when

θi + θr = π/2  according to Brewster’s law

From figure

θi + θr+ π/2 +   = π

As  θi + θr = π/2  

So  π/2+ π/2 +   = π

= 0

The line of zero intensity and the reflected ray will coincide. Thus there will be no reflection of light when the angle of incidence is equal to Brewster’s angle.

Thus the unpolarized light can be polarized by reflection as the reflected beam will contain light waves having electric field vector oscillating along normal to the plane of the incidence only.