Unit - 5

Curve tracing

Q1) What do you understand by curve tracing? Also give important definitions used in tracing of a curve.

A1)

A picture explains more clearly than words and numbers. A curve which is the image of functional relationship gives us a lot information about the relation. We can get information analyzing the equation itself but the associated curve is often easy and understandable.

Let’s study about how to trace the curves of various equations in different forms like Cartesian, parametric and polar.

Important definitions for curve tracing

Here are some terms that we use in curve tracing:

1. Double point- when a curve passes two times through this point is known as double point.

2. Node- a double point at which two real tangents can be drawn.(tangents should not be coincide)

3. Cusp -  a double point is a cusp when two tangents are coincide on it.

Q2) Define vertical asymptote.

A2)

A line x = a which is straight is a vertical asymptote of the graph of the function y = f(x) if atleast one of the following condition does it follow-

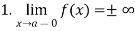
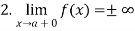
Q3) Determine the vertical asymptote of the function-

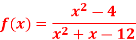
A3)

Here we have-

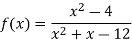
We can write the given function as-

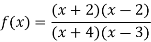
We can notice here as x gets closer to 3 from the left, then the value of the function gets smaller, approaching -∞ and x gets closer 3 from the right, then the value of the function gets larger and approaches +∞.

So that x = 3 is a vertical asymptote same as x = -4 is another vertical asymptote.

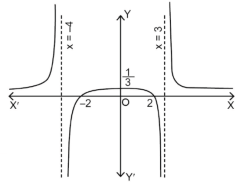
Q4) Find the horizontal asymptote of the function-

A4)

In order to find the horizontal asymptote-

=

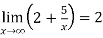
Hence the horizontal asymptote is y = 2.

Q5) Find the horizontal asymptote of the function-

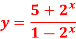
A5)

Find the limits-

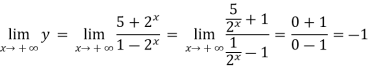
And

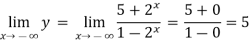
Hence the horizontal asymptotes are y = -1 and y = 5.

Figure will be as follows of the asymptotes of the given function-

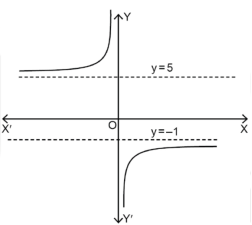
Q6) Find the slant asymptotes of 

A6)

We have,

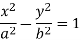
Which can be written as-

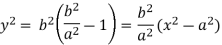
The value of ‘m’ will be-

=     = 

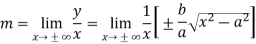
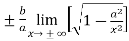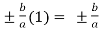
Now we can find ‘c’ as –

c = = =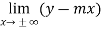

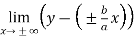= 0

Hence the slant asymptotes are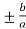

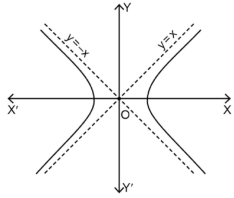
Q7) Find the slant asymptote of the function f(x) = x + .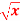

A7)

Find the value of m-

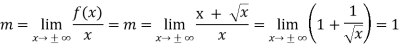
Hence the y = x + c,

Now find c-

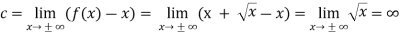
Here c must not be infinite.

So we can say that f does not have a slant asymptote at ∞.

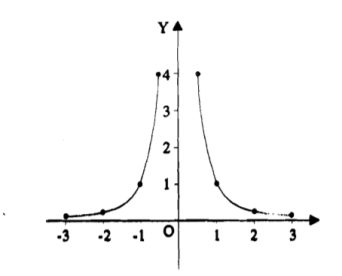
Q8) Trace the curve y = 1/x².

A8)

As we can see y- coordinates of the curve cannot be negative. So the                                              curve must be above x-axis. The curve is also symmetric about y-axis so we can draw the graph only in single side.

Here, we will find the first and second derivatives-

So, dy/dx= -2/x³ and d²y/dx² = 6/ x⁴,  here  dy/dx <0 for all x>0 so we can say that the function is non- increasing so the graph falls as we increase x.

Also second derivative is also non zero so there are no point of inflection.

Here the curve is x²y=1 (rewritten), here both the axes are asymptotes of the curve.

Here is the figure of the curve-:

Q9) Trace the curve y= (x+2)²(x-1).

A9)

Here first we check the slant asymptotes (there is no vertical asymptote)

So, p =

=

=

=

=  ) = +

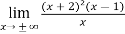
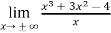
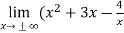Since p is infinity then the function has no slant asymptotes.

Now we will find the point of intersection with axes:

Y(0)= -4;

Put y(x)= 0,     (x+2)²(x-1)= 0

We get by solving,       X1= -2,     x2= 1

Now calculate the first derivative,

Here we will find stationary point by putting first derivative equal to zero:

3x(x+2) = 0

X1 = 0,   x2 = -2

X= -2 is maximum and x= 0 is minimum point.

The value of the function at these points will be:

Y= -4, 0

Now we will find second derivative:

Now, d²y/dx² = 6x+6,

So the function is strictly upward for:

X<-1,

And strictly downward for

x>-1,

Hence x= -1 is the inflection point

We get    y(-1)= -2

Here is the figure of curve:

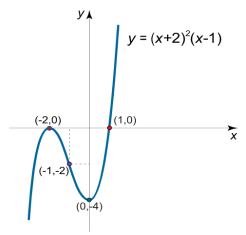
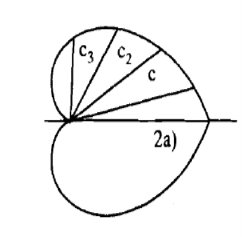
Q10) Trace the curve r = a(1+ cos.

A10)

Here we can see clearly cos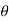 = cos(-), hence the curve is symmetric about initial line.

Since -1, the curve lies inside the circle r = 2a

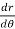= -asin , when  0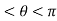, thus r decreases as 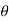increases in the interval 0 to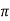

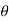	0
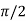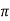r	2a	A	0

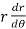= - cot (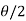) = tan(+ 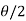),      this shows the angle between r and 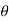and the tangent is 0 or 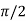according to  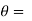 0 or 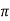. Hence the line joining a point on the curve to the origin is orthogonal to the tangent when 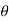= 0 and coincides with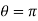

From the above results, we can easily draw the graph above the initial line

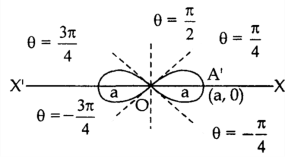
Q11) Trace the curve  .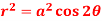

A11)

Symmetry about initial line- Put r = 0,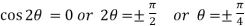

Hence the straight line 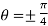are the tangents to the curve at the pole.

Values of ‘r’ as changes from 0 to π-

Table for the values of ‘r’-

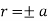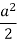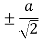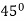	0	0
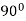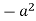		Imaginary
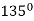	0	0

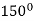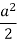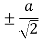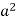Here according to the table the curve does not exist for the value lying between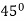 
to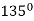

The figure will be as follows of the curve-

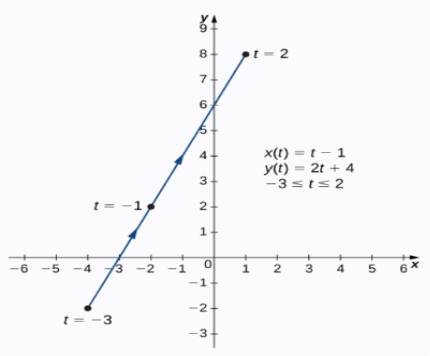
Q12) Trace the curve of the following parametric equations:

x(t) = t-1 ,     y(t) = 2t+4         -3<

A12)

Here we will create the table for t, x(t) and y(t), t is independent variable in both case,

Here value of t lies between -3 to 2.

By plotting these set of point we get a curve as below.

Arrows in the graph indicates the orientation of the graph.