Question bank
Unit 1
Q.1 List the conditions a wave function must satisfy in order to solve the Schrödinger equation.
Answer:
1. The wave function ψ must be continuous. All its partial derivatives must also be continuous.
This makes the wave function “smooth”.
2. The wave function ψ must be quadratically integrable. This means that the integral
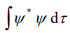must exist.
3. Since
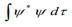
is the probability density, it must be single valued?
4. The wave functions must form an orthonormal set. This means that • the wave functions must be normalized.
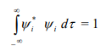
• the wave functions must be orthogonal.
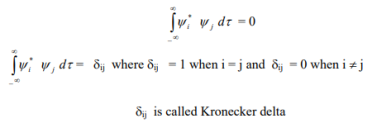
5. The wave function must be finite everywhere.
6. The wave function must satisfy the boundary conditions of the quantum mechanical system it represents.
Q.2 State and Derive the time independent Schrodinger wave equation?
Answer:
According to de-Broglie theory, for a particle of mass ‘m’, moving with a velocity ‘v’, the wavelength associated with it is
λ=h/p
The wave equation for a de-Broglie wave can be written in complex notation as:
…………1
Where, A is the amplitude, ω is angular frequency and k is the wave vector.
Differentiate equation (1) with respect to ‘t’ twice, we get
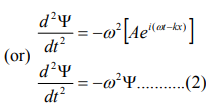
We have differential equation for the traveling wave as,
……….3
Where, y is displacement and ‘v’ is velocity of wave.
By analogy, we can write the wave equation for de-Broglie wave associated with the motion of a free particle as,
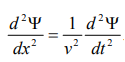………4
This represents the de-Broglie wave propagating along x-direction with a velocity ‘v’ and ‘Ψ’ is the displacement.
From equation (2) and (4),
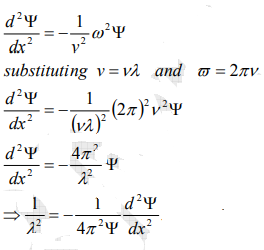…………..5
The kinetic energy of a moving particle of mass ‘m’ and velocity ‘v’ is given by
But we have from equation (1), p = (h/λ)
Substitute for (1/ λ)2 from equation (5),
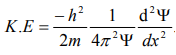…………….6
Let there be a field where the particle is present. Depending on its position in the field, the particle will possess certain potential energy. Then we can write:
Total energy = Kinetic energy + Potential energy
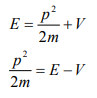
From equation (6), we can write
This is the time independent Schrödinger’s wave equation in one-dimension.
Q. 3 Explain born interpretation?
Answer:
Let’s say you have a function of x, which we will call Ψ(x), and we can plot this wavefunction on a graph. The y axis represents the amplitude of the wave. If you square this, or write (amplitude of the wave) ^2 this is equal to the intensity of the wave.
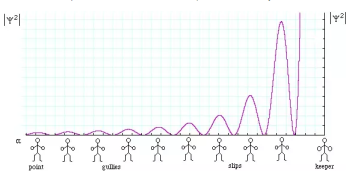
However, the problem is that the wavefunction is complex, it is not necessarily real, i.e. it may have a part to it that is imaginary. Thus, instead of using Ψ^2, which would be impossible to work with, you instead use the complex conjugate of Ψ, which we call Ψ* by changing the sign of the imaginary part, and making it possible to work with mathematically. So now, to get the intensity of the wave, we no longer will use Ψ^2, but instead will use ΨΨ*, which is the absolute magnitude of Ψ^2. We can also plot Ψ(x)Ψ*(x) vs. x.
Let’s say we take just a segment on the graph between x and (x+dx), and the difference between those two points is just dx.
The Born Interpretation says that the wavefunction Ψ(x)Ψ*(x)dx will tell us the probability that the particle is located in the region between x and (x+dx). A region with a large value for Ψ(x)Ψ*(x) will have high probability for the particle in that region. We can normalize this function by taking the integral from negative infinity to positive infinity of dx Ψ(x)Ψ*(x) and set that equal to 1, because that area encompasses 100% probability of where the particle must be.
Q.4 Calculate the minimum energy of free electron trapped in a one-dimensional box of width 0.2 nm. (Given, h = 6.63 –10–34 J-S and electron mass × 9.1 × 10 –31 kg)
Answer:
One-dimensional box of width, L = 0.2 nm = 2 × 10–10 m
Minimum energy of the electron, E1 =?
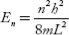
Q.5 What is Quantum mechanics? Give its postulates?
Answer:
Quantum mechanics, science dealing with the behaviour of matter and light on the atomic and subatomic scale. It attempts to describe and account for the properties of molecules and atoms and their constituents—electrons, protons, neutrons, and other more esoteric particles such as quarks and gluons. These properties include the interactions of the particles with one another and with electromagnetic radiation (i.e., light, X-rays, and gamma rays)
Postulate of quantum mechanics:
1. Associated with any particle moving in a conservative field of force is a wave function which determines everything that can be known about the system
2. With every physical observable q there is associated an operator Q, which when operating upon the wavefunction associated with a definite value of that observable will yield that value times the wavefunction.
3. Any operator Q associated with a physically measurable property q will be Hermitian
4. The set of eigenfunctions of operator Q will form a complete set of linearly independent functions.
5. For a system described by a given wavefunction, the expectation value of any property q can be found by performing the expectation value integral with respect to that wavefunction.
6. The time evolution of the wavefunction is given by the time dependent Schrodinger equation.
Q.6 Discuss the Heisenberg’s uncertainty principle and show how it is introduced in the process of measurement?
Answer: Statement: It is impossible to determine simultaneously both the position and momentum of a quantum mechanical particle moving inside the wave packet with equal accuracy. In any simultaneous determination of the position and momentum of a particle, the product of the corresponding uncertainties inherently present in the measurement is equal to, greater than (h/4π).
Explanation: Consider a quantum particle moving inside a wave packet of width Δx. Then inside the wave packet it is not possible to find the exact position of the particle and any attempt to measure it will have an uncertainty (error) which less than or equal to Δx.
The maximum uncertainty involved in measuring the position of the particle within the wave packet is Δx. Since position cannot be measured accurately, there will be an uncertainty in measuring momentum also. According to Heisenberg’s uncertainty principle, the product of uncertainty involved in the measurement of these two quantities is given by the relation,
Where ∆x = uncertainty in position.
∆p = uncertainty in momentum.
It can be applied to any conjugate physical quantities such as energy and time, angular position and angular momentum etc.
Other forms of Uncertainties are:
Where, ΔE, Δt are the uncertainties in the measurement of energy and time, and ΔL, Δθ are the uncertainties in the measurement of angular momentum and angular position.
Q 7. Drive the relation between Group velocity and phase velocity?
Answer:
For a wave group formed by the superposition of number of waves, the group velocity and phase velocity are given by
For any propagating wave packet –
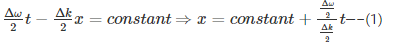
Velocity is the rate of change of displacement given by--
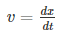
Hence, group velocity is got by differentiating equation (1) with respect to time…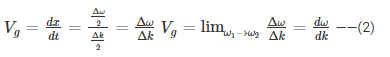
We know that phase velocity is given by
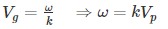
Substituting? = kVp in equation (2) we get-
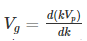
Thus, we arrive at the equation relating group velocity and phase velocity –
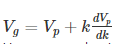
Q 8 Give the Solution of stationary-state Schrodinger equation for one dimensional problem?
Answer:
We will now look at the solutions of a particle of mass m confined to move along the x-axis between 0 to L. This is achieved by making the potential 0 between x = 0 and x = L and V = ∞ for x < 0 and x > L. In quantum mechanics this model is referred to as particle in a box (PIB) of length L.
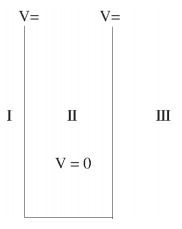
The one-dimensional infinite potential well of length L. It is divided into three regions, with regions I and III having V = ∞ and region II having V = 0
Before we set up and solve the Schrodinger equation let us apply de Broglie’s approach to this problem. De Broglie associates a wave with every material particle traveling with momentum p. The wavelength of the wave is
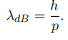
The classical analog of a particle in a box is a string that is fixed at both ends. When such a string is plucked, we know that the amplitude of the oscillations at the fixed ends is zero. In other words, an integer number of half-wavelengths must fit in the length of the box. Applying this idea to the present case, we find that
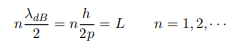
Solving for p we get
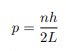
The particle feels no potential energy so all its energy is in the form of kinetic energy. As a result
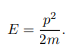
Substituting for p, we get
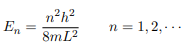
The Schrodinger equation is-
Hψˆ (x) = Eψ(x)
Where V (x) = ∞ in regions I and III and V (x) = 0 in region II. The results are in no way affected if in region II the potential is V because it only has the effect of altering the zero of energy and so without of generality, we will assume that V = 0 within the box. In regions I and III the wave function is identically zero since the potential is infinite so we will only have to consider the solution within the box. We are looking for the solutions to the equation.
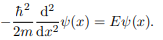
We rewrite this differential equation in the form:
Wave equation
ψ(x) = A cos(kx) + B sin(kx)
The boundary conditions that the wave function should satisfy are
ψ (0) = 0 and ψ(L) = 0. The
First of these conditions implies that A = 0.
The second condition yields
B sin(kL) = 0
Which implies that kL = nπ, n = 1, 2, 3, · · ·
Or in other words k = nπ/L. Because E is related to k we hence obtain that
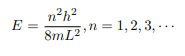
You will notice that the application of the boundary conditions naturally leads to quantization. In addition, observe that we do not consider n = 0 as a possibility because that would imply that the wave function is identically zero for all x or in other words there is no particle! The constant B is yet to be determined. The normalization condition on the wave function yields B.
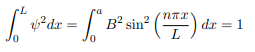
In cases where the wave function is not an eigen function of the operator corresponding to the observable, we will be concerned with the statistical mean of the measured values, averaged over a large number of measurements, the expectation value. The expectation value is defined of an operator is defined a:
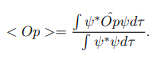
The average momentum is the
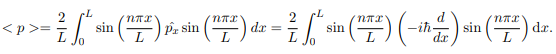
Q 9 Define wave packets and wave function?
Answer:
Wave Packet:
We know that by Heisenberg’s principle, in order to get some localization of the position of a particle, some uncertainty must be allowed in momentum. That means that you must take the initial momentum space wave function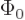 
to be nonzero over at least some small interval of different momentum values 
. Such a combination of component waves is called a wave packet.
The wave function for a typical wave packet is sketched below. The red line is again the real part of the wave function, and the black lines are the envelope enclosing the wave; they equal plus and minus the magnitude of the wave function.
 |
The real part (red) and magnitude or envelope (black) of a wave packet. (Schematic). |
Wave function:
The quantity that characterizes the de–Broglie wave or matter wave is called the wave function. It is usually denoted as Ψ = (x, y, z, t). This gives complete information about the state of a physical system at a particular time.
It is also called the state function and represents the probability amplitude. If ‘Ψ’ is large, the probability of finding the particle is also large and if ‘Ψ’ is small then the probability of finding the particle is small. The wave function gives the likelihood of finding the particle at a given instant and at a given position inside the wave packet.
Q10. What is operator in quantum mechanics?
Answer:
In quantum mechanics, physical observables (e.g., energy, momentum, position, etc.) are represented mathematically by operators. For instance, the operator corresponding to energy is the Hamiltonian operator
 |
|
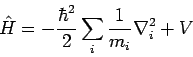Where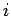 
is an index over all the particles of the system? We have already encountered the single-particle Hamiltonian in equation. The average value of an observable A represented by an operator 
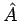for a quantum molecular state 
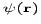is given by the expectation value'' formula
 |
