Module 1
Calculus
- Verify Rolle’s theorem for the function f(x) = x2 for

Solution:
Here f(x) = x2; 
i) Since f(x) is algebraic polynomial which is continuous in [-1, 1]
Ii) Consider f(x) = x2
Diff. w.r.t. x we get
f'(x) = 2x
Clearly f’(x) exist in (-1, 1) and does not becomes infinite.
Iii) Clearly
f(-1) = (-1)2 = 1
f(1) = (1)2 = 1
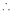 f(-1) = f(1).
f(-1) = f(1).
Hence by Rolle’s theorem, there exist  such that
such that
f’(c) = 0
i.e. 2c = 0
 c = 0
c = 0
Thus  such that
such that
f'(c) = 0
Hence Rolle’s Theorem is verified.
2. Verify Rolle’s Theorem for the function f(x) = ex(sin x – cos x) in 
Solution:
Here f(x) = ex(sin x – cos x); 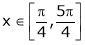
i) Clearly ex is an exponential function continuous for every 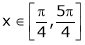 also sin x and cos x are Trigonometric functions Hence (sin x – cos x) is continuous in
also sin x and cos x are Trigonometric functions Hence (sin x – cos x) is continuous in  and Hence ex(sin x – cos x) is continuous in
and Hence ex(sin x – cos x) is continuous in  .
.
Ii) Consider
f(x) = ex(sin x – cos x)
Diff. w.r.t. x we get
f’(x) = ex(cos x + sin x) + ex(sin x + cos x)
= ex[2sin x]
Clearly f’(x) is exist for each  & f’(x) is not infinite.
& f’(x) is not infinite.
Hence f(x) is differentiable in  .
.
Iii) Consider



Also,
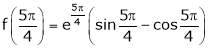
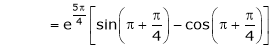

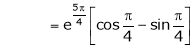


Thus 
Hence all the conditions of Rolle’s theorem are satisfied, so there exist  such, that
such, that
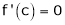
i.e. 
i.e. sin c = 0


But

Hence Rolle’s theorem is verified.
3. Verify the Lagrange’s mean value theorem for

Solution:
Here 
i) Clearly f(x) = log x is logarithmic function. Hence it is continuous in [1, e]
Ii) Consider f(x) = log x.
Diff. w.r.t. x we get,

Clearly f’(x) is exist for each value of 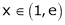 & is finite.
& is finite.
Hence all conditions of LMVT are satisfied Hence at least 
Such that

i.e. 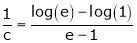
i.e. 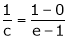
i.e. 
i.e. 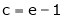
Since e = 2.7183

Clearly c = 1.7183 
Hence LMVT is verified.
4. Verify Cauchy mean value theorems for 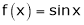 &
& in
in 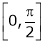
Solution:
Let  &
&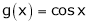 ;
; 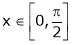
i) Clearly f(x) and g(x) both are trigonometric functions. Hence continuous in 
Ii) Since 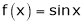 &
&
Diff. w.r.t. x we get,
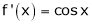 &
&
Clearly both f’(x) and g’(x) exist & finite in 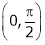 . Hence f(x) and g(x) is derivable in
. Hence f(x) and g(x) is derivable in  and
and
Iii) 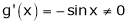

Hence by Cauchy mean value theorem, there exist at least  such that
such that
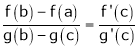
i.e. 

i.e. 1 = cot c

i.e. 
Clearly 
Hence Cauchy mean value theorem is verified.
5.  then
then

Proof:
Here f(x) = tan x
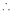 By Maclaurin’s expansion,
By Maclaurin’s expansion,
 … (1)
… (1)
Since
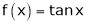
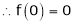
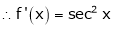








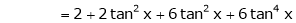
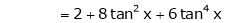



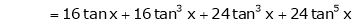

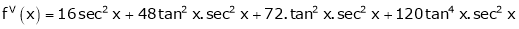
 …..
…..
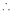 By equation (1)
By equation (1)


6.  Then
Then

Proof:-
Here f(x) = sin hx.
 By Maclaurin’s expansion,
By Maclaurin’s expansion,
 (1)
(1)

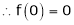

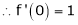
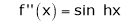



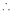 By equation (1) we get,
By equation (1) we get,

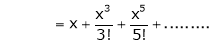
7. Evaluate 0∞ x3/2 e -x dx
Solution: 0∞ x3/2 e -x dx = 0∞ x 5/2-1 e -x dx
= γ(5/2)
= γ(3/2+ 1)
= 3/2 γ(3/2 )
= 3/2 . ½ γ(½ )
= 3/2 . ½ .π
= ¾ π
8. Show that 

Solution : =
= 

= 


= 


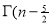 ) .......................
) .......................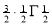
= 
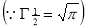
= 

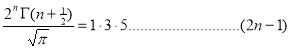
9. Evaluate 
 dx.
dx.
Solution : Let 

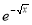 dx
dx
X | 0 | 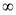 |
t | 0 | 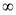 |
Put  or
or 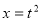 ;dx =2t dt .
;dx =2t dt .
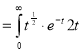 dt
dt
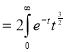 dt
dt

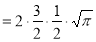
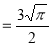
10. Evaluate: I = 02 x2 / (2 – x ) . Dx
Solution:
Letting x = 2y, we get
I = (8/2) 01 y 2 (1 – y ) -1/2dy
= (8/2) . B(3 , 1/2 )
= 642 /15