Question Bank
Unit 2
Que1)The amount of flux present in around magnetic bar was measured at 0.013 weber. If the material has a diameter of 14cm, calculate the flux density.
Sol: Area= r2
r2
Diameter=2r
r=14/2=7cm=0.07m
Area=3.14 x 0.072=0.0154m2
Flux Density B== A=0.013/0.0154=0.843 Tesla
A=0.013/0.0154=0.843 Tesla
Que2) Calculate the radius of the material having flux density of 0.5 T and flux present around the magnetic bar is 0.02T.
Sol: Flux Density B= A
A
A= B=0.02/0.5=0.04 m2
B=0.02/0.5=0.04 m2
Area= r2
r2
r=0.113m
Que3) A wire 2.5m long is bent into a square and into a circle. If the current flowing through the wire is 100 A, find the magnetising force at the centre of the square and the centre of the circle?
Sol: Value of h at centre of square will be H= =
= 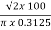 =144 AT/m
=144 AT/m
Value of H at the centre of circle is H=I/2 r
r
=125.6AT/m
Que4) Calculate the magnetising force and flux density at a distance of 4cm from a long straight circular conductor carrying a current of 250A and placed in air?
Sol: H=I/2 r
r
=250/2 x 0.04=994.71 AT/m
x 0.04=994.71 AT/m
B=μoH
=4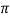 x10-7x994.71=1.25x10-3 Wb/m2
x10-7x994.71=1.25x10-3 Wb/m2
Que5) A closed magnetic circuit of cast steel contains a 6cm long path of cross-sectional area 1 cm2 and a 2cm path of cross sectional area 0.5 cm2. A coil of 200 turns is wound around the 6cm length of the circuit and a current of 0.4A flows. Determine the flux in the 2 cm path, if the relative permeability of the cast steel is 750?
Sol: For 6cm path:
S1 = 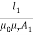 =
=  = 6.37 x 105 /H
= 6.37 x 105 /H
For 2cm path:
S2= 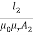 =
= 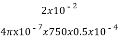 = 4.24 x 105 /H
= 4.24 x 105 /H
Total reluctance S= S1+S2 = 6.37 x 105+4.24 x 105= 10.61 x 105/H
S = mmf/φ
Φ = mmf/S = NI/S = (200x0.4)/( 10.61 x 105) = 7.54x 10-5 Wb
Flux density in the 2cm path B= φ/A = 7.54x 10-5/ = 1.51 T
= 1.51 T
Que6)A laminated soft iron ring of relative permeability 800 has a mean circumference of 600mm and a cross sectional area of 400mm2. A radial air gap of 1mm width is cut in the ring which is wound with 800 turns. Calculate the current required to produce an air gap flux of 0.5mWb if leakage factor is 1.2 and stacking factor 0.9.
Sol: Required Ampere turn =  gSg+
gSg+  iSi
iSi
=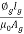 +
+ 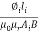
Air gap flux  s=0.5mWb
s=0.5mWb
lg=1mm=1x10-3m
Ag=400mm2=400x10-6
Flux in iron ring  i=1.2x0.5x10-3=0.6mWb
i=1.2x0.5x10-3=0.6mWb
Net cross-sectional area = Ai x stacking factor=400x10-6 x 0.9=360x10-6
Total AT required=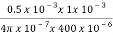 +
+ 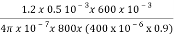 = 994.72
= 994.72
I=A/N=994.72/800=1.24A
Que7) An iron ring has X-section of 4cm2 and a mean diameter of 25cm. An air-gap of 0.3mm has been cut across the section of the ring. The ring is wound with a coil of 220 turns through which a current of 2A is passed. If the total magnetic flux is 0.24mWb, find relative permeability of iron?
Sol: φ=0.24mWb
A=4cm2=4 x 10-4
B=φ/A=0.24 x 10-3/4 x 10-4=0.6Wb/m2
AT for iron ring=H x l=(B/ )/l=(0.6/
)/l=(0.6/ x
x ) x 0.25=1.19 x 105/
) x 0.25=1.19 x 105/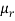
AT for air gap= H x l=( B/ /l=(0.6/
/l=(0.6/ x 0.3x10-3=143.24
x 0.3x10-3=143.24
Total AT required=(1.19 x 105/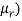 +143.24
+143.24
Total AT provided=220 x 2=440
Therefore,
(1.19 x 105/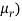 +143.24=440
+143.24=440
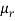 = 400.99
= 400.99
Q8) A ring has a diameter of 20cm and a cross-sectional area of 10 cm2. The ring is made up of semi-circular section of cast iron and cast steel, with each joint having reluctance equal to an air gap of 0.2mm. Find the ampere turns required to produce a flux of 8x10-4Wb. The relative permeability of cast steel and cast iron are 800 and 166 respectively?
Sol: φ = 8x10-4Wb
A= 10 cm2 = 10-3m2
B= Φ/A = 8x10-4/10-3= = 0.8Wb/m2
Air gap
H= B/μo = 0.8/4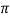 x10-7=6.37x105AT/m
x10-7=6.37x105AT/m
Total air gap length = 2x0.2 =0.4mm=4x10-4m
AT required = H x l = 6.37x105 x 4x10-4 = 255
Cast steel path
H= B/μoμr = 0.8/4 x10-7x800 = 796 AT/m
x10-7x800 = 796 AT/m
Path =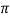 D/2 = 20 x
D/2 = 20 x 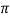 /2 = 31.42mm=0.314m
/2 = 31.42mm=0.314m
AT required = H x l = 796x 0.314 = 250
Cast iron path
H= B/μoμr = 0.8/4 x10-7x166 = 3835 AT/m
x10-7x166 = 3835 AT/m
Path =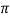 D/2 = 20 x
D/2 = 20 x  /2 = 31.42mm=0.314m
/2 = 31.42mm=0.314m
AT required = 3835x0.314= 1204
Total AT required = 255 + 250 + 1204 = 1709
Q9) An iron ring has a X-section of4cm2 and a mean diameter of 20cm. An air gap of 0.5 mm is cut across the section of the ring. The ring is wound with a coil of 200 turns allowing 2A current passing through it. The total magnetic flux is 0.3mWb. Calculate relative permeability of iron.
Sol:  = 0.3mWb
= 0.3mWb
A=4cm2=4x10-4 m2
B= /A = 0.3x10-3/4x10-4 = 0.75Wb/m2
/A = 0.3x10-3/4x10-4 = 0.75Wb/m2
AT of iron ring = H x l= (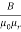 ) x l = (
) x l = (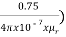 x 0.20 = 119.37x103/
x 0.20 = 119.37x103/
AT for air gap = H x l= (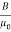 ) x l = (
) x l = (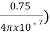 x 0.5 x 10-3 =298.42
x 0.5 x 10-3 =298.42
Total AT required = (119.37x103/ )+ 298.42
)+ 298.42
Also, Total AT required = 200x2= 400
(119.37x103/ )+ 298.42 = 400
)+ 298.42 = 400
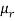 =1175
=1175
Q10) A laminated soft iron ring has a relative permeability 1000 has a mean circumference of 600mm. Cross-sectional area of 250mm2. A radial air gap of 1mm width I cut in the ring which wounds 1000 turns. Calculate the current required to produce an air gap flux of 0.4mWb. (leakage factor = 1.3 and stacking factor = 0.9)
Sol: Total AT required = 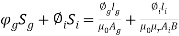
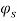 = 0.4mWb
= 0.4mWb
lg = 1mm=1x10-3m
Ag=250mm2=250x10-6m2
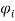 = 1.3 x 0.4x10-3 = 0.52mWb
= 1.3 x 0.4x10-3 = 0.52mWb
Net cross-sectional area = Ai x stacking factor = 250 x 10-6 x 0.9 = 225x10-6m2
Total AT required = 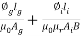 =
= 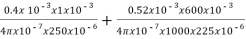 = 1803.75
= 1803.75
I= 1803.75/1000 = 1.803A
Q11) A ring sample of iron fitted with primary and secondary winding is to be tested by the method of reversal to obtain B-H curve. In the test the primary has 350 turns carrying 2A current. ON reversal a change of 8mWb-turns is recorded in secondary winding of 10 turns. The ring is made of 50 laminations, each 0.5mm thick with outer and inner diameter of 25 and 23 cm respectively. Determine B, H and permeability?
Sol: Flux linkage = 2φN2 = 8x 10-3 Wb-turns
2φ x 10 = 8x 10-3
Φ = 4x10-4 Wb
A= 2.5x10-4 m2
B= Φ/A = 4x10-4/2.5x10-4 = 1.6Wb/m2
H= 350 x 2/0.24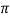 = 928.4 AT/m
= 928.4 AT/m
 = B/H
= B/H
 = B/H
= B/H = 1.6/(4
= 1.6/(4 x10-7x928.4) = 1371
x10-7x928.4) = 1371
Q12) The pole face area of an electromagnet is 0.5m2/pole. It has to lift an iron ingot weighing 860kg. If pole faces are parallel to the surface of the ingot at a distance of 0.8mm determine the coil mmf required.
Sol: Force at two poles = 2x B2A/2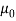 =B2A/
=B2A/
B20.5/ = 860 x 9.8
= 860 x 9.8
B= 0.145Wb/m2
H= B/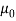 = 0.145/
= 0.145/ = 115.4x103 AT/m
= 115.4x103 AT/m
L=2x0.8mm=1.6x10-3m
AT required = 115.4x103x1.6x10-3 = 184.64
Question Bank
Unit 2
Question Bank
Unit 2
Question Bank
Unit 2
Que1)The amount of flux present in around magnetic bar was measured at 0.013 weber. If the material has a diameter of 14cm, calculate the flux density.
Sol: Area=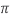 r2
r2
Diameter=2r
r=14/2=7cm=0.07m
Area=3.14 x 0.072=0.0154m2
Flux Density B==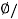 A=0.013/0.0154=0.843 Tesla
A=0.013/0.0154=0.843 Tesla
Que2) Calculate the radius of the material having flux density of 0.5 T and flux present around the magnetic bar is 0.02T.
Sol: Flux Density B=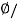 A
A
A= B=0.02/0.5=0.04 m2
B=0.02/0.5=0.04 m2
Area= r2
r2
r=0.113m
Que3) A wire 2.5m long is bent into a square and into a circle. If the current flowing through the wire is 100 A, find the magnetising force at the centre of the square and the centre of the circle?
Sol: Value of h at centre of square will be H=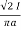 =
= 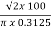 =144 AT/m
=144 AT/m
Value of H at the centre of circle is H=I/2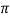 r
r
=125.6AT/m
Que4) Calculate the magnetising force and flux density at a distance of 4cm from a long straight circular conductor carrying a current of 250A and placed in air?
Sol: H=I/2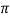 r
r
=250/2 x 0.04=994.71 AT/m
x 0.04=994.71 AT/m
B=μoH
=4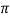 x10-7x994.71=1.25x10-3 Wb/m2
x10-7x994.71=1.25x10-3 Wb/m2
Que5) A closed magnetic circuit of cast steel contains a 6cm long path of cross-sectional area 1 cm2 and a 2cm path of cross sectional area 0.5 cm2. A coil of 200 turns is wound around the 6cm length of the circuit and a current of 0.4A flows. Determine the flux in the 2 cm path, if the relative permeability of the cast steel is 750?
Sol: For 6cm path:
S1 =  =
= 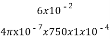 = 6.37 x 105 /H
= 6.37 x 105 /H
For 2cm path:
S2= 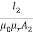 =
= 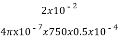 = 4.24 x 105 /H
= 4.24 x 105 /H
Total reluctance S= S1+S2 = 6.37 x 105+4.24 x 105= 10.61 x 105/H
S = mmf/φ
Φ = mmf/S = NI/S = (200x0.4)/( 10.61 x 105) = 7.54x 10-5 Wb
Flux density in the 2cm path B= φ/A = 7.54x 10-5/ = 1.51 T
= 1.51 T
Que6)A laminated soft iron ring of relative permeability 800 has a mean circumference of 600mm and a cross sectional area of 400mm2. A radial air gap of 1mm width is cut in the ring which is wound with 800 turns. Calculate the current required to produce an air gap flux of 0.5mWb if leakage factor is 1.2 and stacking factor 0.9.
Sol: Required Ampere turn =  gSg+
gSg+  iSi
iSi
=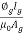 +
+ 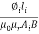
Air gap flux  s=0.5mWb
s=0.5mWb
lg=1mm=1x10-3m
Ag=400mm2=400x10-6
Flux in iron ring  i=1.2x0.5x10-3=0.6mWb
i=1.2x0.5x10-3=0.6mWb
Net cross-sectional area = Ai x stacking factor=400x10-6 x 0.9=360x10-6
Total AT required=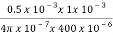 +
+ 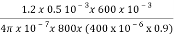 = 994.72
= 994.72
I=A/N=994.72/800=1.24A
Que7) An iron ring has X-section of 4cm2 and a mean diameter of 25cm. An air-gap of 0.3mm has been cut across the section of the ring. The ring is wound with a coil of 220 turns through which a current of 2A is passed. If the total magnetic flux is 0.24mWb, find relative permeability of iron?
Sol: φ=0.24mWb
A=4cm2=4 x 10-4
B=φ/A=0.24 x 10-3/4 x 10-4=0.6Wb/m2
AT for iron ring=H x l=(B/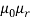 )/l=(0.6/
)/l=(0.6/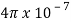 x
x ) x 0.25=1.19 x 105/
) x 0.25=1.19 x 105/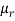
AT for air gap= H x l=( B/ /l=(0.6/
/l=(0.6/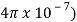 x 0.3x10-3=143.24
x 0.3x10-3=143.24
Total AT required=(1.19 x 105/ +143.24
+143.24
Total AT provided=220 x 2=440
Therefore,
(1.19 x 105/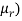 +143.24=440
+143.24=440
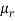 = 400.99
= 400.99
Q8) A ring has a diameter of 20cm and a cross-sectional area of 10 cm2. The ring is made up of semi-circular section of cast iron and cast steel, with each joint having reluctance equal to an air gap of 0.2mm. Find the ampere turns required to produce a flux of 8x10-4Wb. The relative permeability of cast steel and cast iron are 800 and 166 respectively?
Sol: φ = 8x10-4Wb
A= 10 cm2 = 10-3m2
B= Φ/A = 8x10-4/10-3= = 0.8Wb/m2
Air gap
H= B/μo = 0.8/4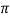 x10-7=6.37x105AT/m
x10-7=6.37x105AT/m
Total air gap length = 2x0.2 =0.4mm=4x10-4m
AT required = H x l = 6.37x105 x 4x10-4 = 255
Cast steel path
H= B/μoμr = 0.8/4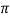 x10-7x800 = 796 AT/m
x10-7x800 = 796 AT/m
Path =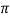 D/2 = 20 x
D/2 = 20 x 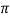 /2 = 31.42mm=0.314m
/2 = 31.42mm=0.314m
AT required = H x l = 796x 0.314 = 250
Cast iron path
H= B/μoμr = 0.8/4 x10-7x166 = 3835 AT/m
x10-7x166 = 3835 AT/m
Path =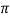 D/2 = 20 x
D/2 = 20 x 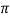 /2 = 31.42mm=0.314m
/2 = 31.42mm=0.314m
AT required = 3835x0.314= 1204
Total AT required = 255 + 250 + 1204 = 1709
Q9) An iron ring has a X-section of4cm2 and a mean diameter of 20cm. An air gap of 0.5 mm is cut across the section of the ring. The ring is wound with a coil of 200 turns allowing 2A current passing through it. The total magnetic flux is 0.3mWb. Calculate relative permeability of iron.
Sol:  = 0.3mWb
= 0.3mWb
A=4cm2=4x10-4 m2
B= /A = 0.3x10-3/4x10-4 = 0.75Wb/m2
/A = 0.3x10-3/4x10-4 = 0.75Wb/m2
AT of iron ring = H x l= (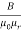 ) x l = (
) x l = (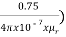 x 0.20 = 119.37x103/
x 0.20 = 119.37x103/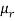
AT for air gap = H x l= (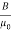 ) x l = (
) x l = (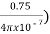 x 0.5 x 10-3 =298.42
x 0.5 x 10-3 =298.42
Total AT required = (119.37x103/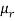 )+ 298.42
)+ 298.42
Also, Total AT required = 200x2= 400
(119.37x103/ )+ 298.42 = 400
)+ 298.42 = 400
 =1175
=1175
Q10) A laminated soft iron ring has a relative permeability 1000 has a mean circumference of 600mm. Cross-sectional area of 250mm2. A radial air gap of 1mm width I cut in the ring which wounds 1000 turns. Calculate the current required to produce an air gap flux of 0.4mWb. (leakage factor = 1.3 and stacking factor = 0.9)
Sol: Total AT required = 
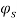 = 0.4mWb
= 0.4mWb
lg = 1mm=1x10-3m
Ag=250mm2=250x10-6m2
 = 1.3 x 0.4x10-3 = 0.52mWb
= 1.3 x 0.4x10-3 = 0.52mWb
Net cross-sectional area = Ai x stacking factor = 250 x 10-6 x 0.9 = 225x10-6m2
Total AT required =  =
= 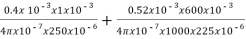 = 1803.75
= 1803.75
I= 1803.75/1000 = 1.803A
Q11) A ring sample of iron fitted with primary and secondary winding is to be tested by the method of reversal to obtain B-H curve. In the test the primary has 350 turns carrying 2A current. ON reversal a change of 8mWb-turns is recorded in secondary winding of 10 turns. The ring is made of 50 laminations, each 0.5mm thick with outer and inner diameter of 25 and 23 cm respectively. Determine B, H and permeability?
Sol: Flux linkage = 2φN2 = 8x 10-3 Wb-turns
2φ x 10 = 8x 10-3
Φ = 4x10-4 Wb
A= 2.5x10-4 m2
B= Φ/A = 4x10-4/2.5x10-4 = 1.6Wb/m2
H= 350 x 2/0.24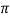 = 928.4 AT/m
= 928.4 AT/m
 = B/H
= B/H
 = B/H
= B/H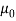 = 1.6/(4
= 1.6/(4 x10-7x928.4) = 1371
x10-7x928.4) = 1371
Q12) The pole face area of an electromagnet is 0.5m2/pole. It has to lift an iron ingot weighing 860kg. If pole faces are parallel to the surface of the ingot at a distance of 0.8mm determine the coil mmf required.
Sol: Force at two poles = 2x B2A/2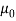 =B2A/
=B2A/
B20.5/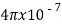 = 860 x 9.8
= 860 x 9.8
B= 0.145Wb/m2
H= B/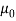 = 0.145/
= 0.145/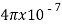 = 115.4x103 AT/m
= 115.4x103 AT/m
L=2x0.8mm=1.6x10-3m
AT required = 115.4x103x1.6x10-3 = 184.64