Question Bank
Unit-4
Question Bank
Unit-4
Q 1) A 10000/ 250 v , 120KVA 1-


Solution : (1) load KVA for max efficiency
= F.L KVA * 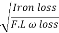 = 120 *
= 120 *  =
=
= 97.98KVA
At 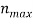 cu loss = Iron loss
cu loss = Iron loss
Total Loss = 1.2+1.2 = 2.4kw
Output = 97.98*1 = 97.98kw
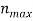 =
= 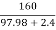 =1.59%
=1.59%
2) cu Loss at half full load =1.8*( )2=0.45kw
)2=0.45kw
Total loss =1.2+0.45=1.65kw
Half F.L output at 0.8 pf = *0.8= 48 kw
*0.8= 48 kw
n= = 0.97
= 0.97
Q2) A 2500/200 V transformer draws a no-load primary current of 0.5 A and absorbs 400 W. Find magnetising and loss currents.
Sol: Iron-loss current = No load input(W) / Primary voltage
= 400/2500 = 0.16 A
I20 = I2w + I2µ
Iµ = √I20 – I2w
= √(0.5)2 – (0.16)2
Iµ = 0.473 A
Q3). A 1-φ transformer has 1000 turns on primary and 200 on secondary. The no load current is 4 amp at p.f of 0.2 lagging. Find primary current and pf when secondary current is 280 A at pf of 0.6 lagging.
Sol : cos-1 0.6 = 53.130 (sin φ = 0.8)
I2 = 280/-53.130A
Φ = cos-1 0.2 = 78.50
Sin φ = 0.98
I1 = I0 + I’2
I’2 = (I2/K) ( -53.130
K = N1/N2 = 1000/200 = 5
I’2 = 280/5 (-53.130
I’2 = 56(-53.130
I1 = I0 + I’2
= 4(0.20 – j0.98) + 56(0.6 – j0.8)
= 0.80 – j3.92 + 33.6 – j44.8
I1 = 34.4 – j48.72
I1 = 59.64 ( -54.770
I lags supply voltage by 54.770
Q4) A 1- φ transformer with ratio of 440/110-V takes a no-load current of 6 A at 0.3 pf lagging. If secondary supplies 120 A at pf of 0.8 lagging. Find current taken by primary.
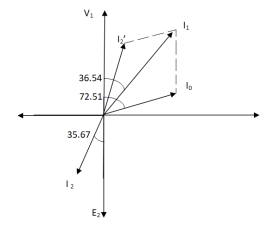
Sol.
Cos φ2 = 0.8
Φ2 = 36.540
Cos φ0 = 0.3
Φ0 = 72.540
K = V2/V1 = 110/440 = ¼
I’2 = KI2 = 120 x ¼ = 30 A
I0 = 6A
Angle between I0& I’2
= 72.54 – 36.54
= 35.670
From vector diagram,
I1 = √(62 + 302 + 2 x 6 x 30 cos 35.67)
I1 = 35.05 A
Q5) The primary and secondary windings of a 30 KVA, 5000/330 V, 1- φ transformer having resistance of 15 Ω and 0.02 Ω. The reactance referred to primary is 34 Ω. Calculate primary voltage required to circulate full-load current when the secondary is S.C. Also calculate the pf?
Sol. K = 330/5000 = 33/500
X01 = 34 Ω
R01 = R1 + R2/K2 = 15 + 0.02(500/33)2 = 19.59 Ω
Z01 = √ R201 – X201
= √ 19.592 + 342
Z01 = 39.23 Ω
F. L I1 = 30,000/5000 = 6A
Vsc = I1 Z01 = 6 x 39.23 = 235.4 V
S.C. Power factor = R01 / Z01 = 19.59/39.23 = 0.5
Q7) In no-load test of 1- φ transformer, the test data are
Primary voltage = 200 V,
Resistance of primary = 0.6 Ω
Secondary voltage = 100 V
Primary current = 0.6 A
Power input = 30 W
Find (a) Turns ratio (b) Magnetising component of no-load current (c) Working component (d) Iron loss
Sol. (a). N1/N2 = 200/100 = 1
(b). W = V1 I0 cos φ0
Cos φ0 = 30/200 x 0.6 = 0.25, sin φ0 = 0.97
Iµ = I0 sin φ0 = 0.6 x 0.97 = 0.58 A
©. Iw = I0 Cos φ0 = 0.6 x 0.25 = 0.15 A
(d). Cu loss = I20 R1 = (0.6)2 x 0.6 = 0.216 W
Iron loss = 30 – 0.216 = 29.78 W
Q8) Obtain the secondary voltage when delivered 10 KW at 0.8 PF.logging . The primary volt be 220v. The 300/600 v 50H2, 1- Transformer has following test results.
Transformer has following test results.
O.C test-:200v, 0.8A 70W-L.V side
S.C test-: 12V,10A 80W-HV side
Sol:- O.C TEST
W=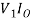 COS
COS 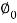
70=200*0.8*Cos
Cos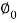 = 0.436
= 0.436
Sin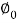 =0.899
=0.899
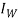 =
= COS
COS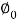 =0.8*0.436=0.35A
=0.8*0.436=0.35A
I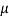 =
=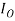 sin
sin = 0.8*0.899=0.72A
= 0.8*0.899=0.72A
 =
= =
= =571.4
=571.4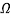
 =
= =
= =277.8𝛺
=277.8𝛺
S.C TEST-:
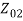 =
= =
=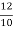 =1.2A
=1.2A
K=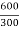 =2
=2
Z01= =
=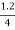 =0.3
=0.3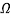
I22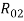 =W
=W
RO2=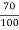 =0.7
=0.7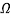
R01=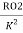 =
=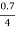 =0.175𝛺
=0.175𝛺
X01=
= 
X01 = 0.244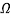
Output KVA = =12.5
=12.5
I2=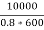 = 20.83A
= 20.83A
Z02=1.2A R02=0.7𝛺
X02= =0.975𝛺
=0.975𝛺
Total transformer drop refereed to secondary
= I2 (RO2COS +X02Sin
+X02Sin )
)
= 20.83(0.7*0.8+0.975*0.6)
= 23.85v
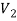 = 600 - 23.85=576.15v
= 600 - 23.85=576.15v
Q9) A 2200/250 V transformer takes 0.7A at a p.f of 0.4 0n open circuit. Find the magnetising and working component of no load primary current?
Sol: I0= 0.7A
Cosφ0=0.4
Iw= I0 cosφ0
Iw=0.7 x 0.4=0.28A
Magnetising component Iµ = √I20 – I2w
= √(0.7)2 – (0.280)2
Iµ = 0.64 A
Q.10) A single phase transformer has 400 turns in primary and 1000 turns in secondary. The cross-sectional area is 80 cm2. If primary is connected to 50hz at 500V. Voltage induced in secondary?
Sol: As we know Emf induced in primary
E1 = 4.44 fN1BmA
500= 4.44x50x1000xBmx(80x10-4)
Bm= 0.28Wb/m2
The voltage induced in secondary is given as
E1/E2 = N1/N2 = K
E2=1000x500/400=1250V
Q.11) A 200 KVA, 1200/200v, 50 Hz, 1-Φ transformer has a leakage impedance of (0.1 + 0.30) Ω for the HV winding and (0.005 + 0.015) Ω for the LV winding. Find the equivalent winding resistance, reactance and impedance referred to the HV and LV side.
Sol: The Turn ratio is given as E1/E2 = N1/N2 = K
K=6
i) Referring to High Voltage Side
Resistance = R1+K2R2= 0.1+ 62 x 0.005 =0.28ohm
Reactance = X1+K2X2= 0.30+ 62 x0.015 = 0.84ohm
Impedance = √0.282+0.842
=0.880hm
Ii) Referring to Low voltage sides
Resistance= R1/K2+R2= (0.1/62) + 0.005=0.007ohm
Reactance = Reactance HV side/K2=0.84/62=0.013ohm
Impedance = Impedance referring HV/K2= 0.88/62 = 0.024ohm
Q.12) When a transformer is connected to a 1200v,50Hz supply the core loss is 900 W, of which 600 is hysteresis and 350 W is eddy current loss. If the applied voltage is raised to 2000V and frequency to 100 Hz. Find new core losses.
Sol: Hysteresis Loss(Wh) α f =P
f =P 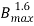 f
f
Eddy current Loss(We)α P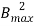 f2 = Q
f2 = Q  f
f
The emf equation of transformer is given as E= 4.44fNBmaxA
Bmaxα E/f
So, the above equations become Wh = P (E/f)2 f = P E1.6f-0.6
600= P x 12001.6 x 50-0.6
P=0.074
The eddy current loss We= Q (E/f)2f2 = QE2
350=Q x 12002
Q=0.243 x 10-3
Now the applied voltage is raised to 2000V so finding new losses with above found P and Q
Wh = P E1.6f-0.6 = 0.074 x 20001.6 x 100-0.6 = 893.06W
We= QE2 = 0.243 x 10-3 x 20002 = 972 W
Q.13) A short circuit test when performed on HV side of a 12kVA,2000/400v,1-Φ transformer the data is:50v, 3A, 100W. If the LV side is delivering full load current at 0.8 p.f and at400v, find the Voltage applied to HV side.
Sol: As the test is done from HV side which is primary side
Z01=50/3 = 16.67ohm
R01= 100/32 = 11.11ohm
X01=  16.672 – 11.112 = 12.42ohm
16.672 – 11.112 = 12.42ohm
I1= 12x1000/2000 = 6A
Voltage drop referred to primary is E = I1(R01cosφ + X01sinφ)
= 6 (11.11 x 0.8 + 12.42 x 0.6)
E = 98.04V
Q.14) A transformer has cu loss of 1.5% and the reactance drop of 3.2% when tested at full load. Calculate its full load regulation at unity power factor.
Sol: Approx. Voltage regulation at unity p.f (full load) = 0.015 cos φ + 0.032 sin φ= 0.015 = 1.5%
Approx. Voltage regulation at 0.08 lagging p.f = 0.015 x 0.8 + 0.032 x 0.6 = 0.032=3.2%
Approx. Voltage regulation at 0.08 leading p.f = 0.015 x 0.8 – 0.032 x 0.6
= 7.2x10-3= -0.72%
Q.15) A 20kVA 440/220V 1-phase transformer 50Hz has iron loss of 289W. The Cu losses found to be 60W and delivers half of full load current. Determine percentage of full load when the efficiency will be maximum?
Sol: 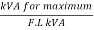 =
= 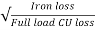
Full load Cu loss = 22 x 100 = 400W
 =
=  =
=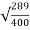 = 0.85
= 0.85
Hence, efficiency will be maximum at 85%
Q16) A 20kVA, 1-Φ transformer,50Hz,500/250v gave following results
OC test (LV side): 230v,2.8A,200W
SC test (LV side): 15v,30A,300W
Calculate the efficiency at full load 0.8 p.f lagging.
Sol: Full load current = 20000/230 = 86.96A
SC current is measured till 30A on low voltage side.
I2R losses 40A n low voltage side = (40/30)2 x 300 = 533.3W
Iron loss for OC test = 200W
Full load output at p.f 0.8 = 20000 x 0.8 =16kW
Efficiency = {(16x1000)/[16000+200+533.33]} = 0.956 = 95.6%
Question Bank
Unit-4
Question Bank
Unit-4
Q 1) A 10000/ 250 v , 120KVA 1-


Solution : (1) load KVA for max efficiency
= F.L KVA * 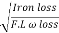 = 120 *
= 120 * 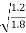 =
=
= 97.98KVA
At 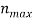 cu loss = Iron loss
cu loss = Iron loss
Total Loss = 1.2+1.2 = 2.4kw
Output = 97.98*1 = 97.98kw
 =
= 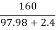 =1.59%
=1.59%
2) cu Loss at half full load =1.8*( )2=0.45kw
)2=0.45kw
Total loss =1.2+0.45=1.65kw
Half F.L output at 0.8 pf =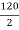 *0.8= 48 kw
*0.8= 48 kw
n= = 0.97
= 0.97
Q2) A 2500/200 V transformer draws a no-load primary current of 0.5 A and absorbs 400 W. Find magnetising and loss currents.
Sol: Iron-loss current = No load input(W) / Primary voltage
= 400/2500 = 0.16 A
I20 = I2w + I2µ
Iµ = √I20 – I2w
= √(0.5)2 – (0.16)2
Iµ = 0.473 A
Q3). A 1-φ transformer has 1000 turns on primary and 200 on secondary. The no load current is 4 amp at p.f of 0.2 lagging. Find primary current and pf when secondary current is 280 A at pf of 0.6 lagging.
Sol : cos-1 0.6 = 53.130 (sin φ = 0.8)
I2 = 280/-53.130A
Φ = cos-1 0.2 = 78.50
Sin φ = 0.98
I1 = I0 + I’2
I’2 = (I2/K) ( -53.130
K = N1/N2 = 1000/200 = 5
I’2 = 280/5 (-53.130
I’2 = 56(-53.130
I1 = I0 + I’2
= 4(0.20 – j0.98) + 56(0.6 – j0.8)
= 0.80 – j3.92 + 33.6 – j44.8
I1 = 34.4 – j48.72
I1 = 59.64 ( -54.770
I lags supply voltage by 54.770
Q4) A 1- φ transformer with ratio of 440/110-V takes a no-load current of 6 A at 0.3 pf lagging. If secondary supplies 120 A at pf of 0.8 lagging. Find current taken by primary.

Sol.
Cos φ2 = 0.8
Φ2 = 36.540
Cos φ0 = 0.3
Φ0 = 72.540
K = V2/V1 = 110/440 = ¼
I’2 = KI2 = 120 x ¼ = 30 A
I0 = 6A
Angle between I0& I’2
= 72.54 – 36.54
= 35.670
From vector diagram,
I1 = √(62 + 302 + 2 x 6 x 30 cos 35.67)
I1 = 35.05 A
Q5) The primary and secondary windings of a 30 KVA, 5000/330 V, 1- φ transformer having resistance of 15 Ω and 0.02 Ω. The reactance referred to primary is 34 Ω. Calculate primary voltage required to circulate full-load current when the secondary is S.C. Also calculate the pf?
Sol. K = 330/5000 = 33/500
X01 = 34 Ω
R01 = R1 + R2/K2 = 15 + 0.02(500/33)2 = 19.59 Ω
Z01 = √ R201 – X201
= √ 19.592 + 342
Z01 = 39.23 Ω
F. L I1 = 30,000/5000 = 6A
Vsc = I1 Z01 = 6 x 39.23 = 235.4 V
S.C. Power factor = R01 / Z01 = 19.59/39.23 = 0.5
Q7) In no-load test of 1- φ transformer, the test data are
Primary voltage = 200 V,
Resistance of primary = 0.6 Ω
Secondary voltage = 100 V
Primary current = 0.6 A
Power input = 30 W
Find (a) Turns ratio (b) Magnetising component of no-load current (c) Working component (d) Iron loss
Sol. (a). N1/N2 = 200/100 = 1
(b). W = V1 I0 cos φ0
Cos φ0 = 30/200 x 0.6 = 0.25, sin φ0 = 0.97
Iµ = I0 sin φ0 = 0.6 x 0.97 = 0.58 A
©. Iw = I0 Cos φ0 = 0.6 x 0.25 = 0.15 A
(d). Cu loss = I20 R1 = (0.6)2 x 0.6 = 0.216 W
Iron loss = 30 – 0.216 = 29.78 W
Q8) Obtain the secondary voltage when delivered 10 KW at 0.8 PF.logging . The primary volt be 220v. The 300/600 v 50H2, 1- Transformer has following test results.
Transformer has following test results.
O.C test-:200v, 0.8A 70W-L.V side
S.C test-: 12V,10A 80W-HV side
Sol:- O.C TEST
W= COS
COS 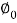
70=200*0.8*Cos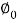
Cos = 0.436
= 0.436
Sin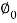 =0.899
=0.899
 =
= COS
COS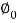 =0.8*0.436=0.35A
=0.8*0.436=0.35A
I =
=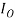 sin
sin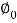 = 0.8*0.899=0.72A
= 0.8*0.899=0.72A
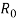 =
=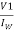 =
=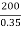 =571.4
=571.4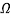
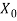 =
= =
= =277.8𝛺
=277.8𝛺
S.C TEST-:
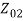 =
= =
=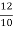 =1.2A
=1.2A
K= =2
=2
Z01= =
=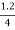 =0.3
=0.3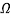
I22 =W
=W
RO2= =0.7
=0.7
R01=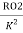 =
=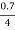 =0.175𝛺
=0.175𝛺
X01=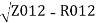
= 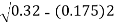
X01 = 0.244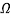
Output KVA =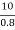 =12.5
=12.5
I2= = 20.83A
= 20.83A
Z02=1.2A R02=0.7𝛺
X02=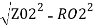 =0.975𝛺
=0.975𝛺
Total transformer drop refereed to secondary
= I2 (RO2COS +X02Sin
+X02Sin )
)
= 20.83(0.7*0.8+0.975*0.6)
= 23.85v
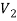 = 600 - 23.85=576.15v
= 600 - 23.85=576.15v
Q9) A 2200/250 V transformer takes 0.7A at a p.f of 0.4 0n open circuit. Find the magnetising and working component of no load primary current?
Sol: I0= 0.7A
Cosφ0=0.4
Iw= I0 cosφ0
Iw=0.7 x 0.4=0.28A
Magnetising component Iµ = √I20 – I2w
= √(0.7)2 – (0.280)2
Iµ = 0.64 A
Q.10) A single phase transformer has 400 turns in primary and 1000 turns in secondary. The cross-sectional area is 80 cm2. If primary is connected to 50hz at 500V. Voltage induced in secondary?
Sol: As we know Emf induced in primary
E1 = 4.44 fN1BmA
500= 4.44x50x1000xBmx(80x10-4)
Bm= 0.28Wb/m2
The voltage induced in secondary is given as
E1/E2 = N1/N2 = K
E2=1000x500/400=1250V
Q.11) A 200 KVA, 1200/200v, 50 Hz, 1-Φ transformer has a leakage impedance of (0.1 + 0.30) Ω for the HV winding and (0.005 + 0.015) Ω for the LV winding. Find the equivalent winding resistance, reactance and impedance referred to the HV and LV side.
Sol: The Turn ratio is given as E1/E2 = N1/N2 = K
K=6
i) Referring to High Voltage Side
Resistance = R1+K2R2= 0.1+ 62 x 0.005 =0.28ohm
Reactance = X1+K2X2= 0.30+ 62 x0.015 = 0.84ohm
Impedance = √0.282+0.842
=0.880hm
Ii) Referring to Low voltage sides
Resistance= R1/K2+R2= (0.1/62) + 0.005=0.007ohm
Reactance = Reactance HV side/K2=0.84/62=0.013ohm
Impedance = Impedance referring HV/K2= 0.88/62 = 0.024ohm
Q.12) When a transformer is connected to a 1200v,50Hz supply the core loss is 900 W, of which 600 is hysteresis and 350 W is eddy current loss. If the applied voltage is raised to 2000V and frequency to 100 Hz. Find new core losses.
Sol: Hysteresis Loss(Wh) α f =P
f =P 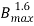 f
f
Eddy current Loss(We)α P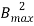 f2 = Q
f2 = Q  f
f
The emf equation of transformer is given as E= 4.44fNBmaxA
Bmaxα E/f
So, the above equations become Wh = P (E/f)2 f = P E1.6f-0.6
600= P x 12001.6 x 50-0.6
P=0.074
The eddy current loss We= Q (E/f)2f2 = QE2
350=Q x 12002
Q=0.243 x 10-3
Now the applied voltage is raised to 2000V so finding new losses with above found P and Q
Wh = P E1.6f-0.6 = 0.074 x 20001.6 x 100-0.6 = 893.06W
We= QE2 = 0.243 x 10-3 x 20002 = 972 W
Q.13) A short circuit test when performed on HV side of a 12kVA,2000/400v,1-Φ transformer the data is:50v, 3A, 100W. If the LV side is delivering full load current at 0.8 p.f and at400v, find the Voltage applied to HV side.
Sol: As the test is done from HV side which is primary side
Z01=50/3 = 16.67ohm
R01= 100/32 = 11.11ohm
X01= 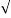 16.672 – 11.112 = 12.42ohm
16.672 – 11.112 = 12.42ohm
I1= 12x1000/2000 = 6A
Voltage drop referred to primary is E = I1(R01cosφ + X01sinφ)
= 6 (11.11 x 0.8 + 12.42 x 0.6)
E = 98.04V
Q.14) A transformer has cu loss of 1.5% and the reactance drop of 3.2% when tested at full load. Calculate its full load regulation at unity power factor.
Sol: Approx. Voltage regulation at unity p.f (full load) = 0.015 cos φ + 0.032 sin φ= 0.015 = 1.5%
Approx. Voltage regulation at 0.08 lagging p.f = 0.015 x 0.8 + 0.032 x 0.6 = 0.032=3.2%
Approx. Voltage regulation at 0.08 leading p.f = 0.015 x 0.8 – 0.032 x 0.6
= 7.2x10-3= -0.72%
Q.15) A 20kVA 440/220V 1-phase transformer 50Hz has iron loss of 289W. The Cu losses found to be 60W and delivers half of full load current. Determine percentage of full load when the efficiency will be maximum?
Sol:  =
= 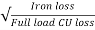
Full load Cu loss = 22 x 100 = 400W
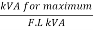 =
=  =
= = 0.85
= 0.85
Hence, efficiency will be maximum at 85%
Q16) A 20kVA, 1-Φ transformer,50Hz,500/250v gave following results
OC test (LV side): 230v,2.8A,200W
SC test (LV side): 15v,30A,300W
Calculate the efficiency at full load 0.8 p.f lagging.
Sol: Full load current = 20000/230 = 86.96A
SC current is measured till 30A on low voltage side.
I2R losses 40A n low voltage side = (40/30)2 x 300 = 533.3W
Iron loss for OC test = 200W
Full load output at p.f 0.8 = 20000 x 0.8 =16kW
Efficiency = {(16x1000)/[16000+200+533.33]} = 0.956 = 95.6%