UNIT 5
UNIT 5
UNIT 5
Q1:Solve the differential equation y’’’+2y’’-y’-2y=0
Solution:
The corresponding characteristic equation is,
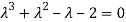
Solving it, we find the roots
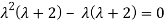
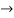
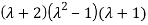=0
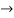
=-1
The general solution for the differential equation is
Y(x)=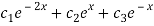 
Where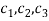 
are arbitrary constants.
Q 2:Solve the equation y’’’+11y’-5y=0
Solution:
The characteristic equation of the give D.E is
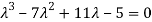
Here one of the root is 
then factorising the term 
from the equation we obtain
(
(
(
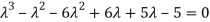
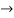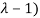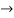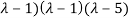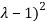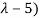=0
(
(
=0
Thus the equation has two roots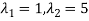 
Hence the general equation of the D.E is
Y(x)=(
Where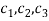 
are arbitrary constants.
Q 3: To find the general solution of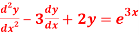 
Solution:
The characteristic equation is r2 -3r+2 =0
The factors are (r-1)(r-2)=0
r=1or r=2
Hence the general solution is A
+B
So in this case the fundamental solutions and their derivatives are as followas:
Y1(x) = ex
Y1’(x) = ex
Y2(x) =e2x
Y2’(x) =2.e2x
Q 4:Find the general solution of the following D.E
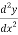-y=2
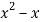-3
Solution :
Consider ,
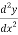-y=0
The characteristic equation is r2 -1 =0
The factors are (r-1)(r+1)=0
r= 1or-1
Hence the general solution is A
+B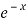
Y1(x) = ex
Y1’(x) =ex
Y2(x) =e-x
Y2’(x) =-e-x
Q 5:Find the value of m for the following D.E
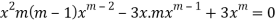
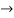
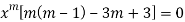
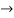m (m-1)-3m+3=0
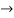m2 – 4m +3=0
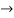(m-1)(m-3)=0
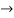m= 1, 3
Q 6:Solve y’=(y+1)x
=
=x
= 
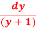= xdx
By integrating on both sides we get,
Ln(y+1) =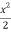
+c
1+y=
y(x)=
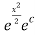-1
Q 7:Find the Legendre’s equation for the following D.E
-
Solution:
n(l+n)=6 , n=2
c2 = -
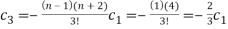
For k= 2,3,....
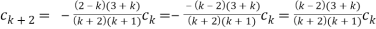
At k=2,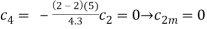 
for m=2,3,.....
the general solution is.,
Y=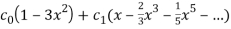
= 
Q 8:Solve the given D.E
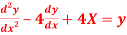
Solution:
The above equation can be writen as,
(
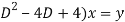....... (1)
(
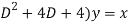........ (2)
Eliminating y from equations (1) and (2)
(
(
x=25x+16et
i.e.
(
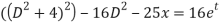
x=A.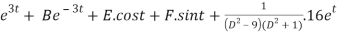
Thus the general equation is,
A.e3t +B.e-3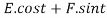t+
-et.
Q 10:Solve the following equations using Legendre’s method:
(1).
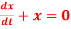
(2).
Solution:
Subtract eq(1)-eq(2)
i.e the given equations can be written as follow:
Dx+y=0......(1)
Dy+x=0.......(2)
(1)
Dx+y=0 (
(2)
D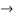
Dx+D2y=0
By subtracting the above two equations we get.,
y-D2y=0
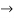(D2 -1)y =0
The general solution is given by,
Aet+Be-t.
Q 11:Verify that 
and 
both satisfy the constant co-efficient linear homogeneous equation:
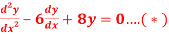
Solution:
Consider,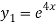 
Differenting on both sides we get,
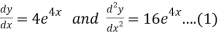
Now consider, 
Differenting on both sides we get,
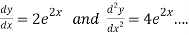(2)
Substituting eq(1) and (2) in equation(*) we get the general solution as follow,
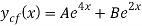
Q 12:Find the order and degree of the following D.E
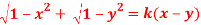
Solution:
Let x= sin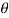
and y=sin
Then the given equation can be written as,
(
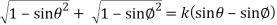
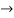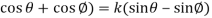
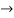2cos
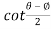
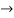
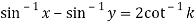
On differentiating both sides we get,
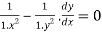
Q 13:A spring with spring constant 18N/m is attached to a 2kg mass with negligible friction. Determine the period that the spring mass system will oscillate for any non-zero initial conditions.
Solution:
From above, we have a spring mass system modelled by the DE
2y’’ + 18y = 0 which has general solution given by
y(t) =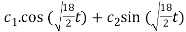 
= c1 cos(3t)+c2 sin(3t)
Since period of cost is 2π, then the period of cos(3t) and sin(3t) is 2π/ 3 .
Therefore, the period of c1 cos(3t) + c2 sin(3t) is also 2π /3 .
Q 14:Two 9-volt batteries are connected to a series in which the inductance is ¼ henry and the resistance is 8 ohms. Determine the current i(t) if the initial current is zero.
Solution:
Given,
L=
R=8 E(t)=18
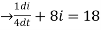
The required D.E is,
L .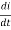
+Rt=E(t)
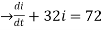
U(t) =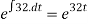 
the above equation can be written as,
Q 15: Given: The rectangular beam, built in at the left end, having length, L, and cross-section of width, b, and height, h, is acted upon by a point load, P, at its free end.
Req'd: Determine the deflection at the end of the beam.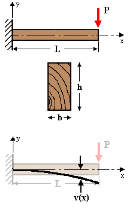 
Sol'n: The bending moment in the beam is given by:
M(x)=-P(L-x)
Therefore the differential equation for bending is:
Elv”(x)=-P(L-x)
Integrating with respect to x
Elv’(x)=-P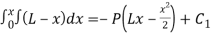
Since the slope at the built-in end is zero, then
v’(x=0)=0
=0
Integrating again gives:
Elv(x)= -P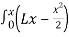
dx= -P
+
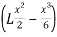The deflection at the built-in end is zero, therefore:
v(x=0)=0
.
Therefore, the equation of the elastic curve is:
v(x)=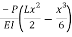 
Deflection at the tip is then:
v(L)=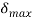
=
Since the sign of v(L) is negative, the deflection is downward.
