Unit 1
Properties of Fluids & Fluid Statics
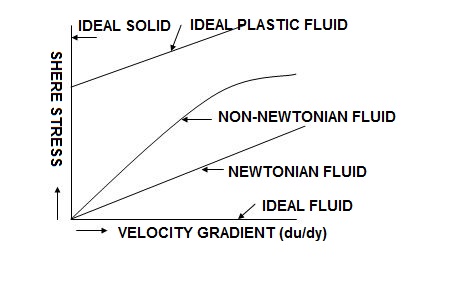
Q1. Explain the Classification of fluids with Rheological Diagram.
Ans- Classification of fluids-
Ideal Fluid:
A fluid which is incompressible and is having no viscosity is known as an ideal fluid. Ideal Fluid is only an imaginary fluid as all the fluids. Which exist have some viscosity.
Real Fluid:
A fluid, which possesses viscosity, is known as real fluid. All the fluids in actual practice are real fluids.
Rheological Diagram
Q2. Explain the Physical properties of a fluid.
Ans- 1) Density or Mass Density:
The density or mass density of a fluid is defined as the ratio of the mass of a fluid to its volume.
It is denoted by the symbol (rho).
The unit of mass density in the SI unit is kg/m3.
= Mass of fluid / Volume of fluid = m/v
The value of the density of water is 1000 kg/m3
2) Specific weight or weight density:
Specific weight or weight density of a fluid is the ratio between the weight of a fluid to its volume.
It is denoted by the symbol w.
w = Weight of fluid / Volume of fluid = W/v
= x g
The value of specific weight or weight density (w) for water is 9810N/m3.
3) Specific Volume:
Specific Volume of a fluid is defined as the volume of a fluid occupied by a unit mass or volume per unit mass of a fluid is called a specific volume.
Mathematically, it is expressed as,
Specific Volume = Volume of Fluid / Mass of Fluid = v/m
= 1/
It is expressed as m3 /kg.
4) Relative density or Specific Gravity:
Specific Gravity is defined as the ratio of the weight density of a fluid to the weight density of the standard fluid.
Specific gravity is also called relative density. It is dimensionless gravity and is denoted by the symbol S.
S (For Liquids ) = Weight density(density) of liquid / Weight density(density) of Water.
The specific gravity of mercury is 13.6 and the specific gravity of water is 1.
5) Viscosity:
Newton’s Law of Viscosity
This law states that the shear stress () on a fluid element layer is directly proportional to the rate of shear strain. The constant of proportionality is called the coefficient of viscosity.
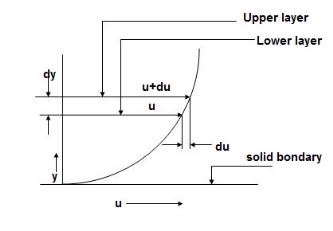
= du / dy
The fluids which follow this law are known as Newtonian fluids.
6) Cohesion & Adhesion
Cohesion:
Cohesion means intermolecular attraction between molecules of the same liquid. It enables a liquid to resist a small number of tensile stresses. Cohesion is a tendency of the liquid to remain as one assemblage of particles.
Adhesion:
Adhesion means attraction between the molecule of a liquid and the molecule of a solid boundary surface in contact with the liquid. This property enables a liquid to stick to another body.;
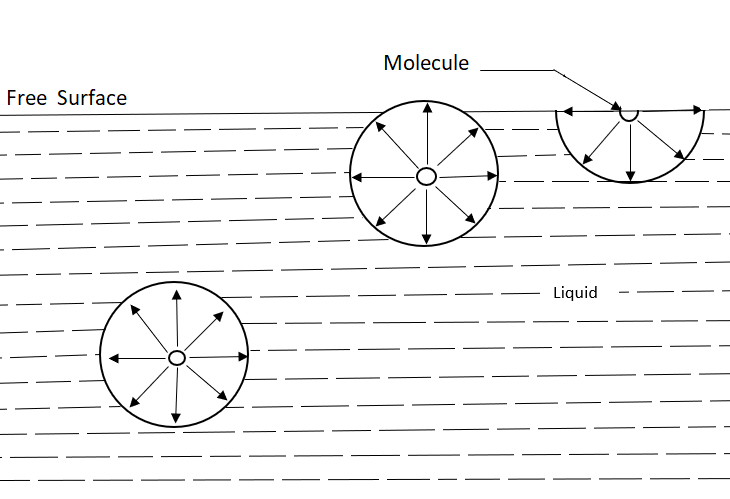
7) Surface tension
Surface tension is caused by the force of cohesion at the free surface.
A liquid molecule in the interior of the liquid mass is surrounded by the other molecules all around and is in equilibrium.
At the free surface of the liquid, there are no liquid molecules above the surface to balance the force of the molecules below it.
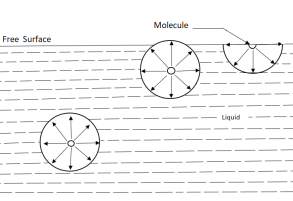
Consequently, as shown in fig there is the net inward force on the molecules at the free surface.
The force is normal to the liquid surface.
At the free surface, a thin layer of molecules is formed.
It is denoted by letter.
It’s SI unit is N/m.
8) Capillarity:
Capillarity is defined as a phenomenon of rising or fall of a liquid surface in a small tube relative to the adjacent general level of liquid when the tube is held vertically in the liquid.
The rise of liquid surface is known as capillary rise while the fall of the liquid surface is known as capillary depression.
It is expressed in terms of cm or mm of liquid.
Its value depends upon the specific weight of the liquid, the diameter of the tube, and the surface tension of the liquid.
h = Height of the liquid in the tube.
d = Diameter of tube
= Surface tension of liquid.
= Angle of contact between liquid and glass tube.
= Density Of liquid.
h= 4cos / e*g*d
9)Vapor Pressure:
All liquid tends to evaporate a vaporize.
Molecules are continuously projected from the free surface to the atmosphere.
These ejected molecules are in a gaseous state and exact their own partial vapor pressure on the liquid surface. This pressure is known as the vapors' pressure of the liquid (Pv).
Vapors pressure increase with the rise in temperature.
Q3. Explain Basic equation of hydrostatics.
Ans- To determine the pressure at any point in a fluid at rest ‘hydrostatic law ‘is used which states that ‘the rate of increase of pressure in a vertically downward direction must be equal to the specific weight of the fluid at that point.
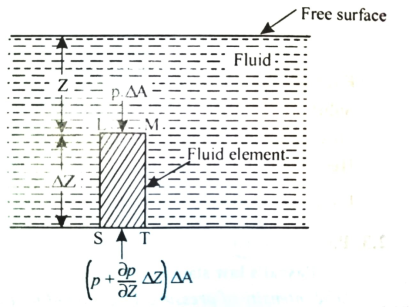
Let p = Intensity of pressure on face LM,
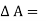 Cross-sectional area of the element,
Cross-sectional area of the element,
Z = Distance of the fluid element from the free surface,
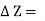 Height of the element,
Height of the element,
The forces acting on the elements are:
Pressure forces on the face LM = p x 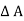 …….acting downward.
…….acting downward.
Pressure forces on the face ST = 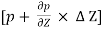 x
x 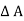 ……. Acting upward
……. Acting upward
Weight of the fluid element = Weight density x Volume
= w x 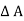 x
x 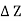
Pressure forces on the face MT & LS are equal and opposite.
For equilibrium of the fluid element,
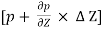 x
x 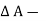 p x
p x 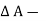 w x
w x 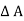 x
x 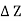 = 0
= 0
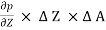 - w x
- w x 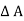 x
x 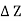 = 0
= 0
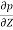 = w
= w
Above eqn states that the rate of increase of pressure in a vertical direction is equal to the weight density of the fluid at that point. This is hydrostatic law.
On integrating above eqn
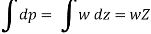
Where p = pressure above atmospheric pressure
Z = pressure head.
w = Specific weight of liquid.
Q4. Explain Pressure head and Pascal’s Law.
Ans- Pressure head
To determine the pressure at any point in a fluid at rest ‘hydrostatic law ‘is used which states that ‘the rate of increase of pressure in a vertically downward direction must be equal to the specific weight of the fluid at that point.
p = w Z
Or
Z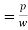
where p = pressure above atmospheric pressure
Z = pressure head.
w = Specific weight of liquid.
-Pascal's Law
Pascal's law states that the pressure applied to a fluid in a closed container is transmitted equally to all points in the fluid and acts in all directions of the container. It is applicable to both solids and liquids.
Application of pascal’s law-
Imp points of pascal law-
Q5. Calculate the specific weight, density & specific gravity of one liter of liquid which weighs 7 N.
Ans –
Given -
V = 1 lit = 1/1000 = 1 x 10-3 m3
W = 7 N
Find -
,, S
Solution –
i). Specific weight =
W/V = 7/1 x 10-3 = 7000 N/m3
ii). Density =
/g = 7000/9.81 = 713.5 kg/m3
iii). Specific gravity S =
/w = 7000/9810 = 0.713
Q6. The absolute viscosity of liquid having a specific gravity of 0.87 is 0.073 poise. Find kinematic viscosity in m2/s & stoke.
Ans- Given-
S = 0.87
= 0.073 poise = 0.073/10
= 7.3 x 10-3 Ns/m2
Find-
v
Solution –
1). Density = S x w
= 0.87 x 1000
= 870 kg/m3
2). Kinematic viscosity = v = / = 7.3 x 10-3 / 870
= 8.39 x 10-6 m2/s
= 8.39 x 10-6/10-4
= 0.0839 stoke
Q7. A flat plate of area 1.5 x 106 mm2 is pulled with a speed of 0.4 m/s relative to another plate located at a distance of 0.15 mm from it. Find the force & power required to maintain this speed, if the fluid separating them is having a viscosity as 1 poise.
Ans-
Given -
A = 1.5 x 106 mm2 = 1.5 m2
du = 0.4 m/s
dy = 0.15 mm = 0.15 x 10-3 m
= 1 poise = 1/10 = 0.1 Ns/m2
Find-
F, P
Solution –
1). = du/dy = 0.1 x 0.4/0.15 x 10-3 = 266.67 N/m2
2). F = x A = 266.67 x 1.5 = 400 N
3). Power (P) = F x u
= 400 x 0.4
= 160 watts
Q8. Calculate the dynamic viscosity of an oil, which is used for lubrication between a square plate of size 0.8 m x 0.8 m & an inclined plane with an angle of inclination 300 as shown in fig. The weight of the square plate is 300 N & it slides down the inclined plane with a uniform velocity of 0.3 m/s. The thickness of the oil film is 7.5 mm.
Ans-
Given-
A = 0.8 x 0.8 = 0.64 m2
= 300
W = 300 N
u = 0.3 m/s
dy = 1.5 mm = 1.5 x 10-3 m
Solution –
1). F = 300Sin = 300 sin30 = 150 N
2). = F/A = 150/0.64 = 234.375 N/m2
3). = du/dy
= x dy/du
=234.375 x 1.5 x 10-3/0.3
= 1.17 Ns/m2
Q9. The dynamic viscosity of an oil, used for lubrication between a shaft & sleeve is 6 poise. The shaft is of diameter 0.4m & rotates at 190 rpm. Calculate the power lost in the bearings for a sleeve length of 90 mm. The thickness of the oil film is 1.5 mm.
Ans-
Given-
= 6 poise = 6/10 = 0.6 Ns/m2
D = 0.4 m
N = 190 rpm
L = 90 mm = 0.09 m
dy = 1.5 mm = 1.5 x 10-3 m
Find -
Power lost
Solution –
1). u = πDN/60 = π x 0.4 x 190/60 = 3.98 m/s
2). du = u-0 = 3.98 m/s
3). = du/dy = 0.6 x 3.98/1.5 x 10-3 = 1592 N/m2
4). F = x Area
= 1592 x π x D x L
= 1592 x π x 0.4 x 0.09
= 180.05 N
5). Torque = F x D/2 = 180.05 x 0.4/2 = 36.01 Nm
6). Power lost = 2πNT/60 = 2π x 190 x 36.01/60 = 716.48 W
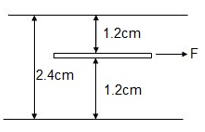
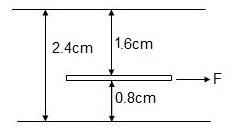
Que 10. Two large plane surfaces are 2.4 cm apart. The space between the surfaces is filled with glycerin. What force is required to drag a very thin plate of surface area as square meter between the two large plane surfaces at a speed of 0.6 m/s if,
i) The thin plate is in the middle of the two plane surfaces.
ii) The thin plate is at a distance of 0.8 cm from one of the plane surfaces?
Take = 8.10 x 10-1 Ns/m2
Ans- Given-
A = 0.5 m2
u = 0.6 m/s
= 8.10 x 10-1 Ns/m2
Case 1.When the thin plate is in the middle of the two planes.
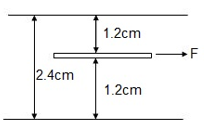
dy = 0.024/2 = 0.012 m
= du/dy
= 8.10 x 10-1 x 0.6/0.012 = 40.5 N/m2
F = x A x 2
= 40.5 x 0.5 x 2
= 40.5 N
Case (ii). When the thin plate is at a distance of 0.8 cm from one of the planes.
dy1 = 0.008 m
dy2 = 0.024 – 0.008 = 0.016 m
1 = du/dy1 = 8.10 x 10-1 x 0.6/0.008
= 60.75 N/m2
F1 = 1 x A = 60.75 x 0.5 = 30.375 N
2 = du/dy2 = 8.10 x 10-1 x 0.6/0.016
= 30.375 N/m2
F2 = 2 x A = 30.375 x 0.5 = 15.187 N
F = F1 + F2
= 30.375 + 15.187
= 45.562 N
Q11. A vertical gap 2.2 cm wide of infinite extent contains a fluid of viscosity 2 Ns/m2& sp.gr 0.9. A metallic plate 1.2m x 1.2m x 0.2cm is to be lifted with a constant velocity of 0.15 m/sec, through the gap. If the plate is in the middle of the gap. Find the force required.
Ans- Given-
Width of gap = 2.2 cm = 0.022 m
= 2 Ns/m2
S = 0.9
= S x 9810 = 0.9 x 9810
= 8829 N/m3
Size of plate = (1.2 x 1.2 x (2 x 10-3))m
du = 0.15 m/sec
Weight of plate = 40 N
Find –
F.
Solution –
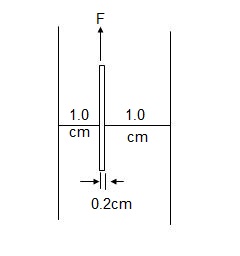
1). the distance of plate from the vertical surface of the gap, when the plate is in the middle.
dy = 0.022 – 2 x 10-3/2 = 0.01 m
2). = x du/dy = 2 x 0.15/0.01 = 30 N/m2
3). Total shear force = 2 x x A
F = 2 x 30 x (1.2 x 1.2)
= 86.4 N
4). the upward thrust = weight of fluid displaced
= x v
= 8829 x 1.2 x 1.2 x 2 x 10-3
= 25.43 N
5). Effective weight of the plate
= weight of plate – Upward thrust
= 40 – 25.43
= 14.57 N
6). Total force required to lift the plate up
= F + 14.57
= 86.4 + 14.57
= 100.97 N
Q12.Explain the basic equation of hydrostatics.
Ans- To determine the pressure at any point in a fluid at rest ‘hydrostatic law ‘is used which states that ‘the rate of increase of pressure in a vertically downward direction must be equal to the specific weight of the fluid at that point.
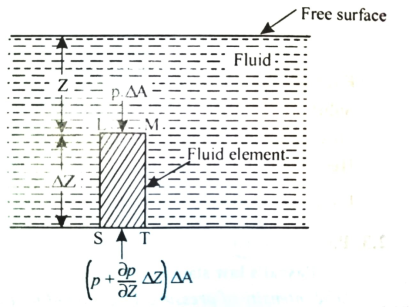
Let p = Intensity of pressure on face LM,
 Cross-sectional area of the element,
Cross-sectional area of the element,
Z = Distance of the fluid element from the free surface,
 Height of the element,
Height of the element,
The forces acting on the elements are:
Pressure forces on the face LM = p x 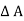 …….acting downward.
…….acting downward.
Pressure forces on the face ST = 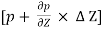 x
x 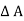 ……. Acting upward
……. Acting upward
Weight of the fluid element = Weight density x Volume
= w x 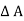 x
x 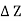
Pressure forces on the face MT & LS are equal and opposite.
For equilibrium of the fluid element,
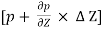 x
x 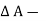 p x
p x 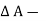 w x
w x 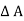 x
x 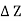 = 0
= 0
 - w x
- w x 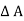 x
x 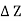 = 0
= 0
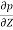 = w
= w
Above eqn states that the rate of increase of pressure in a vertical direction is equal to the weight density of the fluid at that point. This is hydrostatic law.
On integrating above eqn
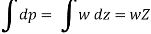
Where p = pressure above atmospheric pressure
Z = pressure head.
w = Specific weight of liquid.
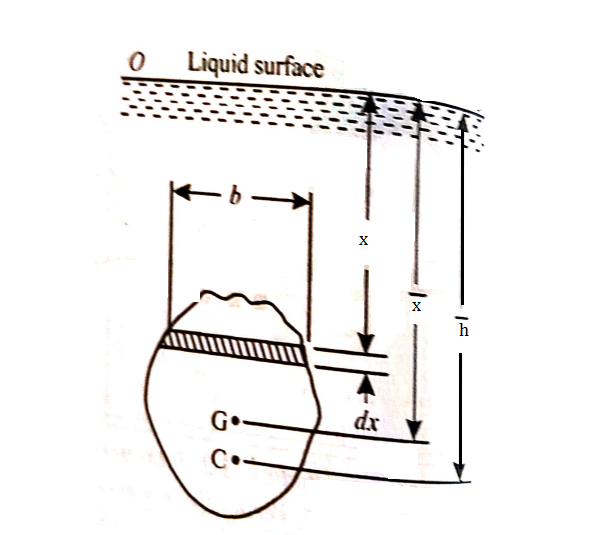
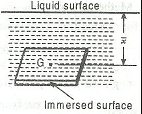
Q13.Explain the total pressure and center of pressure for horizontal plane & vertical plane.
Ans-
Consider a thin horizontal strip of the surface of thickness dx and breadth b.
Let the depth of the strip be x.
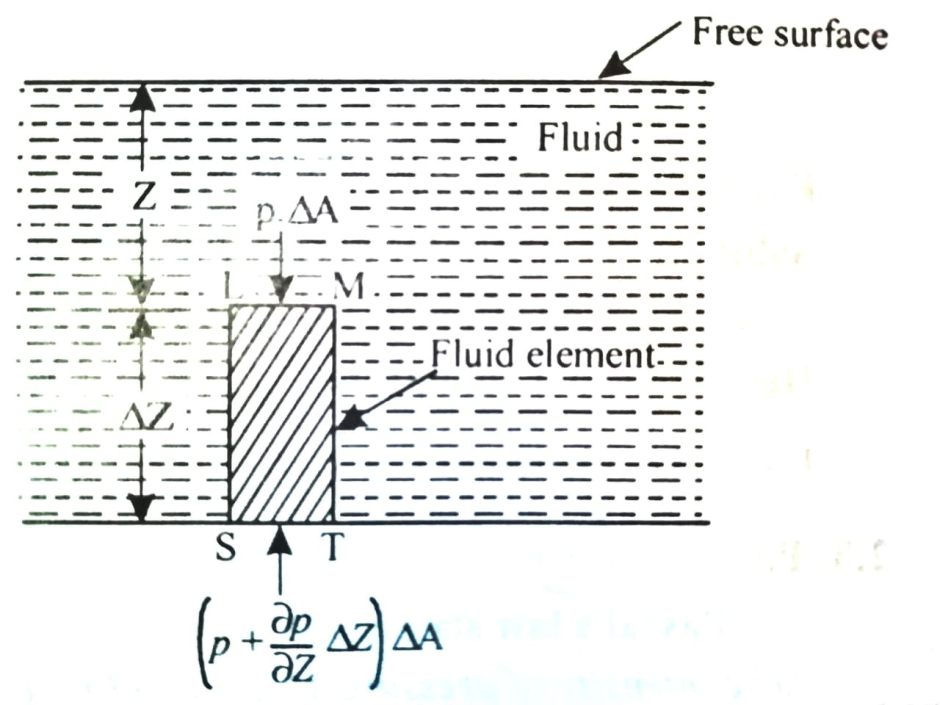
Let the intensity of pressure on strip be p.
p =wx
Total pressure on the strip = p.bdx = wx.bdx
Total pressure on the whole area P =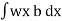
=w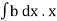
But 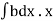 = Moment of the surface area about the liquid level = A
= Moment of the surface area about the liquid level = A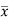
P=wA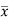
Moment of the pressure about free surface OO = (w.x.b. dx).x = w.x2.b.dx
Total Moment, M = 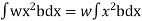
But 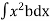 = Moment of inertia of the surface about free surface OO (I0)
= Moment of inertia of the surface about free surface OO (I0)
Hence, M = wI0
The sum of the moment of the pressure is also equal to P×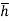 .
.
P×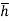 = wI0
= wI0
wA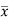 x
x 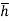 = wI0
= wI0
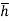 =
= 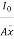
But 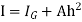
Above eqn becomes
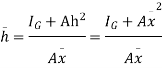
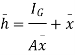
2. Horizontal plane surface
The total pressure on a horizontally immersed surface, as shown in Fig.above, is given by
Where
w = Specific weight of the liquid,
A = Area of the immersed surface, and
x (bar) = Depth of the centre of gravity of the immersed surface from the liquid surface.
Q14. Explain the total pressure and center of pressure for Inclined plane & Curved plane.
Ans-
Let A = area of the surface
 = depth of the center of gravity from the free liquid surface.
= depth of the center of gravity from the free liquid surface.
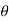 =angle at which the immersed surface is inclined with the liquid surface.
=angle at which the immersed surface is inclined with the liquid surface.
w=specific weight of liquid.
Consider a strip of thickness dx width b at a distance l from O.
The intensity of pressure on the strip = wlsin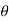
Area of strip = b.dx
Pressure on the strip =Intensity of pressure × area = wlsin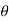 × b.dx
× b.dx
The total pressure on the surface
P = 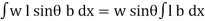
But 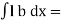 Moment of surface about OO =
Moment of surface about OO = 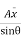
P= = w.
= w.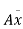
Let, 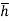 = Depth of center of pressure below the free liquid surface
= Depth of center of pressure below the free liquid surface
IG = Moment of inertia of the immersed surface about 00.
Moment of pressure about OO = wl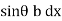 × l = wl2
× l = wl2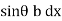
Sum of moments about O
M = 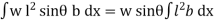
But  = I0 = Moment of inertia of the surface about point O
= I0 = Moment of inertia of the surface about point O
M = 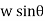 Io
Io
Sum of the moment of all such pressure about O is also equal to 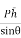
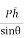 = w
= w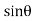 Io
Io
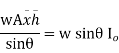
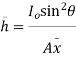
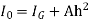
Where 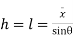
 (
(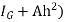
=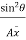 (
(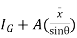 2)
2)
=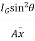 +
+ 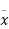
2. Curved surface
Consider a curved immersed surface LM submerged in a static fluid as shown in fig.
At any point on the curved surface, the pressure acts normal to the surface.
Thus, if dA is the area of a small element of the curved surface lying at a vertical depth of h from the surface of the liquid, then total pressure on the elemental area is
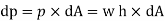
This force dP acts normal to the surface. Further integration of the above eqn would provide the total pressure on the curved surface and hence

But in the case of a curved surface, the direction of the total pressure on the elementary areas is not in the same direction but varies from point to point.
Thus, the integration of eqn. for the curved surface is impossible. The problem, however, can be solved by resolving the force P into horizontal and vertical components PH and PV. Then total force on the curved surface is
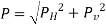
Hence, the direction of the resultant force P

Where,
PH = Total pressure force on the projected area of the curved surface on the vertical plane.
PV = Weight of the liquid supported by the curved surface of the liquid.
Q15. Explain Stability of floating and submerged bodies.
Ans- Stability of floating Body :
The stability of a floating body is determined from the position of Meta-centre (M).
a) Stable Equilibrium
If the point M is above G, the floating body will be in stable equilibrium as shown in Fig.
M is above G (stable equilibrium) M is below G (unstable equilibrium)
If a slight angular displacement is given to the floating body in the clockwise direction, the center of buoyancy shifts from B to B1, such that the vertical line through B1 cuts at M.
Then the buoyant force FB through B1 and weight W through G constitute a couple acting in the anti-clockwise direction and thus bringing the floating body in the original position.
b) Unstable Equilibrium :
If the point M is below G, the floating body will be in unstable equilibrium as shown in fig.
The disturbing couple is acting in the clockwise direction.
The couple due to buoyant force FB and W is also acting in clock direction and thus overturning the floating body.
c) Neutral Equilibrium :
If the point M is at the center of gravity of the body. The floating body will be in neutral equilibrium.
Stability of submerged Body:
The position of the center of gravity ¢er of buoyancy in case of a completely submerged body is fixed.
Weight W acting vertically downward through G, while the buoyant force FB is acting vertically up through B.
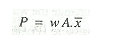
a) Stable Equilibrium
When W = FB& point B is above G, the body is said to be in stable equilibrium.
b) Unstable Equilibrium :
If W = FB , but the center of buoyancy B is below the center of gravity G, the body is in unstable equilibrium.
c) Neutral Equilibrium :
If W = FB & point B & G are at the same point, the body is said to be in neutral equilibrium.