Unit 5
Open Flow Channel
Q1. Explain Classification of open channel flow.
Ans- Channels can be classified as follows:
2. Prismatic and Non-prismatic :
3. Rigid and Mobile Boundary Channels:
Q2. Explain the geometric elements of the channel flow.
Ans- Geometric elements of a channel:
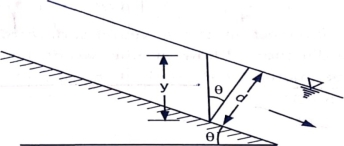
2. Depth of Flow Section’d’: It is the depth of flow normal to the direction of flow
 . Cos
. Cos 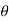 or y =
or y = 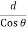
If the slope angle 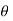 is very small, y
is very small, y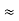 d.
d.
3. Stage: It is the elevation or vertical distance of the free surface above a datum.
4. Top Width 'T': It is the width of the channel section at the free surface.

5 Water Area (or area of flow) 'A': it is the cross-sectional area of flow normal to the direction of flow.
6. Wetted Perimeter (p): It is the length of the line of intersection of the wetted surface of the channel with the cross-sectional plane normal to the direction of flow.
7. Hydraulic Radius 'R': It is the ratio of the area of flow (A) to the wetted perimeter (P). R=
8. Hydraulic depth 'D': It is the ratio of the area of flow (A) to the top width (T).
D = 
9. The Section Factor for Critical Flow 'Z': It is the product of the area of flow (A) and the square root of hydraulic depth (D). = A 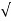 D
D
10.The Section Factor for Uniform Flow Computation: It is the product of the area of flow (A) and the two-thirds power of hydraulic radius (R)
Section factor for uniform flow computations = A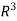
Q3. Explain the Basic governing continuity equation of Channel.
Ans- Continuity Equation:
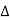
Inflow in the element = [Q -  ].
].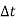 ................1
................1
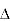
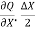
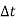
= [Q - 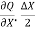 ] .
] .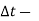 [Q +
[Q + 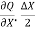 ].
].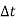
= - 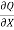 .
. 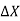 .
.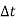 .............3
.............3
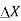
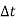
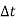
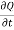
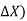
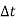
- 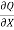 .
. 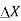 .
.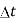 =
= 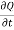 (A.
(A. 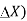 .
.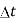
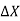
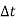
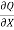
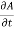
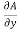
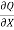 +
+ 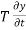 =0 ........6
=0 ........6
Q = AV, where A is the area and V the average velocity
Q = AV in equation 6 , we get
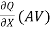 +
+ 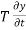 =0 ..................7
=0 ..................7
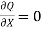 Or Q=constant
Or Q=constant
Q= A1 V1= A2 V2 =A3 V3 = constant. ..........8
Q4. Explain the Basic governing Energy equation of Channel.
Ans-
Z+ +
+ 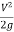 = constant
= constant
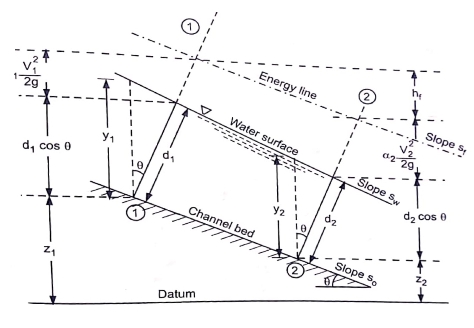
Z1 + d1 cos 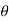 +
+ 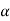 1
1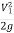 = Z2 + d2 cos
= Z2 + d2 cos 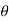 +
+ 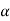 2
2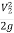 +hf .....................9
+hf .....................9
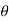
Z1 + y1 + 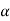 1
1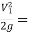 Z2 + y2 +
Z2 + y2 + 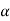 2
2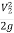 +hf ...........................10
+hf ...........................10
If further,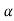 1 =
1 =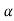 2 = 1
2 = 1
Z1 + y1 + 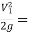 Z2 + y2 +
Z2 + y2 + 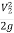 +hf ..........................11
+hf ..........................11
Q5. Explain the Basic governing Momentum Equation of Channel.
Ans-

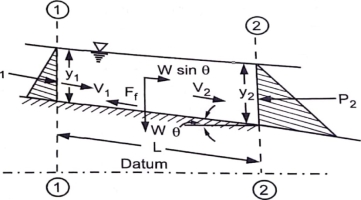
(1) F1 and F2, the hydrostatic forces acting on faces 1-1 and 2-2.
(2) Ff, the frictional force at the boundary of the channel.
(3) Fa, the force due to air resistance at the free surface.
(4) W sin 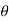 , the body force i.e. a component of the weight of the liquid in the direction of motion.
, the body force i.e. a component of the weight of the liquid in the direction of motion. is the slope of the bed of the channel.
is the slope of the bed of the channel.
F1 - F2 + W sin 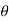 - Ff - Fa =
- Ff - Fa = 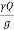 (V2 – V1) .....................12
(V2 – V1) .....................12
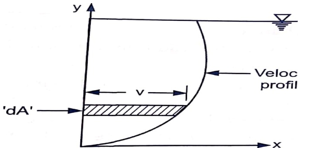
Q6. Explain One dimensional approach.
Ans- The characteristics of flow like velocity, pressure, etc. in open channel flow generally vary in longitudinal as well as normal directions.
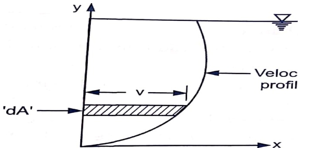
(A) Average Velocity of Flow :
PQ = v dA
Q =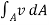
Q = A V
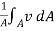
(B) The Discharge :
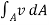
(C) Kinetic Energy :
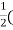
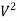
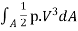
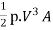
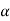

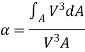
The energy correction factor, 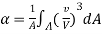 ...................13
...................13
K.E.=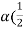 p
p ) .....................14
) .....................14
(d) Momentum :
= (pv dA) .v
=p 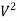 dA
dA
=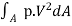
=p 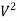 A
A
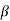
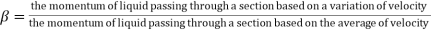
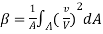
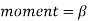 .p A
.p A 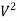
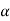
Q7. Explain Factors affecting Manning's roughness coefficient.
Ans- Following are the important factor affecting's roughness coefficient ' n :
1) Surface roughness
2) Vegetation
3) Channel irregularities
4) Channel alignment
5) Silting and scouring.
6) Stage and discharge
7) Transport of suspended and bed material
8) Size and shape
9) Obstruction
1) Surface roughness :
2) Vegetation :
3) Channel irregularities :
4) Channel alignment :
5) Silting and scouring:
6) Stage and discharge:
7) Transport of suspended and bed material :
Que (8) Explain Chezy's Formula with Assumptions.
Ans-
Assumptions Made in Chezy's Equation :
1) The flow is steady
2) Channel is prismatic.
3) The bed slope of the channel is small.
Chezy's Formula for Steady Uniform Flow-through Open Channel :
Let, P = Wetted perimeter of cross-section
A = Uniform cross-sectional area
L = Length of the channel
v = Mean velocity of flow
f = Frictional resistance per unit area at unit velocity
Y = Specific weight of water
According to Froude law , the frictional resistance in a length L of the channel
F = f x contact area x ( velocity )2 = f x PL x v2
Work done against the friction per second
Work done = Frictional resistance x distance
= f PLv2 x v = f PLv3
Weight of water between the two section =  AL
AL
This amount of water will fall vertical downward by a distance v in one second
height of fall = v S
Loss of potential energy in one second = Weight x height of fall = ( AL ) v S
We know ,
Work done against friction = Loss in potential energy
f PLv3 = (  AL ) v S
AL ) v S
v3 =  x
x  S
S
v = 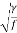 x
x 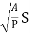
v = C 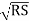
Where C = Chezy's constant = 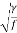
R = hydraulic mean depth = 
Discharge Q = A x v = AC 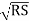
Q9. Explain Important terms pertaining to uniform flow.
(1) Normal depth, (2) conveyance,(3) section factor,(4) the concept of second hydraulic exponent.
Ans-
1) Normal depth :
2) Conveyance :
Q = C A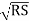 = K
= K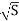 Where K = C A
Where K = C A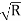
For manning's formula
Q = K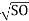 , Where K =
, Where K =  A R2/3
A R2/3
3) Section factor:
Z = A R2/3
4) Hydraulic exponent for uniform flow :
K 2= C yN
Q10. Explain the efficient channel sections: (1)Rectangular,(2) Triangular, and (3)Trapezoidal
Ans-
1) Rectangular section:
Consider rectangular channel of cross-sectional area A
Area of section A = By
Perimeter of section P = B + 2Y =  + 2y
+ 2y
Discharge through channel,
Q = A • v = AC 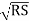
= AC 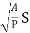
= C 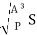
= 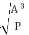 C
C 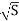
Since A, C, and S are constant in the above equation, the discharge will be maximum when perimeter P in minimum.
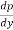 = 0
= 0
 (
(  + 2y) = 0
+ 2y) = 0
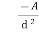 = 0
= 0
A = 2y2
By = 2y2
B = 2y
Y = 
The maximum discharge for the rectangular section occurs when depth is one – half of the width of the channel.
Hydraulic mean depth for this section
R =  =
= 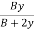
=  =
= 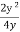
R = 
Trapezoidal section:
Consider a channel of trapezoidal cross-section
Let, A = are of cross-section
b = bottom width
y = depth of flow
 = side slope ( i.e. 1 vertical to m horizontal)
= side slope ( i.e. 1 vertical to m horizontal)
Area of section, A = ( b + my) y
b =  – my
– my
Discharge Q = A • v = AC 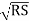
= AC 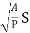
Since A, C, and S are constant in the above equation, the discharge will be maximum when perimeter P in minimum.
P = AB + BC + CD = b + 2(AB)
= b + 2 (y 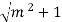 )
)
=  – my + 2y
– my + 2y 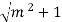
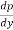 = 0
= 0
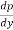 =
= 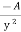 – m + 2
– m + 2 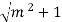 = 0
= 0
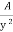 + m = 2
+ m = 2 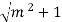
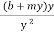 + m = 2
+ m = 2 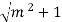
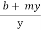 + m = 2
+ m = 2 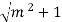
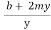 = 2
= 2 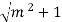
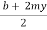 = y
= y 
 (Top width) = Sloping side
(Top width) = Sloping side
Hydraulic mean depth for this section
R =  =
= 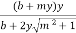
= 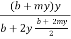
= 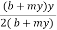
R = 
Best side slope for the trapezoidal channel for maximum discharge :
P =  – my + 2y
– my + 2y 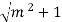
Maximum Discharge will be,
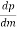 = 0
= 0
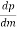 = - y + 2y x
= - y + 2y x  ( m2 + 1) -1/2 2m = 0
( m2 + 1) -1/2 2m = 0
- Y 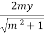 = 0
= 0
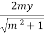 = 1
= 1
m2 + 1 = 4m2
3m2 = 1
m = 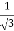
tan 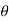 =
= 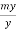 = m =
= m = 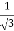
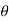 = 30°
= 30°
Condition for economical cross-section,
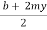 = y
= y 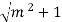
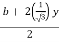 = y
= y 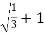
 +
+ 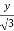 =
= 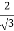 y
y
 =
= 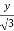
b = 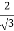 y
y
Perimeter P = b + 2y 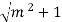
= 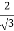 y + 2y
y + 2y 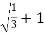
P = 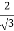 y +
y + 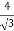 y =
y = 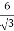 y =
y = 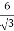 x
x 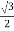 b = 3b
b = 3b
But, P = b + (AB ) + CD = b + 2 ( AB)
3b = b + 2 (AB)
AB = 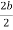 = b
= b
Sloping side of channel = Bed width of channel
2) Triangular section:
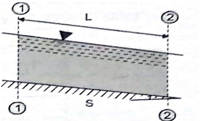
Consider a triangular section ABC
Let 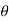 be the angle of each side with respect to vertical
be the angle of each side with respect to vertical
tan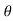 =
= 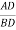 =
= 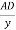
AD = DC = y tan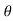
Top width AC = AD + DC = 2y tan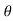
Area of section A =  x base depth =
x base depth =  ( 2y tan
( 2y tan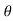 ) y
) y
A = y2 tan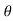 ………………………….eqn 1
………………………….eqn 1
Wetted perimeter P = AB + BC
= 2 (AB) = 2 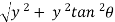 = 2y
= 2y 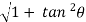
P = 2y 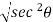 = 2y sec
= 2y sec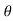 …………………eqn 2
…………………eqn 2
From equation 1, y = 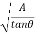
P = 2 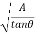 x sec
x sec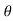
= 2 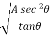
= 2 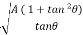
= 2 
For maximum discharge, the perimeter will be minimum,
 = 0
= 0
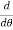 (cot
(cot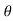 + tan
+ tan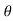 ) = 0
) = 0
Cosec2 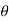 + sec2
+ sec2 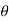 = 0
= 0
sec2 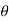 = Cosec2
= Cosec2 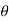
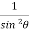 =
= 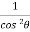
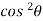 =
= 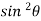
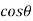 =
= 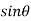
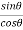 = 1
= 1
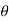 = 45°
= 45°
m = 1
From eqn 1, A = y2 tan45° = y2
Wetted perimeter P = 2y sec 45° = 2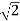 y
y
Hydraulic mean depth R =  =
= 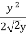 =
= 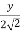
Q11. Design a most economical trapezoidal open channel with side slope 2H: 1V and a bed slope of 0.00059 to carry a discharge of 58 cumecs. Assume chezy's constant as 55.
Ans:
Side slope = 2:1
S = 0.00059
Q = 58 m3/sec
C = 55
For most economical trapezoidal section
 (Top width) = Sloping side
(Top width) = Sloping side
 = y
= y 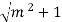 = y
= y 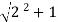
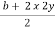 = y
= y 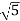
B + 4y = 2 y 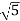
b = 0.472y
Area of trapezoidal A = (b + my) y = ( 0.472 y + 2y)y
= 2.472y2
Hydraulic mean radius R =  = 0.5y
= 0.5y
Discharge by chezy’s formula,
Q = AC 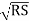
58 = 2.472y2 x 55 x 
58 = 2.3352y5/2
Y = 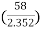 2/5 = (24)2/5 = 3.614
2/5 = (24)2/5 = 3.614
b = 0.472y =0.472 x 3.614 = 1.796m
Q12. A trapezoidal channel has to convey a discharge of 20 m3/s of water of a velocity of 1.75 m/s. The side slope of the channel is 1H to 1V. Find the sectional dimensions for the most economical conditions. Fin also the necessary bed slope of the channel if chezy’s constant is 50.
Ans:
Given:
Q = 20 m3/s
V = 1.75 m/s
Side slope m:1 = 1:1
C = 50
To find: b, y & S
Area of section A =  =
= 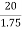 = 11.429m2
= 11.429m2
For most economical trapezoidal section
 (Top width) = Sloping side
(Top width) = Sloping side
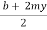 = y
= y 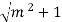 = y
= y 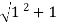
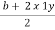 = 2
= 2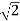 y
y
B + 2y = 2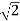 y
y
b = 0.828y
Area of trapezoidal A = (b + my) y
11.429 = (0.828 y + 1y)y
11.429 = 0.828 y2 + y
0.828 y2 + y – 11.429 = 0
Y = 3.16 m
Bottom width of section b = 0.828 *3.16 = 2.62 m
Hydraulic mean radius R =  =
= 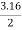 = 1.58 m
= 1.58 m
Discharge by chezy’s formula,
Q = AC 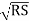
1.75 = 50 x 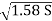
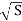 =
= 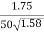
= 0.0278
= 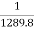
Q13. Explain in detail Specific energy and Specific force diagram.
Ans- Specific Energy:

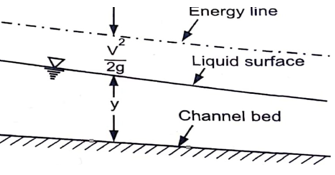
The total head (energy per unit weight) at any section with respect to any selected datum is given by
H = z + d cos 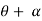
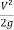 ..........................1
..........................1
Thus, from equation (1),
Specific energy E = d cos 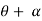
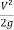 .................2
.................2
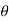
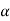
E = y + 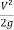 ........................3
........................3
The concept of specific energy.
Specific force:
Where y = unit weight of the liquid,
Q = discharge and
W = weight of liquid between sections 1-1 and 2-2. Ff force due to friction at the boundary of the channel. Force due to air resistance is neglected.
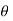
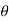
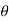
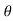
 (V2-V1) = P1-P2 ...........................2
(V2-V1) = P1-P2 ...........................2
P1 = 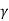 A1
A1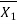 =
=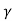 A1
A1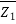
P2 =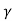 A2
A2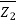
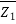
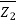
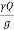 (V2-V1) =
(V2-V1) = 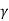 A1
A1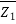 -
-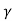 A2
A2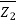
Q = A1V1 =A2V2 or V1= and V2=
and V2=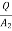
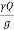 (
(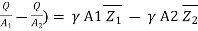
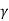
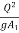 +
+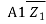 =
= 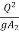 +
+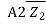 ..............................3
..............................3
F =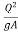 + A
+ A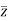 .................................4
.................................4
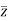
Q14. Explain the Important terms pertaining to critical flow (1)section factor, (2)the concept of first hydraulic exponent.
Ans- Critical Flow Criteria: conditions and characteristics
1) Specific energy is a minimum for a given discharge.
2) Discharge is a maximum for a given specific discharge
3) Specific force is a minimum for a given discharge.
4) Velocity head is equal to half the hydraulic depth in a channel of small slope.
5)Froude number is equal to unity.
6) Velocity of flow in a channel is equal to celebrity of small gravity waves in shallow water channel.
Section Factor-
The section factor for critical flow computation of a channel is the product of the water area and the square root of the hydraulic depth


Equation 1 & 2 are very useful for critical flow analysis.
Hydraulic exponent-
Since the section factor is a function of the depth of flow y, and A may be assume that
Z=CyM
Where C is the coefficient and M is the parameter called hydraulic exponent for the critical flow.
