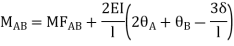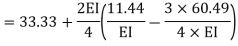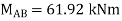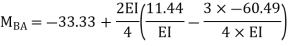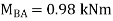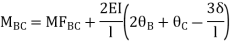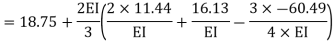Unit - 5
Stiffness method
Q1) Explain concept of fundamental concepts of flexibility and stiffness relation between them?
A1) 1. Fundamental Concepts of Stiffness Method of Analysis
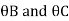

Let 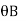 and
and 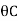 have been positive (anticlockwise).
have been positive (anticlockwise).
3. Applying imaginary restraints at the joints to prevent all joint displacements.
4. The restrained structure is assumed to be acted upon by all loads except those that correspond to the unknown displacements.
5. The actions (moments) ADL, and ADL are corresponding to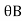 and
and 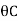 respectively and caused by the loads acting on the structure.
respectively and caused by the loads acting on the structure.
It is a matrix method that makes use of the members' stiffness relations for computing member forces and displacements in structures. The direct stiffness method is the most common implementation of the finite element method (FEM).
It is also called a displacement method.
[S]+[D]+[AM]=[AJ]
S = Stiffness Matrix
[D]=unknown joint displacement
i.e. 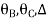
AM=Fixed end moments at particular points
AJ=External moment
2. Important Points
When 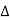 is present in your frame
is present in your frame

3. Steps for analysis at stiffness matrix
1. Find known rotation i.e., 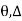
2. Find fixed end moment
3. Find AM value
4. Find S matrix
5. Put in compatibility equation [S]+[D]+[AM]=[AJ]
6. Apply S.D. equation
Q2) What is stiffness method – Structure approach only?
A2) This method is advanced tool for analyzing determinate structures. It is also referred as a displacement method. One of its advantages over the flexibility method is that it is conductive to computer programming. In the flexibility method the unknown quantities are redundant reactions that must be arbitrarily chosen, but in the stiffness method the unknowns are the joint displacements in the structure, which are automatically specified.
In this method, the structure is restrained from undergoing displacements at the joints. The restraining forces are determined at the joints. The number of artificial restraints added to make the structure restrained however, structure does not satisfy the equilibrium of forces at the joints. Displacements are then allowed to take place at the joints until artificial restraining forces vanish. The displacements are evaluated by satisfying the equilibrium conditions of the joints with the joint displacements known, the forces on the structure are determined by the superposition of the forces in the restrained structure and the forces due to displacements at the joints.
Essential features of stiffness method
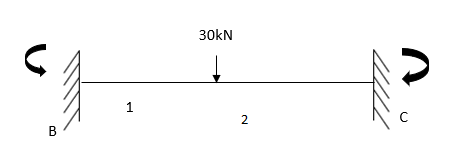
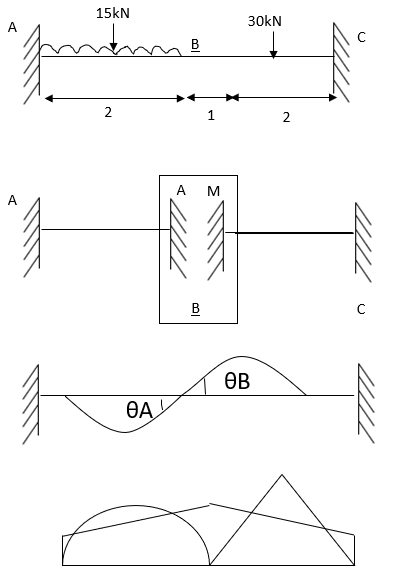
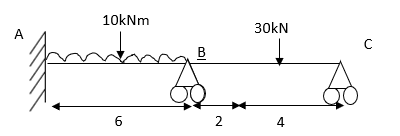
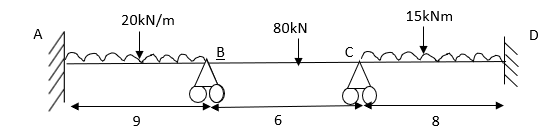
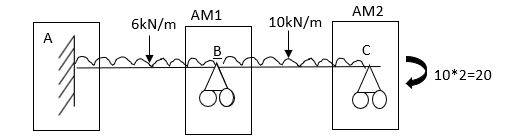
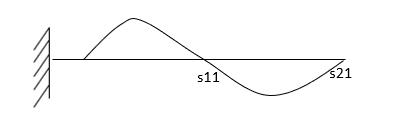
Q3) Analyze the beam shown in the figure by stiffness method Take EI= constant
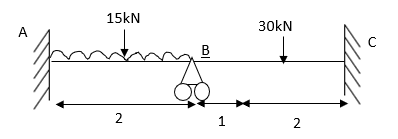
A3) Step 1) Find fixed end moment
For Span AB
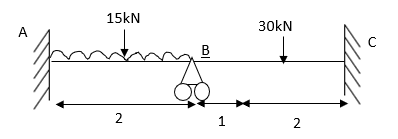
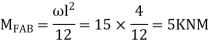
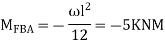
Span BC
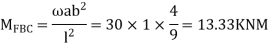
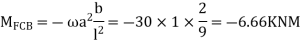
Step 2) Find AM value and Find S matrix
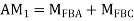
= -5+13.33
= 8.33
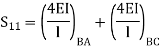
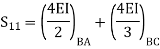
Step 3) Use compatibility equation
[S]+[D]+[AM]=[AJ]
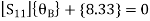
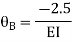
Apply SD equation for span AB

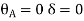
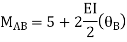
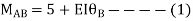
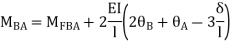
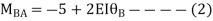
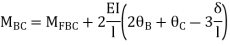
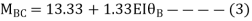
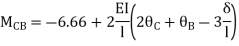
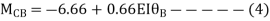
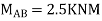
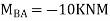
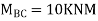
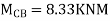
Q4) Analyze the given figure by Stiffness Method
A4) Step 1) Find Dki 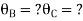
Step 2) Fixed end moments
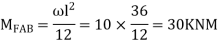
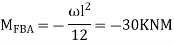
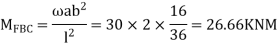
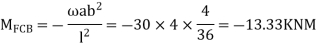
Step 3) S.D. equation
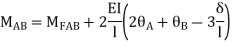
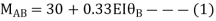
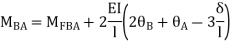
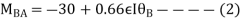
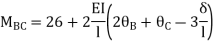
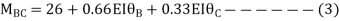
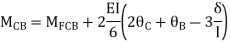
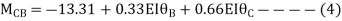
Step 4) Stiffness Matrix
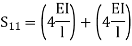
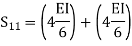
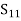 =1.33
=1.33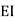
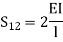
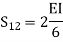
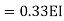
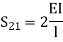
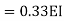
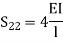

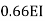

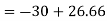
= -3.34
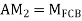
=-13.33
Step 5) Compatibility Equation
[S+[D]+[AM]= [AJ]
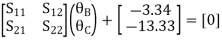
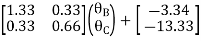
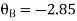 /EI
/EI
 /EI
/EI
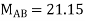
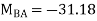
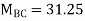
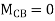
Q5) Analyse the given figure by Stiffness Method
A5) Step 1) 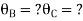
Step 2) Find fixed end moments
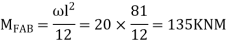
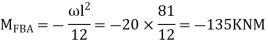
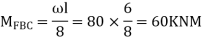
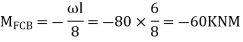

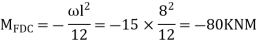
Step 3) S.D. equation
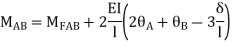
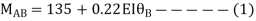
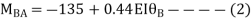
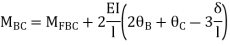
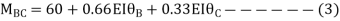
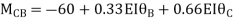 -------(4)
-------(4)

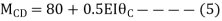

Step 4) Stiffness Matrix
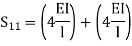

=1.11 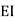
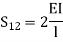
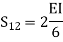
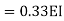
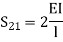
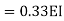
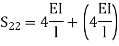
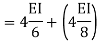
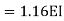
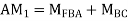
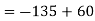
= -75
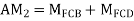
= -60+80
= 20
Step 5) Use compatibility equation
[S]{D} +{AM}={AJ}
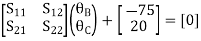
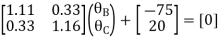
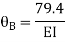

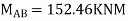
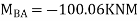
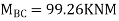
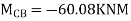
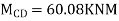
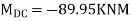
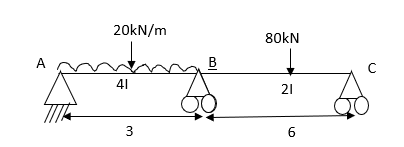
Q6) Analyze the given beam shown in the figure by stiffness method Take EI=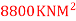
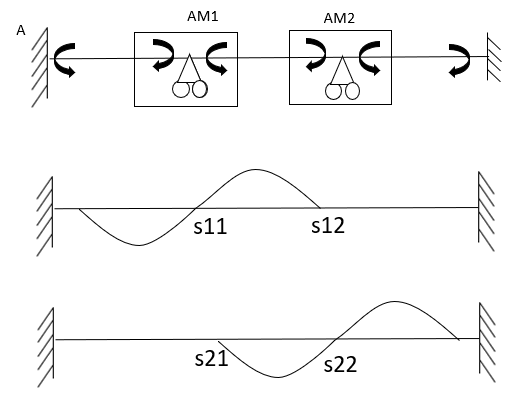
A6) Step 1) Dki =?
Step 2) Find the fixed end
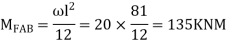
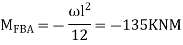
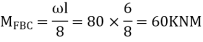
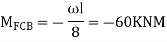
Step 3) Find AM value
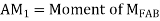
=125
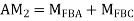
= -135+60
=-75
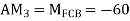
Step 4) S.D. equation
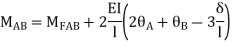
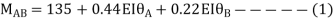
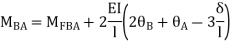

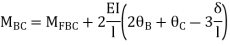
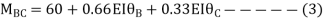
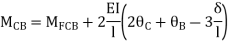
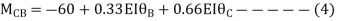
Step 5) Stiffness Matrix
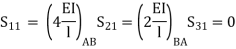
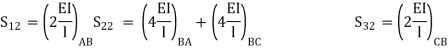
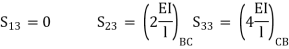
Step 6) Use compatibility equation
[S]{D} +{AM}={AJ}

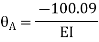
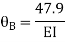
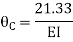
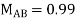 KNM
KNM
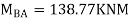
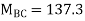 KNM
KNM
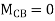 KNM
KNM
Q7) Analyze the given beam shown in the figure by stiffness method
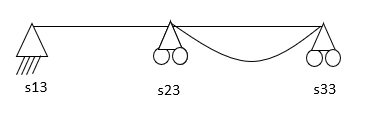
Step 1) Dki = 2
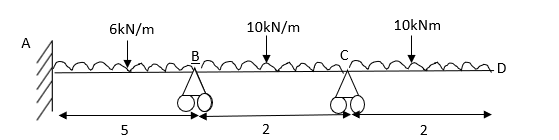
Step 2) Fixed End Moments

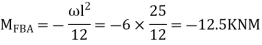
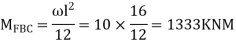
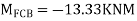
Step 3) S.D. equation
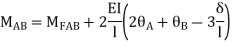
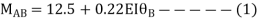
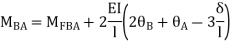
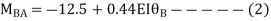
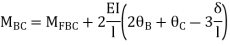

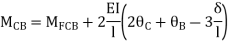
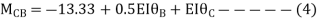
Step 4) Find AM value
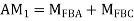
=-12.5+13.33
=0.83
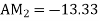
Step 5) Apply compatibility equation
[S]{D} +{AM}={AJ}
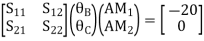
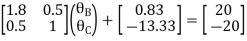
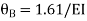
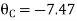 /EI
/EI
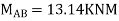
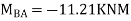
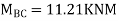
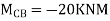
Q8) Analyze the given fig. By stiffness Matrix method take EI IS Constant

A8) Step 1) Find Dki
Step 2) Fixed end moment
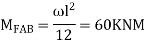
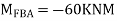
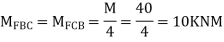
Step 3) SD equation
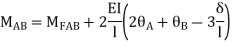

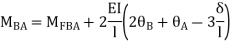
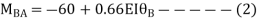

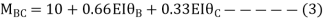
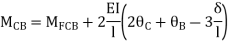
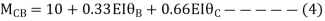
Step 4) Find value AM
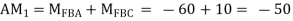
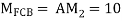
Step 5) Stiffness matrix
Stiffness Matrix
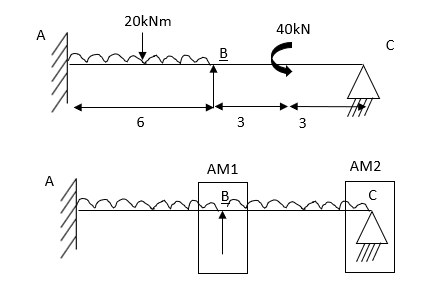
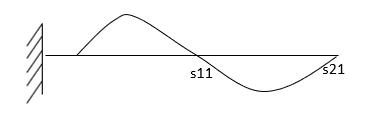
Step 6) Apply compatibility equation
[S]{D} +{AM}={AJ}
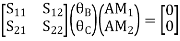
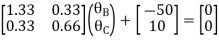

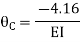
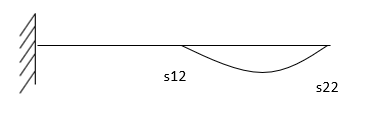
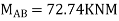
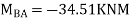
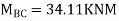
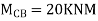
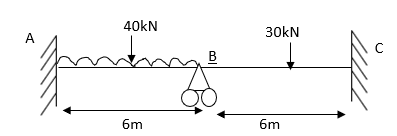
Q9) Analyze the given beam by stiffness method B is sink by 10mmEI=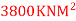
A9)
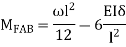
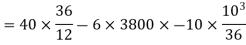
=126.33KNM
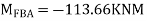
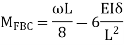

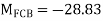
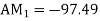
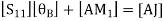
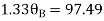
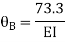
Apply S.D. equation
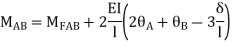
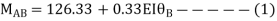
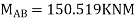
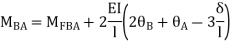
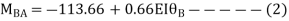
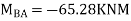
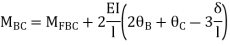

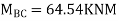
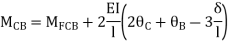
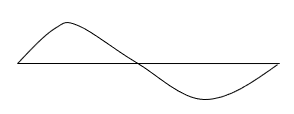
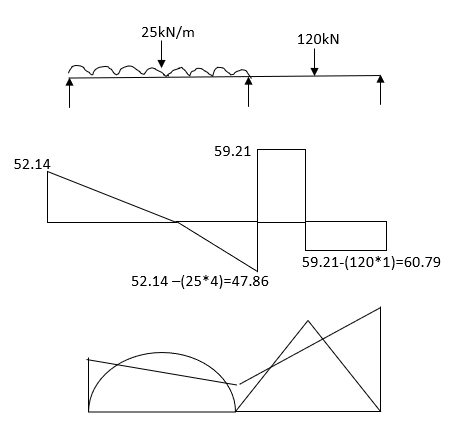
Q10) Analyze the beam by stiffness matrix method draw SFD & BMD
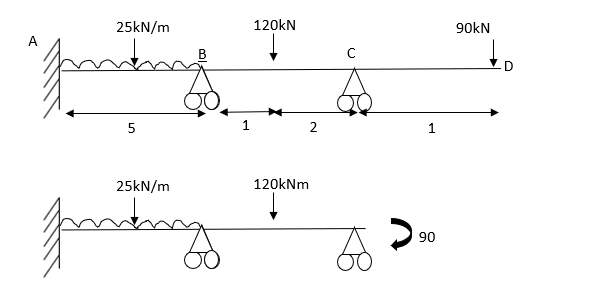
A10)
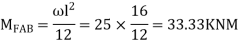
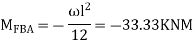
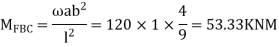
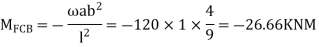
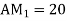
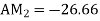
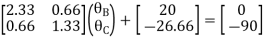
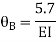
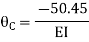
Apply S.D. equation
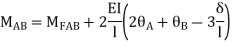

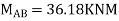
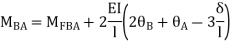
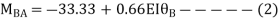
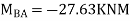
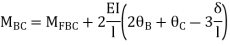
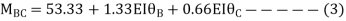
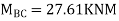
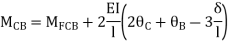
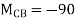
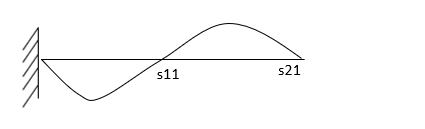
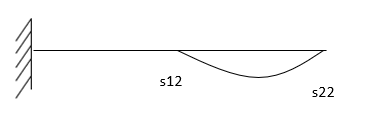
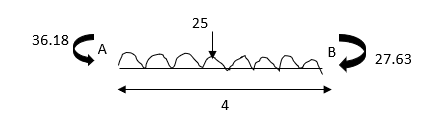
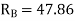
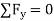
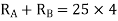
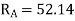
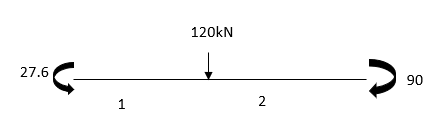
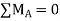
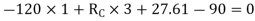
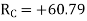

Draw SFD &BMD
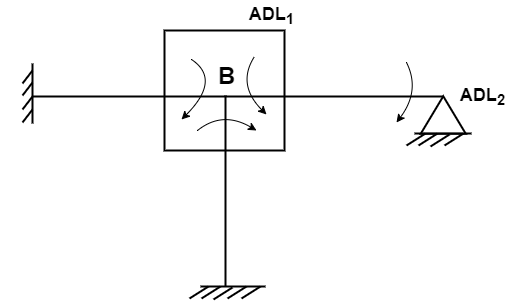
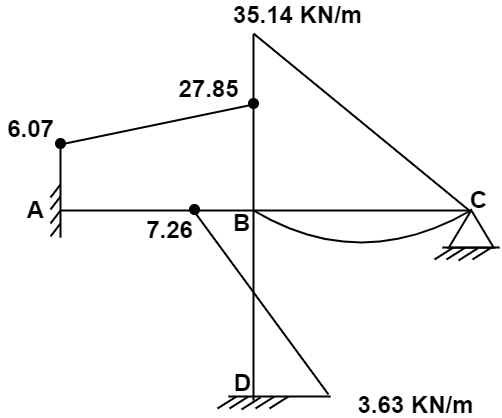
Q11) Analyse portal frame by stiffness matrix. Draw BMD Take EI= constant
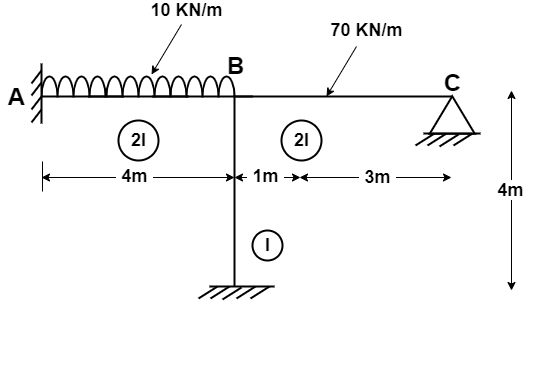
A11)
Step 1: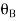 =?
=?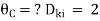
Step 2: Find fixed end Moments
For 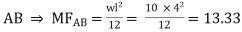
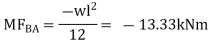
for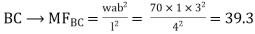
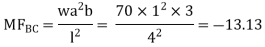
for 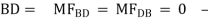 No loading
No loading
Step 3: Find ADL
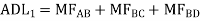
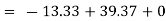

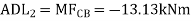
Step 4: Find stiffness matrix
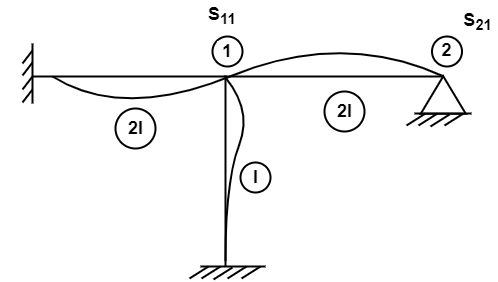
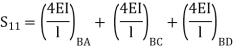
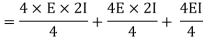
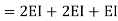
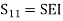
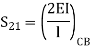
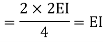
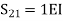
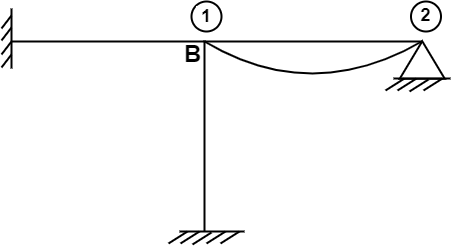

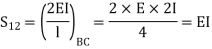
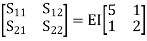
Step 5: Compatibility Equation
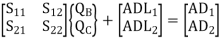
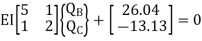
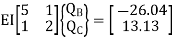
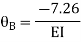
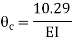
Step 6: Find final moments by slope deflection equation
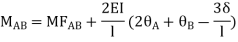
For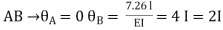
δ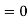

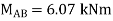

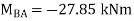
For 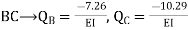

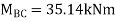
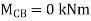
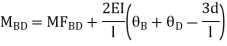
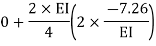
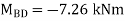
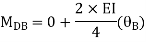
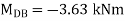
Step 7 Draw BMD
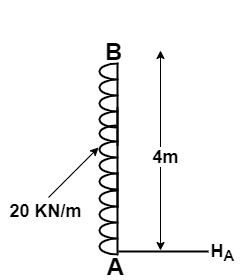
Q12) Analyze the portal frame by stiffness matrix method take EI=cost.
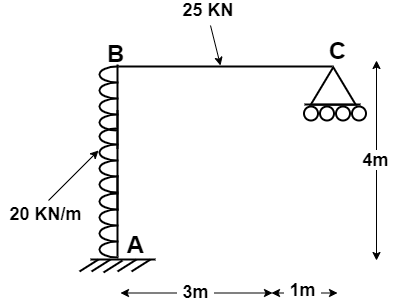
A12) Step 1: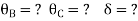
Step 2: Find fixed end moments
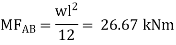
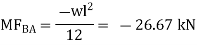
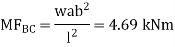
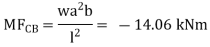
Step 3: Find 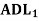
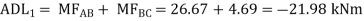
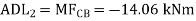
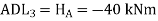
Step 4: Stiffness Matrix
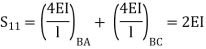
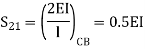
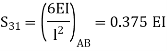
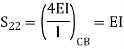
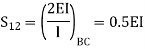
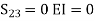
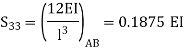
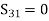
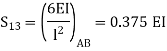
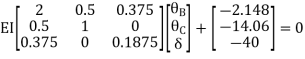
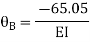
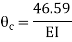
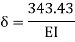
Step 5: Find final moments by S.D Equation for AB
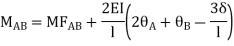
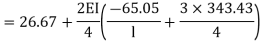
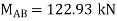
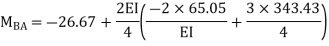
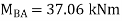
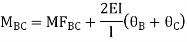
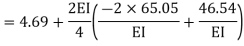
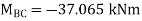
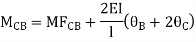
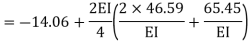
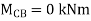
Q13) Analyze the portal frame by stiffness matrix method take EI=cost. Draw SFD and BMD
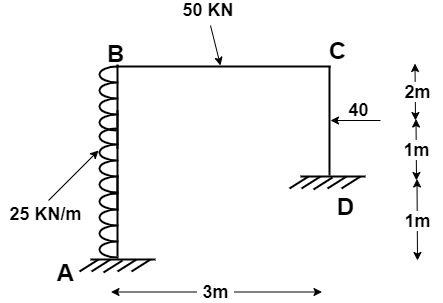
A13) Step 1: Find Dki
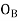 = ?
= ? 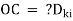 = 3
= 3 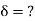
Step 2: Find fixed end moments.
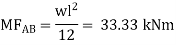

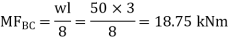
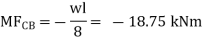
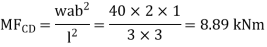
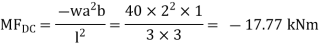
Step: 3 Find ADL


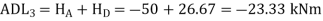
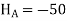
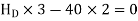
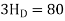
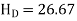

Step 4: Stiffness of Matrix
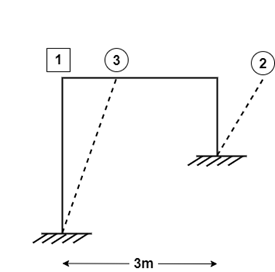
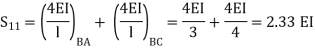
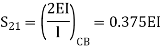
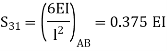
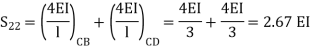
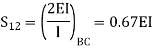
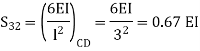
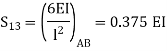
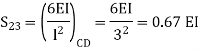

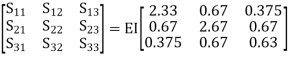
Step 5: Compatibility Equation
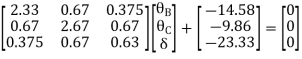
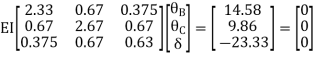
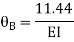
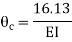
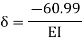
Step 6: Find final moments for AB