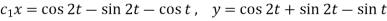Unit-1

Linear differential equations

Question-1: Solve (4D² +4D -3)y =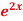 

Solution: Auxiliary equation is 4m² +4m – 3 = 0

    We get,             (2m+3)(2m – 1) = 0

                               m =   ,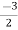

complementary function:  CF is  A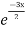+ B

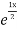
now we will find particular integral,

                         P.I. = 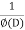f(x)

=   .

=    .

=    .

=   . =   .

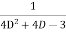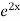
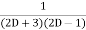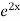
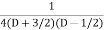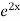
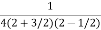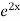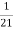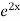
 General solution is y =  CF + PI

                                                    = A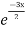+ B  .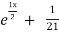

Question-2: Solve

Ans. Given,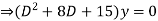

Here the Auxiliary equation is

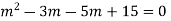
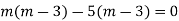
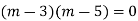
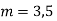
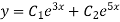
Question-3: Solve

Ans. Given,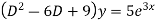

Auxiliary equation is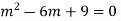

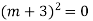
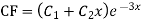
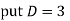
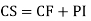
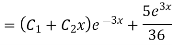
Question-4:

Ans. Auxiliary equation is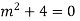

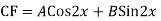
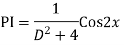
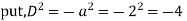
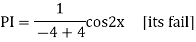
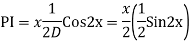
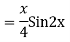
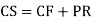
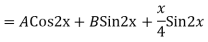
Question-5: Solve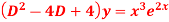

Ans. AE=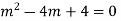

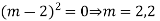
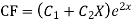
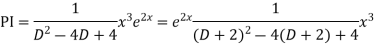
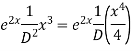
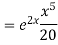
The complete solution is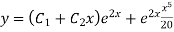

Question-6: Solve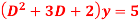

Ans. The AE is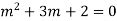

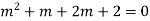
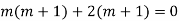
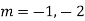
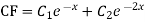
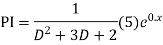
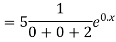
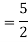
Complete solution = CF + PI

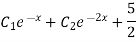
Question-7: Find the PI of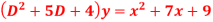

Ans.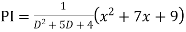

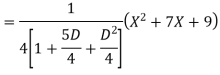
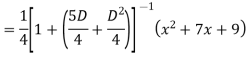
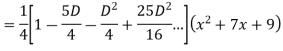
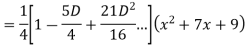
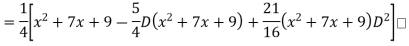
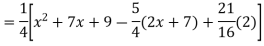
Question-8: Solve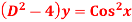

Ans. The AE is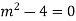

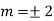
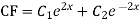
We know,

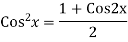
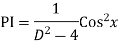
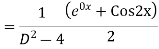
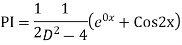
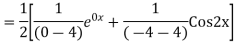
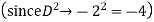
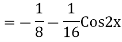
Complete solution is y= CF + PI

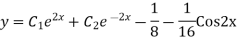
Question-9: Solve(D3-7D-6) y=e2x (1+x)

 Ans. The auxiliary equation i9s

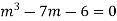
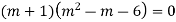
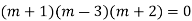
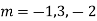
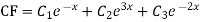
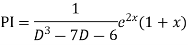
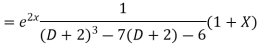
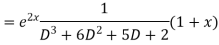
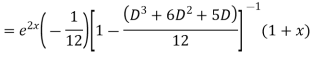
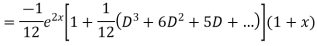
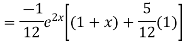
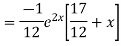
Hence complete solution is y= CF + PI

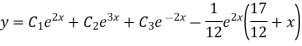
Question-10: Solve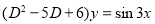

Solution:

Auxiliary equation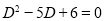

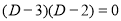
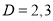
C.F is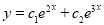

    []

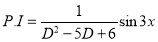
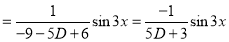
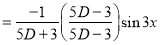
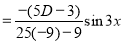
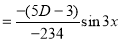
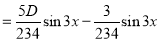
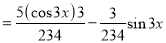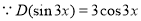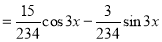
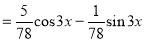
   The Complete Solution is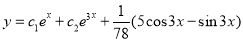  

Question-11: Solve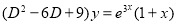

Solution:

The auxiliary equation is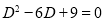     

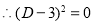
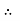The C.F is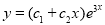

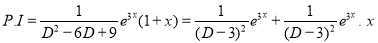
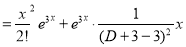
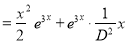
But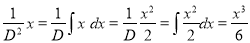

   The Complete Solution is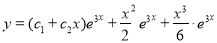

Question-12: Solve the following DE by using a variety of parameters-

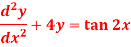
Sol. We can write the given equation in symbolic form as-

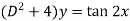
To find CF-

It’s A.E. is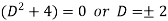

So that CF is-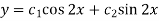     

To find PI-

Here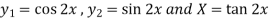

Now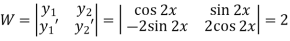

Thus PI = 

            = 

            = 

             = 

             = 

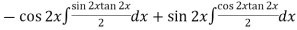
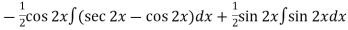
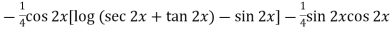
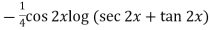
So that the complete solution is-

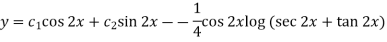
Question-13: Solve the following by using the method of variation of parameters.

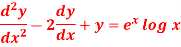
Sol. This can be written as-

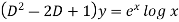
C.F.-

The auxiliary equation is-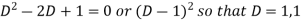

So that the C.F. will be-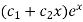

P.I.-

Here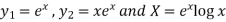

Now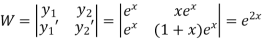

Thus PI =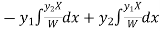 

            = 

            = 

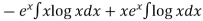
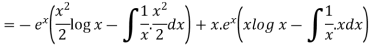
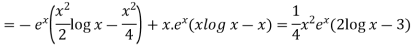
So that the complete solution is-

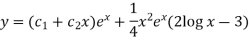
Question-14: Solve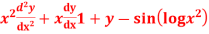

Ans. Let,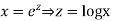

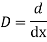
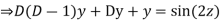
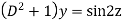
AE is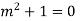

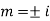
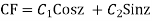
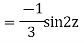
y= CF + PI

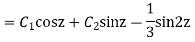
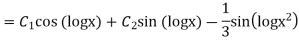
Question-15: Solve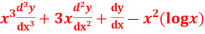

Ans. Let, 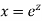so that z = log x

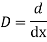
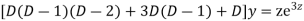
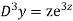
AE is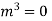

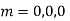
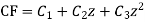
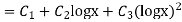
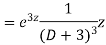
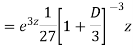
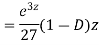
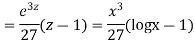
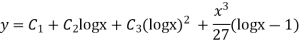
Question-16: Solve the following simultaneous differential equations-

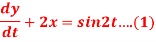
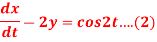
Given that x(0)=1  and y(0)= 0

Solution:

Consider the given equations,

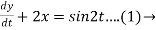Dy +2x = sin2t

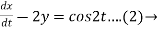Dx -2y = cos2t

By solving the above equations we get,

(D2 +4)Y =0

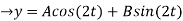
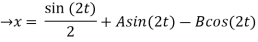
X(0) = 1, y(0) = 0

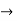A =0, B=-1

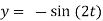
Question-17: Solve the following simultaneous differential equations-

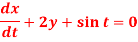
It is given that x = 0 and y = 1 when t = 0.

Sol. Given equations can be written as-

Dx + 2y = - sin t ………. (1)    and     -2x + Dy = cos t  ……… (2)

Eliminate x by multiplying (1) by 2 and (2) by D then add-

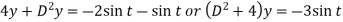
Here A.E =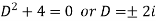

So that C.F. =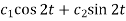

And P.I. =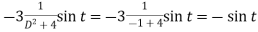

So that- 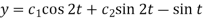   …………. (3)

And 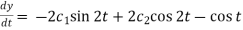  ………….. (4)

Substitute (3) in (2), we get-

 2x = Dy – cos t =

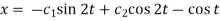  ………… (5)

When t = 0, x = 0, y = 1. (3) and (5) gives-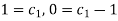 

Hence