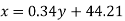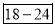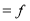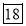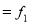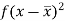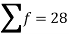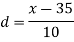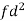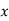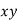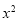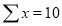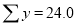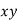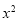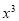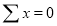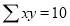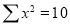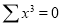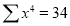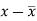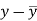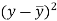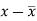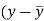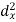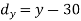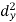undefined | unit 3 statistics

Unit-3

Statistics

Question-1: Find the mean of the following dataset.

x	20	30	40
f	5	6	4

Sol.

We have the following table-

X	F	Fx
20	5	100
30	6	180
40	7	160
	Sum = 15	Sum = 440

Then Mean will be-

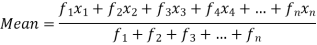
Question-2: Find the median of the following dataset-

Sol.

Class interval	Frequency	Cumulative frequency
0 - 10	3	3
10 – 20	5	8
20 – 30	7	15
30 – 40	9	24
40 – 50	4	28

So that median class is 20-30.

Now putting the values in the formula-

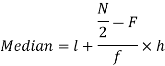
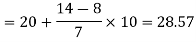
So that the median is 28.57

Question-3: Find the mode from the following data:

Age	0-6	6-12	12-18	18-24	24-30	30-36	36-42
Frequency	6	11	25	35	18	12	6

Solution.

Age	Frequency	Cumulative frequency
0-6 6-12 12-18 24-30 30-36 36-42	6 11 25 35 12 6	6 17 42 77 95 107 113

Mode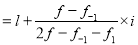

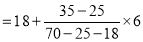
Question-4: Find the quartile deviation of the following data-

Class interval	0-10	10-20	20-30	30-40	40-50
Frequency	3	5	7	9	4

Sol.

Here N/4 = 28/4 = 7 so that the 7’th observation falls in class 10 – 20.

And

3N/4 = 21, and 21’st observation falls in the interval 30 – 40 which is the third quartile.

The quartiles can be calculated as below-

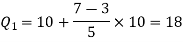
And

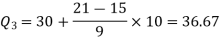
Hence the quartile deviation is-

Question-5: Find the mean deviation from the mean of the following data-

Class interval	0-6	6-12	12-18	18-24	24-30
Frequency	8	10	12	9	5

Sol.

Class interval	Mid-value	Frequency	d = x - a	f.d	\|x - 14\|	f \|x - 14\|
0-6	3	8	-12	-96	11	88
6-12	9	10	-6	-60	5	50
12-18	15	12	0	0	1	12
18-24	21	9	6	54	7	63
24-30	27	5	12	60	13	65
Total		44		-42		278

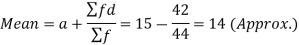
Then mean deviation from mean-

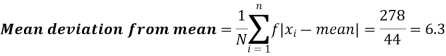
Question-6: Compute the variance and standard deviation.

Class	Frequency
0-10	3
10-20	5
20-30	7
30-40	9
40-50	4

Sol.

Class	Mid-value (x)	Frequency (f)
0-10	5	3	1470.924
10-20	15	5	737.250
20-30	25	7	32.1441
30-40	35	9	555.606
40-50	45	4	1275.504
Sum			4071.428

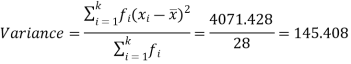
Then standard deviation,

Question-7: If the coefficient of skewness is 0.64. The standard deviation is 13 and mean is 59.2, then find the mode and median.

Sol.

We know that-

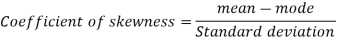
So that-

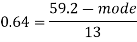
And we also know that-

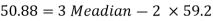
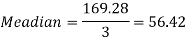
Question-8: Calculate Karl Pearson’s coefficient of skewness of marks obtained by 150 students.

Sol. The mode is not well defined so that first we calculate mean and median-

Class	f	x	CF		fd
0-10	10	5	10	-3	-30	90
10-20	40	15	50	-2	-80	160
20-30	20	25	70	-1	-20	20
30-40	0	35	70	0	0	0
40-50	10	45	80	1	10	10
50-60	40	55	120	2	80	160
60-70	16	65	136	3	48	144
70-80	14	75	150	4	56	244

Now,

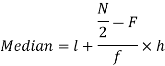
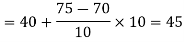
And

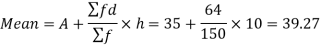
Standard deviation-

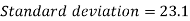
Then-

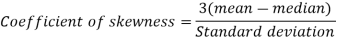
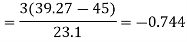
Question-9: Find the best values of a and b so that 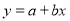fit the data given in the table.

X	0	1	2	3	4
Y	1	2.9	4.8	6.7	8.6

Solution: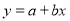


0	1	0	0
1	2.9	2.9	1
2	4.8	9.6	4
3	6.7	20.1	9
4	8.6	13.4	16

Normal equations 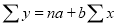…. (2)

…. (3)

On putting the values of 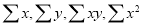in (2) and (3), we have

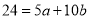…. (4)

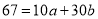…. (5)

On solving (4) and (5), we get

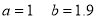
On Substituting the values of a and b in (1), we get

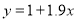
Question-10: Find the least-squares approximation of the second degree for the discrete data.

	-2	-1	0	1	2
	15	1	1	3	19

Solution:

Let the equation of second-degree polynomial be


-2	15	-30	4	60	-8	16
-1	1	-1	1	1	-1	1
0	1	0	0	0	0	0
1	3	3	1	3	1	1
2	19	38	4	76	8	16

Normal equations are

On putting the values of 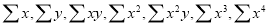in equations (2), (3), (4), we have

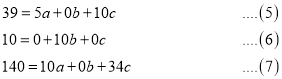
On solving (5), (6), (7), we get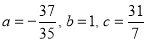

The required polynomial of the second degree is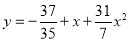

Question-11: Fit a second-degree parabola to the following data:

x	0	1	2	3	4
y	1	1.8	1.3	2.5	6.3

Solution: Let 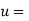and so that the parabola of fit 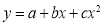becomes

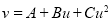…. (i)

The normal equations are

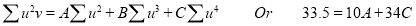
Saving these as simultaneous equations we get

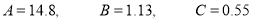
(i) becomes

Hence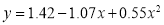

Question-12: Find the correlation coefficient between age and weight of the following data-

Age	30	44	45	43	34	44
Weight	56	55	60	64	62	63

Sol.

x	y					())
30	56	-10	100	-4	16	40
44	55	4	16	-5	25	-20
45	60	5	25	0	0	0
43	64	3	9	4	16	12
34	62	-6	36	2	4	-12
44	63	4	16	3	9	12
Sum= 240	360	0	202	0	70	32

Karl Pearson’s coefficient of correlation-

Here the correlation coefficient is 0.27.which is the positive correlation (weak positive correlation), this indicates that as age increases, the weight also increases.

Question-13: Find the correlation coefficient between the values X and Y of the dataset given below by using the short-cut method-

X	10	20	30	40	50
Y	90	85	80	60	45

Sol.

X	Y
10	90	-20	400	20	400	-400
20	85	-10	100	15	225	-150
30	80	0	0	10	100	0
40	60	10	100	-10	100	-100
50	45	20	400	-25	625	-500
Sum = 150	360	0	1000	10	1450	-1150

Short-cut method to calculate correlation coefficient-

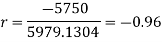
Question-14: Two variables X and Y are given in the dataset below, find the two lines of regression.

x	65	66	67	67	68	69	70	71
y	66	68	65	69	74	73	72	70

Sol.

The two lines of regression can be expressed as-

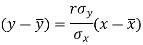
And

x	y			xy
65	66	4225	4356	4290
66	68	4356	4624	4488
67	65	4489	4225	4355
67	69	4489	4761	4623
68	74	4624	5476	5032
69	73	4761	5329	5037
70	72	4900	5184	5040
71	70	5041	4900	4970
Sum = 543	557	36885	38855	37835

Now-

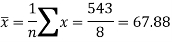
And

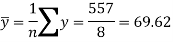
The standard deviation of x-

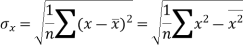
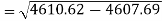
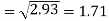
Similarly-

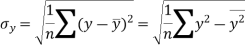
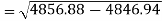
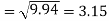
Correlation coefficient-

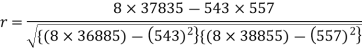
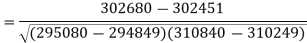
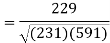
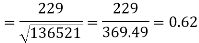
Put these values in the regression line equation, we get

Regression line y on x-

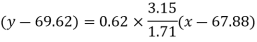
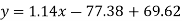
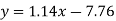
Regression line x on y-

Sign Up