Unit-1

Power System Stability

1. What is stability?

Stability is the tendency of the power system (and of the synchronous machines in particular) to continue to operate steadily in the intended mode. It is also a measure of the inherent ability of the system to recover from extraneous disturbances (such as faults, lightning, and changes of load), as well as from planned disturbances (such as switching operations).

2.     What is Power Stability?

Power system stability may be broadly defined as the property of a power system that enables it to remain in the state of operating equilibrium under normal operating conditions and to regain an acceptable state of equilibrium after being subjected to a disturbance.

3. Explain the dynamics of synchronous machine?

Kinetic Energy (KE) at rotor of synchronous machine is given by

KE=12Jsm2*10-6         (1)

J = Rotor moment of inertia in Kg-m2

ωsm = Synchronous speed in rad(Mech)/s

ω=P2sm= Rotor Speed in rad (Elect)/s

P= Number of poles of machine

KE=12J2P2Js2*10-6s   (2)

=12Ms (3)

Where, 

M=J2P2s2*10-6 (4)

= Moment of inertia in MJ-s/rad (Elect)

The inertia constant H should be such that

GE=KE==12Ms*MJ (5)

Where,

G= Machine raring (Base) in MVA (3 Phase)

H=Inertia constant in MJ/MVA or MW-s/MVA

It follows,

M=2GHs=2GHf  MJ-s/Degree (Elect) (6)

M is also called the inertia constant.

Taking G as base, the inertia constant in p.u. Is

Mpu=Hπf  s2/ Degree (Elect) (7)

4. What is swing equation?

Under normal operating conditions the relative position of rotor axis and the resultant magnetic field axis Is fixed stop the angle between the two is known as power angle or torque angle. During any disturbance, the rotor will decelerate or accelerate with respect to the synchronously rotating air gap MMF, and a relative motion begins. The equation describing this relative motion is known as the swing equation.

Figure 1 shows the torque, speed and flow of mechanical and electrical were in a synchronous machine. It is assumed that the windage, friction and n-loss torque is negligible. The differential equation governing the rotor namics can then be written as

Jd2mdt2=Tm-Te Nm (8)

Where, 

θm = angle in rad (mech)

Tm = turbine torque in Nm; it acquires a negative value for a motoring machine 

Te = electromagnetic torque developed in Nm; it acquires negative value for a motoring machine

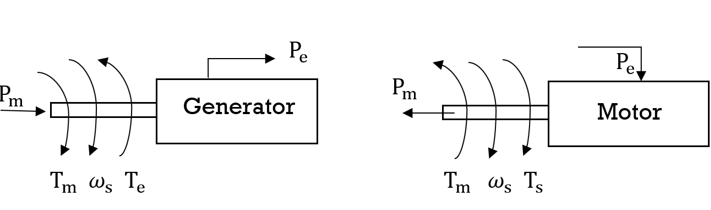
Figure 1. Flow of Mechanical and Electrical power in Synchronous Machine 

While the rotor undergoes Dynamics of Synchronous Machine as per Eq. (8), the rotor speed changes by insignificant magnitude for the time period of interest (1s). Equation (8) can therefore be converted into its more convenient power form by assuming the rotor speed to remain constant at the synchronous speed (ωsm). Multiplying both sides of Eq. (8) by ωsm′we can write

Jωsmⅆ2mⅆt210-6=Pm-PMW (9)

Where

Pm = mechanical power input in MW

Pe = electrical power output in MW; stator copper loss is assumed negligible.

Rewriting Eq. (9)

(J(2P)2s10-6)ⅆ2eⅆt2=Pm-PeMW  (10)

Where,

e=angle in rad(elect)

Or                                   

Mⅆ2eⅆt2=Pm-Pe (11)

It is more convenient to measure the angular position of the rotor with respect to a synchronously rotating frame of reference. 

Let,

δ=θe– ωst; (12)

Rotor angular displacement from synchronously rotating reference frame (called torque angle/power angle)             

ⅆ2eⅆt2=ⅆ2ⅆt2                                                            (13)

Hence Eq. (11) can be written in terms of δ as

Mⅆ2ⅆt2=Pm-PeMW  (14)

With M as defined in Eq. (6), we can write

GHπfⅆ2ⅆt2=Pm-PeMW  (15)

Dividing throughout by G, the MVA rating of the machine,

M(pu)ⅆ2ⅆt2=Pm-Pe (16)

In pu of machine rating base.

Where,

M(pu)ⅆ2ⅆt2=Pm-Pe (17)

In pu of machine rating base.

This equation (16) & (17), is called theswing equation and it describes the rotor dynamics for a synchronous machine (generating/motoring). It is a second-order differential equation where the damping term (proportional to dδ/dt) is absent because of the assumption of a lossless machine and the fact that the torque of damper winding has been ignored. Damping helps to stabilize the system. Damping must of course be considered in a dynamic stability study. Since the electrical power Pe depends upon the sine of angle δ, the swing equation is a non-linear second-order differential equation.

5. Explain multimachine system?

In a multimachine system a common system base must be chosen.

Let,

Gmach=machine rating(base)

Gsystem=system base

Equation (17) can then be written as

GmachGsystem=(Hmachfⅆ2ⅆt2)=(Pm-Pe)GmachGsystem

Hsystemπfⅆ2ⅆt2=Pm-Pe  pu in system base                                                           (18)

Where,

Hsystem=Hmach(GmachGsystem) (19)

= System inertia constant in system Base

6. Explain how the machine swings coherently?

Consider the swingequations of two machines on a common system base. 

H1πfⅆ21ⅆt2=Pm1-Pe1pu (20)

H2πfⅆ22ⅆt2=Pm2-Pe2pu (21)

Since the machine rotors swing together (coherently or in unison)

1=2=δ

Adding Equations (20) and (21)

Heqπfⅆ2ⅆt2=Pm-Pe (22)

Where,

Pm=Pm1+Pm2 

Pe=Pe1+Pe2 (23)

Heq=H1+H2

The two machines swinging coherently are thus reduced to a single machine as in Eq. (22). The equivalent inertia in Eq. (23) can be written as

Heq=H1machG1machGsystem+H2machG2machGsystem (24)

The above results are easily extendable to any number of machines swinging coherently.

7. Explain power angle curve?

Power Angle curve and power Angle equation

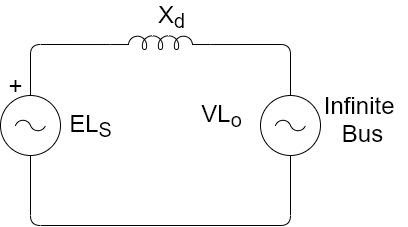
Figure A synchronous machine loaded at its terminal

Figure shows a synchronous machine having a direct axis synchronous reactance Xd.

E=E<δ Voltage behind synchronous reactance of generator

V=Terminal voltage of generator The complex power output of generator is

S=VI*

=VE<δ-VXd*

=|E||V|)Xd<(90-8)-(J|V|2)Xd

=|E||V|)XdSinδ+jEVXdCosδ-V|2Xd

The real power output Pe of generator is

Pe=ReS=EVXdsinδ=Pmsinδ

Pe=EVXdsinδ

Pm=(|E||V|)Xd

Thus, the real power output depends upon |E|,|V|, Xd and power angle ,

The reactive power Q is:-

Qe=ImS=EVXdcosδ-V|2Xd

Figure below shows the steady state Real power variation with Power angle for both generator and motor action for constant values of E, V and Xd.  Thus, the curve is known as Power Angle curve.

+δ=E leading V,=>Generator Action

-δ=E Lagging V,=>Motor Action

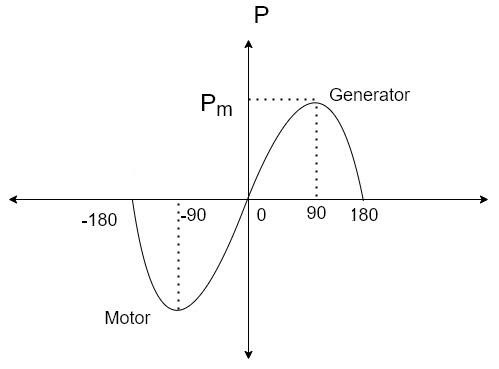
The maximum value of for successful operation is 90. In actual practice  is kept around 30.

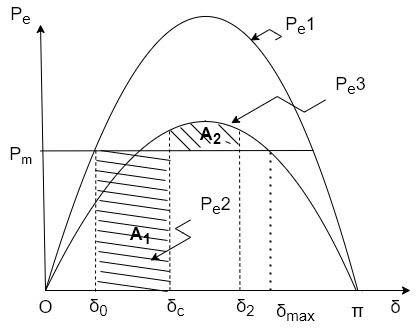
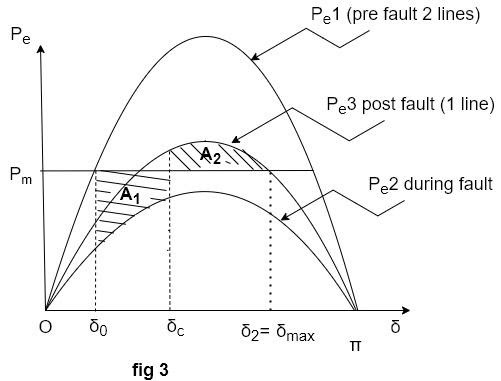
8. Explain equal Area Criterion?

The stability of single machine swing with respect to an infinite bus can be studied by use of equal area criterion. Figure shows power angle curve for an equivalent generator and representing a power exporting area connected to infinite bus.

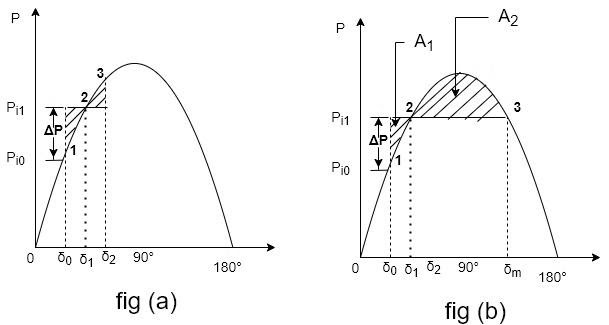
1. Equal area criterion for a large sudden change in load.
2. Limiting case when m=180-1

Suppose under steady state condition at 0  large local load ∆P within the power exporting area is switched off. Assuming mechanical input remains same. The excess input is ∆P the rotor is accelerated leading to increase in .

If Pi1=Pi0+ΔP

Then the accelerating power Pa decreases from ∆P to zero.

By the time 0 goes to 1,  the rotor absorbs kinetic energy, equals to the sheded area A1 At point 2 the accelerating power is zero. But rotor acquires speed slightly greater than the synchronous speed, and   continuous to increase beyond 1.

As Becomes greater than. δ,Pa becomes negative and rotor retards. Rotor swings to continues to increase till load angle 2 is and rotor attains the speed equal to synchronous speed

Neglecting all losses, the load angle. 2  Can be obtained from the condition that kinetic energy (KE) 

KE during swing from 0 to δ1

=KE returned at its swing 1 to δ2.

This lead to conclusion that 

Area A1=Area A2

This is referred as Equal area criterion.

The accelerating area A1 is given by

A1=01Pi1-PinSinδⅆδ

=Pi1(1-0)-Pm(cosδ0-cosδ1)

The decelerating area A2 is given by

A2=12PmSinδ-Pi1ⅆδ

=Pm(cosδ1-cosδ2)-Pi1(2-1)

For stability A1=A2

The equal area criterion can be Proved Mathematically as follows:

ⅆ2ⅆt2=PaM

Multiplying above equation by ⅆδⅆt on both sides.

ⅆδⅆt=∫2PaMⅆδⅆtⅆt0.5=2M∫Paⅆδ0.5

The load angle will have a maximum value when 

ⅆδⅆt=0 i.e. when 0mPaⅆδ=0

Thus, the rotor swings from to maximum value.   Which time the relative velocity is zero. The two shaded areas A1 and A2 must be equal and opposite sign this is shown in figure b

9.Explain the application of equal angle criterion?

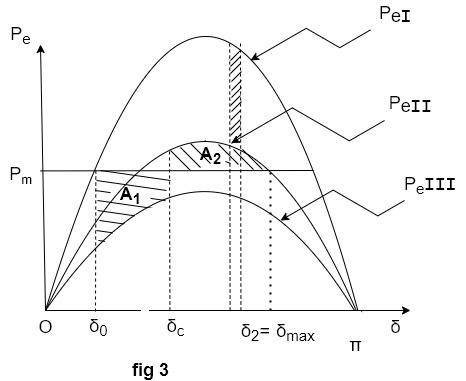
1. Switching operation sudden loss of one of parallel line.

Figure a show inter connector consisting of two parallel lines connecting an equivalent generator (representing a power exporting area) equal area criterion can be used to study the transient stability of the system which one of the lines is switched out (simultaneously at both ends). To power angle diagrams are involved.

When both lines are operating power transfer is given by

Pe=EVXd+X1X2X1+X2=Sinδ           [curve-1]

In figure (b) when line 2 is switched off(out), the transfer reactance increases and the power transfer is given by

Pe'=EVXd'+X1sinδ        curve-2

Figure(a) A machine connected to an infinite bus through an interconnector

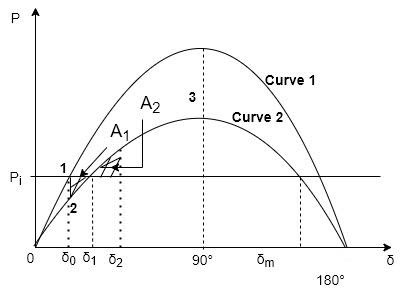
Figure equal area criterion as applied to the stability study of a switching operation.

The input to generator is Pi and therefore the initial conditions are represented by point 1 and the initial load angle is0.   When line 2 is switched off the operating point shifts to point 2 on curve 2 

The power output is less than the power input and rotor accelerates, at =1  input and output are equal but angle continues to increase because rotor has acquired speed slightly greater than the synchronous speed. When δ>1  deceleration of rotar starts swinging back. After few oscillations the load angle will stabilize at 1  If the initial power transfer is increased (line Pi shifts up words in figure b) a limit is reached beyond which the decelarating area A2 cannot be equal to accelerating area A1. The maximum value which  can attain without loss of system stability as m   And equals (π-1)  radians.

Sudden change in Mechanical input:

Figure above shows the transient model of a single machine connected to infinite bus bar. The electrical power transmitted is given by

Pe=(|E||V|)Xd'+Xesinδ=Pmax Sinδ

Under steady operating condition 

Pma=Pea=PmaxSinδ0

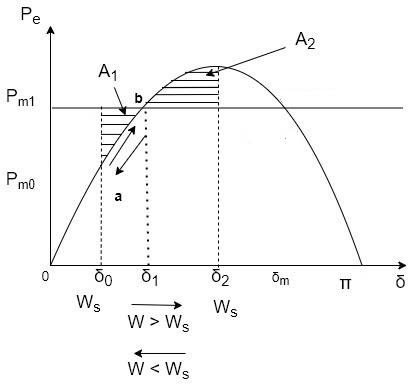
Let the mechanical input to the rotor be suddenly increased to Pm1 .the accelerating power pa=Pm1-Pe causes the rotor speed to increase (w>ws)and so dose the rotor angle. 

At angle pa=Pm1-Pe (PmaxSinδ0)=0 at point b but the rotor angle continues 

Increases as w>ws

Pa now become negative (decelerating)the rotor speed continuous to increase till at angle s2 ,

W=wsonce again (state point a,b,c) the decelerating area A1 (shaded). i.e

02Paⅆδ=0

As the rotor speed is decelerating, the speed reduces below ws , and rotor angle begins to reduce. The sate point now traverses the Pe-δ curve in opposite direction as indicated by arrows in figure The system oscillations about new steady state point b.

After oscillations die out due to inherent system damping, new style state arrives where  Pm1=Pe=PmaxSinδ1 

From figure areas A1 and A2 are given by 

A1=01Pm1-Peⅆδ

A2=12Pe-Pm1ⅆδ

As Pm1 is increased limiting condition is finally reached when A1 equals to the area above Pm1line  as shown in figure © below . Under this condition 82 acquires the maximum value such that 

2=max=π-1=π-sin-1Pm1Pmax

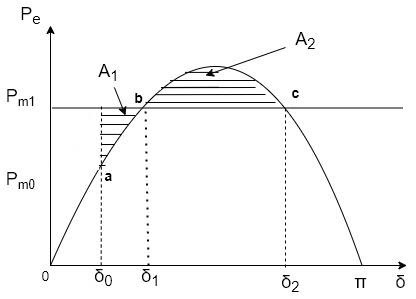
Fig.(c) limited case of transient stability with mechanical input suddenly increased 

Any further increase in Pm1 means area A1 is less than A2 ,excess kinetic energy causes the s to increase beyond point © and accelerating power with system consequently becoming unstable. 

It can be seen from above fig that through the rotor may oscillate beyond 90=s the system will remain in stable state as long as equal area criterion is met. [=90 for stability does not apply for transient stability].

10. Explain the effect of clearing time on stability?

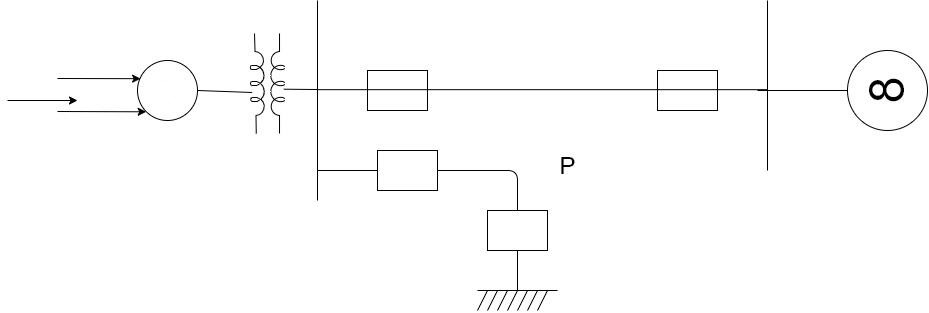
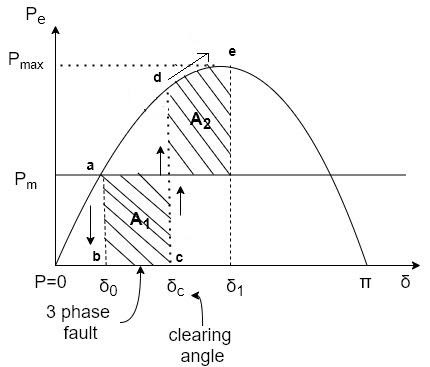
The system in fig (a) operating the mechanical input pm at steady state angle of s0(Pm-Pe) at point in fig (b) 

If a 3-phase fault occurs at point p (fig-a) pe=0 rotor state point drop to b, the acceleration begins also rotor angles moves b to c.

At time tc and angle sc, fault is cleared by opening circuit breaker tc=clearing time. c=clearing angle. After this system transmits  Pe=PmaxSinδ0. The rotor decelerates and curve moves along area A2 (i.e d point towards e point) if S1 is such that area A1=A2

System is stable. And system settles down to point a in an oscillatory manner.

Relationship both tcandc

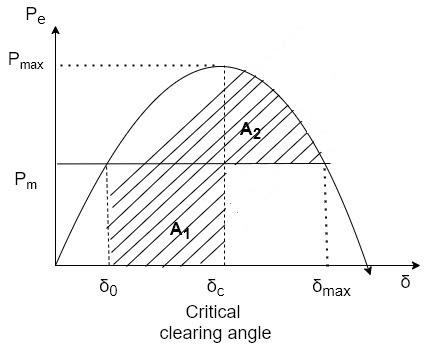
If fault clearing is delayed the angle 1 (fig-b) moves further to point  max (fig-c) for clearing time is larger than this (Also the angle) then A2<A1 (i.e. system unstable).

The critical time =maximum allowable value of clearing time.

Critical angle clearing= Maximum allowable clearing angle

Relationship for critical angle c and critical time tc -Angle are in radians

From figure ©

Max=π-0-----1

Pm=Pmaxsinδ0-----2

A1=0crPm-0ⅆδ=Pmcr-0

A2=crmaxPmaxsinδ-Pmⅆδ

=Pmax(cosδcr-cosδmax)-Pm(max-cr)

For stable system A1=A2

Cosδcr=PmPmaxmax-0+cosδmax------3

Values from equation I and II putting in equation III

Cr=cos-1π-20sinδ0-cosδ0----4

During this period fault persists, the swing equation is:-

ⅆ2ⅆt2=πfHPm;Pe=0

For getting values of cr and tcr integrating above equation twice:-

δ=πf2HPmt2+0

Cr=πf2HPmtcr2+0---Critical clearing angle

Tcr=2Hcr-0πfPm---Critical clearing Time

Cr is given by equation IV also

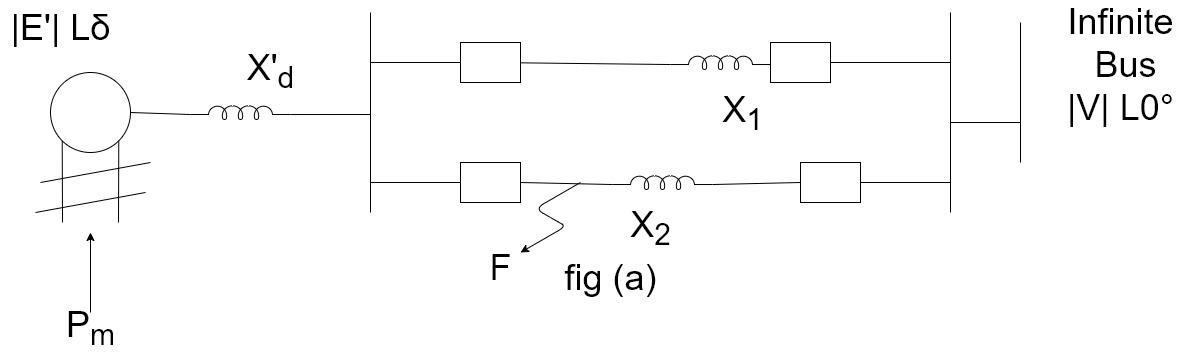
11.What is Sudden short circuit on one of parallel line

Case-a Short circuit at one end of line.

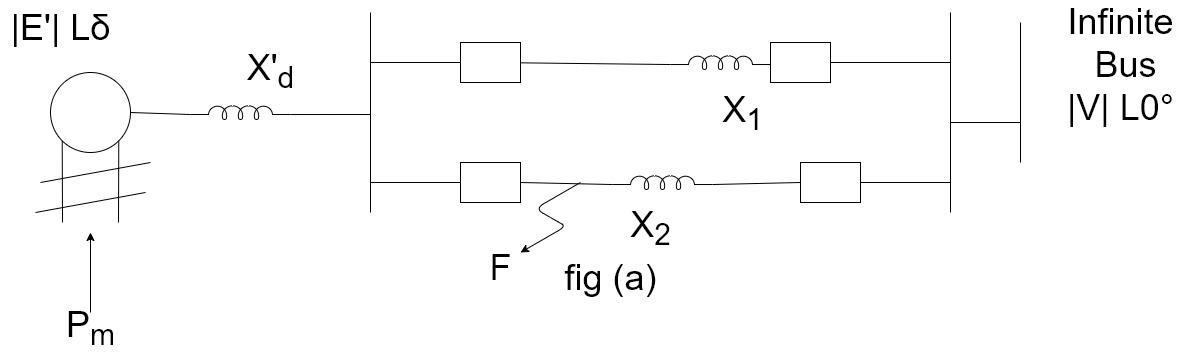
For Fig a assume a 3-phase fault F at one line 2

Before fault Power Angle curve is given by 

Pe1=E'VX'd+X1||X2Sinδ=Pma×1Sinδ

Plotted in fig (b) below

During fault Pe=0

The rotor accelerates, angle  increases the circuit breakers are opened at time tc (corresponding to c) clearing time, disconnecting the faulted line.

The power flow is now restored and reactance is now higher. (i.e. X2 instead of X1||X2).

Pe3=E'VXd'+X1Sinδ

Rotor now decelerates.

As area A1 depends upon clearing time tc(corresponding to clearing angle c) clearing time must be less than a value (critical clearing angle cr) critical clearing angle is important as we can find in form curve not critical time.

System will be stable if A1=A2before  reachesmax.

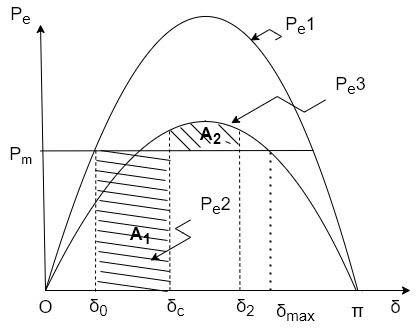
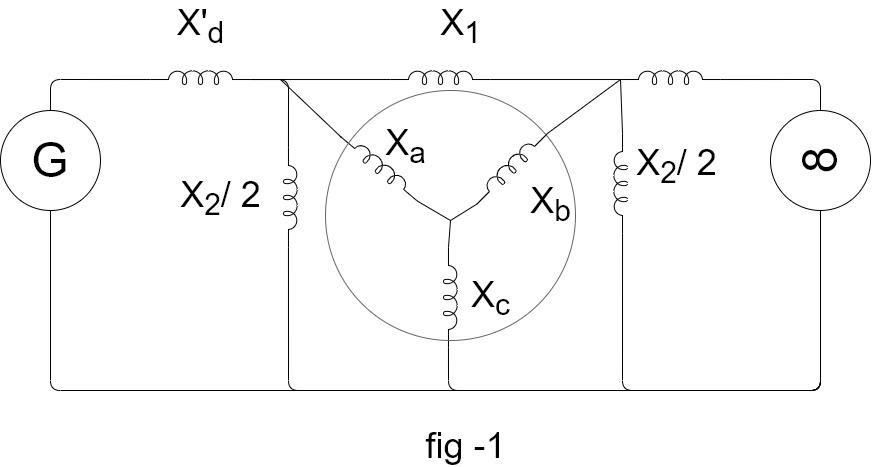
Case-b Short circuit away from line ends (Middle of  a line)

[Refer fig a in Case-a]

If Fault occurs in middle of line, power flow changes and the system can be modelled as shown in fig (1) below

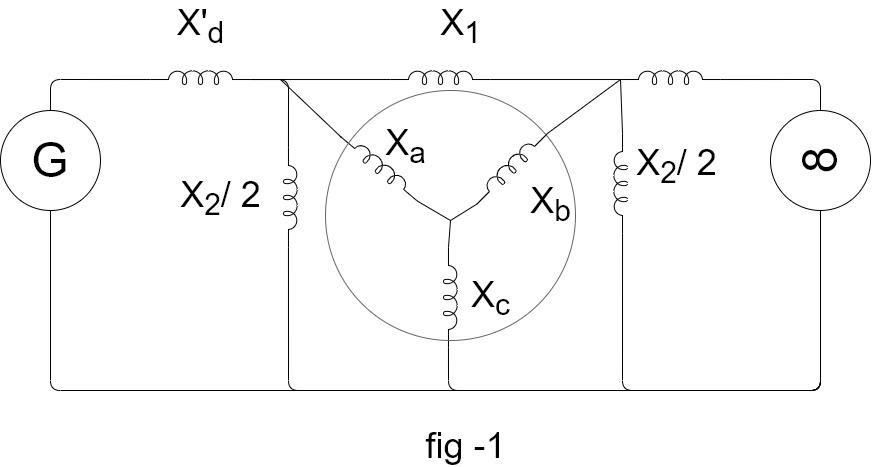
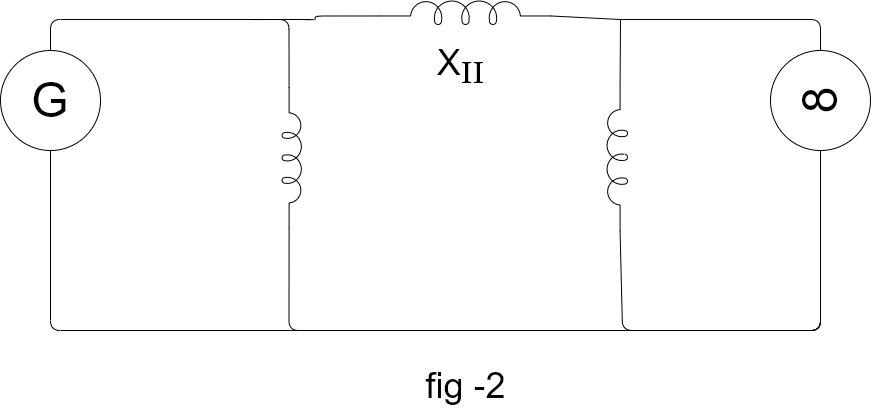
First converted to star and then Delta above Figure becomes

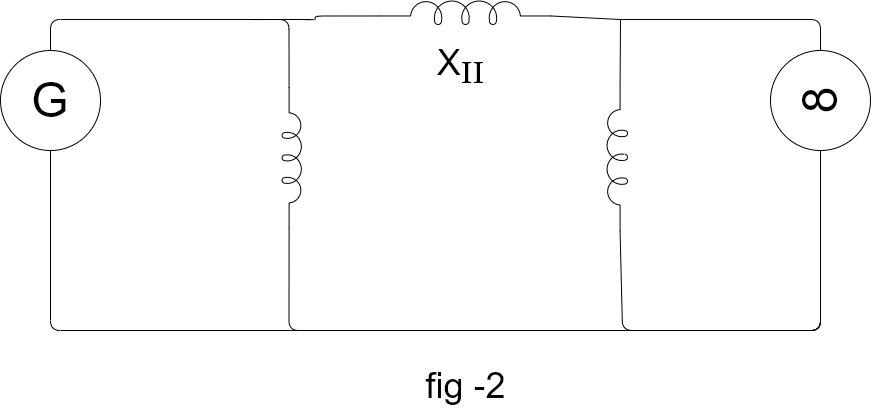
The power angle curve during fault is given by 

PeII=E'VXIISinδ=PmaxIISinδ

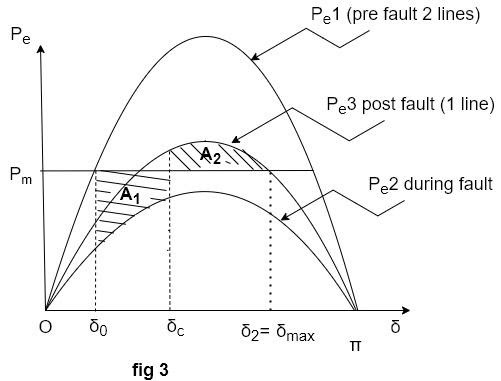
Area A1 corresponding to given clearing angle c is less than case-a1

As the clearing angle is increased area A1 increases, and to find A1=A22 is increased till it has a value max. This critical clearing angle maximum is shown in fig 3

Applying equal area criterion 

0crCPm-PmaxIIISinδⅆδ=crmaxPmaxIIISinδ-Pmⅆδ

Max=π-Sin-1PmPmaxIII

Integrating-> 

Pmδ+PmaxIICosδ|cr0+PmaxIIICosδ+Pm|maxcr=0

Or Pmcr-0+PmaxiCosδcr-Cosδ0+Pmmax-cr+PmaxIIICosδmax-Cosδcr=0

Or

Cosδcr=Pmmax-0-PmaxIICosδ0+PmaxIIICosδmaxPmaxIII-PmaxIIi

Critical clearing angle can be calculated from above equation for Case-b

Case-C Reclosure

[Refer Fig a in case -a]

If the circuit breaker on line -2 (on which fault has occurred ) is closed after clearing a transient fault. The power transfer becomes –

Pe±V=PeI=Pmax1Sinδ

As the chances of stable operation improve, shown in figure below.

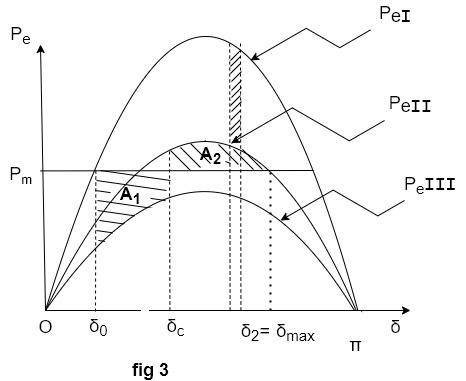
For critical clearing Angle

1=max=π-sin-1PmPmaxI

0crPm-PmaxIISinδⅆδ=crδrcPmaxIIISinδ-Pmⅆδ+rcmaxcPmaxISinδ-Pmⅆδ

Where trc=tcr+τ;{τ=Time between clearing and reclosure)

Sudden short circuit on one of parallel line

Case-a Short circuit at one end of line.

For Fig a assume a 3-phase fault F at one line 2

Before fault Power Angle curve is given by 

Pe1=E'VX'd+X1||X2Sinδ=Pma×1Sinδ

Plotted in fig (b) below

During fault Pe=0

The rotor accelerates, angle  increases the circuit breakers are opened at time tc (corresponding to c) clearing time, disconnecting the faulted line.

The power flow is now restored and reactance is now higher. (i.e. X2 instead of X1||X2).

Pe3=E'VXd'+X1Sinδ

Rotor now decelerates.

As area A1 depends upon clearing time tc(corresponding to clearing angle c) clearing time must be less than a value (critical clearing angle cr) critical clearing angle is important as we can find in form curve not critical time.

System will be stable if A1=A2before  reachesmax.

Case-b Short circuit away from line ends (Middle of  a line)

[Refer fig a in Case-a]

If Fault occurs in middle of line, power flow changes and the system can be modelled as shown in fig (1) below

First converted to star and then Delta above Figure becomes

The power angle curve during fault is given by 

PeII=E'VXIISinδ=PmaxIISinδ

Area A1 corresponding to given clearing angle c is less than case-a1

As the clearing angle is increased area A1 increases, and to find A1=A22 is increased till it has a value max. This critical clearing angle maximum is shown in fig 3

Applying equal area criterion 

0crCPm-PmaxIIISinδⅆδ=crmaxPmaxIIISinδ-Pmⅆδ

Max=π-Sin-1PmPmaxIII

Integrating-> 

Pmδ+PmaxIICosδ|cr0+PmaxIIICosδ+Pm|maxcr=0

Or Pmcr-0+PmaxiCosδcr-Cosδ0+Pmmax-cr+PmaxIIICosδmax-Cosδcr=0

Or

Cosδcr=Pmmax-0-PmaxIICosδ0+PmaxIIICosδmaxPmaxIII-PmaxIIi

Critical clearing angle can be calculated from above equation for Case-b

Case-C Reclosure

[Refer Fig a in case -a]

If the circuit breaker on line -2 (on which fault has occurred ) is closed after clearing a transient fault. The power transfer becomes –

Pe±V=PeI=Pmax1Sinδ

As the chances of stable operation improve, shown in figure below.

For critical clearing Angle

1=max=π-sin-1PmPmaxI

0crPm-PmaxIISinδⅆδ=crδrcPmaxIIISinδ-Pmⅆδ+rcmaxcPmaxISinδ-Pmⅆδ

Where trc=tcr+τ;{τ=Time between clearing and reclosure)

12. Explain the numerical solution to swing equation?

Point-by-Point method for one machine tied to infinite bus-bar Consider a swing equation.

ⅆ2ⅆt2=1MPm-PmaxSinδ=PaM

M=GH or in pu system M=Hπf)

The solution δ(t) is obtained at descrete intervals of time with interval spread of Δt uniform throughout.

1. Accelerating power Pa computed at the beginning of interval is assumed to remain constant from the middle of the next interval to middle of interval being considered.
2. The rotor velocity ω=ⅆδⅆt Assumed constant throughout any interval,

Fig: Point by point solution of swing equation 

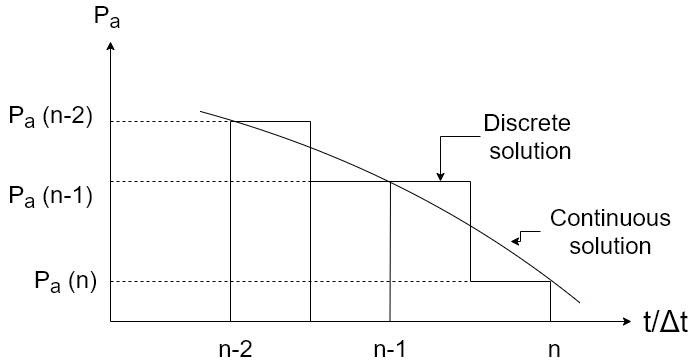
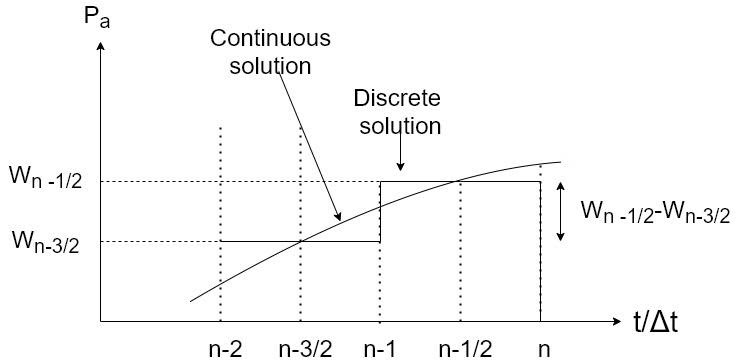
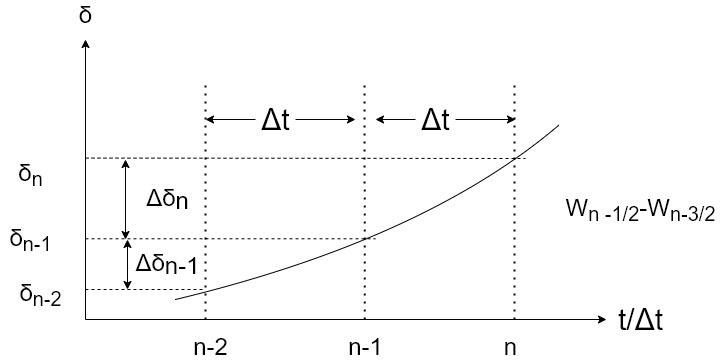
In figure The numbering on tΔt axis belong to the end of intervals at the end of (n-1)th interval 

• The accelerating power is 

Pan-1=Pm-PmaxSinδ-1---I

Where n-1 is previously calculated 

• Change in velocity from

(n-32)to(n-12) is 

Wn-12-Wn-32=ΔtMPan-1---ii

• Change in  during (n-1)th interval is

Δδn-1=n-1-n-2=Δtn-32----III

During the nth interval

Δδn=n-n-1=Δtn-12-----IV

Subtracting equation IV from III and using equation II we get

Δδn=Δδn-1+Δt2MPan-1-----V

We can now write

n=n-1+Δδn------VI

The process is repeated to obtain Pa(n),Δδn+1. Normally time solution length is 0.5 seconds for discrete form can be reduced to get smooth curve. Continuous solution is obtained by drawing a smooth curve as shown in figure.

The occurrence or removal of fault or initiation of any switching event causes discontinuity in accelerating power Pa. In such case then the average values of Pa before and after the discontinuity must be used.

Thus increment of angle occurrence during first interval after a fault is applied at t=0 is given by:-

Δδ1=Δt2M+Pa02

Where Pa0+=Accelerating power immediately after occurrence of fault.

Pa0-=Before the fault

13. Explain the methods to improve steady state stability?

Maximum power can be transferred to load/sending end given by-

Pmax=EVX

We can improve steady state stability by

• Increasing inertia constant M of machine (But not employed due to economical reasons in practically and slowing down the response of speed governor loop.)
• The maximum steady state power of system can be increased by rising the voltage profile of system.
• Reducing Transfer reactance

Methods of improving Transient stability limit of system

1. Increase of system voltages by use of AVR (Automatic voltage Regulator):

When fault occurs, voltages at all buses are reduced. At generator terminal this is sensed by AVR, which acts to restore generator terminal voltage.

2.                  Use of High-speed Excitation systems: -

With the help of AVR within excitation system, modern exciter systems having solid state control quickly respond to bus voltage reduction within ½ to 1 and ½ (0.5 to 1.5) cycles.

3.                  Reducing Transfer reactance:

Reactance of a transmission line can be decreased by

I Reducing the conductor spacing 

II Increasing conductor diameter

4.                  By using compensation for reactance of line by series compensation:

For more than 350Km length of line series capacitors can be used.

Switched Series Capacitors

Simultaneously decrease fluctuation of load voltages and raise transient stability limit.

Switching shunt capacitors on or switching shunt reactors off also raises system stability.

5.                  Use of high-speed reclosing 

Breakers:

As most of the faults are transient in nature isolation of unhealthy lines rapidly improves system stability.

Single pole reclosing also improves the system stability to good extent.

14.A 50 Hz, four pole turbo generator rated 100 MVA, 11 kv has an inertia Constant of 8.0 MJ/ MVA. 

1. Find the stored energy in the rotor at synchronous speed.
2. If the mechanical input is suddenly raised to 80 MW for an electrical load of 50 MW, find rotor acceleration, neglecting mechanical and electrical losses.
3. If the acceleration calculated in part (b) is maintained for 10 cycles, find the change in torque angle and rotor speed in revolutions per minute at the end of this period.

Solution: 

1. Stored energy =GH=100*8=800MJ
2. Pa=80-50=30MW=Mⅆ2ⅆt2

M=GH180f=800180×50=445MJ-selectdeg

445ⅆ2ⅆt2=30

Or 

α=ⅆ2ⅆt2=337.5electdegs2

© 10 cycles=0.2s

Change in δ=12337.50.22=6.75electdegrees

60×337.52×360°=28.125rpms

Rotor speed at the end of 10 cycles

=120×504+28.125×0.2

=1505.625rpm

Example 1.

The system shown in figure, 3-phase static capacitive reactor of reactance 1pu per phase is connected through a switch at the motor busbar. Calculate the limit of steady state power with and without reactors switch closed. Re calculate the power limit with capacitive reactance placed by an inductive reactor of the same value. 

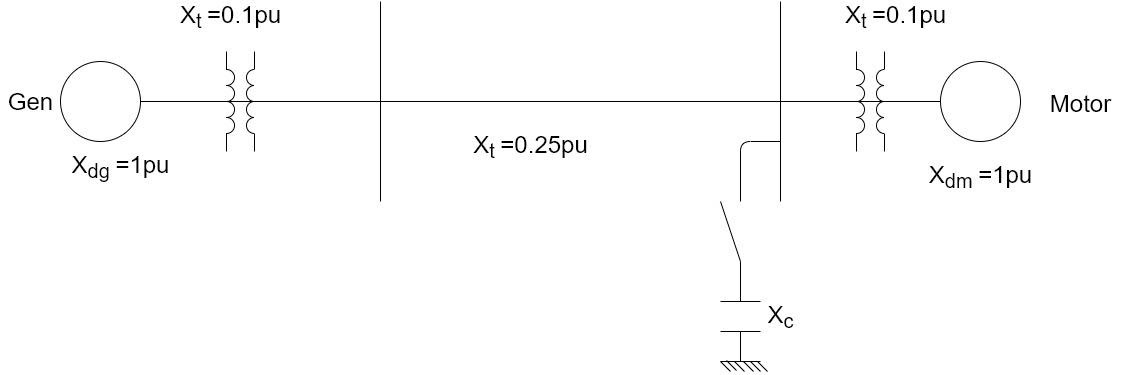
Assume the internal voltage of generator to be 1.2 pu and that of the motor to be 1.0 pu.

1. Steady state power limit without reactor

=(|E||Em|)X(total)=1.2×11+0.1+0.25+0.1+1=0.49pu

2.                  Equivalent circuit with capacitive reactor is shown in Fig 12.11(a)

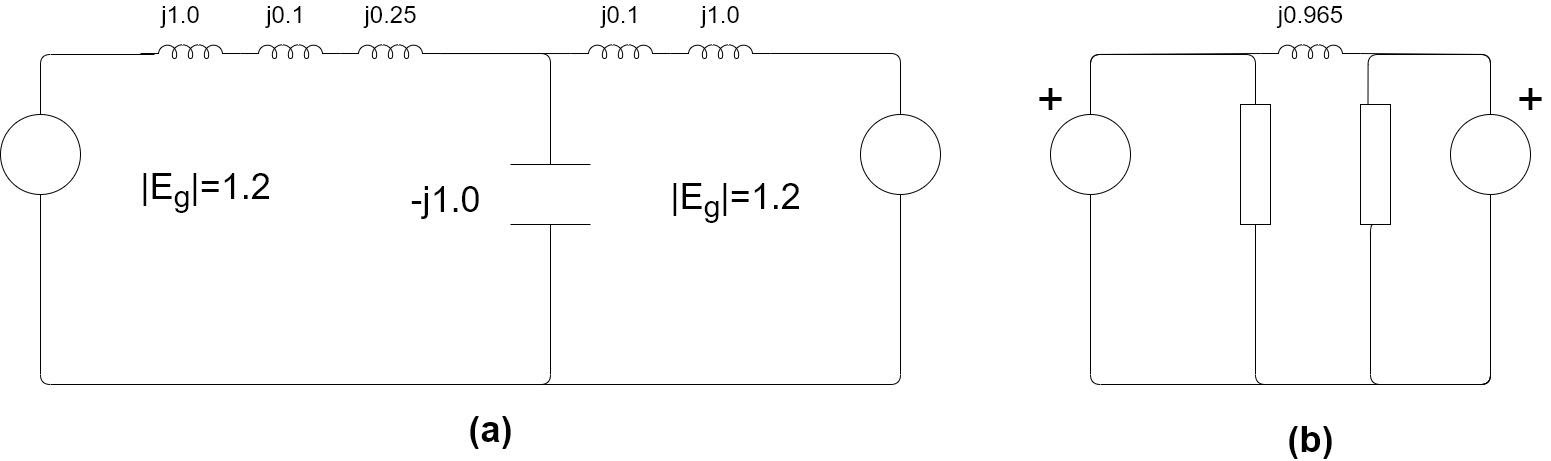
Converting Star to Delta the network of figure (a) in is to that of figure (b) where

JXtransfer=j1.35j1.1+j1.1-j1.0+-j1.0j1.35-j1.0

=j0.965

Steady state power limit 1.2×10.965=1.244pu

3.                  With capacitive reactance replaced by inductive reactance, we get the equivalent circuit of Fig 12.12 Converting star to delta, we have the transfer reactance of

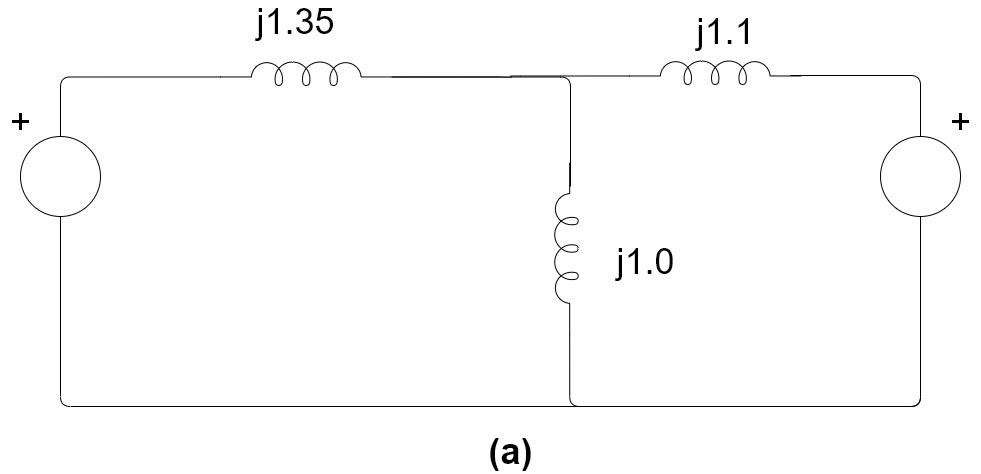
JXtransfer=j1.35j1.1+j1.1j1.0+j1.0j1.35j1.0

=j3.935

Steady state power limit=12×13.935=0.304pu

15.For figure shown  below where is ford is applied at point P  as shown.

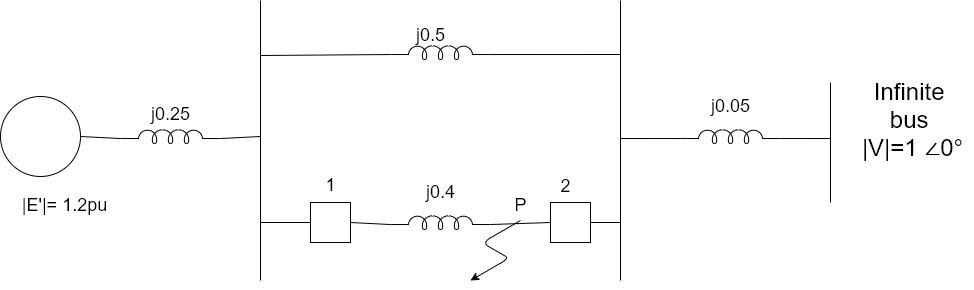
Find the critical clearing angle for clearing the fault with simultaneous opening of circuit breaker 1 and 2. The reactance values of various components are indicated on the diagram. The generator is delivering 1.0 power at instant preceding the fault.

Solution 

With reference to Fig 12.31 three separate power angle curves are involved.

I Normal operation(prefault)

X1=0.25+0.5×0.40.5+0.4+0.05

=0.522pu

Pe1=E'VX1sinδ=1.2×10.522sinδ

=2.3sinδ

Prefault operating power angle is given by

1.=2.3sinδ0

Or                                           0=25.8°=0.45radians

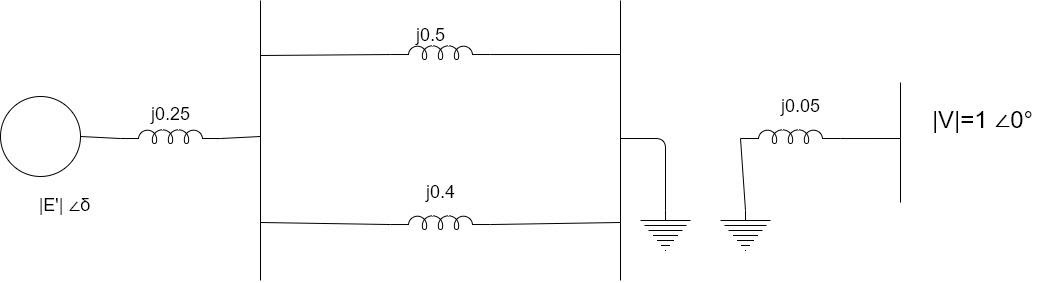
III Post fault operation (fault cleared by opening the faulted line)

X111=0.25+0.5+0.05=0.8

PeIII=1.2×1.0.8sinδ=1.5sinδ

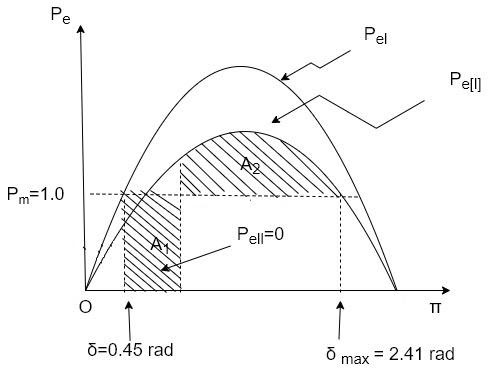
The maximum permissible angle max for area A1=A2 see Figure given by

Max=π-sin-111.5=2.41radians

Applying equal area criterion for critical clearing angle c

A1=Pmcr-0

=1.(cr-0.45)=cr-0.45

A2=crmaxPeIII-Pmⅆδ

=cr2.41(1.5sinδ-1)ⅆδ

=-1.5cosδ-δ|2.41cr

=-1.5(Cos2.41-cosδcr)-(2.41-cr)

=1.5cosδcr+cr-1.293

Setting A1=A2 and solving

Cr-0.45=1.5cosδcr+cr-1.293

Cosδcr=0.8431.5=0.562

Cr=55.8°