EEE
UNIT 4Three Phase Induction MotorsQ1) Working principle of three phase induction motorA1) IM works on principle of electromagnetic induction. When 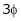 supply is given to
supply is given to 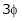 stator winding, a rotating magnetic field of constant magnitude is produced, the speed of this rotating magnetic field is synchronous speed (NS) in rpm.
stator winding, a rotating magnetic field of constant magnitude is produced, the speed of this rotating magnetic field is synchronous speed (NS) in rpm. 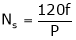 = Speed of rotating magnetic field.C. This rotating magnetic field produces on effect of rotating poles around a rotor.D. Let direction of rotation of this rotating magnetic field is clockwise. (Fig a)
= Speed of rotating magnetic field.C. This rotating magnetic field produces on effect of rotating poles around a rotor.D. Let direction of rotation of this rotating magnetic field is clockwise. (Fig a)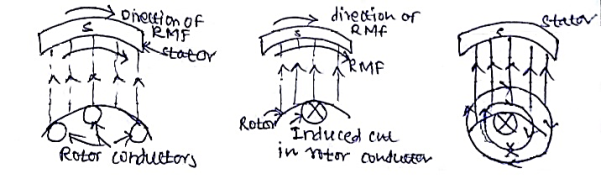 Now at this instant rotor is stationery and stator flux RMF is rotating. So, it’s obvious that there exists a relative motion between RMF and motor conductors.E. Whenever conductor cuts the flux, emf gets induced in it.F. As rotor forms closed circuit, induced emf circulates current though rotor called rotor current fig.(b).G. Any current carrying conductor produces its own flux, called rotor flux fig. (c).
Now at this instant rotor is stationery and stator flux RMF is rotating. So, it’s obvious that there exists a relative motion between RMF and motor conductors.E. Whenever conductor cuts the flux, emf gets induced in it.F. As rotor forms closed circuit, induced emf circulates current though rotor called rotor current fig.(b).G. Any current carrying conductor produces its own flux, called rotor flux fig. (c).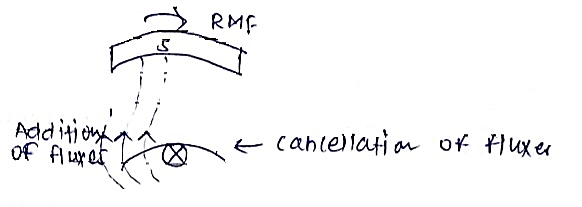 H. Both fluxes interact each other as shown in fig (d)I. On left of rotor conductor, two fluxes are in same direction hence add each other. While on ri9ght side of rotor, fluxes cancel each other to produce low flux area.J. High flux density area exerts a push on rotor conductor towards low flux density area, so rotor conductor experiences forces from left to right fig. (d)K. As all rotor conductors experience a force, the overall rotor experiences a torque and start rotation so interaction of fluxes is very essential for a motion action.L. The cause of rotor current is the induced E.M.F which is induced because of relative motion present between rotating magnetic field and rotor conductors.M. To oppose relative motion i.e. to reduce relative speed, rotor experiences a torque in same direction as that of RMF and tries to catch up the speed of rotating magnetic field.N. NS = Speed of rotating magnetic field.N = Speed of rotor i.e. motor speed in rpm (NS – N) = Relative speed between two, rotating magnetic field and rotor.O. Thus, rotor always rotates in same direction as that of RMF. (N < NS).Slip of Induction Motor
H. Both fluxes interact each other as shown in fig (d)I. On left of rotor conductor, two fluxes are in same direction hence add each other. While on ri9ght side of rotor, fluxes cancel each other to produce low flux area.J. High flux density area exerts a push on rotor conductor towards low flux density area, so rotor conductor experiences forces from left to right fig. (d)K. As all rotor conductors experience a force, the overall rotor experiences a torque and start rotation so interaction of fluxes is very essential for a motion action.L. The cause of rotor current is the induced E.M.F which is induced because of relative motion present between rotating magnetic field and rotor conductors.M. To oppose relative motion i.e. to reduce relative speed, rotor experiences a torque in same direction as that of RMF and tries to catch up the speed of rotating magnetic field.N. NS = Speed of rotating magnetic field.N = Speed of rotor i.e. motor speed in rpm (NS – N) = Relative speed between two, rotating magnetic field and rotor.O. Thus, rotor always rotates in same direction as that of RMF. (N < NS).Slip of Induction Motor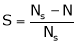
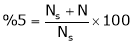 N = NS()Can NS = NAlso, Never NS> N
N = NS()Can NS = NAlso, Never NS> NEffect of slip on Rotor Parameters The slip affects the rotor parameters when an I.M runs with speed N rpm.The various parameter which gets affected by the slip are-F Induced emf Current p.f Impedance 1) Effect on Rotor FrequencyAt start speed of I.M. is zero (N = 0) & slip s = l.Then the frequency of rotor induced emf is same as the stator supply frequency.IfFr = Frequency of rotor induced emff = Stator supply frequencyFr = F at start when S = l.But when motor rotates at speed N, there exists a slip S & then in running condition, frequency of rotor induced emf is slip times the stator supply frequency.Fr = Sf … in running conditionAs slip is small, Fr is very small in2) Effect on magnetic of rotor induced emfLetE2 = Rotor induced emf/ Ph in stand stillWhileE2r = Rotor induced emf/ Ph in runningThe induced emf depends on relative motion between rotor &RmF.It is max. at start hence E2 is max at start.In running condition, relative speed becomes Ns – N hence induced emf reduce, by slip times. The max. emf E2 at stand still.E2r = SE23) Effect on ImpedanceThe rotor imp. Has two comp. resistance and inductive reactance. The XL depends on the frequency. Let,R2 = Rotor resistance/ ph. On standstill condition.X2 = ReactanceZ2 = R2 + jx2 = Rotor imp. On stand.(N = 0)In running condition, rotor frequency. (Fr = SF)Hence,  Hence rotor imp. In running condition (Z2r) isZ2r = R2 + jx2r = R2 + js x 24) Effect on Rotor P.F.The P.F. is the ratio of R toZ
Hence rotor imp. In running condition (Z2r) isZ2r = R2 + jx2r = R2 + js x 24) Effect on Rotor P.F.The P.F. is the ratio of R toZ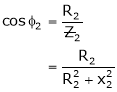 While in running condition,
While in running condition,  changes to
changes to 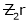 . Hence P.F. in running condition is given by
. Hence P.F. in running condition is given by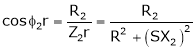 5) Effect on rotor currentThe ratio of rotor induced emf to rotor imp. Is the rotor current.Hence rotor current on stand still is
5) Effect on rotor currentThe ratio of rotor induced emf to rotor imp. Is the rotor current.Hence rotor current on stand still is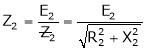 While in running condition, E2 changes to SE2 and
While in running condition, E2 changes to SE2 and 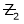 changes to Z2r hence rotor current becomes.
changes to Z2r hence rotor current becomes.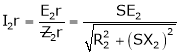 Q2) Explain Torque equationA2) The torque produced in I.m depend on following factors1) The port of RMF which react with rotor & is responsible to produce
Q2) Explain Torque equationA2) The torque produced in I.m depend on following factors1) The port of RMF which react with rotor & is responsible to produce .2) The magnetic of rotor current in running condition.3) The P.F. of rotor ckt in running condition.Mathematically, the relationship can expressed as
.2) The magnetic of rotor current in running condition.3) The P.F. of rotor ckt in running condition.Mathematically, the relationship can expressed as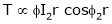 … (1)
… (1) = Flux responsible to produce induced emfI2r = Rotor running current.
= Flux responsible to produce induced emfI2r = Rotor running current.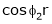 = Running P.F. of rotorThe flux
= Running P.F. of rotorThe flux  produced by stator is proportional to E1 i.e. stator voltage.
produced by stator is proportional to E1 i.e. stator voltage.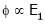 … (2)While E1 and E2 are related to each other through ratio of stator runs to rotor turns i.e.
… (2)While E1 and E2 are related to each other through ratio of stator runs to rotor turns i.e.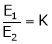 … (3)Using equation (3) in (2) we can write
… (3)Using equation (3) in (2) we can write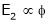 Thus, in equation (1)
Thus, in equation (1)  can replace by E2Also
can replace by E2Also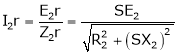
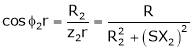
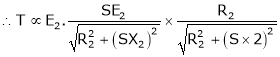
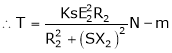 Where K = constant of proportionality.The constant K is proved to be
Where K = constant of proportionality.The constant K is proved to be 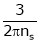 for
for 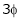 I.M.
I.M. Where,ns = syn. Speed in rps =
Where,ns = syn. Speed in rps = 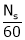 where NS in rpm
where NS in rpm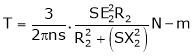 Case IStarting TorqueA) Starting torque is nothing but the torque produced by an I.m at startAt start, N = 0 & slip S = 1
Case IStarting TorqueA) Starting torque is nothing but the torque produced by an I.m at startAt start, N = 0 & slip S = 1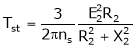 From the above equation it is clear that by changing R2, the starting torque Tst can be controlled.B) The change in R2 at start is possible in case of slip ring I.m. only.This is the principle used in case of slip ring I.m. to control starting torque Tst.Case IICondition for max. Torque (Tm)A) The only parameter which controls torque is slip S other parameters E2, R2, X2& ns are constant.B) Mathematically for the max. torque e can write,
From the above equation it is clear that by changing R2, the starting torque Tst can be controlled.B) The change in R2 at start is possible in case of slip ring I.m. only.This is the principle used in case of slip ring I.m. to control starting torque Tst.Case IICondition for max. Torque (Tm)A) The only parameter which controls torque is slip S other parameters E2, R2, X2& ns are constant.B) Mathematically for the max. torque e can write,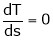 where,
where,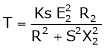
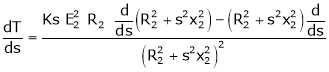
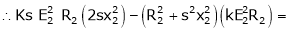

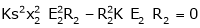
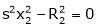 Taking
Taking 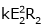 con.
con.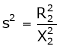
 This is the slip at which torque is max. and is denoted as Sm
This is the slip at which torque is max. and is denoted as Sm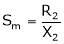 It is the ratio of standstill rotor resistance and reactance.Case IIIMagnitude of Max. TorqueThis can be obtained by putting
It is the ratio of standstill rotor resistance and reactance.Case IIIMagnitude of Max. TorqueThis can be obtained by putting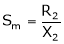 in the torque equation.
in the torque equation.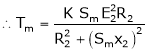
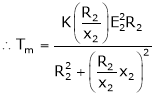
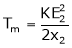 N.m.It is observed that Tm is -
N.m.It is observed that Tm is - Inversely proportional to rotor reactance Directly proportional to the sq. of rotor induced emf. At standstill. Not dependent on the rotor risis R2But the slip at which it occurs i.e. speedat which it occurs depends on the valves of rotor resistance R2. Q3) Explain Torque slip characteristicA3)1) As the I.M. is loaded from no load to fall load it’s speed decreases hence slip increases.2) Due to increased load, motor has to produce more torque to satisfy load.3) The torque ultimately depends on slip.4) The behaviour of motor can be easily judged by sketching a curve obtained by plotting torque produced against slip of I.M.5) The curve obtain by plotting torque against slip from S = 1 (at start) to S = 0 (at syn. Speed) is called torque slip chs. of the I.M.We have seen that for a constant supply voltage, E2 is also constant.We can write torque equation as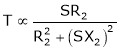 6) To judge the nature of torque – slip ch. Let us divide the slip range S = 0 to S = 1 into two parts and analyse them independently.Low Slip RegionIn this region, S is very small.Due to this the terms (SX2)2 is so small as compared to
6) To judge the nature of torque – slip ch. Let us divide the slip range S = 0 to S = 1 into two parts and analyse them independently.Low Slip RegionIn this region, S is very small.Due to this the terms (SX2)2 is so small as compared to 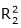 that it can be neglected.
that it can be neglected.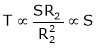 Hence in low slip region torque is directly proportional to slip. So as load increases, speed decreases, increasing the slip.This increases the torque which satisfied load demand.Hence the graph is st. line in natue.At N = Ns, S = 0, hence T = 0As no torque is generated at N = Ns, motor stops. If it trial to achieve the syn. Speed.Torque increases linearly in this Regin, of low slip values.High slip RegionIn this region, slip is high i.e. slip value is approaching to 1, Here it can be assumed that the term
Hence in low slip region torque is directly proportional to slip. So as load increases, speed decreases, increasing the slip.This increases the torque which satisfied load demand.Hence the graph is st. line in natue.At N = Ns, S = 0, hence T = 0As no torque is generated at N = Ns, motor stops. If it trial to achieve the syn. Speed.Torque increases linearly in this Regin, of low slip values.High slip RegionIn this region, slip is high i.e. slip value is approaching to 1, Here it can be assumed that the term 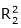 is very small as compared to
is very small as compared to 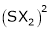 Hence neglecting
Hence neglecting  from the denominator
from the denominator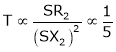 T where R2& X2 are constants.So in high slip region torque is inversely proportional to the slip. Hence its nature is like rectangular hyperbola.
T where R2& X2 are constants.So in high slip region torque is inversely proportional to the slip. Hence its nature is like rectangular hyperbola. 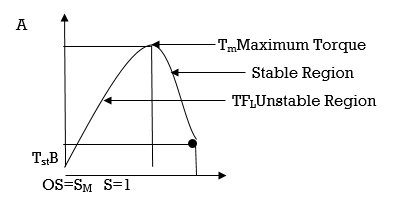 OA = Stable RegionAB = Unstable RegionA = Maximum RegionB = Starting TorqueC = Full and Torque Q4) What is the effect of change in Resistance on TorqueA4) We know that in slip ring I.M. externally resistance can be added in the rotor.Let, R2 = rotor resistance per ph.
OA = Stable RegionAB = Unstable RegionA = Maximum RegionB = Starting TorqueC = Full and Torque Q4) What is the effect of change in Resistance on TorqueA4) We know that in slip ring I.M. externally resistance can be added in the rotor.Let, R2 = rotor resistance per ph. Now externally resistance is added in each phase of rotor thro slip rings.Let
Now externally resistance is added in each phase of rotor thro slip rings.Let 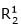 = Now rotor resistance per phase.
= Now rotor resistance per phase. Similarly starting torque at s = 1For
Similarly starting torque at s = 1For 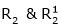 can be written as
can be written as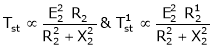 Maximum Torque
Maximum Torque 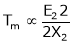 TM Max. Torque Line
TM Max. Torque Line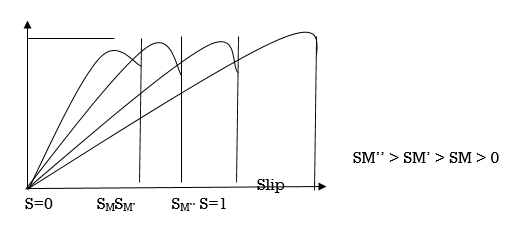 Q5) What is the effect of rotor resistance on characteristicsA5) In slip ring induction motor, externally resistance can be added in the rotor.To see the effect of change in rotor resistance on the torque produced.The torque produced by an induction motor is given byT = KT s α / (s2 + α2)where KT is torque constant = 3 (E1)2 / ωs X’2And α is the machine constant = R’2 / X’2Now when an external resistance is added up in series with rotor then
Q5) What is the effect of rotor resistance on characteristicsA5) In slip ring induction motor, externally resistance can be added in the rotor.To see the effect of change in rotor resistance on the torque produced.The torque produced by an induction motor is given byT = KT s α / (s2 + α2)where KT is torque constant = 3 (E1)2 / ωs X’2And α is the machine constant = R’2 / X’2Now when an external resistance is added up in series with rotor thenValue of Slip corresponding to maximum torque gets changed as R’2 changed. Maximum torque remains unaltered. Let ‘x’ represent per phase value of inserted resistance in series with rotor then the machine constant α gets changed or increased to a new value.Hence value of slip corresponding to maximum torque smax is equal to α gets shifted.Therefore, smax = ( R’2 + x ) / X’2Tm = KT / 2 (When s = α) = 1.5 (E1)2 / ωs X’2It is observed that Tm is independent of R’2 hence whatever may be the rotor resistance, maximum torque produced never changes but the slip and speed at which it occurs depends on R’2. Because of this we get a new torque – slip characteristics for rotor resistance ( R’2 + x ). This new characteristics is parallel to the characteristics for with same Tm but occurring at different value of slip. 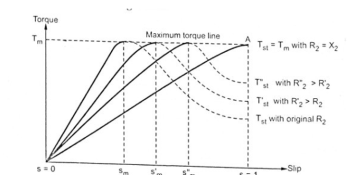 Effect of rotor resistance on torque-slip curvesFrom the figure it can be seen that the starting torque T’st for R’2 is more than Tst for ( R’2 + x ) . Thus by changing rotor resistance the starting torque can be controlled.
Effect of rotor resistance on torque-slip curvesFrom the figure it can be seen that the starting torque T’st for R’2 is more than Tst for ( R’2 + x ) . Thus by changing rotor resistance the starting torque can be controlled.
 Now at this instant rotor is stationery and stator flux RMF is rotating. So, it’s obvious that there exists a relative motion between RMF and motor conductors.E. Whenever conductor cuts the flux, emf gets induced in it.F. As rotor forms closed circuit, induced emf circulates current though rotor called rotor current fig.(b).G. Any current carrying conductor produces its own flux, called rotor flux fig. (c).
Now at this instant rotor is stationery and stator flux RMF is rotating. So, it’s obvious that there exists a relative motion between RMF and motor conductors.E. Whenever conductor cuts the flux, emf gets induced in it.F. As rotor forms closed circuit, induced emf circulates current though rotor called rotor current fig.(b).G. Any current carrying conductor produces its own flux, called rotor flux fig. (c). H. Both fluxes interact each other as shown in fig (d)I. On left of rotor conductor, two fluxes are in same direction hence add each other. While on ri9ght side of rotor, fluxes cancel each other to produce low flux area.J. High flux density area exerts a push on rotor conductor towards low flux density area, so rotor conductor experiences forces from left to right fig. (d)K. As all rotor conductors experience a force, the overall rotor experiences a torque and start rotation so interaction of fluxes is very essential for a motion action.L. The cause of rotor current is the induced E.M.F which is induced because of relative motion present between rotating magnetic field and rotor conductors.M. To oppose relative motion i.e. to reduce relative speed, rotor experiences a torque in same direction as that of RMF and tries to catch up the speed of rotating magnetic field.N. NS = Speed of rotating magnetic field.N = Speed of rotor i.e. motor speed in rpm (NS – N) = Relative speed between two, rotating magnetic field and rotor.O. Thus, rotor always rotates in same direction as that of RMF. (N < NS).Slip of Induction Motor
H. Both fluxes interact each other as shown in fig (d)I. On left of rotor conductor, two fluxes are in same direction hence add each other. While on ri9ght side of rotor, fluxes cancel each other to produce low flux area.J. High flux density area exerts a push on rotor conductor towards low flux density area, so rotor conductor experiences forces from left to right fig. (d)K. As all rotor conductors experience a force, the overall rotor experiences a torque and start rotation so interaction of fluxes is very essential for a motion action.L. The cause of rotor current is the induced E.M.F which is induced because of relative motion present between rotating magnetic field and rotor conductors.M. To oppose relative motion i.e. to reduce relative speed, rotor experiences a torque in same direction as that of RMF and tries to catch up the speed of rotating magnetic field.N. NS = Speed of rotating magnetic field.N = Speed of rotor i.e. motor speed in rpm (NS – N) = Relative speed between two, rotating magnetic field and rotor.O. Thus, rotor always rotates in same direction as that of RMF. (N < NS).Slip of Induction Motor Hence rotor imp. In running condition (Z2r) isZ2r = R2 + jx2r = R2 + js x 24) Effect on Rotor P.F.The P.F. is the ratio of R toZ
Hence rotor imp. In running condition (Z2r) isZ2r = R2 + jx2r = R2 + js x 24) Effect on Rotor P.F.The P.F. is the ratio of R toZ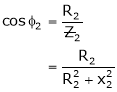 While in running condition,
While in running condition, 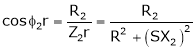 5) Effect on rotor currentThe ratio of rotor induced emf to rotor imp. Is the rotor current.Hence rotor current on stand still is
5) Effect on rotor currentThe ratio of rotor induced emf to rotor imp. Is the rotor current.Hence rotor current on stand still is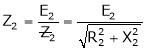 While in running condition, E2 changes to SE2 and
While in running condition, E2 changes to SE2 and 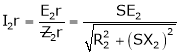 Q2) Explain Torque equationA2) The torque produced in I.m depend on following factors1) The port of RMF which react with rotor & is responsible to produce
Q2) Explain Torque equationA2) The torque produced in I.m depend on following factors1) The port of RMF which react with rotor & is responsible to produce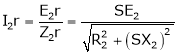
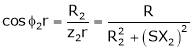
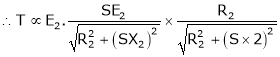
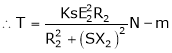 Where K = constant of proportionality.The constant K is proved to be
Where K = constant of proportionality.The constant K is proved to be 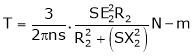 Case IStarting TorqueA) Starting torque is nothing but the torque produced by an I.m at startAt start, N = 0 & slip S = 1
Case IStarting TorqueA) Starting torque is nothing but the torque produced by an I.m at startAt start, N = 0 & slip S = 1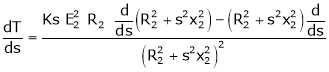
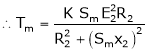
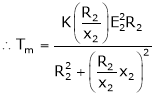
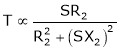 6) To judge the nature of torque – slip ch. Let us divide the slip range S = 0 to S = 1 into two parts and analyse them independently.Low Slip RegionIn this region, S is very small.Due to this the terms (SX2)2 is so small as compared to
6) To judge the nature of torque – slip ch. Let us divide the slip range S = 0 to S = 1 into two parts and analyse them independently.Low Slip RegionIn this region, S is very small.Due to this the terms (SX2)2 is so small as compared to 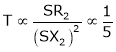 T where R2& X2 are constants.So in high slip region torque is inversely proportional to the slip. Hence its nature is like rectangular hyperbola.
T where R2& X2 are constants.So in high slip region torque is inversely proportional to the slip. Hence its nature is like rectangular hyperbola.  OA = Stable RegionAB = Unstable RegionA = Maximum RegionB = Starting TorqueC = Full and Torque Q4) What is the effect of change in Resistance on TorqueA4) We know that in slip ring I.M. externally resistance can be added in the rotor.Let, R2 = rotor resistance per ph.
OA = Stable RegionAB = Unstable RegionA = Maximum RegionB = Starting TorqueC = Full and Torque Q4) What is the effect of change in Resistance on TorqueA4) We know that in slip ring I.M. externally resistance can be added in the rotor.Let, R2 = rotor resistance per ph. Now externally resistance is added in each phase of rotor thro slip rings.Let
Now externally resistance is added in each phase of rotor thro slip rings.Let  Similarly starting torque at s = 1For
Similarly starting torque at s = 1For  Q5) What is the effect of rotor resistance on characteristicsA5) In slip ring induction motor, externally resistance can be added in the rotor.To see the effect of change in rotor resistance on the torque produced.The torque produced by an induction motor is given byT = KT s α / (s2 + α2)where KT is torque constant = 3 (E1)2 / ωs X’2And α is the machine constant = R’2 / X’2Now when an external resistance is added up in series with rotor then
Q5) What is the effect of rotor resistance on characteristicsA5) In slip ring induction motor, externally resistance can be added in the rotor.To see the effect of change in rotor resistance on the torque produced.The torque produced by an induction motor is given byT = KT s α / (s2 + α2)where KT is torque constant = 3 (E1)2 / ωs X’2And α is the machine constant = R’2 / X’2Now when an external resistance is added up in series with rotor then Effect of rotor resistance on torque-slip curvesFrom the figure it can be seen that the starting torque T’st for R’2 is more than Tst for ( R’2 + x ) . Thus by changing rotor resistance the starting torque can be controlled.
Effect of rotor resistance on torque-slip curvesFrom the figure it can be seen that the starting torque T’st for R’2 is more than Tst for ( R’2 + x ) . Thus by changing rotor resistance the starting torque can be controlled. 0 matching results found