Unit – 6
Compressor
Q1) A two stage air compressor with perfect intercooling takes in air at 1 bar & 270c. The law of compression in both stages is pv1.3 = c. The compressed air is delivered at 9 bar from HP cylinder to an air receiver. Calculate per kg of air.
a). Minimum work of compression
b). Heat rejected in intercooler
c). Work required for single stage compression to the same delivery pressure.
A1)
Given:
P1 = 1 x 105 N/m2
T3 = T1 = 270c = 27 +-273 = 300 K
n = 1.3
P3 = 9 x 105 N/m2
Minimum Work = 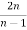 mRT1 [ (
mRT1 [ (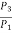 )(n-1)/2n - 1 ]
)(n-1)/2n - 1 ]
required to compress
= 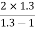 x1 x 287 x 300 [ (
x1 x 287 x 300 [ ( )(1.3 -1)/(2 x 1.3) - 1]
)(1.3 -1)/(2 x 1.3) - 1]
= 215.32 x 103N.m or Joule
For Perfect intercooling
P2= 
= 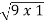
= 3 bar = 3 x 105 N/m2
(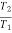 ) = (
) = ( )(n -1)/n
)(n -1)/n
(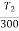 ) = (
) = ( )(1.3 -1)/1.3
)(1.3 -1)/1.3
T2 = 386.57 K
Heat rejected = mCp(T2 – T3)
in intercooler
= 1 x 1.005 x (386.57 - 300)
= 87 KJ/kg
Work required for = 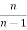 mRT1[ (
mRT1[ (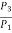 )(n-1)/n - 1 ]
)(n-1)/n - 1 ]
Single stage compression
=  x1 x 287 x 300 [ (
x1 x 287 x 300 [ ( )(1.3 -1)/( 1.3) - 1]
)(1.3 -1)/( 1.3) - 1]
= 246.39 x 103N.m
Q2) A two stage single reciprocating air compressor takes in air 1 bar & 200c. it discharges air at 40 bar. The temperature rise in intercooler is 220c. Assume polytrophic compression & expansion with n = 1.33, perfect intercooling with ideal intermediate pressure. Calculate work of compression, % saving in work of compression as compared to single stage. C). Mass of cooling water required in intercooler per kg of air. Assume Cp = 1.005 & 4.187 kj/kg k for air & water respectively.
A2)
Given
P1 = 1 bar = 1 x 105 N/m2
T3 = T1 = 200c = 20 + 273 = 293 K
P3 = 40 bar = 40 x 105 N/m2
Δtw = 220c
n = 1.33
Work required = 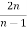 mRT1 [ (
mRT1 [ ( )(n-1)/2n - 1 ]
)(n-1)/2n - 1 ]
for compression
=  x1 x 287 x 293 [ (
x1 x 287 x 293 [ (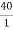 )(1.33 -1)/(2 x 1.33) - 1]
)(1.33 -1)/(2 x 1.33) - 1]
W1= 393.37 x 103N.m or Joule
Work required for = 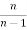 mRT1 [ (
mRT1 [ (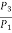 )(n-1)/n - 1 ]
)(n-1)/n - 1 ]
single stage compression
=  x1 x 287 x 293 [ (
x1 x 287 x 293 [ (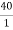 )(1.33 -1)/( 1.33) - 1]
)(1.33 -1)/( 1.33) - 1]
W2= 507.51 x 103 Joule
% Saving in work = (W2 – W1)/W2 x 100
= (507.51 – 393.37)/507.51 x 100
= 22.49 %
P2 = 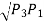
= 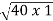
= 6.32 bar
(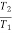 ) = (
) = ( )(n -1)/n
)(n -1)/n
( ) = (
) = (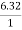 )(1.33 -1)/1.33
)(1.33 -1)/1.33
T2 = 462.960c
Heat rejected = Heat gain
in intercooler by water
mCp(T2 – T3) = mwCpwΔtw
1 x 1.005 x (462.96 - 293) = mw x 4.18 x 22
mw = 1.85 kg
Q3) A single cylinder double acting air compressor sucks air at the rate of 5 m3/min at a pressure of 100 kPa & 250c. it delivers air to the receiver at a pressure 6 bar. The speed of the compressor is 250 rpm & stroke is 1.5 times cylinder diameter. Neglect the effect of clearance & if law of compression is PV1.25 = C.
Find cylinder dimensions, indicated power, shaft power in the mechanical is 90%.
A3)
Given:
V1 = 5 m3/min = 5/60 = 0.0833 m3/sec
P1 = 100 x 103 N/m2
T1 = 250c = 25 + 273 = 298 k
P2 = 6 bar = 6 x 105 N/m2
N = 250 m
L = 1.5 D
n = 1.25
Indicated power = 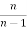 P1V1 [ (
P1V1 [ (  )n-1/n - 1 ]
)n-1/n - 1 ]
=  x 100 x 103 x 0.0833 [ (
x 100 x 103 x 0.0833 [ (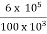 )(1.25-1)/( 1.25) - 1]
)(1.25-1)/( 1.25) - 1]
= 17.95 x 103 watts
ɳmech= 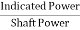 x 100
x 100
90 = 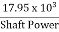 x 100
x 100
Shaft Power = 19.94 x 103 watts
V1 = Vs = π/4 x D2 x L
0.0833 = π/4 x D2 x 1.5 D
D = 0.41 m
L = 1.5 D = 0.619 m
Q4) A single stage reciprocating compressor has a swept volume 2000 cm3, runs at 800 rpm & takes in air 1.013 bar & 150c. It operates on a pressure ratio of 8, with a clearance of 5% of the swept volume. Assume polytrophic compression & expansion with n = 1.25. Calculate volumetric efficiency, indicated power, isothermal efficiency, actual power needed to drive the compressor, if mechanical efficiency is 85%.
A4)
Given:
Vs = 2000 cm3 = 2 x 10-3 m3
N = 800 rpm
P1 = 1.013 bar = 1.013 x 105 N/m2
T1 = 150c = 15 + 273 = 288 k
P3/P4 = P2/P1 – 8
Vc = 5/100 Vs = 5/100 x 2 x 10-3
= 1 x 10-4 m3
ɳ = 1.25
ɳmech = 85%
V1 = Vs + Vc = 2.1 x 10-3 m3
V3 = Vc = 1 x 10-4
(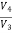 ) = (
) = (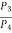 )1/n
)1/n
( ) = (8)1/1.25
) = (8)1/1.25
V4 = 5.27 x 10-4 m3
Work done = 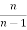 P1(V1 – V4) [ (
P1(V1 – V4) [ (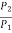 )n-1/n - 1 ]
)n-1/n - 1 ]
W = 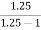 x 1.013 x 105 x (2.1 x 10-3- 5.27 x 10-4)[ (8)(1.25-1)/( 1.25) - 1]
x 1.013 x 105 x (2.1 x 10-3- 5.27 x 10-4)[ (8)(1.25-1)/( 1.25) - 1]
= 410.88 J
Indicated Power = W x N/60 = 410 x 800/60
= 5478.45 watts
ɳv =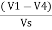 x 100
x 100
= 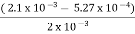
= 78.60 %
Isothermal work = P1(V1 – V4) x ln(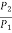 )
)
= 1.013 x 105 (2.1 x 10-3 – 5.27 x 10-4) x ln(8)
= 331.34 J
Isothermal power = WI x N/60
= 331. 34 x 800/60
= 4417.97 watts
ɳiso= 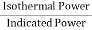 x 100
x 100
= 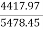 x 100
x 100
= 80.64 %
ɳmech = 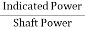 x 100
x 100
85 =  x 100
x 100
Shaft Power = 6445.23 Watts
= 6.44 kw
Q5) Explain the construction and working of single stage compressor.
A5)
Construction:
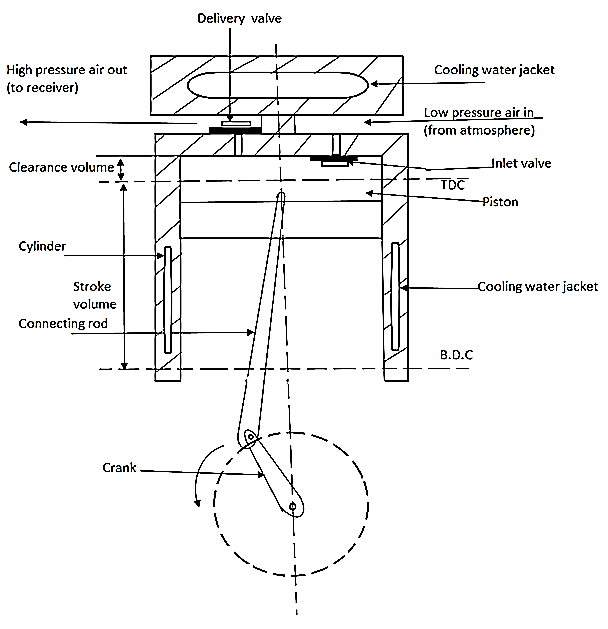
Working:
a). Suction Stroke:
b). Compression Stroke:
Q6) Derive the expression for work done on air in a reciprocatingcompressor without clearance.
A6)
Expression for work done on air in a reciprocating compressor without clearance.
A P-V diagram of a reciprocating compressor without clearance has been shown in fig.
Process 0-1 Constant pressure suction
Process 1-2 Polytrophic compression of air
Process 2-3 Constant pressure delivery.
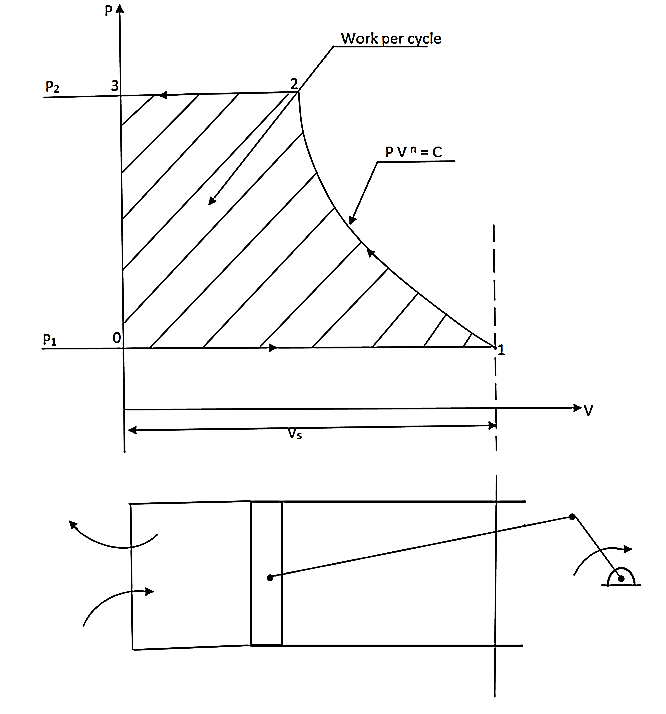
Work transfer will be given by area under the curve on P-V diagram.
Work transfer per cycle = -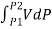 --- (1)
--- (1)
For process 1-2
PVn = C
Vn = C/P
V =  where K = C1/n
where K = C1/n
:. Put in eqn (1)
Work transfer per cycle = -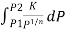
= 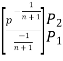
= - K[ P-1/(n + 1) / -1/n + 1 ]P2P1
= - K *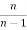 [ P2-1/(n + 1) – P1-1/(n + 1) ]
[ P2-1/(n + 1) – P1-1/(n + 1) ]
= 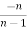 [ V2P21/n.P2-1/n. P2 – V1P11/n . P1-1/n. P1 ]
[ V2P21/n.P2-1/n. P2 – V1P11/n . P1-1/n. P1 ]
= 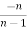 [ P2V2 – P1V1 ]
[ P2V2 – P1V1 ]
Hence,
Work done on the airper cycle= 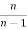 [ P2V2 – P1V1 ]
[ P2V2 – P1V1 ]
= 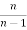 P1V1[
P1V1[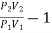 ]
]
=  P1V1[
P1V1[ 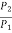 (
(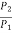 )-1/n– 1. . . (
)-1/n– 1. . . (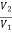 ) = (
) = (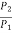 )-1/n
)-1/n
=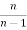 P1V1[
P1V1[ - 1]
- 1]
=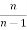 mRT1[
mRT1[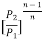 - 1]
- 1]
Q7) Define and Derive the Volumetric efficiency and Isentropic efficiency.
A7)
Volumetric efficiency:
It is defined as the ratio of actual volume of air sucked in per cycle to the swept volume per cycle.
ɳv = 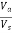
= 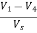
= 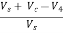
= 
= 1- 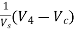
= 1- 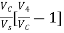
= 1-  ……… k = clearance ratio.
……… k = clearance ratio.
=1- k[( )1/n -1] (
)1/n -1] (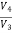 ) = (
) = (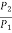 )1/n
)1/n
Isothermal efficiency:
It is defined as ratio of isothermal power to the indicated power. It is also called as compressor efficiency.
Isothermal efficiency = 
ɳiso = 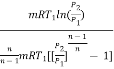 .. Single stage compressor
.. Single stage compressor
ɳiso = 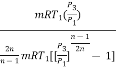 .. Double stage compressor
.. Double stage compressor
Q8) Describe the Actual indicator diagram for air compressor.
A8)
Actual indicator diagram for air compressor:
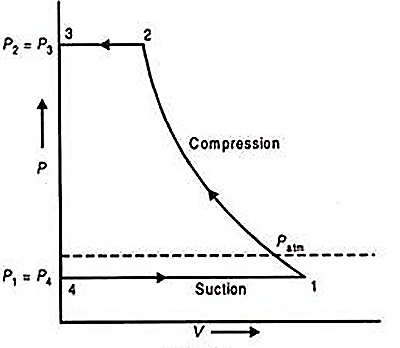
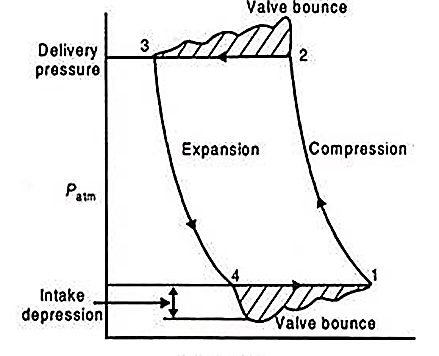
Theoretical Indicator Diagram Actual Indicator Diagram
Q9) Explain the construction and working of Roots blower.
A9)
Construction of Roots blower:
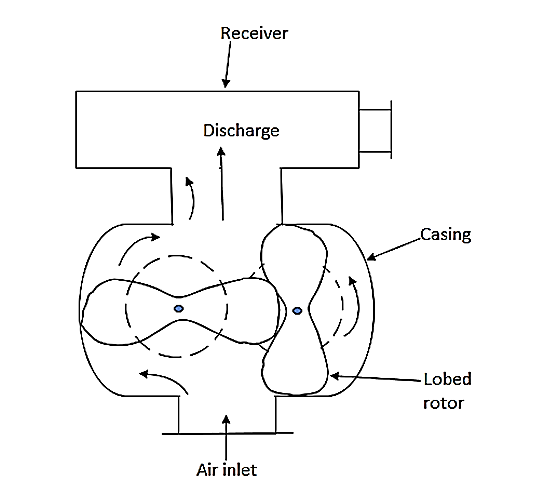
Fig. Roots Blower
Working:
Q10) Explain the construction and working of vane type rotary compressor.
A10)
Construction:
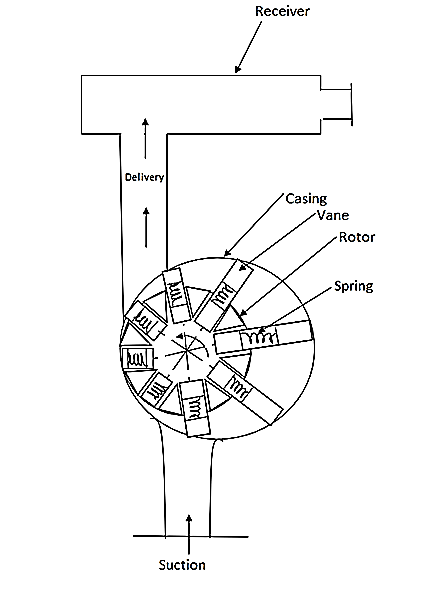
Fig. Vane type rotary compressor
Working:
Q11) Explain the construction and working of screw compressor.
A11)
Construction:
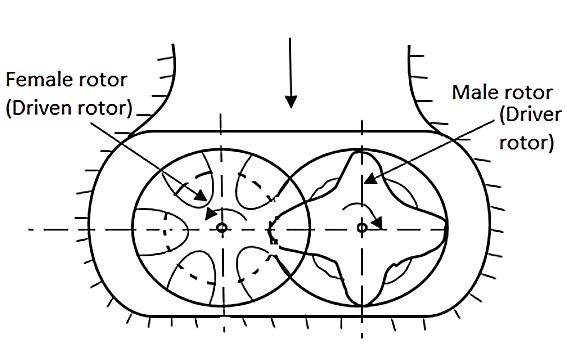
Fig. Screw compressor
Working: