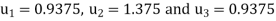Unit - 2

Numerical Solution of Differential Equation

Q1) Solve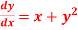, 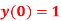using Taylor’s series method and compute .

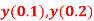A1)

Here 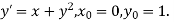This implies that .

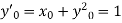Differentiating, we get

.

.

.

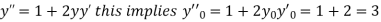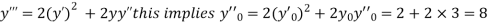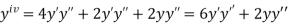
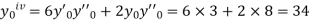The   Taylor’s series at ,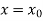

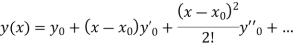
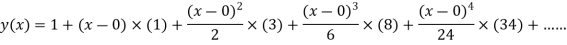
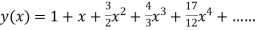(1)

At 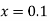in equation (1) we get

At 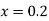in equation (1) we get

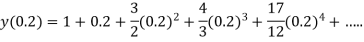
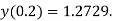
Q2) Solve 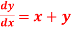numerically, start from 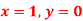and carry to using Taylor’s series method.

A2)

Here .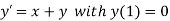

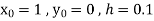
We have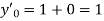

Differentiating, we get

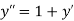implies that 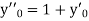or

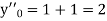
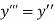implies that 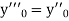or .

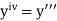implies that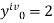

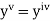implies that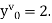

The   Taylor’s series at ,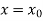

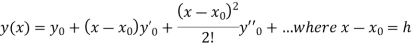
Or

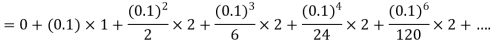
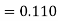
Here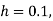

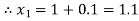
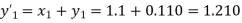
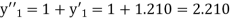
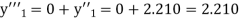
The   Taylor’s series

.

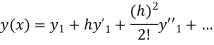
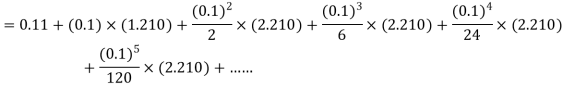
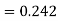Q3) Define Euler’s method.

A3)

In this method the solution is in the form of a tabulated values.

Integrating both side of the equation (i) we get

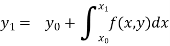
Assuming that 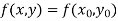 in 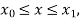 this gives Euler’s formula

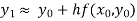
In general formula

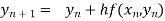, n=0,1, 2…...

Error estimate for the Euler’s method

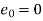
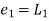
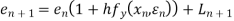
Q4) Use Euler’s method to find y (0.4) from the differential equation

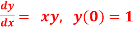    with h=0.1

A4)

Given equation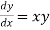  

Here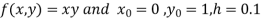

We break the interval in four steps.

So that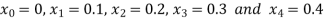

By Euler’s formula

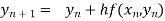, n=0,1,2,3   ……(i)

For n=0 in equation (i) we get

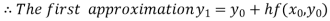
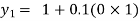
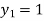
For n=1 in equation (i) we get

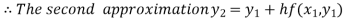
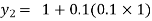
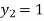.01

For n=2 in equation (i) we get

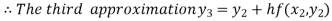
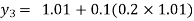
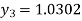
For n=3 in equation (i) we get

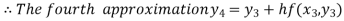
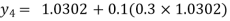
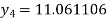
Hence y (0.4) =1.061106.

Q5) Using Euler’s method solve the differential equation for y at x=1 in five steps

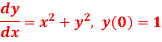
A5)

Given equation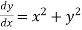  

Here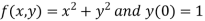 

No. Of steps n=5 and so that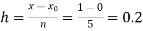

So that

Also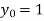

By Euler’s formula

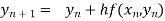, n=0,1,2,3,4   ……(i)

For n=0 in equation (i) we get

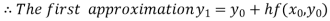
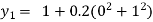
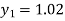
For n=1 in equation (i) we get

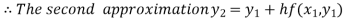
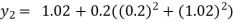
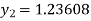
For n=2 in equation (i) we get

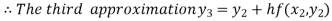
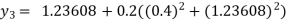
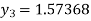
For n=3 in equation (i) we get

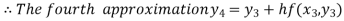
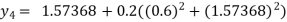
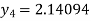
For n=4 in equation (i) we get

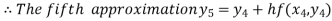
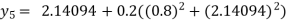
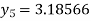
Hence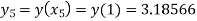 

Q6) Given 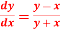 with the initial condition y=1 at   x=0.Find y for x=0.1 by Euler’s   method (five steps).

A6)

Given equation is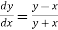  

Here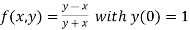   

No. Of steps n=5 and so that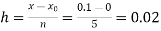

So that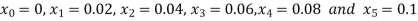

Also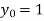

By Euler’s formula

, n=0,1,2,3,4   ……(i)

For n=0 in equation (i) we get

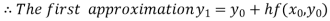
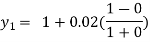
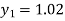
For n=1 in equation (i) we get

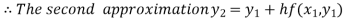
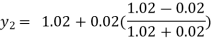
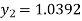
For n=2 in equation (i) we get

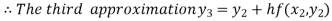
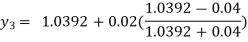
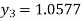
For n=3 in equation (i) we get

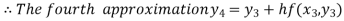
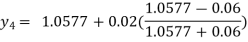
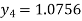
For n=4 in equation (i) we get

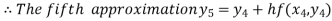
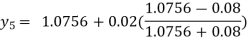
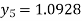
Hence  

Q7) Use modified Euler’s method to compute y for x=0.05.  Given that

Result correct to three decimal places.

A7)

Given equation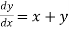  

Here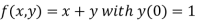

Take h = 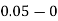 = 0.05

By modified Euler’s formula the initial iteration is

)

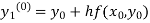
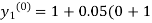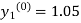
The iteration formula by modified Euler’s method is

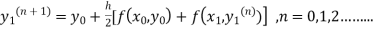-----(i)

For n=0 in equation (i) we get

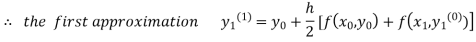
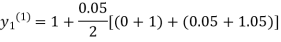
Where 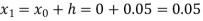  and 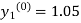 as above

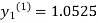
For n=1 in equation (i) we get

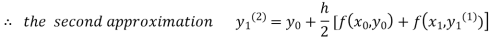
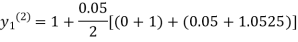
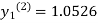
For n=3 in equation (i) we get

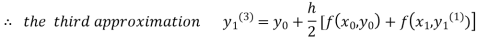
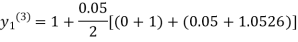
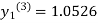
Since third and fourth approximation are equal.

Hence y=1.0526 at x = 0.05 correct to three decimal places.

Q8) Using modified   Euler’s method, obtain a solution of the equation

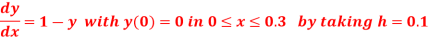
Given equation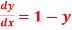 

A8)

Here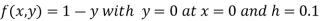

By modified Euler’s formula the initial iteration is

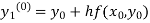
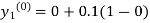
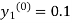
The iteration formula by modified Euler’s method is

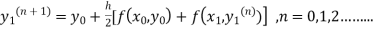-----(i)

For n=0 in equation (i) we get

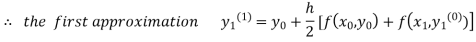
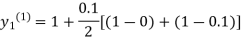
Where 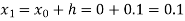  and 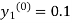 as above

For n=1 in equation (i) we get

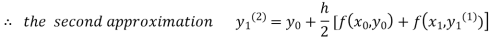
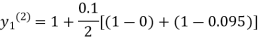
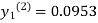
For n=2 in equation (i) we get

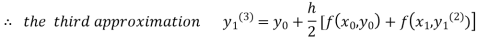
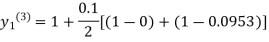
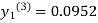
For n=3 in equation (i) we get

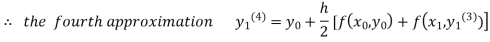
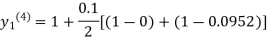
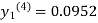
Since third and fourth approximation are equal.

Hence y=0.0952 at x=0.1

To calculate the value of 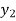 at x=0.2

By modified Euler’s formula the initial iteration is

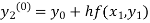
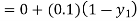
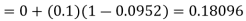
The iteration formula by modified Euler’s method is

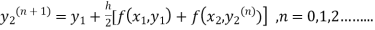-----(ii)

For n=0 in equation (ii) we get

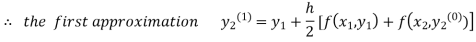
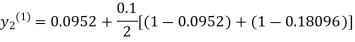
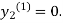1814

For n=1 in equation (ii) we get

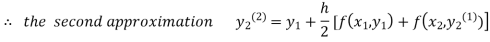
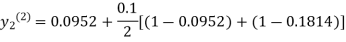
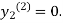1814

Since first and second approximation   are equal.

Hence y = 0.1814 at x=0.2

To calculate the value of 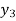 at x=0.3

By modified Euler’s formula the initial iteration is

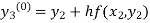
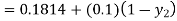
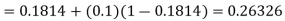
The iteration formula by modified Euler’s method is

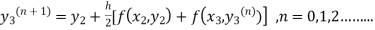-----(iii)

For n=0 in equation (iii) we get

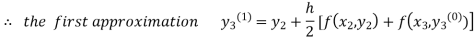
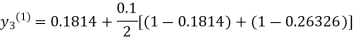
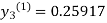
For n=1 in equation (iii) we get

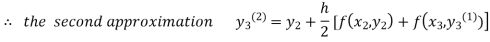
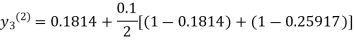
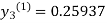
For n=2 in equation (iii) we get

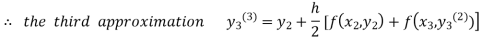
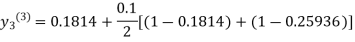
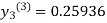
For n=3 in equation (iii) we get

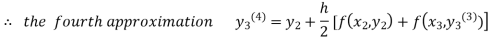
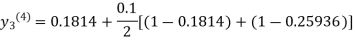
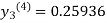
Since third and fourth approximation are same.

Hence y = 0.25936 at x = 0.3

Q9) What do you understand by Runge kutta fourth order method?

A9)

Consider the differential equation of first order

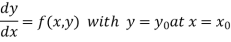
Let 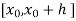 be the first interval.

A second order Runge Kutta formula  

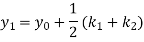
Where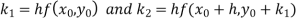

Rewrite as 

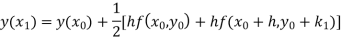
A fourth order Runge Kutta formula:

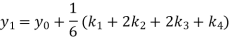
Where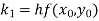 

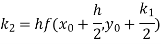
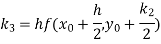
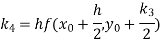
Q10) Use Runge Kutta method to find y when x=1.2 in step of h=0.1 given that

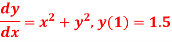
A10)

Given equation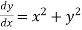

Here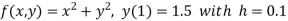

Also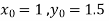

By Runge Kutta formula for first interval

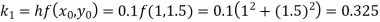
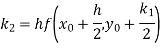
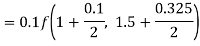
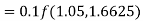
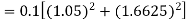
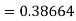
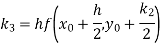
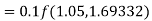
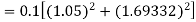
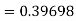
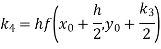
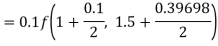
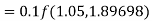
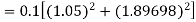
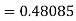
Again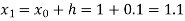

A fourth order Runge Kutta formula:

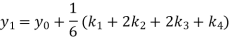
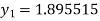
To find y at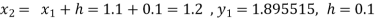 

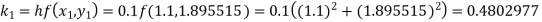
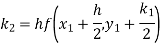
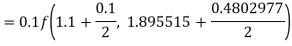
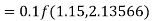
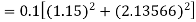
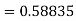
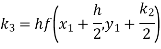
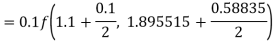
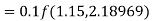
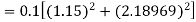
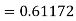
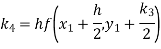
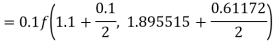
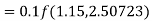
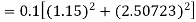
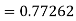
A fourth order Runge Kutta formula:

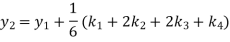
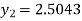
Q11) Apply Runge Kutta fourth order method to find an approximate value of y for x=0.2   in step of 0.1, if

A11)

Given equation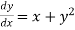 

Here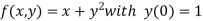 

Also

By Runge Kutta formula for first interval

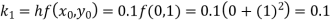
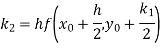
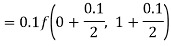
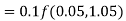
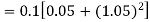
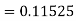
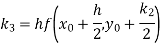
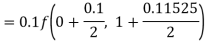
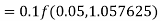
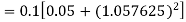
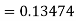
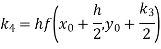
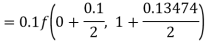
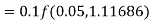
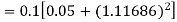
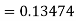
A fourth order Runge Kutta formula:

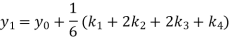
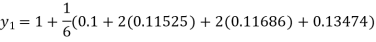
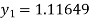
Again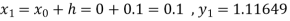

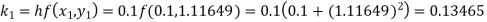
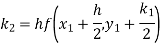
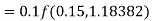
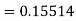
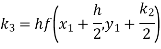
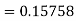
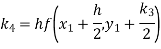
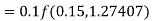
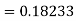
A fourth order Runge Kutta formula:

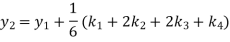
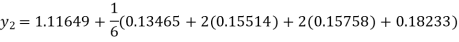
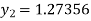
Q12) Using Runge Kutta method of fourth order, solve

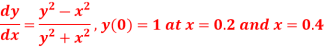
A12)

Given equation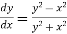 

Here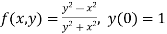 

Also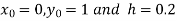

By Runge Kutta formula for first interval

)

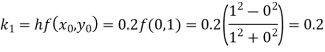
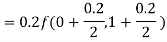
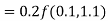
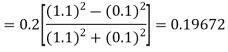
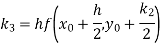
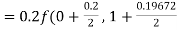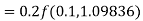
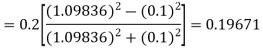
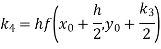
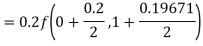
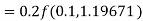
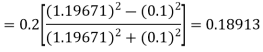
A fourth order Runge Kutta formula:

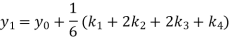
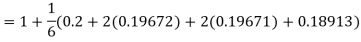
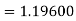
Hence at x = 0.2 then y = 1.196

To find the value of y at x=0.4. In this case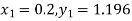

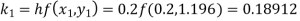
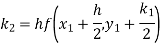
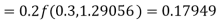
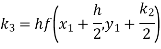
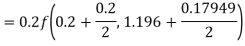
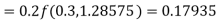
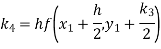
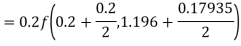
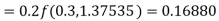
A fourth order Runge Kutta formula:

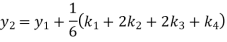
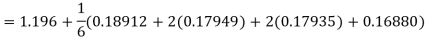
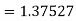
Hence at x = 0.4 then y=1.37527

Q13) Using Runge Kutta method of order four, solve   to find

A13)

Given second order differential equation is

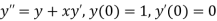
Let 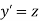  then above equation reduces to

Or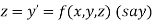

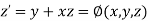(say)

Or .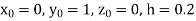

By Runge Kutta Method we have

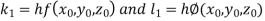
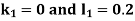
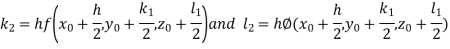
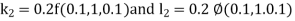
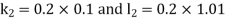
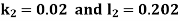
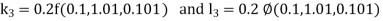
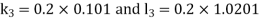
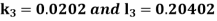
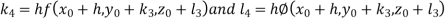
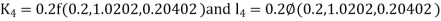
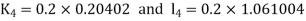
A fourth order Runge Kutta formula:

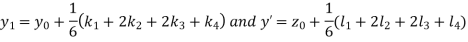
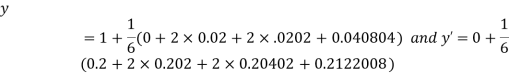
Q14) Using Runge Kutta method, solve

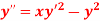for 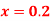correct to four decimal places with initial condition .

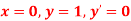A14)

Given second order differential equation is

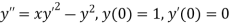
Let 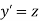  then above equation reduces to

Or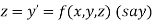

(say)

Or .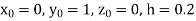

By Runge Kutta Method we have

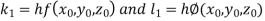
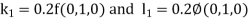
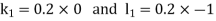
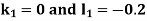
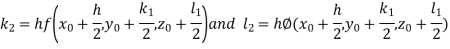
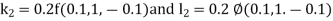
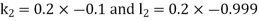
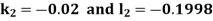
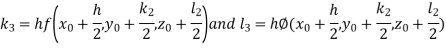
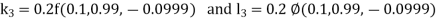
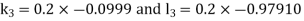
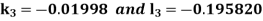
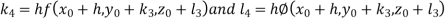
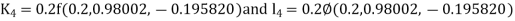
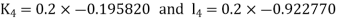
A fourth order Runge Kutta formula:

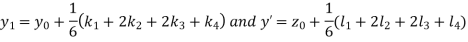
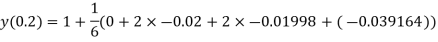
And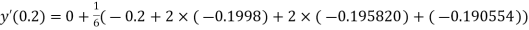  

.

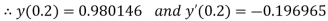Q15) Give the classification of partial differential equations.

A15)

The general linear partial differential equation of the second order in two independent variables is of the form.

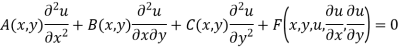
Such a PDE is said to be

1. Elliptic: if
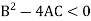
2. Parabolic: if
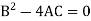
3. Hyperbolic: if

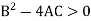
Q16) Solve the Laplace’s equation  in the domain

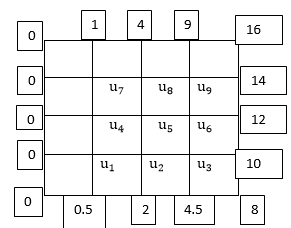
A16)

The initial values using five diagonal formula we have

Here ,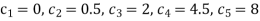 

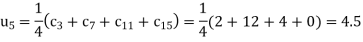
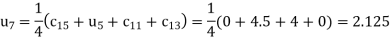
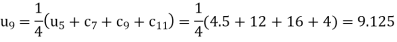
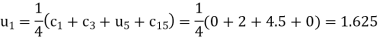
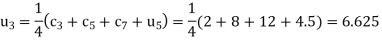
The remaining quantities are calculated by using standard five-point diagonal formulas.

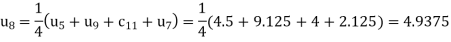
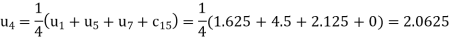
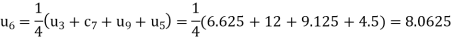
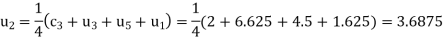
Hence 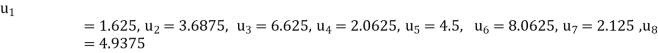and .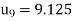

Q17) Solve the elliptical equation for

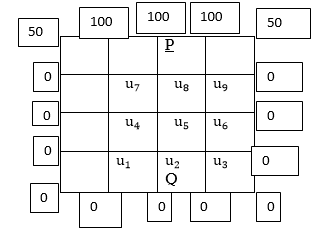
A17)

The initial values using five diagonal formula we have

Here , 

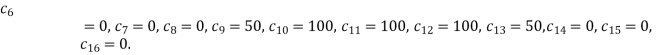
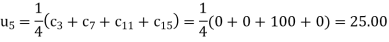
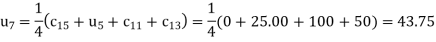
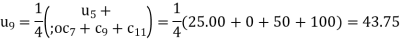
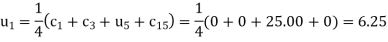
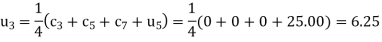
The remaining quantities are calculated by using standard five-point diagonal formulas.

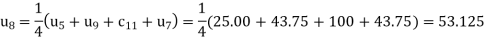
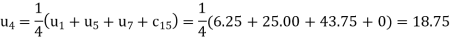
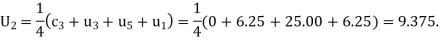
The Above is symmetric about PQ, so that .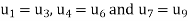

We will have iteration process using the Gauss Seidel Formula

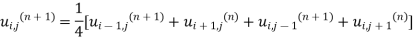
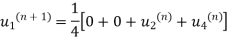
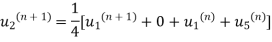
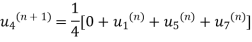
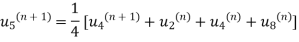
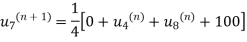
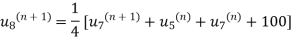
First iteration: Putting 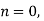we get

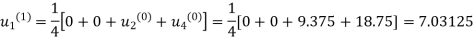
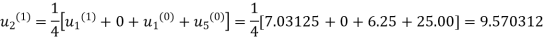
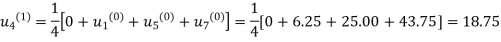
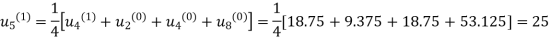
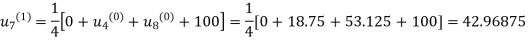
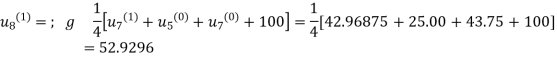
Second Iteration: Putting 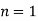, we get

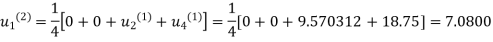
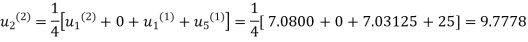
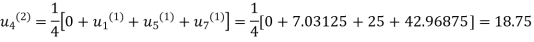
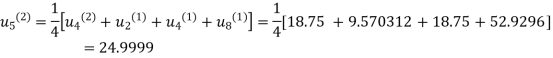
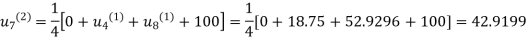
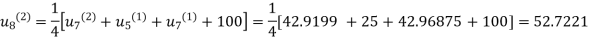
Third Iteration: Putting 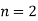, we get

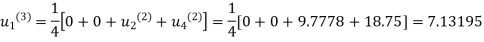
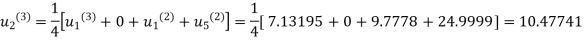
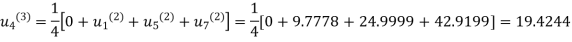
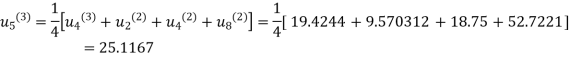
Fourth Iteration: Putting 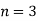, we get

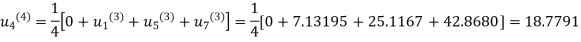
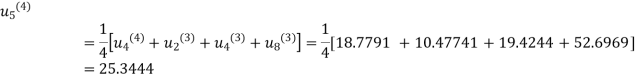
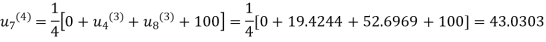
Fifth iteration: Putting n=4 we get

.

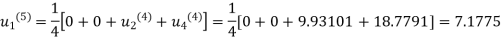
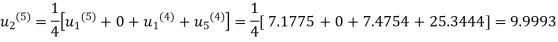
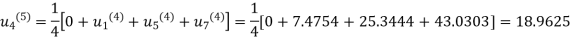
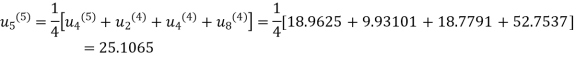
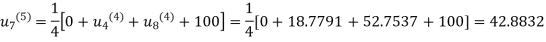
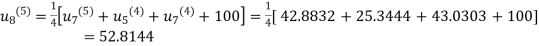Q18) Solve the equation 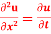with the conditions 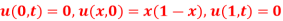. Assume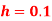. Tabulate u for 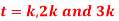choosing appropriate value of k?

A18)

Here 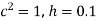and let   ,

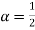Since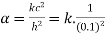

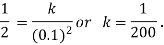
The Bendre-Schmidt recurrence formula we have

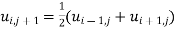  …. (i)

Also given .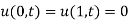

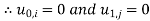for all values of j, i.e., the entries in the first and the last columns are zero.

Since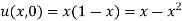

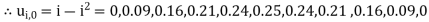  (Using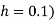

For .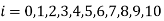

Putting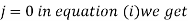

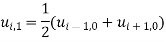
Putting 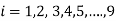successively we get

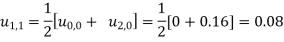
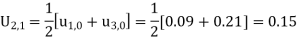
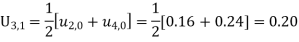
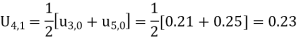
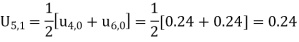
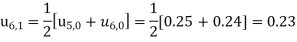
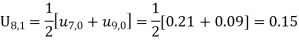
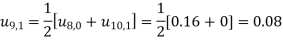
These will give the entries in the second row.

Putting 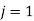in equation (i), we will get the entries of the third row.

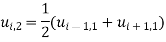
Similarly, 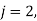successively in (i), the entries of the fourth rows are

Obtained.

Hence the values of  are as given in the below the table:

	0	1	2	3	4	5	6	7	8	9	10
0	0	0.09	0.16	0.21	0.24	0.25	0.24	0.21	0.16	0.09	0
1	0	0.08	0.15	0.20	0.23	0.24	0.23	0.20	0.15	0.08	0
2	0	0.075	0.14	0.19	0.22	0.23	0.22	0.19	0.14	0.075	0
3	0	0.07	0.133	0.18	0.21	0.22	0.21	0.18	0.133	0.07	0

Q19) Use the Bendre-Schmidt formula to solve the heat conduction problem

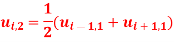
With the condition 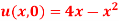and .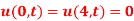

A19)

Let 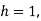we see 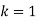when .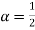

The initial condition is .

Also .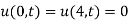

The iteration formula is

=

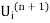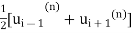
First iteration: Putting n=0, we get

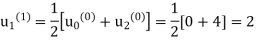
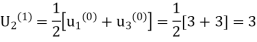
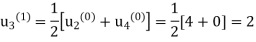
Second iteration: Putting n=1, we get

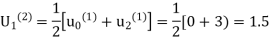
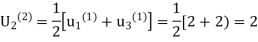
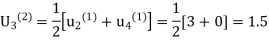
Third Iteration: putting n=3, we get

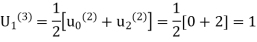
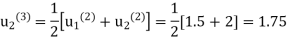
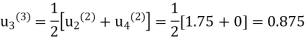
Fourth Iteration: putting n=3, we get

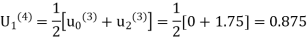
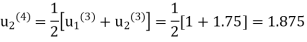
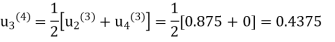
Fifth Iteration: putting n=4, we get

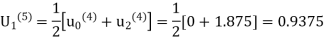
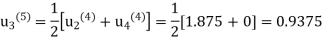
Hence the approximate solution is