UNIT 1

UNIT 1

UNIT 1

QUESTION/ANSWER BANK

1. Write the difference between Potential Difference and EMF ?

Ans. EMF:

POTENTIAL DIFFERENCE:

2.     Find the current I, through a resistor of resistance R = 4 Ω if the voltage across the resistor is 8 V.
Ans. Substitute  R by 4 and V by 8 in Ohm's law i.e. V = R I.
8 = 4 I
Solve for I,
I = 8 / 4 = 2 A

            Ans- 2A

3.     A heating element is marked 220 V, 660 W. What is the value of the current drawn by the element when connected to a 220 V DC Source?

Ans. Given that P= 660 W and V= 220V,

In a dc source, P=VI,

So, I = P/V

I= 660/220 = 3A

Ans = 3A

4.     If the current and voltage of an electric circuit are given as 3A and 9V respectively. Calculate the electrical power?

Ans. Given measures are,
I = 3A and V = 9V
The formula for electric power is,
P = VI
P = 9 × 3 = 27watts

5.     Calculate the power of an electrical circuit consisting of resistance 2Ω and a current 3A flowing through this circuit?

            Ans. Given parameters are,
               I = 3A and R = 2Ω
             Electric power formula is,

              P= I2R,

               P= 32×2
                 P = 9×2 = 18 watts

6.     There are n resistor each of resistance R. First they all are connected in series and equivalent resistance is Q. Now they are connected in parallel and equivalent resistance is S.  What is the ratio of Q and S?

Ans. Series combination
Q=R+R+R+......n times = nR

Parallel combination
1/S =1/R+1/R+1/R+...= n/R

Or S= R/n

So Q : S=n2 : 1

7.     An electric bulb draws a current of 0.5 A and works on 220 V on the average 8 hours a day. Find the power consumed by the bulb ?

Ans. Power of the electrical bulb is given by,
         P=V×I

          P=0.5×220 =110W

8.     Explain Kirchhoff’s current and voltage law?

Ans. The algebraic sum of currents meeting at a junction or node in a electric circuit is zero ② or the summation of all incoming current is always equal to summation of all outgoing current in an electrical network.

Explanation

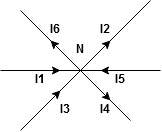
Assuming the incoming current to be positive and outgoing current negative we have

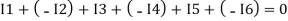
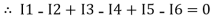
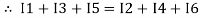
Ie incoming current = ∑ outgoing current thus, the above Law can also be stated as the sum of current flowing towards any junction in an electric circuit is equal to the sum of currents flowing away from that junction

Kirchhoff’s Voltage Law (KVL)

Statement : the algebraic summation of all Voltage in any closed circuit or mesh of loop zero.

Ie  ∑ Voltage in closed loop = 0 the summation of the Voltage rise (voltage sources) is equal to summation of the voltage drops around a closed loop in 0 circuit for explanation from here

Determination of sigh and direction of currents (Don’t write in exams just for understanding)

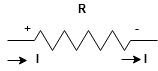
Current entering a resistor is +ve and leaving should be –ve

Now

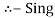
Potential RisePotential Drop

We are reading from +V to –Vwe are reading from –V to +V

potential drops potential rise

-V +V

9.     Resistors of R1= 10Ω, R2 = 4Ωand  R3 = 8Ω are connected up to two batteries (of negligible resistance) as shown. Find the current through each resistor.

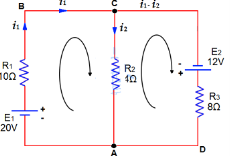
Ans. Assume currents to flow in directions indicated by arrows.
Apply KCL on Junctions C and A.
Therefore, current in mesh ABC = i1
Current in Mesh CA = i2
Then current in Mesh CDA = i1 – i2

Now, Apply KVL on Mesh ABC, 20V are acting in clockwise direction. Equating the sum of IR products, we get;
10i1 + 4i2 = 20 ……………. (1)

In mesh ACD, 12 volts are acting in clockwise direction, then:
8(i1–i2) – 4i2= 12

8i1 – 8i2 – 4i2= 12
8i1 – 12i2 = 12 ……………. (2)

Multiplying equation (1) by 3;
30i1 + 12i2 = 60

Solving for i1
30i1 + 12i2 = 60
8i1 – 12i2 = 12

______________
38i1 = 72

The above equation can be also simplified by Elimination or Cramer’s Rule.

i1 = 72/38 = 1.895 Amperes = Current in 10 Ohms resistor

Substituting this value in (1), we get:
10(1.895) + 4i2 = 20

4i2 = 20 – 18.95

i2 = 0.263 Amperes = Current in 4 Ohms Resistors.

Now,
i1 – i2 = 1.895 – 0.263 = 1.632 Amperes

10.  Show that the units 1V2/ Ω = 1W as implies by the equation P=V2/R

Ans. 1V2/Ω=  V2/(V/A)          ……………………….   (as we know 1 Ohm= V/A)

                   = AV = (C/s) * (J/C) = J/s  =1W

Hence,proved.