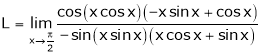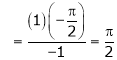Unit - 5

Expansion of Functions and Indeterminate forms

Question and answer

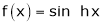1. Then

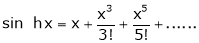
Proof:-

Here f(x) = sin hx.

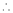By Maclaurin’s expansion,

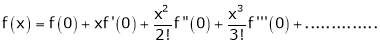(1)

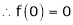
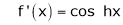
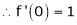
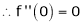
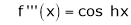
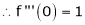
By equation (1) we get,

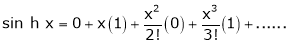
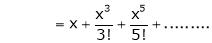
2.     . Then

Proof:-

Here f(x) = cos hx

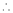By Maclaurin’s expansion

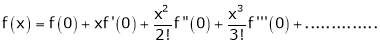(1)

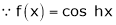
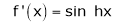
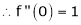
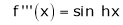
By equation (1)

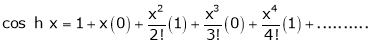
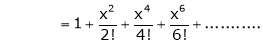
3.     f(x) = tan hx

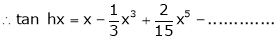
Proof:

Here f(x) = tan hx

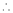By Maclaurin’s series expansion,

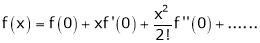… (1)

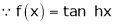
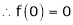
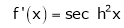
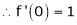
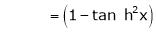
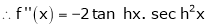
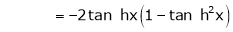
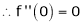
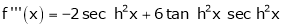
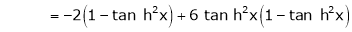
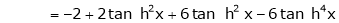
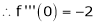
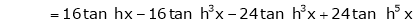
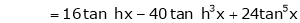
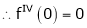
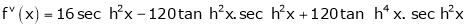
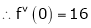
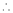By equation (1)

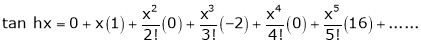
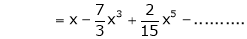
4. 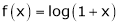    then

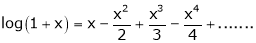
Proof:-

Here f(x) = log (1 + x)

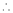By Maclaurin’s series expansion,

… (1)

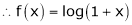
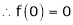
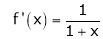
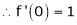
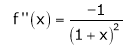
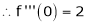
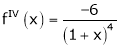
By equation (1)

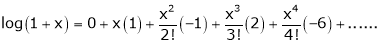
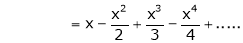
5.    

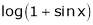
Solution:

Here f(x) = log (1 + sin x)

By Maclaurin’s Theorem,

… (1)

……..

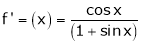
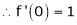
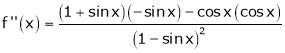
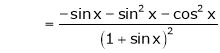
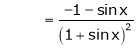
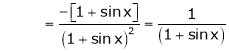
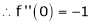
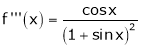
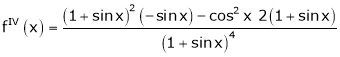
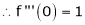
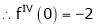equation (1) becomes,

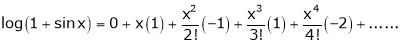
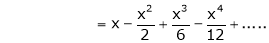
6.     Expand 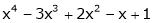in power of (x – 3)

Solution:

Let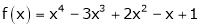

Here a = 3

Now by Taylor’s series expansion,

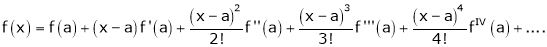… (1)

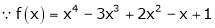
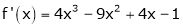
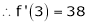
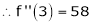
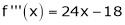
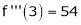
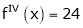
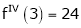
equation (1) becomes.

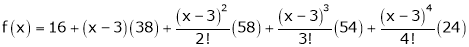
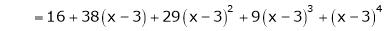
7.     Using Taylors series method expand

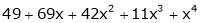in powers of (x + 2)

Solution:

Here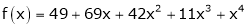

a = -2

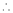By Taylors series,

… (1)

Since

,, …..

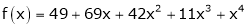
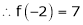
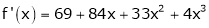
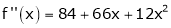
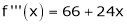
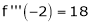
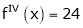
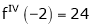Thus equation (1) becomes

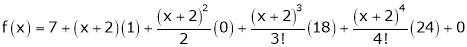
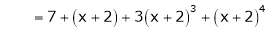
8.     Expand 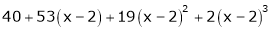in ascending powers of x.

Solution:

Here

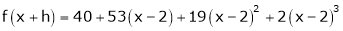
i.e.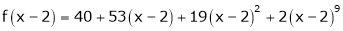

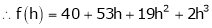
Here h = -2

By Taylors series,

… (1)

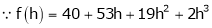
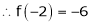
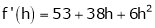
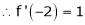
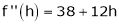
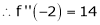
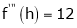
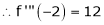
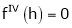
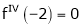
equation (1) becomes,

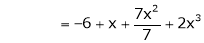
Thus

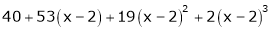
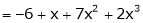
9.     Expand 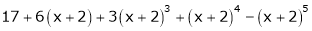in powers of x using Taylor’s theorem,

Solution:

Here

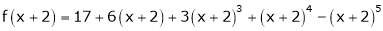
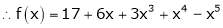
i.e.

Here

h = 2

By Taylors series

… (1)

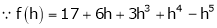
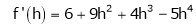
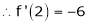
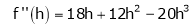
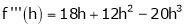
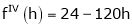
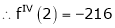
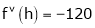
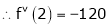
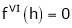
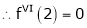
By equation (1)

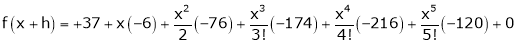
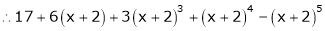
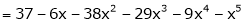
10. Evaluate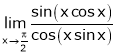

Solution:

Let

…

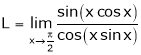By L – Hospital rule