Age | Strength percentage |
1 days | 16 % |
3 days | 40 % |
7 days | 65 % |
14 days | 90 % |
28 days | 99 % |
|
|
|
Longitudinal type 'e' in diagonal AC = (AC '- AC) / AC = (AC ’- AE) / AC = EC ’/ AC ----------- (1) When CE is specified from C to AC '. Since CC’s are very small, consider Angle ACB = angle ACB = 450 So EC '= CC'cos450 = CC' / √2 Long-term type ‘e’ = CC ’/ AC√2 = CC ’/ √2.BC.√2 = tan Φ / 2 = Φ / 2 = e S / 2 ------------------ (2) Where, Φ = CC ’/ BC represents the shear type (e S) For shear pressure τ and modulus of rigidity G, shear type (e S) = τ / G ------------ (3) Setting shear type (e S) = 2. Longitudinal type Longitudinal stiffness of diagonal AC = τ / 2 ----------- (4) Strain in a disconnected AC is also provided by = Strain due to the tensile stress in the AC - strain due to the compressive stress in the BD = τ / E - (–τ / m E) = τ / E (1 + 1 / m) ------------- (5) From equals (4) and (5), we obtain τ / 2G = τ / E (1 + 1 / m) or E = 2G (1 + 1 / m) or E = 2G (1 + µ) ------------- (6) Relationships between E, G and K: With reference to the (1) and (6) relationships obtained above, E = 2G (1 + µ) = 3K (1- 2 µ) Subtracting 1 / m from these two E expressions, we have E = 9KG / (G + 3K) Finally; E = 2G (1 + µ) = 3K (1 - 2 µ) or E = 9KG / (G + 3K) |
|
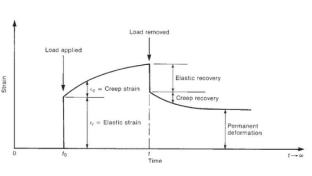
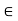 cc=( Stress / Et )×
cc=( Stress / Et )× 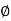 Where:E t: is a modulus of concrete reinforcement with age loading t
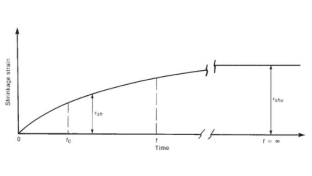
Where:E t: is a modulus of concrete reinforcement with age loading t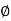 is the creep coefficient that can be taken from Figure 7.1 of BS8110: Part 2ShrinkageShrinkage is a reduction in concrete when drying and hardening due to evaporation of moisture content. The amount of reduction increases over time. The composite content contained in concrete are the most important factors contributing to the reduction. This is because the greater the concentration, the lower the shrinkage and the higher the composite content, the lower the water content of the cement and the performance will be. Decreased humidity also increases the risk of dehydration.
is the creep coefficient that can be taken from Figure 7.1 of BS8110: Part 2ShrinkageShrinkage is a reduction in concrete when drying and hardening due to evaporation of moisture content. The amount of reduction increases over time. The composite content contained in concrete are the most important factors contributing to the reduction. This is because the greater the concentration, the lower the shrinkage and the higher the composite content, the lower the water content of the cement and the performance will be. Decreased humidity also increases the risk of dehydration.
|
|
|