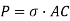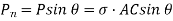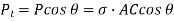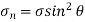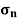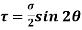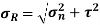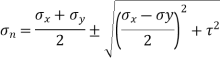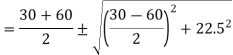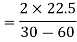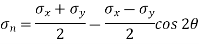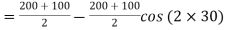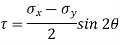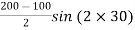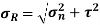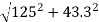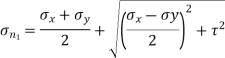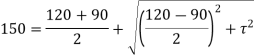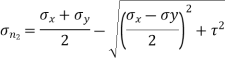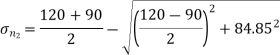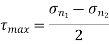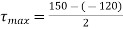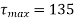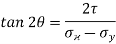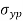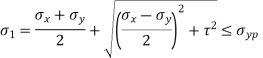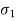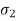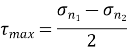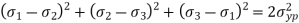|
Let, σ = tensile stress across the face AC, θ= angle, which AB makes with BC i.e. with the X-X axis We know that horizontal force acting on the face AC is Resolving the force perpendicular to the section AB, Now resolving the force tangential to section AB We know that normal stress across the section AB,
Shear stress acting, Resultant stress is given by,
|
Given: σx = 30 N/mm2, σy = 60 N/mm2 τ = 22.5 N/mm2 Solution To Find: σn1 ,σn2,θp1 ,θp2 Principal stress,
= 40 ± 27.04 Major principal stress σn1 = 45 + 27.04 = 72.04 N/mm2 Minor principal stress σn2 = 45 – 27.04 = 17.96 N/mm2 Position of principal plane,
= -1.5 tan (180 – 2θ) = –tan 2θ = 1.5 180 – 2θ = 56.3 2θ = 123.69 Θp1 = 61.8° Θp2 = 90 + θp1 = 90 + 61.8° = 151.8 |
= 150 – 50 × 0.5 = 125 MPa
Shear stress on the inclined plane,
= =50 sin60 τ = 43.3 MPa Resultant stress on the inclined plane,
= = 132.3 MPa
|
|
|
|
σn1 = 150 MPa, σx = 120 MPa, σ = –90 MPa Major principal stress is given by,
(150 – 15)2 = 1052 + τ2 τ = 84.85 N/mm2 Minor principal stress,
= -120N/mm2 Maximum shear stress,
Position of principal plane,
θp1 = 19.47 or 90 + 19.47 = 109.47 Position of maximum shear stress, θs1 = θp1+ 45° = 19.47° + 45° = 64.47° θs2 = θp2+ 45° = 154.47
|
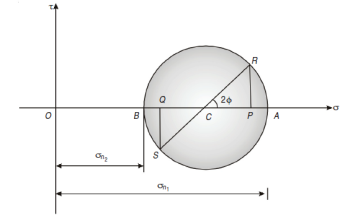
Given: σx = 360 kg/cm2, σy = 200 kg/cm2, τxy = 60 kg/cm2 To Find: σ1, σ2, φ |
| |
σn1 = OA × scale = 7.6 × 50 = 380 kg/cm2 σn1= OB × scale = 3.5 × 50 = 175 kg/cm2 Angle φ between the direction σx and σ1 ∠PCR = 2φ = 35 φ = 35/2 = 17.5°
| |
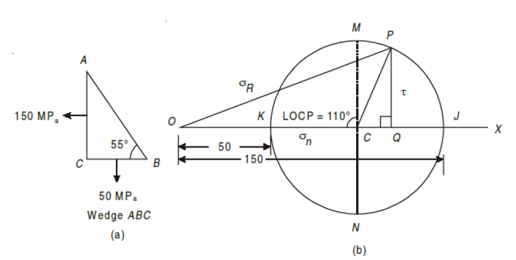
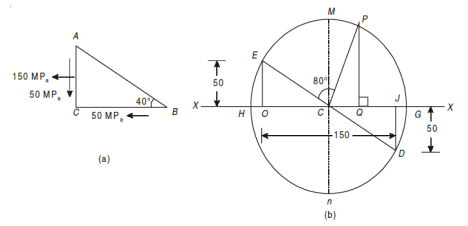
Given: Tensile stress along horizontal X – X axis (σx) = 150 MPa; shear stress (τxy) = – 50 MPa (Minus sign due to anticlockwise) and angle made by section with the tensile stress (θ) = 40°.
|
|
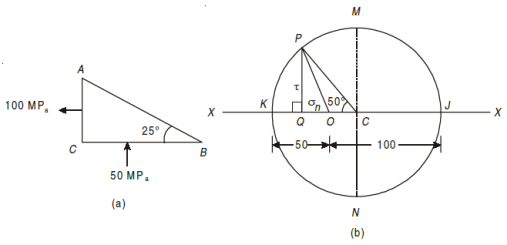
1. First of all take some suitable point O, and through it draw a horizontal line XOX. 2. Cut off OJ equal to the tensile stress on the plane AC (i.e. 150 MPa) to some suitable scale towards right. 3. Now erect a perpendicular at J below the line X – X and cut off JD equal to the negative shear stress on the plane AC (i.e. 50 MPa) to the scale. The point D represents the stress system on the plane AC. Similarly erect a perpendicular at O above the line X – X and cut off OE equal to the negative shear stress on the plane BC (i.e. 50 MPa) to the scale. The point E represents the stress systems on the plane BC. Join DE and bisect it at C. 4. Now with C as centre and radius equal to CD or CE draw the Mohr’s Circle of stresses meeting the line X – X at G and H. 5. Through C, draw two lines CM and CN at right angles to the line X – X meeting the circle at M and N. Also through C, draw a line CP making an angle of 2 × 40° = 80° with CE in clockwise direction meeting the circle at P. The point P represents the stress system on the section AB. By measurement, we find that the Normal stress (σn) = OQ = 112.2 MPa; Shear stress (τ) = QP = 82.5 MPa and maximum shear stress, that can exist on element (τmax) = ± CM = CN = 90.14 MPa.
|
|
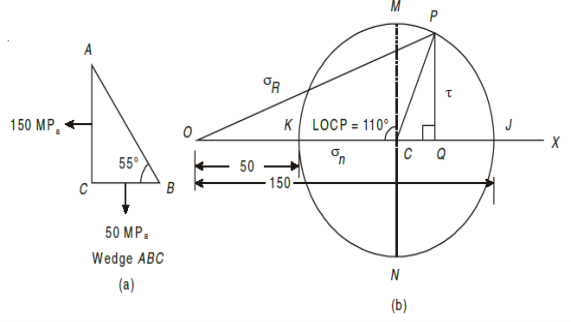
2. Cut off OJ and OK equal to the tensile stresses σx and σy respectively i.e., 150 MPa and 50 MPa to some suitable scale towards right. The point J represents the stress system on plane AC and the point K represents the stress system on plane BC. Bisect KJ at C. 3. Now with C as centre and radius equal to CJ or CK draw the Mohr’s circle of stresses. 4. Now through C draw two lines CM and CN at right angles to the line OX meeting the circle at M and N. Also through C draw a line CP making an angle of 2 × 55° = 110° with CK in clockwise direction meeting the circle at P. The point P represents the stress systems on the plane AB. 5. Through P, draw PQ perpendicular to the line OX. Join PO. By measurement we find, normal stress (σn) = OQ = 117.1 MPa, shear stress (τ) = QP = 47.0 MPa, resultant stress (σR) = OP = 126.17 MPa and maximum shear stress (τmax) = CM = ± 50 MPa.
|
|
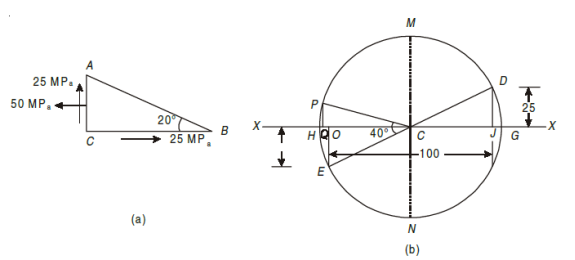
By measurement, we find that the normal stress σn = OQ = –23.21 MPa, shear stress (τ) = PQ = 57.45 MPa. Direction of the resultant stress ∠ POQ = 68.1° and maximum shear stress (τmax) = ± CM = CN = ± 75 MPa.
|
By measurement, we find that the normal stress σn = OQ = –23.21 MPa, shear stress (τ) = PQ = 57.45 MPa. Direction of the resultant stress ∠ POQ = 68.1° and maximum shear stress (τmax) = ± CM = CN = ± 75 MPa.
|
|
By measurement: (i) Normal stress (σn) = OQ = 4.4 MPa (Comp.), (ii) Shear stress τ = QP = 13.0 MPa, and (iii) Maximum shear (τmax) = CM = 55.9 MPa |
According to this theory maximum principal stress induced in material under complex load condition exceeds the maximum normal strain in simple test, the material fails. Failure condition,
This theory is not suitable to design machine elements as
Maximum Shear Stress Theory According to this theory when maximum shear strength in actual case exceeds maximum allowable shear stress in tension test then material fails.
This theory is widely used in the analytical way to calculate for the ductile materials. This has proved to be widely used theory for simple calculations in designing of simple machine elements.
Maximum Strain Energy Theory The energy stored in the material when the material is said to be deformed is called as strain energy. According to this theory when total strain energy exceeds the total strain energy in simple tensile test then material fails. The condition is given by,
This condition gives the limiting condition of the strain energy in the material or else the material will fail. |