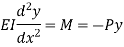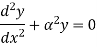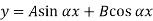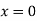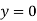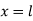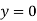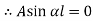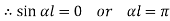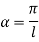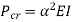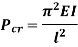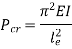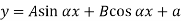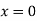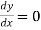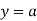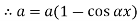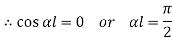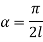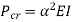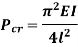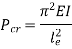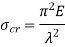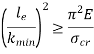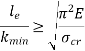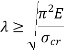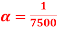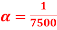|
|
|
|
|
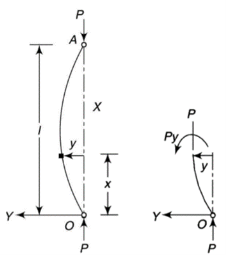
Y-axis is taken in such a way that deflection is positive. From the equation of bending, viewing from right end,
The equation can be written as
Where, The solution of the above equation is
When both ends are hinged. At And at If A = 0, y is zero for all values of load, hence, there is no bending.
Therefore, Euler’s Crippling load,
Comparing above equation with
We get,
|
|
Take Y-axis towards right for positive value of y. Viewing from left we get,
The equation can be written as
Where, The solution of the above equation is
When one end is fixed and other is free. At At
At
Therefore, Euler’s Crippling load,
Comparing above equation with
We get,
|
Crippling stress is given by,
The critical stress is directly proportional to modulus of elasticity and inversely proportional to square of slenderness ratio of column. |
|
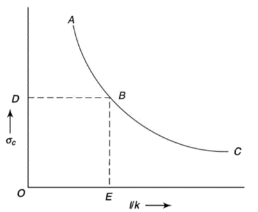
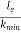 at point B is called as critical value of slenderness ratio.For any slenderness ratio above this value, column fails for buckling and for any value of slenderness ratio less than this value, the column fails by crushing and not by buckling.Hence, for short column, Euler’s Formula is not applicable.Mathematically, Euler’s Formula is applicable,If crushing stress
at point B is called as critical value of slenderness ratio.For any slenderness ratio above this value, column fails for buckling and for any value of slenderness ratio less than this value, the column fails by crushing and not by buckling.Hence, for short column, Euler’s Formula is not applicable.Mathematically, Euler’s Formula is applicable,If crushing stress 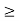 buckling stress
buckling stress
|
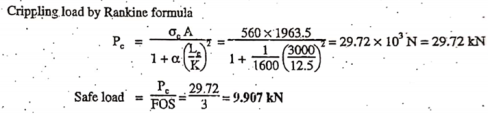
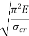 , then the Euler’s formula is applicable. This is the limitation of Euler’s formula. Q9) A 1.5 m long column has circular cross-section of 50 mm diameter. One end of column is fixed in direction and position and another end is free. Taking a factor of safety of 3, calculate safe load using Rankine’s formula. Take
, then the Euler’s formula is applicable. This is the limitation of Euler’s formula. Q9) A 1.5 m long column has circular cross-section of 50 mm diameter. One end of column is fixed in direction and position and another end is free. Taking a factor of safety of 3, calculate safe load using Rankine’s formula. Take  and
and 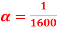 .
.
|
Take |
|