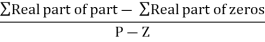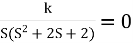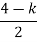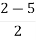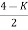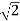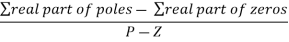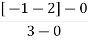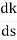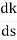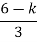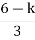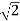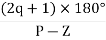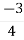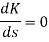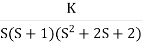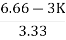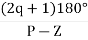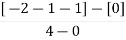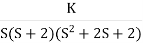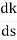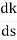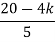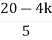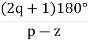undefined | unit 4 root locus techniques

UNIT-4ROOT LOCUS TECHNIQUE Q1)Sketch the root locus for given open loop transfer function G(S) =

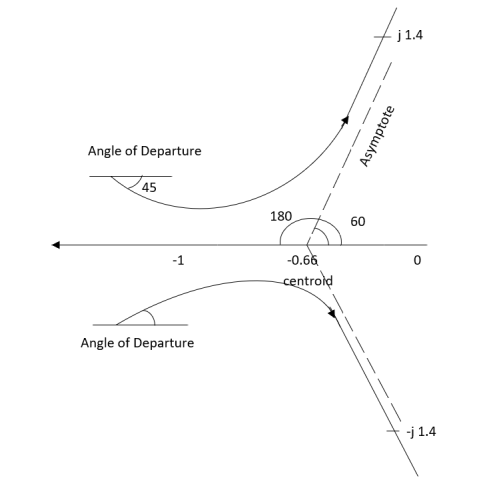
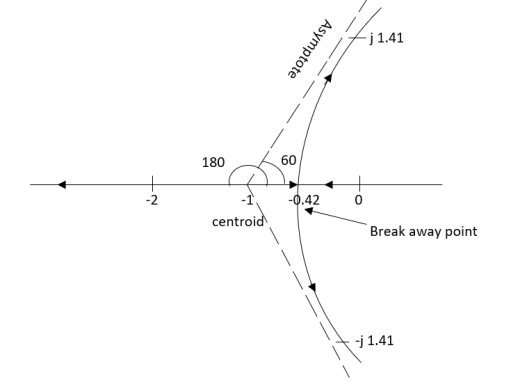
.A1) G(s) =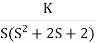

Number of Zeros = 0Number of polls S = (0, -1+j, -1-j) = (3).1) Number of Branches = max (P, Z) = max (3, 0) = 3.2) As there are no zeros in the system so, all branches terminate at infinity.3) As P>Z, branches terminate at infinity through the path shown by asymptotes

Asymptote = × 180° q = 0, 1, 2………..(p-z-1)

P=3, Z=0.

q= 0, 1, 2.

For q=0

Asymptote = 1/3 × 180° = 60°

For q=1

Asymptote = × 180°

= 180°

For q=2

Asymptote = × 180° = 300°

Asymptotes = 60°,180°,300°.

5) Asymptote intersects real axis at centroid

Centroid =

Centroid = -0.66

6) As poles are complex so angle of departure

øD = (2q+1)×180°+ø

ø = ∠Z –∠P.

Calculating ø for S=0

Join all the other poles with S=0

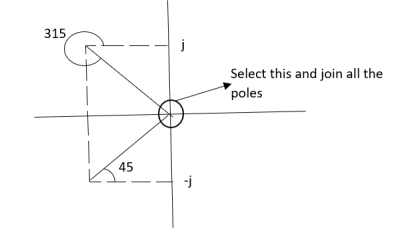
ø = ∠Z –∠P.

= 0-(315°+45°)

= -360°

ØD = (2q + 1)180 + ø.

= 180° - 360°

ØD = -180° (for q=0)

= 180° (for q=1)

=540° (for q=2)

Calculation ØD for pole at (-1+j)

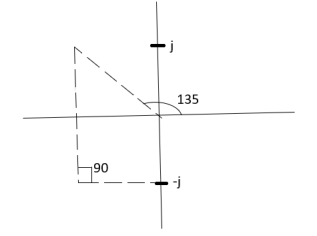
ø = ∠Z –∠P.

= 0 –(135°+90°)

= -225°

ØD = (2q+1) 180°+ø.

= 180-225°

= -45°

ØD = -45° (for q = 0)

= 315° (for q = 1)

= 675° (for q =2)

7) The crossing point on imaginary axis can be calculated by Routh Hurwitz the characteristic equation is.

1+G(s) H (s) = 0

S (S2+2s+2)+k = 0

S3+2s2+2s+K = 0

S3	1	1	0
S2	2	K	0
S1		0
S0	K

For stability > 0. And K > 0.

0<K<2.

So, when K=2 root locus crosses imaginary axis

S3 + 2S2 + 2S + 2 =0

For k

Sn-1 = 0 n : no. of intersection

S2-1 = 0 at imaginary axis

S1 = 0

= 0

K<4

For Sn = 0 for valve of S at that K

S2 = 0

2S2 + K = 0

2S2 + 2 = 0

2(S2 +1) = 0

32 = -2

S = ± j

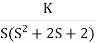The root locus plot is shown in figure 1

Fig 1 Root Locus for G(S) =

Q2)Sketch the root locus plot for the following open loop transfer function G(s) =

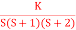A2)

Number of zero = 0, number of poles = 3

As P>Z, branches will terminates at infinity

There are no zeros so all branches will terminate at infinity.

The path for the branches is shown by asymptote

Asymptote = ×180°. q=0,1,………p-z-1

P=3, Z=0

q= 0,1,2.

For q = 0

Asymptote = × 180° = 60.

For q=1

Asymptote = × 180° = 180°

For q=2

Asymptote = × 180° = 300°

Asymptote intersect real axis at centroid

Centroid =

= = -1

As root locus lies between poles S= 0, and S= -1

So, calculating breakaway point.

= 0

The characteristic equation is

1+ G(s) H (s) = 0.

1+ = 0

K = -(S3+3S2+2s)

= 3S2+6s+2 = 0

3s2+6s+2 = 0

S = -0.423, -1.577.

So, breakaway point is at S=-0.423

because root locus is between S= 0 and S= -1

The intersection of root locus with imaginary axis is given by Routh criterion.

Characteristics equation is

S3+3S3+2s+K = 0

S3	1	2
S2	3	K
S1		0
S0	K

For k

Sn-1= 0 n: no. of intersection with imaginary axis

n=2

S1 = 0

= 0

K < 6 Valve of S at the above valve of K

Sn = 0

S2 = 0

3S2 + K =0

3S2 +6 = 0

S2 + 2 = 0

S = ± j

Fig 2 Root Locus for G(s) =

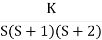The root locus plot is shown in fig. 2.Q3) Plot the root locus for the given open loop transfer function G(s) =

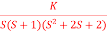A3)

Number of zeros = 0 number of poles = 4

P = (S=0,-1,-1+j,-1-j) = 4

As P>Z all the branches will terminated at infinity.

As no zeros so all branches terminate at infinity.

The path for branches is shown by asymptote.

Asymptote = q = 0,1,…..(Þ-z-1)

q=0,1,2,3. (P-Z = 4-0)

for q=0

Asymptote = ×180° =45°

For q=1

Asymptote = ×180° =135°

For q=2

Asymptote = ×180° =225°

For q=3

Asymptote = ×180° =315°

Asymptote intersects real axis at unmarried

Centroid =

Centroid = = = -0.75

6) As poles are complex so angle of departure is

ØD = (2q+1) ×180 + ø ø = ∠Z –∠P.

A) Calculating Ø for S=0

ø = ∠Z –∠P.

= 0 –[315° + 45°]

Ø = -360°

For q = 0

ØD = (2q+1) 180° + Ø

= 180 - 360°

ØD = -180°

b) Calculating Ø for S=-1+j

ø = ∠Z –∠P.

= 0-[135° + 90° + 90°]

Ø = -315°

For q=0

ØD= (2q+1) 180° +Ø

= 180° -315°

ØD = -135°

ØD for S=1+j will be ØD = 45°

7) As the root locus lie between S=0 and S=-1

So, the breakaway point is calculated

1+ G(s)H(s) = 0

1+ = 0

(S2+S)(S2 +2S+2) + K =0

K = -[S4+S3+2s3+2s2+2s2+2s]

= 4S3+9S2+8S+2=0

S = -0.39, -0.93, -0.93.

The breakaway point is at S = -0.39 as root locus exists between S= 0 and S=-1

8) Intersection of root locus with imaginary axis is given by Routh Hurwitz

I + G(s) H(s) = 0

K+S4+3S3+4S2+2S=0

S4	1	4	K
S3	3	2
S2	3.33	K
S1
S0	K

For system to be stable

6.66>3K

0<K<2.22.

For K = 2.22

3.3352+K =0

3.3352 + 2.22 = 0

S2 = -0.66

S = ± j 0.816.

The root locus plot is shown in figure 3.

Fig 3 Root Locus for G(s) =

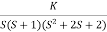Q4) Plot the root locus for open loop system G(s) =

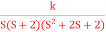A4)

Number of zero = 0 number of poles = 4 located at S=0, -2, -1+j, -1-j.

As no zeros are present so all branches terminated at infinity.

As P>Z, the path for branches is shown by asymptote

Asymptote =

q = 0,1,2……p-z-1

For q = 0

Asymptote = 45°

q=1

Asymptote = 135°

q=2

Asymptote = 225°

q=3

Asymptote = 315°

Asymptote intersects real axis at centroid.

Centroid =

Centroid = -1.

As poles are complex so angle of departure is

ØD=(2q+1)180° + Ø

ø = ∠Z –∠P

= 0-[135°+45°+90°]

= 180°- 270°

ØD = -90°

As root locus lies between two poles so calculating point. The characteristic equation is

1+ G(s)H(s) = 0

1+ = 0.

K = -[S4+2S3+2S2+2S3+4S2+4S]

K = -[S4+4S3+6S2+4S]

= 0

= 4s3+12s2+12s+4=0

S = -1

So, breakaway point is at S = -1

Intersection of root locus with imaginary axis is given by Routh Hurwitz.

S4+4S3+6S2+4s+K = 0

S4	1	6	K
S3	4	4
S2	5	K
S1
S0	K

≤ 0

K≤5.

For K=5 valve of S will be.

5S2+K = 0

5S2+5 = 0

S2 +1 = 0

S2 = -1

S = ±j.

The root locus is shown in figure 4.

Fig 4 Root Locus For G(s) =

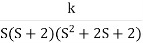Q5) Plot the root locus for open loop transfer function G(s) =

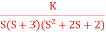A5)

Number of zeros = 0. Number of poles = 4 located at S=0, -3, -1+j, -1-j.

As no. zero so all branches terminate at infinity.

The asymptote shows the both to the branches terminating at infinity.

Asymptote = q=0,1,….(p-z).

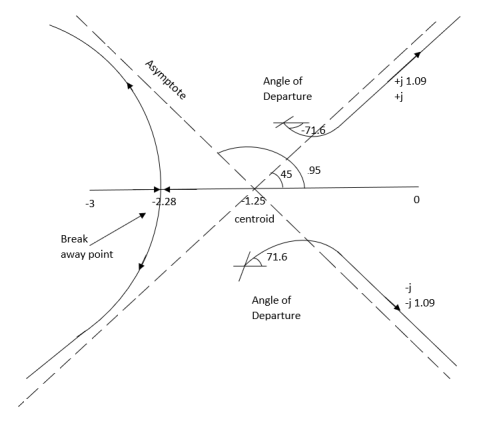
For q = 0

Asymptote = 45

For q = 1

Asymptote = 135

For q = 2

Asymptote = 225

For q = 3

Asymptote = 315

(4). The asymptote intersects real axis at centroid.

Centroid = ∑Real part of poles - ∑Real part of zero / P – Z

= [-3-1-1] – 0 / 4 – 0

Centroid = -1.25

(5). As poles are complex so angle of departure

φD = (29 + 1)180 + φ

ø = ∠Z –∠P.

= 0 – [ 135 + 26.5 + 90 ]

= -251.56

For q = 0

φD = (29 + 1)180 + φ

= 180 – 215.5

φD = - 71.56

(6). Break away point dk / ds = 0 is at S = -2.28.

(7). The intersection of root locus on imaginary axis is given by Routh Hurwitz.

1 + G(S)H(S) = 0

K + S4 + 3S3 + 2S3 + 6S2 + 2S2 + 6S = 0

S4 1 8 K

S3 5 6

S2 34/5 K

S1 40.8 – 5K/6.8

K ≤ 8.16

For K = 8.16 value of S will be

6.8 S2 + K = 0

6.8 S2 + 8.16 = 0

S2 = - 1.2

S = ± j1.09

The plot is shown in figure 20.

Fig 5 Root Locus for G(s) =

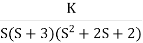Q6) Sketch the root locus for open loop transfer function. G(S) = K(S + 6)/S(S + 4)A6)

Number of zeros = 1(S = -6)

Number of poles = 2(S = 0, -4)

As P > Z one branch will terminate at infinity and the other at S = -6.

For Break away and breaking point

1 + G(S)H(S) = 0

1 + K(S + 6)/S(S + 4) = 0

dk/ds = 0

S2 + 12S + 24 = 0

S = -9.5, -2.5

Breakaway point is at -2.5 and Break in point is at -9.5.

Root locus will be in the form of a circle. So finding the centre and radius. Let S =  + jw.

G( + jw) = K( + jw + 6)/(  + jw)(  + jw + 4 ) = +- π

tan-1 w/  + 6 - tan-1 w/  – tan-1 w /  + 4 = - π

taking tan of both sides.

w/  + w/  + 4 / 1 – w/  w/  + 4 = tan π + w /  + 6 / 1 - tan π w/  + 6

w/  + w/  + 4 = w/  + 6[ 1 – w2 /  ( + 4) ]

(2 + 4)(  + 6) = (2 + 4  – w2)

2 2 + 12 + 4 + 24 = 2 + 4 – w2

22 + 12 + 24 = 2 – w2

2 + 12 – w2 + 24 = 0

Adding 36 on both sides

( + 6)2 + (w + 0)2 = 12

The above equation shows circle with radius 3.46 and centre (-6, 0) the plot is shown in figure 6

Fig 6 Root locus for G(S) = K (S + 6)/S (S + 4)

Q7) What are the effects of adding poles on a closed to system?A7) Effect of Addition of Poles:1) The root locus is shifted towards imaginary axis.2) The system becomes oscillatory.3) The stability of system decreases.4) The settling time increases.5) The range of k reduces.Q8) What are the effects of adding zero on a closed to system?A8) Effect of addition of zeros1) The root locus shifts away from imaginary axis.2) Stability of system increases.3) The settling time decreases.4) The gain margin increases.5) The system becomes less oscillatory.Q9) Explain angle and magnitude criterion for root locus?A9) Angle condition:-LG(S)H(S) = +-1800(2 KH) (K = 1,2,3,--)If angle is odd multiple of 1800 it satisfies above condition.Magnitude condition:| G(S)H(S) = 1 | at any point on root locus. The magnitude condition can be applied only if angle condition is satisfied.Q10) How can we comment on systems stability from its root locus plot?A10) Absolutely stable: If the root locus (all the branches) lies within the left side of S-plane it is absolutely stable.Conditionally Stable: If some part of the root locus lies on the left half and same Part on the right of S-plane then it is conditionally stable.Unstable: If the root locus lies completely on right side of S-plane then it is unstable.

Sign Up