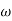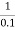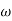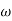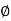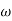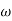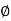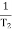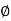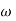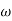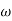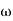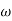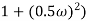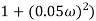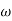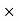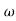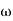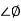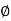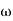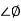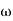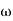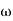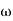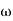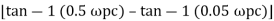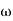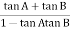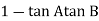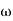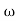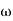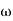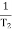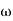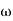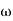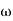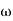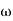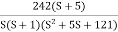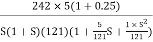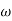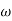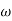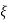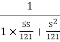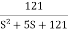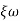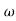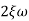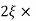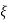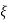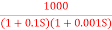 A1)
A1)
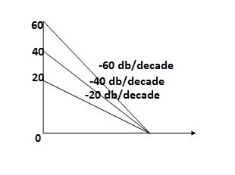
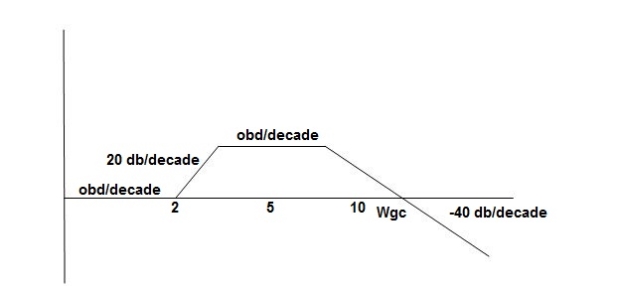
G(j This is type 0 system . so initial slope is 0 dB decade. The starting point is given as 20 log10 K = 20 log10 1000 = 60 dB Corner frequency
Slope after 2. For phase plot
For phase plot
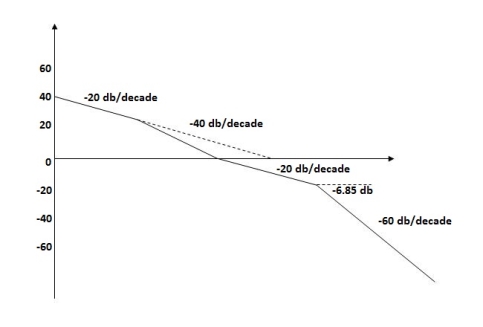
100 -900 200 -9.450 300 -104.80 400 -110.360 500 -115.420 600 -120.00 700 -124.170 800 -127.940 900 -131.350 1000 -134.420 The plot is shown in figure 1 |
|
|
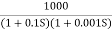 Q2) For the given transfer function determine G(S) =
Q2) For the given transfer function determine G(S) = 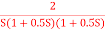 Gain cross over frequency phase cross over frequency phase mergence and gain marginA2)
Gain cross over frequency phase cross over frequency phase mergence and gain marginA2)Initial slope = 1 N = 1 , (K)1/N = 2 K = 2 Corner frequency
2. phase
1 -119.430 5 -172.230 10 -195.250 15 -209.270 20 -219.30 25 -226.760 30 -232.490 35 -236.980 40 -240.570 45 -243.490 50 -245.910
Finding M = 4 =
Let X3 (6.25 X1 = 2.46 X2 = -399.9 X3 = -6.50 For x1 = 2.46
for phase margin PM = 1800 -
= -164.50 PM = 1800 - 164.50 = 15.50 For phase cross over frequency (
-1800 = -900 – tan-1 (0.5 -900 – tan-1 (0.5 Taking than on both sides Tan 900 = tan-1 Let tan-1 0.5
1 =0.5
The plot is shown in figure 2. |
|
|
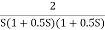 Q3)For the given transfer function G(S) =
Q3)For the given transfer function G(S) = 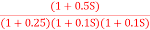 Plot the rode plot find PM and GMA3)
Plot the rode plot find PM and GMA3)T1 = 0.5 Zero so, slope (20 dB/decade) T2 = 0.2 Pole , so slope (-20 dB/decade) T3 = 0.1 = T4 = 0.1
Phase plot
500 -177.30 1000 -178.60 1500 -179.10 2000 -179.40 2500 -179.50 3000 -179.530 3500 -179.60
GM = 00 PM = 61.460 The plot is shown in figure 3 |
|
|
|
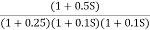 Q4) For the given transfer function plot the bode plot (magnitude plot) G(S) =
Q4) For the given transfer function plot the bode plot (magnitude plot) G(S) = 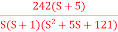 A4) Given transfer function
A4) Given transfer functionG(S) = Converting above transfer function to standard from G(S) = =
T1 = T2 = 1 , 4. Initial slope will cut zero dB axis at (K)1/N = 10 i.e 5. finding T(S) = T(S)= Comparing with standard second order system equation S2+2
5. Maximum error M = -20 log 2 = +6.5 dB |
|
|
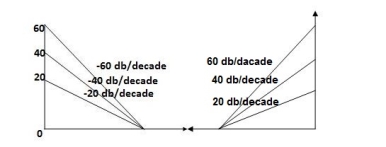
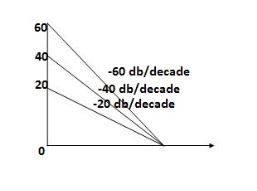
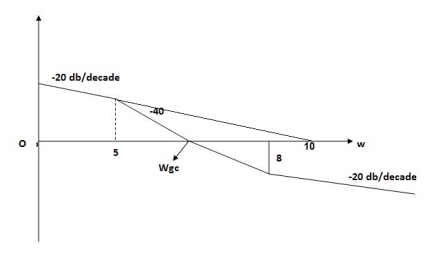
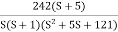 Q5) For the given plot determine the transfer function
Q5) For the given plot determine the transfer function
|
 = 10 so
= 10 so 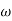 1 =
1 =  = 0.2 rad/sec
= 0.2 rad/sec 2 =
2 =  = 0.125 rad/sec3. At
= 0.125 rad/sec3. At 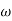 = 5 the slope becomes -40 dB/decade, so there is a pole at
= 5 the slope becomes -40 dB/decade, so there is a pole at 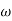 = 5 as slope changes from -20 dB/decade to -40 dB/decade4. At
= 5 as slope changes from -20 dB/decade to -40 dB/decade4. At 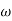 = 8 the slope changes from -40 dB/decade to -20 dB/decade hence 5. is a zero at
= 8 the slope changes from -40 dB/decade to -20 dB/decade hence 5. is a zero at 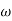 = 8 (-40+(+20) = 20)Hence transfer function is T(S) =
= 8 (-40+(+20) = 20)Hence transfer function is T(S) = 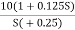 Q6) Explain correlation between time and frequency response?A6) The transfer function of second order system is shown as
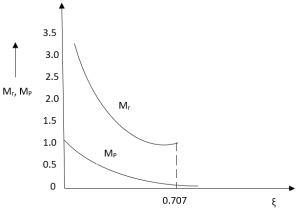
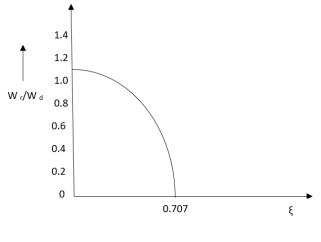
Q6) Explain correlation between time and frequency response?A6) The transfer function of second order system is shown asC(S)/R(S) = W2n / S2 + 2ξWnS + W2n - - (1) ξ = Ramping factor Wn = Undamped natural frequency for frequency response let S = jw C(jw) / R(jw) = W2n / (jw)2 + 2 ξWn(jw) + W2n Let U = W/Wn above equation becomes T(jw) = W2n / 1 – U2 + j2 ξU so, | T(jw) | = M = 1/√(1 – u2)2 + (2ξU)2 - - (2) T(jw) = φ = -tan-1[ 2ξu/(1-u2)] - - (3) For sinusoidal input the output response for the system is given by C(t) = 1/√(1-u2)2 + (2ξu)2Sin[wt - tan-1 2ξu/1-u2] - - (4) The frequency where M has the peak value is known as Resonant frequency Wn. This frequency is given as (from eqn (2)). dM/du|u=ur = Wr = Wn√(1-2ξ2) - - (5) from equation(2) the maximum value of magnitude is known as Resonant peak. Mr = 1/2ξ√1-ξ2 - - (6) The phase angle at resonant frequency is given as Φr = - tan-1 [√1-2ξ2/ ξ] - - (7)
As we already know for step response of second order system the value of damped frequency and peak overshoot are given as Wd = Wn√1-ξ2 - - (8) Mp = e- πξ2|√1-ξ2 - - (9) |
| |
|
|
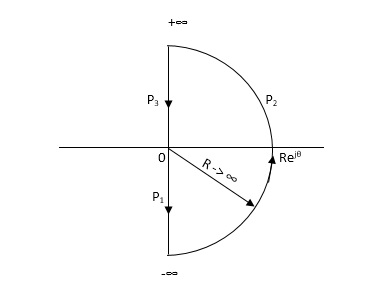
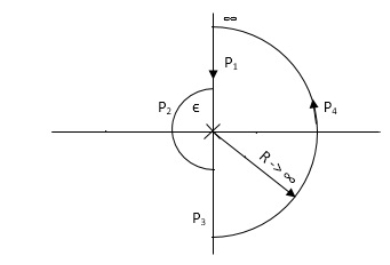
NYQUIST PATH :- P1 = W – (0 to - ∞) P2 = ϴ( - π/2 to 0 to π/2 ) P3 = W(+∞ to 0) |
|
Substituting S = jw G(jw) = 1/jw + 1 M = 1/√1+W2 Φ = -tan-1(W/I) for P1 :- W(0 to -∞) W M φ 0 1 0 -1 1/√2 +450 -∞ 0 +900 Path P2 :- W = Rejϴ R ∞ϴ -π/2 to 0 to π/2 G(jw) = 1/1+jw = 1/1+j(Rejϴ) (neglecting 1 as R ∞) M = 1/Rejϴ = 1/R e-jϴ M = 0 e-jϴ = 0 Path P3 :- W = -∞ to 0 M = 1/√1+W2 , φ = -tan-1(W/I) W M φ ∞ 0 -900 1 1/√2 -450 0 1 00 The Nyquist Plot is shown in fig below
|
|
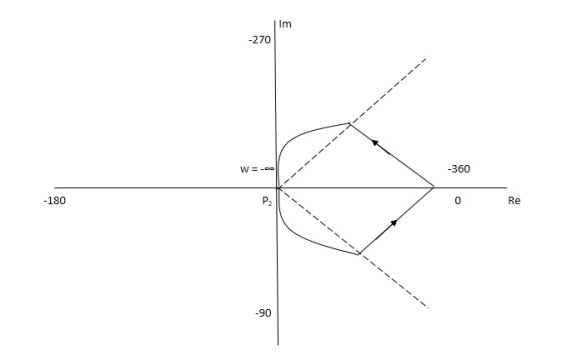
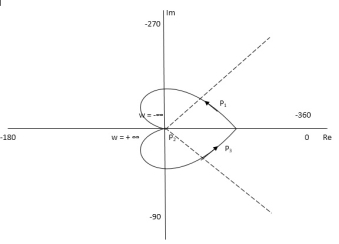
Path P1 W(0 to -∞) M = 1/√42 + w2 √52 + w2 Φ = -tan-1(W/4) – tan-1(W/5) W M Φ 0 1/20 00 -1 0.047 25.350 -∞ 0 +1800 Path P3 will be the mirror image across the real axis. Path P2 :ϴ(-π/2 to 0 to +π/2) S = Rejϴ G(S) = 1/(Rejϴ + 4)( Rejϴ + 5) R∞ = 1/ R2e2jϴ = 0.e-j2ϴ = 0 The plot is shown in fig below. From plot N=0, Z=0, system stable.
|
|
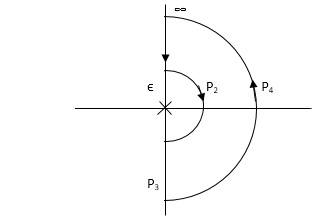
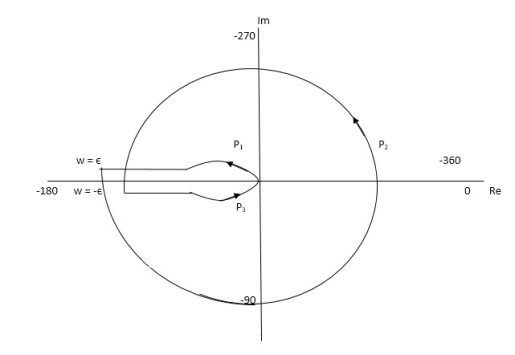
P1 W(∞ Ɛ) where Ɛ 0 P2 S = Ɛejϴ ϴ(+π/2 to 0 to -π/2) P3 W = -Ɛ to -∞ P4 S = Rejϴ, R ∞, ϴ = -π/2 to 0 to +π/2 For P1 M = 1/w.w√102 + w2 = 1/w2√102 + w2 Φ = -1800 – tan-1(w/10) W M Φ ∞ 0 -3 π/2 Ɛ ∞ -1800 Path P3 will be mirror image of P1 about Real axis. G(Ɛ ejϴ) = 1/( Ɛ ejϴ)2(Ɛ ejϴ + 10) Ɛ 0, ϴ = π/2 to 0 to -π/2 = 1/ Ɛ2 e2jϴ(Ɛ ejϴ + 10) = ∞. e-j2ϴ [ -2ϴ = -π to 0 to +π ] Path P2 will be formed by rotating through -π to 0 to +π Path P4 S = Rejϴ R ∞ ϴ = -π/2 to 0 to +π/2 G(Rejϴ) = 1/ (Rejϴ)2(10 + Rejϴ) = 0 N = Z – P No poles on right half of S plane so, P = 0 N = Z – 0 |
|
|
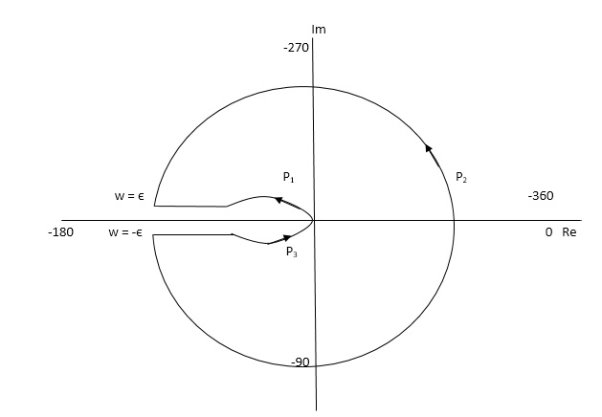
P1 W(∞ to Ɛ) Ɛ 0 P2 S = Ɛejϴ Ɛ 0 ϴ(+π/2 to +π to +3π/2) P3 W(-Ɛ to -∞) Ɛ 0 P4 S = Rejϴ, R ∞, ϴ(3π/2 to 2π to +5π/2) M = 1/W2√102 + W2 , φ = - π – tan-1(W/10) P1 W(∞ to Ɛ) W M φ ∞ 0 -3 π/2 Ɛ ∞ -1800
P3( mirror image of P1) P2 S = Ɛejϴ G(Ɛejϴ) = 1/ Ɛ2e2jϴ(10 + Ɛejϴ) Ɛ 0 G(Ɛejϴ) = 1/ Ɛ2e2jϴ(10) = ∞. e-j2ϴϴ(π/2 to π to 3π/2) -2ϴ = (-π to -2π to -3π) P4 = 0 |
|