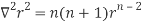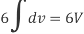Question Bank

UNIT–2

Question-1: If

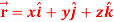
1.

2.

Sol.

Suppose 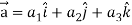  and

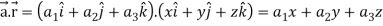
Now taking L.H.S,

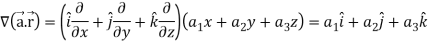
Which is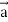

                                                                                               Hence proved.

2.

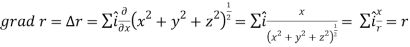
So that

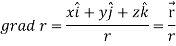
Question-2: If 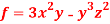then find grad f at the point (1,-2,-1).

Sol.

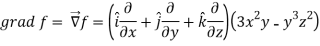
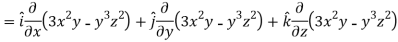
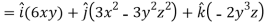
Now grad f at (1 , -2, -1) will be-       

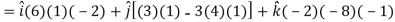
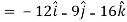
Question-3: If then prove that grad u , grad v and grad w are coplanar.

Sol.

Here-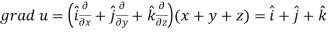                     

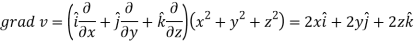
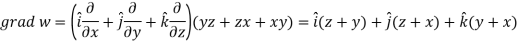
Now-

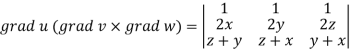
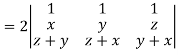
Apply

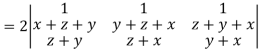
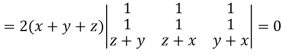
Which becomes zero.

So that we can say that grad u, grad v and grad w are coplanar vectors.

Question-4: Show that-

1.

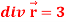
2.

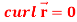
Sol. We know that-

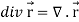
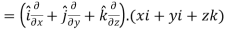
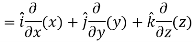
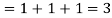
2. We know that-

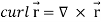
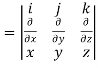
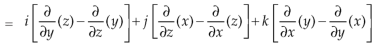
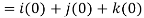= 0

Question-5: If then find the divergence and curl of .

Sol.  we know that-

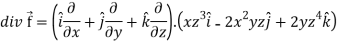
Now-

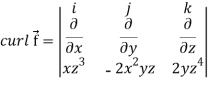
Question-6: Prove that

Note- here is a constant vector and

Sol. Here 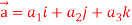   and

So that

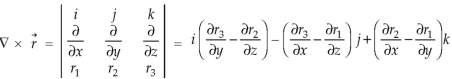
Now-

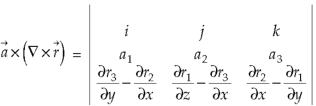
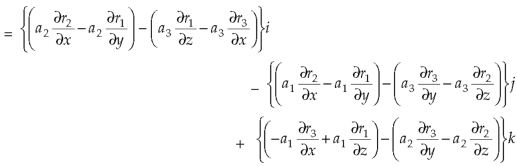
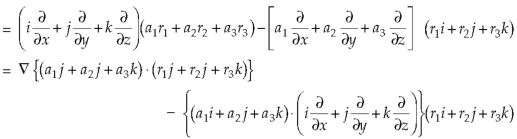
So that-

Question-7: Find the curl of F(x,y,z) = 3i+2zj-xk

Ans.

Curl F =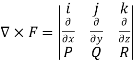

                          =

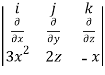
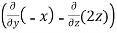                          = i -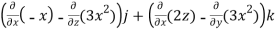

= (0-2)i-(-1-0)j+(0-0)k

= -2i+j

Question-8: Find the curl of F = ()i +4zj +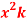

Solution:

Curl F=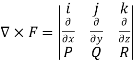

             =

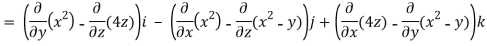
=(0-4)i-(2x-0)j+(0+1)k

=(-4)i – (2x)j+1k

=(-4,-2x,1)

Question-9: Evaluat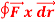e where 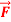= (2xy +z2) I +x2j +3xz2 k along the curve x=t, y=t2, z= t3 from (0,0,0) to (1,1,1).

Solution:  F x dr =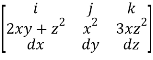

Put x=t, y=t2, z= t3

Dx=dt ,dy=2tdt,                dz=3t2dt.

F x dr =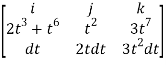

=(3t4-6t8) dti  – ( 6t5+3t8 -3t7) dt j +( 4t4+2t7-t2)dt k

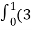=t4-6t3)dti –(6t5+3t8-3t7)dt j+(4t4 + 2t7 – t2)dt k

=

=+

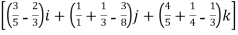
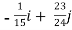Question-10: Evaluate , where S is the surface of the sphere 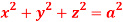in the first octant.

Sol. Here-

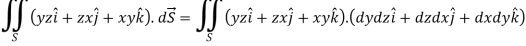
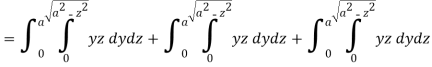
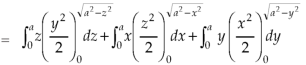
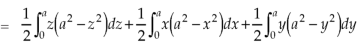
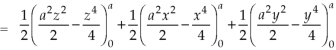
Which becomes-

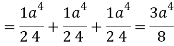
Question-11: Evaluate 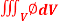, where and V is the closed reason bounded by the planes 4x + 2y + z = 8, x = 0, y = 0, z = 0.

Sol.

Here-   4x + 2y + z = 8

Put y = 0 and z = 0 in this, we get

4x = 8 or x = 2

Limit of x varies from 0 to 2 and y varies from 0 to 4 – 2x

And z varies from 0 to 8 – 4x – 2y

So that-

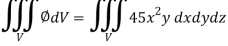
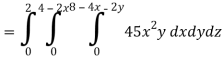
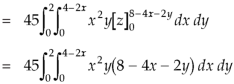
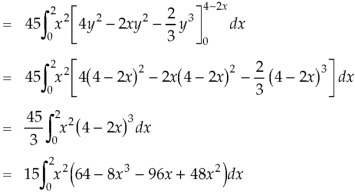
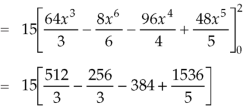
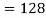
So that-

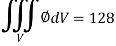
Question-12: Evaluate 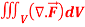if V is the region in the first octant bounded by 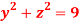and the plane x = 2 and .

Sol.

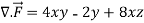
x varies from 0 to 2

The volume will be-

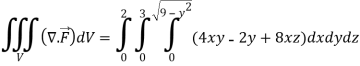
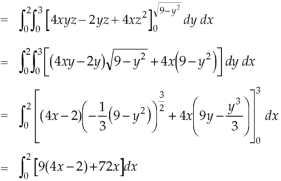
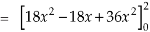
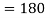
Question-13: Apply Green’s theorem to evaluate where C is the boundary of the area enclosed by the x-axis and the upper half of circle

Sol. We know that by Green’s theorem-

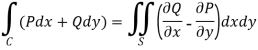
And it it given that-

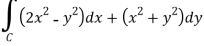
Now comparing the given integral-

P = 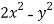  and   Q =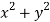

Now-

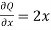and

So that by Green’s theorem, we have the following integral-

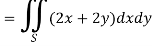
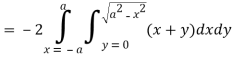
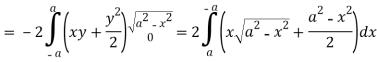
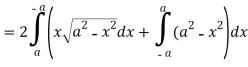
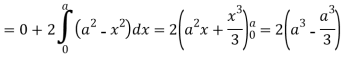
Question-14: Evaluate 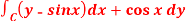by using Green’s theorem, where C is a triangle formed by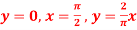

Sol. First we will draw the figure-

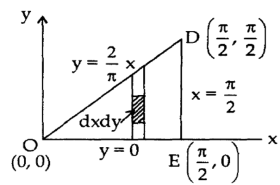
Here the vertices of triangle OED are (0,0), (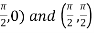

Now by using Green’s theorem-

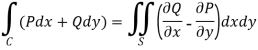
Here P = y – sinx, and Q  =cosx

So that-

and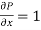

Now-

                              =

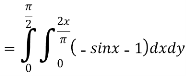
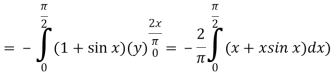
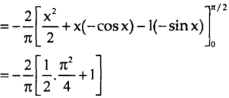
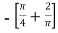
Which is the required answer.

Question-15: Evaluate 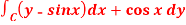by using Green’s theorem, where C is a triangle formed by

Sol. First we will draw the figure-

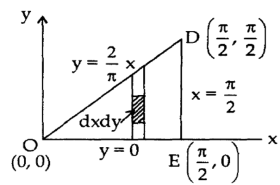
Here the vertices of triangle OED are (0,0), (

Now by using Green’s theorem-

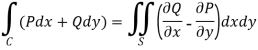
Here P = y – sinx, and Q  =cosx

So that-

and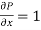

Now-

                              =

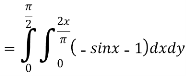
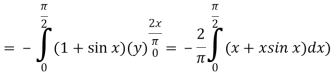
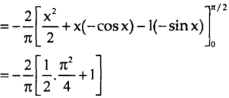
Which is the required answer.

Question-16: Verify stoke’s theorem when 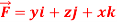and surface S is the part of sphere 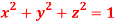, above the xy-plane.

Sol.

We know that by stoke’s theorem,

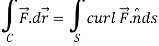
Here C is the unit circle-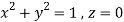

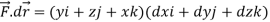
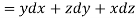
So that-

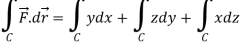
Now again on the unit circle C, z = 0

Dz = 0

Suppose,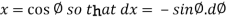 

And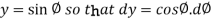           

Now

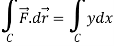
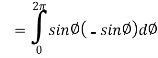
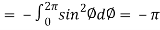  ……………… (1)

Now-

                 Curl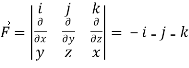

Using spherical polar coordinates-

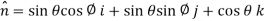
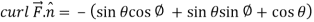
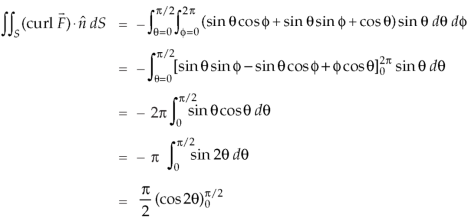
  ………………… (2)

From equation (1) and (2), stoke’s theorem is verified.

Question-17: Verify Stoke’s theorem for the given function-

Where C is the unit circle in the xy-plane.

Sol. Suppose-

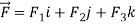
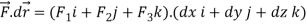
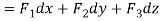
Here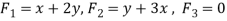

We know that unit circle in xy-plane-

Or

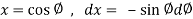
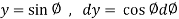
So that,

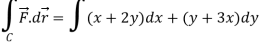
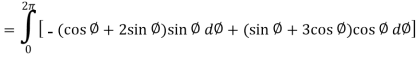
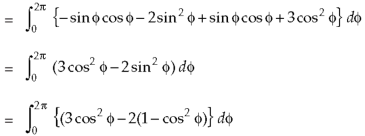
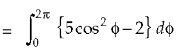
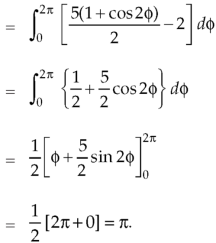
Now

Curl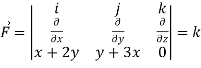

Now,

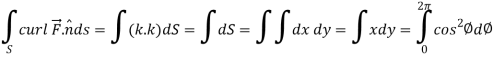
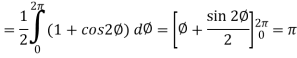
Hence the Stoke’s theorem is verified.

Question-18: Prove the following by using Gauss divergence theorem-

1.

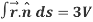
2.

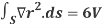
Where S is any closed surface having volume V and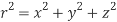

Sol. Here we have by Gauss divergence theorem-

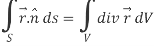
Where V is the volume enclose by the surface S.

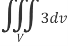
We know that-

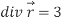
                                             = 3V

2.

Because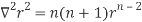 

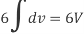
Question Bank

UNIT–2

Question-1: If

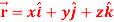
1.

2.

Sol.

Suppose 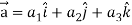  and

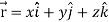
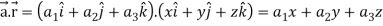
Now taking L.H.S,

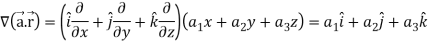
Which is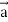

                                                                                               Hence proved.

2.

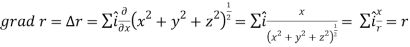
So that

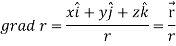
Question-2: If 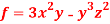then find grad f at the point (1,-2,-1).

Sol.

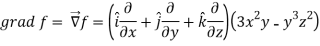
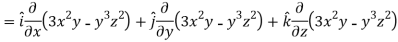
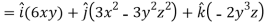
Now grad f at (1 , -2, -1) will be-       

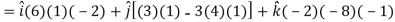
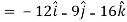
Question-3: If 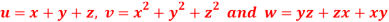then prove that grad u , grad v and grad w are coplanar.

Sol.

Here-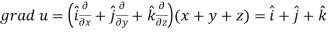                     

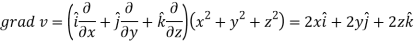
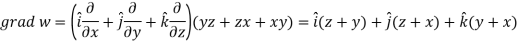
Now-

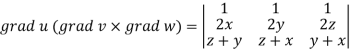
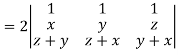
Apply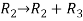

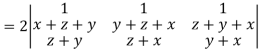
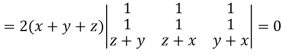
Which becomes zero.

So that we can say that grad u, grad v and grad w are coplanar vectors.

Question-4: Show that-

1.

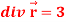
2.

Sol. We know that-

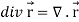
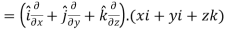
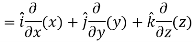
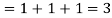
2. We know that-

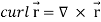
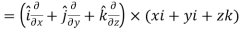
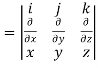
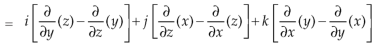
= 0

Question-5: If then find the divergence and curl of .

Sol.  we know that-

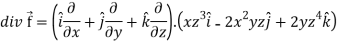
Now-

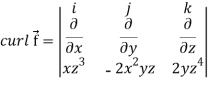
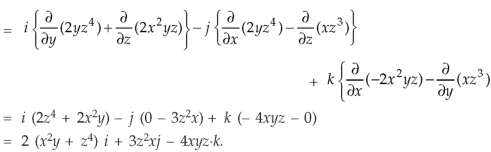
Question-6: Prove that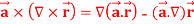

Note- here is a constant vector and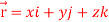

Sol. Here 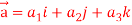   and

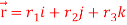
So that

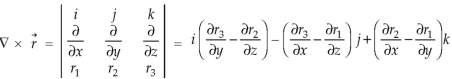
Now-

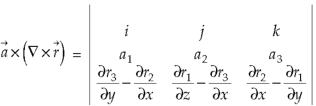
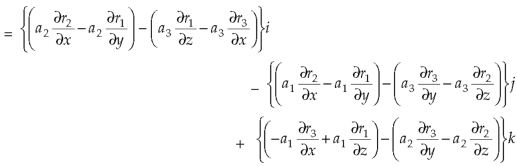
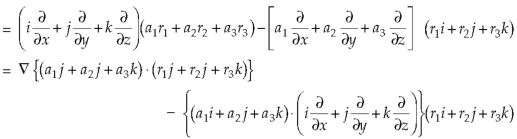
So that-

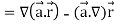
Question-7: Find the curl of F(x,y,z) = 3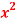i+2zj-xk

Ans.

Curl F =

                          =

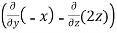                          = i -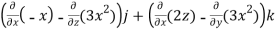

= (0-2)i-(-1-0)j+(0-0)k

= -2i+j

Question-8: Find the curl of F = ()i +4zj +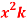

Solution:

Curl F=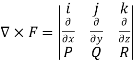

             =

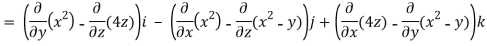
=(0-4)i-(2x-0)j+(0+1)k

=(-4)i – (2x)j+1k

=(-4,-2x,1)

Question-9: Evaluate where 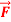= (2xy +z2) I +x2j +3xz2 k along the curve x=t, y=t2, z= t3 from (0,0,0) to (1,1,1).

Solution:  F x dr =

Put x=t, y=t2, z= t3

Dx=dt ,dy=2tdt,                dz=3t2dt.

F x dr =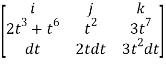

=(3t4-6t8) dti  – ( 6t5+3t8 -3t7) dt j +( 4t4+2t7-t2)dt k

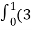=t4-6t3)dti –(6t5+3t8-3t7)dt j+(4t4 + 2t7 – t2)dt k

=

=+

Question-10: Evaluate 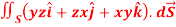, where S is the surface of the sphere 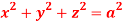in the first octant.

Sol. Here-

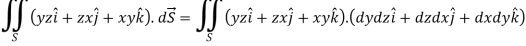
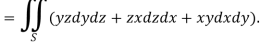
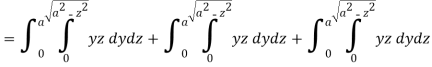
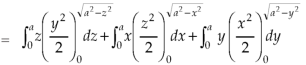
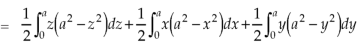
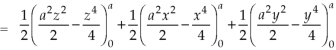
Which becomes-

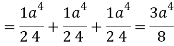
Question-11: Evaluate 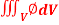, where and V is the closed reason bounded by the planes 4x + 2y + z = 8, x = 0, y = 0, z = 0.

Sol.

Here-   4x + 2y + z = 8

Put y = 0 and z = 0 in this, we get

4x = 8 or x = 2

Limit of x varies from 0 to 2 and y varies from 0 to 4 – 2x

And z varies from 0 to 8 – 4x – 2y

So that-

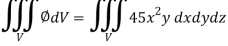
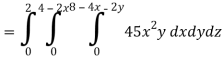
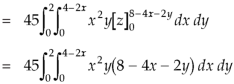
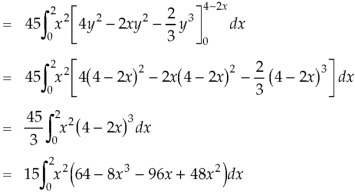
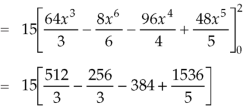
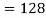
So that-

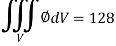
Question-12: Evaluate 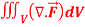if V is the region in the first octant bounded by and the plane x = 2 and .

Sol.

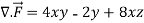
x varies from 0 to 2

The volume will be-

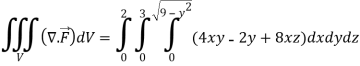
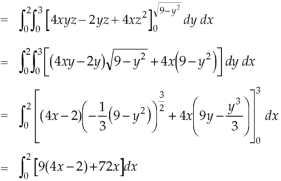
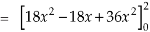
Question-13: Apply Green’s theorem to evaluate 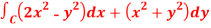where C is the boundary of the area enclosed by the x-axis and the upper half of circle

Sol. We know that by Green’s theorem-

And it it given that-

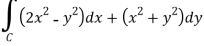
Now comparing the given integral-

P =   and   Q =

Now-

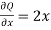and

So that by Green’s theorem, we have the following integral-

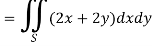
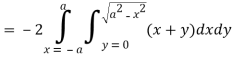
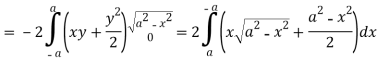
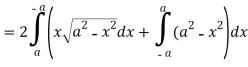
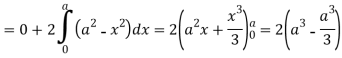
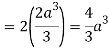
Question-14: Evaluate 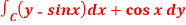by using Green’s theorem, where C is a triangle formed by

Sol. First we will draw the figure-

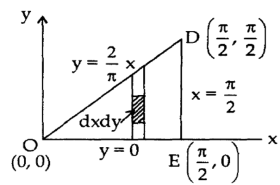
Here the vertices of triangle OED are (0,0), (

Now by using Green’s theorem-

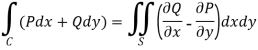
Here P = y – sinx, and Q  =cosx

So that-

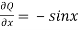and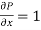

Now-

                              =

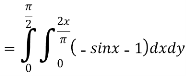
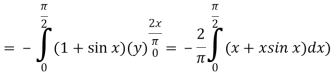
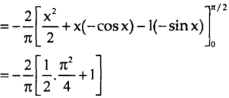
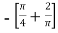
Which is the required answer.

Question-15: Evaluate 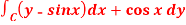by using Green’s theorem, where C is a triangle formed by

Sol. First we will draw the figure-

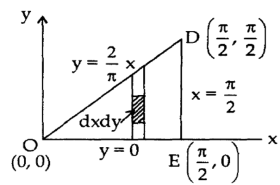
Here the vertices of triangle OED are (0,0), (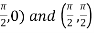

Now by using Green’s theorem-

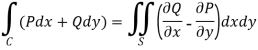
Here P = y – sinx, and Q  =cosx

So that-

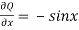and

Now-

                              =

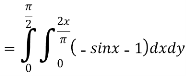
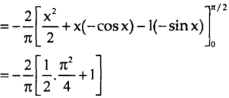
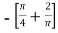
Which is the required answer.

Question-16: Verify stoke’s theorem when 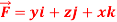and surface S is the part of sphere 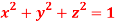, above the xy-plane.

Sol.

We know that by stoke’s theorem,

Here C is the unit circle-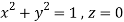

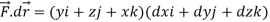
So that-

Now again on the unit circle C, z = 0

Dz = 0

Suppose,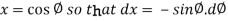 

And           

Now

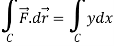
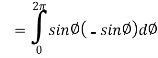
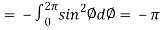  ……………… (1)

Now-

                 Curl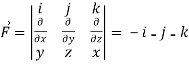

Using spherical polar coordinates-

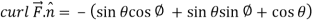
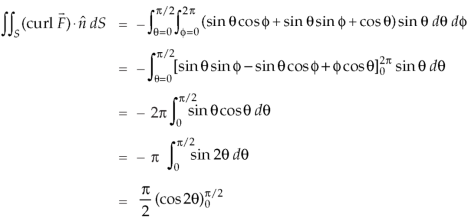
  ………………… (2)

From equation (1) and (2), stoke’s theorem is verified.

Question-17: Verify Stoke’s theorem for the given function-

Where C is the unit circle in the xy-plane.

Sol. Suppose-

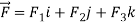
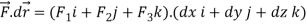
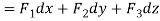
Here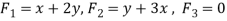

We know that unit circle in xy-plane-

Or

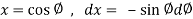
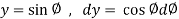
So that,

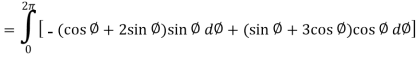
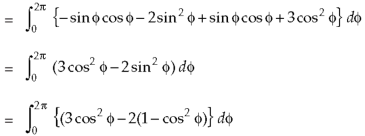
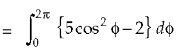
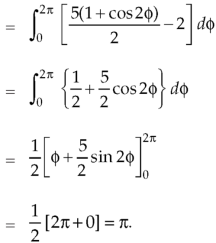
Now

Curl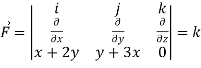

Now,

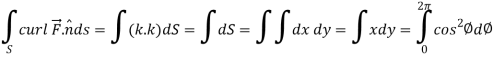
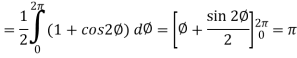
Hence the Stoke’s theorem is verified.

Question-18: Prove the following by using Gauss divergence theorem-

1.

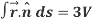
2.

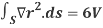
Where S is any closed surface having volume V and

Sol. Here we have by Gauss divergence theorem-

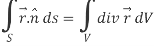
Where V is the volume enclose by the surface S.

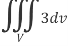
We know that-

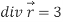
                                             = 3V

2.

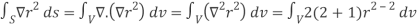
Because