Unit 3
Valuation of Annuities
Q1) Define Types of Annuities.
A1)
Annuities are divided into several categories based on the frequency and form of payments they make. Annuities, for example, may have their cash flows charged at various times. Payments can be made once a week, twice a week, or once a month. The following are the most common forms of annuities:
Fixed annuities
Fixed-payment annuities are a form of annuity. Although the payments are assured, the rate of return is typically low.
Variable annuities
Annuities that allow a person to choose from a variety of investments and receive a monthly income based on the performance of those investments. Variable annuities do not guarantee a certain amount of money, but they provide a higher rate of return than fixed annuities.
Life annuities
Life annuities guarantee a set amount of money to their owners before they die.
Perpetuity
An annuity that pays out cash flows indefinitely with no end date. Financial instruments that provide the holder with everlasting cash flows are exceedingly rare.
CONSOL, a UK government bond, is the most well-known example. Consols were first published in the mid-eighteenth century. The bonds had no set end date and could be redeemed at the discretion of the Parliament. The UK government, on the other hand, redeemed all consols in 2015.
Q2) What are the steps that are used to calculate the formula for a deferred annuity using an ordinary annuity.
A2)
● Step 1: To begin, figure out how much the annuity payment will be and whether it will be made at the end of each period. The symbol for it is P Ordinary.
● Step 2: Next, divide the annualised rate of interest by the amount of periodic payments in a year to get the effective rate of interest, which is denoted by r. r = Annualized rate of interest / No. periodic payments in a year
● Step 3: Next, find the total number of periods, which is the product of the number of years and the number of periodic payments in a year, and is denoted by n. n = Number of years * Number of periodic payments in a year
Step 4: Next, calculate the payment deferment duration, which is denoted by t.
Step 5: Finally, as shown below, the deferred annuity can be calculated using ordinary annuity payments (step 1), an acceptable rate of interest rate (step 2), a number of payment intervals (step 3), and deferred periods (step 4).

Q3) Consider the case of John, who received a deal to lend $60,000 today in exchange for twenty-five annual payments of $6,000 each. The annuity will begin five years from now, with a 6-percent effective rate of interest. If the payout is an ordinary annuity with annuity due, determine if the agreement is feasible for John.
A3)
● Given, P Ordinary = $6,000,000
● r = 6%
● n = 25 years
● t = 5 years
Deferred Annuity Calculation If the payment is Ordinary Due, the deferred annuity can be computed as follows:
Annuity postponed
$6,000*[1 – (1 + 6%)-25] / [(1 + 6%)5* 6%]
Deferred Annuity = $57,314.80 ~ $57,315
Deferred Annuity will be –
John does not lend the money in this situation since the deferred annuity is worth less than $60,000.
Calculation of Deferred Annuity if payment is Annuity Due
● Given, P Due = $6,000,000
● r = 6%
● n = 25 years
● t = 5 years
As a result, the deferred annuity can be determined using the formula:
Deferred Annuity = $6,000*[1 – (1 + 6%)-25] / [(1 + 6%)5-1 * 6%]
Deferred Annuity = $60,753.69 ~ $60,754
John can lend the money in this situation because the deferred annuity is worth more than $60,000.
Q4) Define future value of annuity.
A4)
An annuity's future value is essentially the amount of each payment's future value. The number of the geometric series is the equation for the future value of an annuity due:
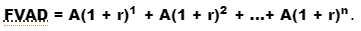
The number of the geometric series is the equation for the future value of an ordinary annuity:
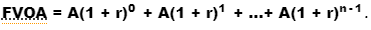
Without going into detail, just remember that the future value of an annuity is the number of the geometric sequences shown above, and that these quantities can be simplified using the formulas below: where A denotes the annuity payment or periodic rent, r denotes the interest rate per year, and n denotes the number of periods.
The future value of an ordinary annuity (FVOA) is calculated as follows:
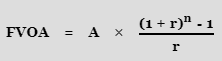
And the future value of an annuity due (FVAD) is:
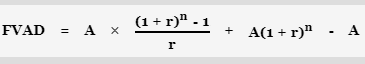
Note that the difference between FVAD and FVOA is:
FVAD = 0 + A(1 + r)1 + A(1 + r)2 + ...+ A(1 + r)n-1+ A(1 + r)n.
FVOA = A(1 + r)0 + A(1 + r)1 + A(1 + r)2 +... + A(1 + r)n-1 + 0.
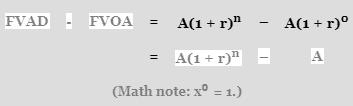
To put it another way, the difference is simply the interest received during the previous compounding era. The last payment of an ordinary annuity receives no interest since it is made at the end of the term, while the last payment of an annuity due earns interest during the last compounding period.
Q5) Define present value of annuity.
A5)
The sum of the present values of each annuity payout is the present value of an annuity (PVA). The present value of an annuity is the amount of the present values of each of those instalments, since the present value of a lump sum payment is essentially the future value of that payment divided by the interest factor (1 + r)n:
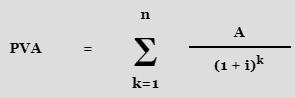
The sum of this geometric progression can be simplified to:

Q6) Define Valution of annuities .
A6)
The present value of annuities is determined by discounting potential cash flows and calculating the present value of the cash flows. The following is a general method for calculating the value of an annuity:

Where:
● PV = Present value of the annuity
● P = Fixed payment
● r = Interest rate
● n = Total number of periods of annuity payments
Perpetuity valuation differs from other valuations in that it does not have a set end date. As a result, the value of the perpetuity is calculated using the formula:
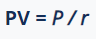
Q7) What is Present value of deferred annuity? Derive its formula.
A7)
The deferred annuity formula is used to calculate the present value of a deferred annuity that is promised to be paid after a certain period of time. It is calculated by estimating the present value of a future payout by taking the rate of interest and time into account.
The word "deferred annuity" applies to a postponed annuity in the form of instalment or lump-sum payments rather than an immediate stream of profits. It's simply the current value of an annuity payout in the future. Ordinary annuity payment, effective rate of interest, number of payment terms, and deferred periods are all included in the formula for a deferred annuity based on an ordinary annuity (where the annuity payment is made at the end of each period).
The following is a representation of a Deferred Annuity based on an ordinary annuity:

where,
● P Ordinary = Ordinary annuity payment
● r = Effective rate of interest
● n = No. of periods
● t = Deferred periods
Annuity payment due, effective rate of interest, amount of payment intervals, and deferred periods are all included in the formula for a deferred annuity based on annuity due (where the annuity payment is made at the start of each period).
The following is a representation of a Deferred Annuity dependent on annuity due:

● P Due = Annuity payment due
● r = Effective rate of interest
● n = No. of periods
● t = Deferred periods
Deferred Annuity Calculation (Step by Step)