Unit 4

Analysis of Annuity

Q1) What Is an Annuity?

A1)

An annuity is a financial product that provides a fixed stream of payments to a person, and it is mainly used by retirees as a source of income. Annuities are contracts that financial institutions grant and distribute (or sell) to invest funds from individuals. They assist people in dealing with the possibility of outliving their savings. The holding institution would release a stream of payments at a later date after annuitization.

The accumulation process refers to the period between when an annuity is purchased and when pay-outs begin. The contract enters the annuitization process once payments begin.

Q2) Give a simple example of an Annuity.

A2)

A life insurance policy is an example of a fixed annuity, in which a person pays a set amount per month for a set period of time (typically 59.5 years) and earns a set income stream during their retirement years.

An immediate annuity is one in which a person pays a single premium to an insurance provider, say $200,000, and then collects monthly payments, say $5,000, for a set period of time. The size of an immediate annuity payment is determined by market conditions and interest rates.

Q3) How is EMI calculated?

A3)

The mathematical formula to calculate EMI is: EMI = P × r × (1 + r)n/((1 + r)n - 1) where P= Loan amount, r= interest rate, n=tenure in number of months.
For instance, the EMI for a principal amount for Rs 1 lakh, 10% interest rate and 12 months tenure is shown in the following table:

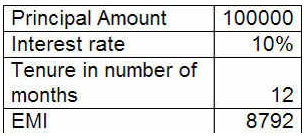
The EMI payments are directly proportional to the loan volume and interest rates, and inversely proportional to the loan tenure, when the three governing variables are taken into account. The EMI payments increase in proportion to the loan volume or interest rate, and vice versa. While the total amount of interest to be charged increases as the loan tenure lengthens, the EMI payments decrease as the loan term lengthens.

Q4) What are the factors that impact EMI?

A4)

If the bank charges a high EMI, the borrower will have trouble repaying his debt. As a result, banks only lend a certain amount to ensure that home loan repayments do not exceed 40% of a borrower's monthly income. The principal sum, rate of interest, and loan tenure all affect a borrower's EMI outflow.

 
When the loan amount is high, the EMI repayments are also large. On the opposite, borrowing less means less EMI obligations. If interest rates do not change, EMIs will remain constant for the entire loan repayment period. EMIs on floating rate loans, on the other hand, rise as the interest rate rises and fall when the interest rate falls. Short-term home loans are normally from 5-8 years in duration. The higher the EMI due each month, the shorter the loan term. If the loan is for a limited period of time, the borrower would be debt-free earlier. Loan terms will range from 25 to 30 years. In the case of a longer term, a borrower's monthly EMI outflow decreases dramatically.

Q5) What are the Guidelines for auditors in Audit of Outstanding Liabilities?

A5)

The auditor must consider the following when reviewing outstanding liabilities:

1. The unpaid expenses must be checked using supporting documentation, such as documents, correspondence, and so on.

2. The profits earned in advance must be checked using counterfoils such as receipts, correspondence, and so on.

3. The auditor may be able to detect over-provision, under-provision, or non-provision of outstanding liabilities by comparing outstanding liabilities from the previous year to the current year under audi.

4. The auditor should verify randomly the payments made with regard to outstanding liabilities in the subsequent accounting year.

5. The auditor should verify the calculation of expenses outstanding and ensure that the amount is correctly arrived at (e.g., interest payable, commission payable etc). If the amount outstanding is to be estimated, the auditor should ensure that a reasonable method is applied to arrive at the estimation.

Q6) What are the Advantages of sinking funds?

A6)

Advantages of sinking funds are

Brings in investors

Investors are very well aware that companies or organizations with a large amount of debt are potentially risky. However, once they know that there is an established sinking fund, they will see a certain level of protection for them so that in the case of a default or bankruptcy, they will still be able to get their investment back.

The possibility of lower interest rates

Investors would be unable to invest in a business with a low credit rating unless it offers higher interest rates. A sinking fund provides investors with an alternative form of insurance, allowing businesses to offer lower interest rates.

Stable finances

The financial position of a company is not always clear, and some financial problems may cause it to lose its footing. A sinking fund, on the other hand, ensures that a company's ability to repay loans and buy back bonds is not jeopardised. As a result, you'll have a decent credit rating and customers who are trusting in you.

Few examples

Consider a 7-Eleven franchisee who issues $50,000 in bonds with a sinking fund clause and maintains a sinking fund into which the franchisee deposits $500 on a regular basis with the intention of using it to gradually buy back bonds until they mature.

If the stock price drops, he can buy back the bonds at a reduced price, or at face value, if the market price rises. Depending on how much was bought back, the principal sum owed would eventually be lower. It's important to keep in mind, though, that the number of bonds that can be bought back before the maturity date is restricted.

Another example will be a company offering $1 million in bonds with a 10-year maturity. As a result, it establishes a sinking fund and invests $100,000 per year to ensure that all bonds are purchased by the maturity date.

Q7) Mr. A paid the Rs 25,000 annual premium on a quarterly basis, and the sum assured is Rs 5 lakh for a policy term of 20 years. If Mr. A stop paying after three years, that is, have paid 12 premiums, the paid-up value will be Rs 5,00,000X (12/80).

A7)

In this case, 80 (20X4) is the number of premiums you were supposed to pay and 12 (3X4) is the number of premiums you have actually paid.

The paid-up value is Rs 75,000. This is the sum you will get at maturity or your nominee will get after you die. Paid-up value plus bonus is the total paid-up value. Mr. A has accumulated bonus of Rs. 60,000 as bonus.

Total Premium payment terms= 20*4=80

Total Premium paid=3*4= 12

Total Paid Up Value= Total Premium Paid + Bonus Accumulated=Rs. 75000+ Rs. 60000=Rs. 135000

Surrender Value factor= 27.76%

Special Cash/Surrender Value= {500000*12/80+60000)} Surrender Value Factor=135000*27.76%= Rs. 37,260/-

Q8) What is Intrinsic Value?

A8)

A security's market value is the price at which it is sold in the market, and it is usually close to its intrinsic value. When it comes to the relationship between intrinsic value and market price, there are many schools of thought. Market prices are those that govern the market as a result of supply and demand powers. The intrinsic price of a stock is its true value, which is determined by its earning ability and true worth. The value of a security must be equal to the discounted value of the potential income stream, according to the fundamentalist approach to security valuation. If the stock price is below this value, the investor purchases the securities.

Thus, for fundamentalists, earnings and dividends are the essential ingredients in determining the market value of a security. The discount rate used in such present value calculations is known as the required rate or return. Using this discount rate all future earnings are discounted back to the present to determine the intrinsic value.

The price of a security, according to the technical school, is determined by market demand and supply and has very little to do with intrinsic values. For different periods of time, market fluctuations follow those patterns. The predictable shifts in demand and supply are represented by changes in trend. According to this school, current trends are a byproduct of the past, and history repeats itself.

Market prices are good substitutes for intrinsic values in a reasonably broad defence market where competitive conditions prevail, according to the efficient market hypothesis. After taking into account all of the information available to market participants, security prices are calculated. A share is thus generally worth whatever it is selling for in the market.

Generally, fundamental school is the basis for security valuation and many models are in use, based on these tenets.