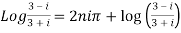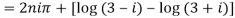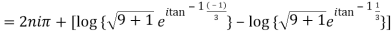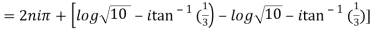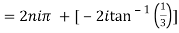Unit 2
Unit 2
Hyperbolic function and Logarithm of complex numbers
Question and answer
- Simplify

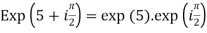
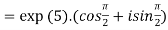
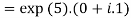
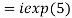
2. Find the value of 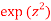

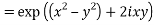
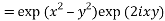
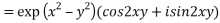
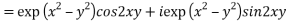
3. Expand the function 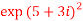
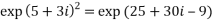
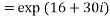
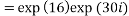
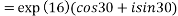
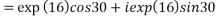
4. Find out the value of 
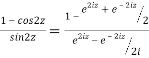
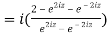
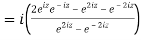
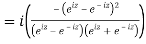
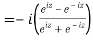
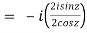
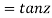
5. Prove that 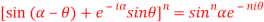
LHS 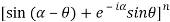


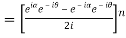

= 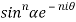
6. Solve 
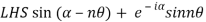
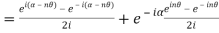
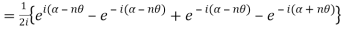

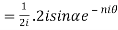
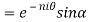
7. If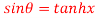 then prove that
then prove that 
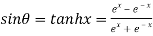 ……(i)
……(i)
Squaring both sides
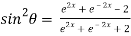
Now, 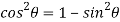
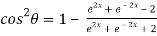
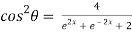

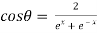 ……(ii)
……(ii)
Dividing (i) by (ii)
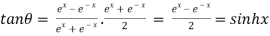
8. Prove that 
Let 
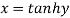
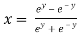
Squaring on both sides
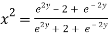
Again 
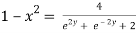
Taking square root on both side

Now, 
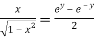

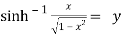
Hence 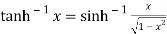
9. Separate the real and imaginary part of 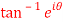
Let 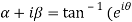 )….(1)
)….(1)
 …..(2)
…..(2)
On adding (1) and (2) we get

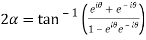
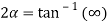
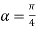
Subtracting (1) and(2) we get

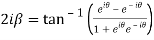
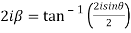
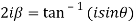


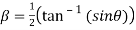
Which are the required real and imaginary parts.
10. Find the general value of 
The general value is

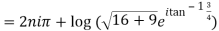

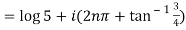
11. Separate the real and imaginary part of 
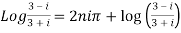

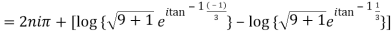
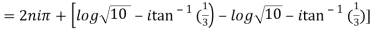
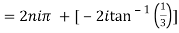
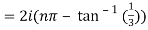
Unit 2
Hyperbolic function and Logarithm of complex numbers
Question and answer
- Simplify

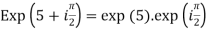


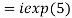
2. Find the value of 
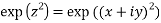


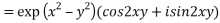

3. Expand the function 
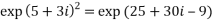
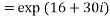
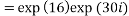
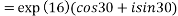
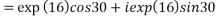
4. Find out the value of 
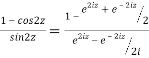
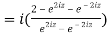
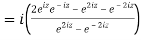
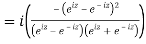
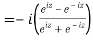
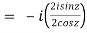
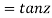
5. Prove that 
LHS 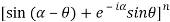
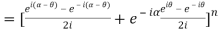

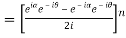
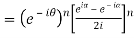
= 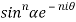
6. Solve 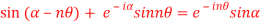
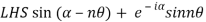

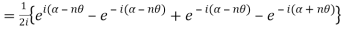

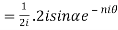
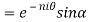
7. If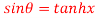 then prove that
then prove that 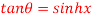
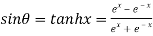 ……(i)
……(i)
Squaring both sides

Now, 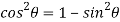
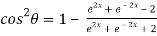
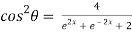
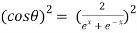
 ……(ii)
……(ii)
Dividing (i) by (ii)
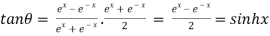
8. Prove that 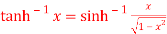
Let 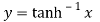

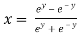
Squaring on both sides
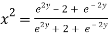
Again 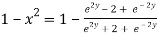
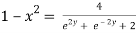
Taking square root on both side

Now, 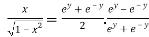
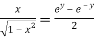

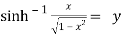
Hence 
9. Separate the real and imaginary part of 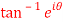
Let  )….(1)
)….(1)
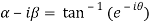 …..(2)
…..(2)
On adding (1) and (2) we get
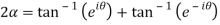

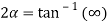

Subtracting (1) and(2) we get

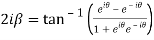

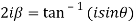

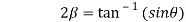
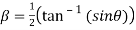
Which are the required real and imaginary parts.
10. Find the general value of 
The general value is
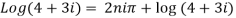


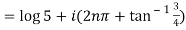
11. Separate the real and imaginary part of