Question Bank
Unit 5
Q.1 Explain energy density in capacitor?
Answer:
The amount of energy that can be stored in a capacitor per mass of that capacitor is called its specific energy. Specific energy is measured gravimetrically (per unit of mass) in watt-hours per kilogram (Wh/kg).
The amount of energy can be stored in a capacitor per volume of that capacitor is called its energy density. Energy density is measured volumetrically (per unit of volume) in watt-hours per litre (Wh/l).
As of 2013 commercial specific energies range from around 0.5 to 15 Wh/kg. For comparison, an aluminium electrolytic capacitor stores typically 0.01 to 0.3 Wh/kg, while a conventional lead-acid battery stores typically 30 to 40 Wh/kg and modern lithium-ion batteries 100 to 265 Wh/kg. Supercapacitors can therefore store 10 to 100 times more energy than electrolytic capacitors, but only one tenth as much as batteries. For reference, petrol fuel has a specific energy of 44.4 MJ/kg or 12300 Wh/kg (in vehicle propulsion, the efficiency of energy conversions should be considered resulting in 3700 Wh/kg considering a typical 30% internal combustion engine efficiency).
Commercial energy density (also called volumetric specific energy in some literature) varies widely, but in general range from around 5 to 8 Wh/l. Units of litres and dm3 can be used interchangeably. In comparison, petrol fuel has an energy density of 32.4 MJ/l or 9000 Wh/l
Q.2 Deference between battery and capacitor?
Answer:
The batteries are widely used with a specific volume and weight, also have better energy density. Supercapacitors are high-capacity capacitors with high power density. When compared to a battery, the supercapacitor has a fast charging-discharging capacity, can handle low-high temperature, high reliability, and low impedance.
The cost of the battery is low whereas the cost of a supercapacitor is high. Supercapacitors have the self-discharging capacity. In the battery, the operating voltage determines the charging and discharging modes. In a supercapacitor, the allowable voltage depends on the type of dielectric material used between the plates. And also, the electrolyte in the capacitor may increase the capacitance.
Batteries are available in lead-acid batteries, Ni-MH, Li-Po, Li-ion, LMP, etc. Supercapacitors are available with organic electrolyte, aqueous electrolyte, ionic liquid, hybrid, and pseudo supercapacitors. Batteries are used to store large amounts of energy and supercapacitors are used to deliver high power density
Q.3 Calculate the energy stored in the capacitor network when the capacitors are fully charged and when the capacitances are C1=12.0μF, C2=2.0μFC1=12.0μF,C2=2.0μF, and C3=4.0μFC3=4.0μF, respectively.
Answer:
We identify C1=12.0μand V1=4.0V, C2=2.0μF and V1=4.0V, and V2=8.0V,C3=4.0μF and V3=8.0VV3=8.0V. The energies stored in these capacitors are
U1=12C1V21=12(12.0μF) (4.0V)2=96μJ
U2=12C2V22=12(2.0μF) (8.0V)2=64μJ
U3=12C3V23=12(4.0μF) (8.0V)2=130μJ,
The total energy stored in this network is
UC=U1+U2+U3=96μJ+64μJ+130μJ=0.29mJ
Q.4 Define super capacitor?
Answer:
A superconductor is a material that can conduct electricity or transport electrons from one atom to another with no resistance. This means no heat, sound or any other form of energy would be released from the material when it has reached "critical temperature" (Tc), or the temperature at which the material becomes superconductive. Unfortunately, most materials must be in an extremely low energy state (very cold) in order to become superconductive.
Research is underway to develop compounds that become superconductive at higher temperatures. Currently, an excessive amount of energy must be used in the cooling process making superconductors inefficient and uneconomical.
Superconductors come in two different flavours: type I and type II.
Q.5 Explain critical magnetic field in super conductor?
Answer:
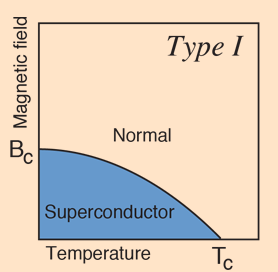
The superconducting state cannot exist in the presence of a magnetic field greater than a critical value, even at absolute zero. This critical magnetic field is strongly correlated with the critical temperature for the superconductor, which is in turn correlated with the bandgap. Type II superconductors show two critical magnetic field values, one at the onset of a mixed superconducting and normal state and one where superconductivity ceases.

Q.6 what do you mean by type 1 and type II super conductor?
Answer:
Type I Superconductors
A type I superconductor consists of basic conductive elements that are used in everything from electrical wiring to computer microchips. At present, type I superconductors have Tcs between 0.000325 °K and 7.8 °K at standard pressure. Some type I superconductors require incredible amounts of pressure in order to reach the superconductive state. One such material is sulphur which, requires a pressure of 9.3 million atmospheres (9.4 x 1011 N/m2) and a temperature of 17 °K to reach superconductivity. Some other examples of type I superconductors include Mercury - 4.15 °K, Lead - 7.2 °K, Aluminium - 1.175 °K and Zinc - 0.85 °K. Roughly half of the elements in the periodic table are known to be superconductive.
Type II Superconductors
A type II superconductor is composed of metallic compounds such as copper or lead. They reach a superconductive state at much higher temperatures when compared to type I superconductors. The cause of this dramatic increase in temperature is not fully understood. The highest Tc reached at standard pressure, to date, is 135 °K or -138 °C by a compound (HgBa2Ca2Cu3O8) that falls into a group of superconductors known as cuprite perovskites. This group of superconductors generally has a ratio of 2 copper atoms to 3 oxygen atoms, and is considered to be a ceramic. Type II superconductors can also be penetrated by a magnetic field whereas a type I can not
Q.7 give brief description of Meissner effect?
Answer:
When a material makes the transition from the normal to superconducting state, it actively excludes magnetic fields from its interior; this is called the Meissner effect.
This constraint to zero magnetic field inside a superconductor is distinct from the perfect diamagnetism which would arise from its zero electrical resistance. Zero resistance would imply that if you tried to magnetize a superconductor, current loops would be generated to exactly cancel the imposed field (Lenz's law). But if the material already had a steady magnetic field through it when it was cooled trough the superconducting transition, the magnetic field would be expected to remain. If there were no change in the applied magnetic field, there would be no generated voltage (Faraday's law) to drive currents, even in a perfect conductor. Hence the active exclusion of magnetic field must be considered to be an effect distinct from just zero resistance. A mixed state Meissner effect occurs with Type II materials.
One of the theoretical explanations of the Meissner effect comes from the London equation. It shows that the magnetic field decays exponentially inside the superconductor over a distance of 20-40 nm. It is described in terms of a parameter called the London penetration depth
Q.8 what is the principal of super capacitor?
Answer:
In an ordinary capacitor, the plates are separated by a relatively thick dielectric made from something like mica (a ceramic), a thin plastic film, or even simply air (in something like a capacitor that acts as the tuning dial inside a radio). When the capacitor is charged, positive charges form on one plate and negative charges on the other, creating an electric field between them. The field polarizes the dielectric, so its molecules line up in the opposite direction to the field and reduce its strength. That means the plates can store more charge at a given voltage
Q.9 what is mean by Mixed-State Meissner Effect?
Answer:
In Type II superconductors the magnetic field is not excluded completely, but is constrained in filaments within the material. These filaments are in the normal state, surrounded by supercurrents in what is called a vortex state. Such materials can be subjected to much higher external magnetic fields and remain superconducting.
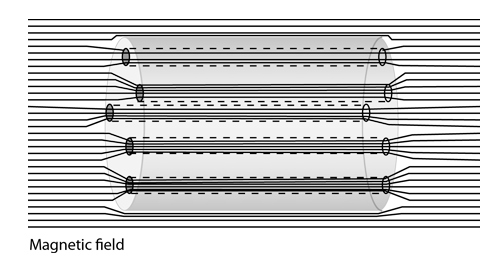
Q. 10 what is perfect diamagnetism in super conductor?
Answer:
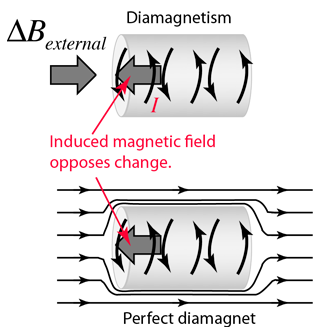
A conductor will oppose any change in externally applied magnetic field. Circulating currents will be induced to oppose the build-up of magnetic field in the conductor (Lenz's law). In a solid material, this is called diamagnetism, and a perfect conductor would be a perfect diamagnet. That is, induced currents in it would meet no resistance, so they would persist in whatever magnitude necessary to perfectly cancel the external field change. A superconductor is a perfect diamagnetic, but there is more than this involved in the Meissner effect
 |