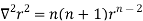Unit - 4
Line integrals
Q1) Define line integral.
A1)
Let- F be vector function defined throughout some region of space and let C be any curve in that region. ṝ is the position vector of a point p (x,y,z) on C then the integral ƪ F .dṝ is called the line integral of F taken over
Now, since ṝ =xi+yi+zk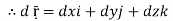
And if F͞ =F1i + F2 j+ F3 k

Q2) Evaluate 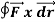 where
where 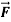 = (2xy +z2) I +x2j +3xz2 k along the curve x=t, y=t2, z= t3 from (0,0,0) to (1,1,1).
= (2xy +z2) I +x2j +3xz2 k along the curve x=t, y=t2, z= t3 from (0,0,0) to (1,1,1).
A2)
F x dr = 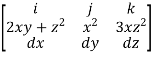
Put x=t, y=t2, z= t3
Dx=dt ,dy=2tdt, dz=3t2dt.
F x dr = 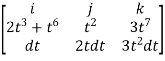
=(3t4-6t8) dti – ( 6t5+3t8 -3t7) dt j +( 4t4+2t7-t2)dt k
= t4-6t3)dti –(6t5+3t8-3t7)dt j+(4t4 + 2t7 – t2)dt k
t4-6t3)dti –(6t5+3t8-3t7)dt j+(4t4 + 2t7 – t2)dt k
=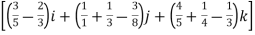
= +
+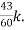
Q3) Prove that ͞͞͞F = [y2cos x +z3] i+(2y sin x – 4) j +(3xz2 + 2) k is a conservative field. Find (i) scalar potential for͞͞͞ F (ii) the work done in moving an object in this field from (0, 1, -1) to (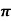 / 2,-1, 2)
/ 2,-1, 2)
A3)
(a) The field is conservative if cur͞͞͞͞͞͞F = 0.
 Now, curl͞͞͞F =
Now, curl͞͞͞F = 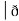 ̷̷
̷̷ X
X  /
/  y
y  /
/  z
z
Y2COS X +Z3 2y sin x-4 3xz2 + 2
; Cur 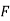 = (0-0) – (3z2 – 3z2) j + (2y cos x- 2y cos x) k = 0
= (0-0) – (3z2 – 3z2) j + (2y cos x- 2y cos x) k = 0
; F is conservative.
(b) Since F is conservative there exists a scalar potential ȸ such that
F = ȸ
 (y2cos x=z3) i + (2y sin x-4) j + (3xz2 + 2) k =
(y2cos x=z3) i + (2y sin x-4) j + (3xz2 + 2) k =  i +
i + 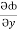 j +
j + 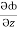 k
k
 = y2cos x + z3,
= y2cos x + z3,  = 2y sin x – 4,
= 2y sin x – 4,  = 3xz2 + 2
= 3xz2 + 2
Now,  =
=  dx +
dx + 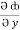 dy +
dy + 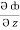 dz
dz
= (y2cos x + z3) dx +(2y sin x – 4)dy + (3xz2 + 2)dz
= (y2cos x dx + 2y sin x dy) +(z3dx +3xz2dz) +(- 4 dy) + (2 dz)
=d(y2 sin x + z3x – 4y -2z)
 ȸ = y2 sin x +z3x – 4y -2z
ȸ = y2 sin x +z3x – 4y -2z
(c) now, work done =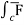 .d ͞r
.d ͞r
= 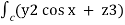 dx + (2y sin x – 4) dy + ( 3xz2 + 2) dz
dx + (2y sin x – 4) dy + ( 3xz2 + 2) dz
= 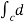 (y2 sin x + z3x – 4y + 2z) (as shown above)
(y2 sin x + z3x – 4y + 2z) (as shown above)
= [ y2 sin x + z3x – 4y + 2z ](  /2, -1, 2)
/2, -1, 2)
= [ 1 +8  + 4 + 4 ] – { - 4 – 2} =4
+ 4 + 4 ] – { - 4 – 2} =4 + 15
+ 15
Q4) What is exact differentia test?
A4)
Exact differential test
For  =F1
=F1 + F2
+ F2  + F3
+ F3  , the necessary and sufficient condition that
, the necessary and sufficient condition that
F1 + F2 dy+ F3
+ F2 dy+ F3  ,
,
Be an exact differential is that F must be conservative.
Area of a rectangular region bounded by the curve c
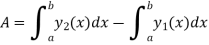

Similarly
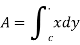

Q5) State and prove fundamental theorem for line integrals
A5)
Statement- Suppose that C is a smooth curve given by 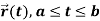 . Also suppose that f is a function whose gradient vector,
. Also suppose that f is a function whose gradient vector, 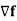 is continuous on C, then
is continuous on C, then
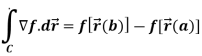
Proof:
The line integral,

We use the chain rule to simplify the integral,

To finish this off, we use the fundamental theorem of calculus for single integral,
We get
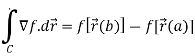
Q6) Evaluate 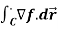 where f(x, y, z) =
where f(x, y, z) = 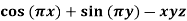 and C is any path that starts at (1, ½, 2) and ends at (2, 1, -1).
and C is any path that starts at (1, ½, 2) and ends at (2, 1, -1).
A6)
Let 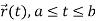 be any path that starts at (1, ½, 2) and ends at (2, 1, -1). Then,
be any path that starts at (1, ½, 2) and ends at (2, 1, -1). Then,

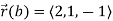
The integral is then,
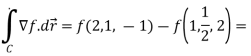

Q7) What do you understand by conservative vector field and independence of path?
A7)
Conservative vector fields-
If 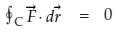 then the field is said to be conservative which means no work is done in displacement from a point a to another point in the field and back to a and the mechanical energy is conserved.
then the field is said to be conservative which means no work is done in displacement from a point a to another point in the field and back to a and the mechanical energy is conserved.
Note- every irrotational field is conservative.
Independence of path-
If in a conservative field 

Along any closed curve C.
Which is the condition of the independence of path.
Note- if 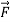 is a conservative vector field then
is a conservative vector field then 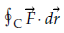 is independent of path.
is independent of path.
Q8) Check whether 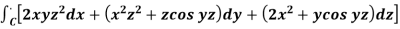 is independent of path C.
is independent of path C.
A8)
The line integral of F is independent of path of integration if 
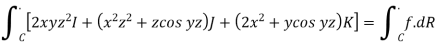
And
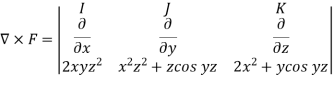

Hence the given integral is independent of path C,
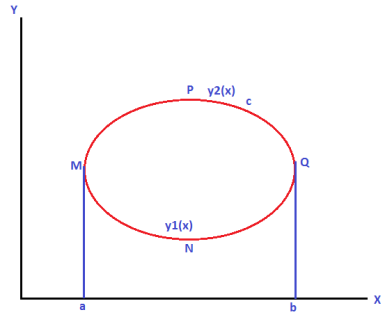
Q9) State and prove green’s theorem
A9)
Statement- If C be a regular closed curve in the xy-plane and S is the region bounded by C then,

Where P and Q are the continuously differentiable functions inside and on C.
Proof:
Let the equation of the curves AEB and AFB are y = f1(x) and y = f2 (x) respectively.
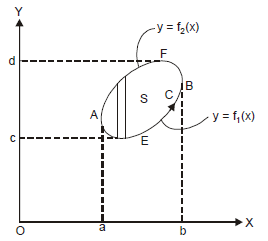
Consider
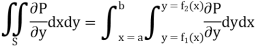
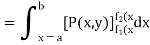
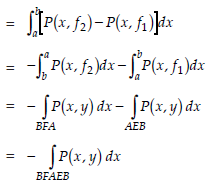
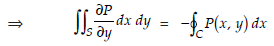 ….(1)
….(1)
Similarly, let the equations of the curve EAF and EBF be x = f1 (y) and x = f2 (y) respectively, then
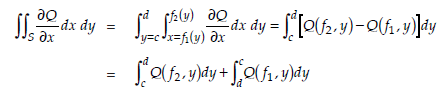
 …(2)
…(2)
Adding equation (1) and (2), we obtain
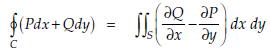
Q10) Evaluate  by using Green’s theorem, where C is a triangle formed by
by using Green’s theorem, where C is a triangle formed by 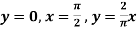
A10)
First we will draw the figure-

Here the vertices of triangle OED are (0,0), (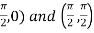
Now by using Green’s theorem-
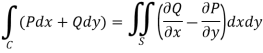
Here P = y – sinx, and Q =cosx
So that-
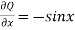 and
and 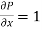
Now-
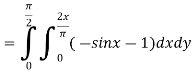
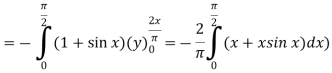
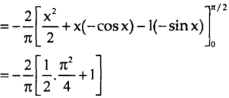
= 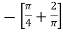
Which is the required answer.
Q11) Verify green’s theorem in xy-plane for 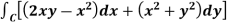 where C is the boundary of the region enclosed by
where C is the boundary of the region enclosed by 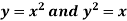
A11)
On comparing with green’s theorem,
We get-
P = 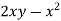 and Q =
and Q = 
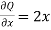 and
and 
By using Green’s theorem-
 ………….. (1)
………….. (1)
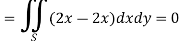
And left hand side= 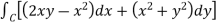
 ………….. (2)
………….. (2)
Now,
Along 

Along 
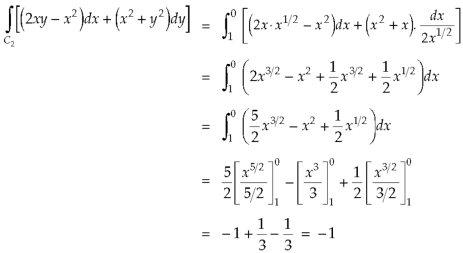
Put these values in (2), we get-
L.H.S. = 1 – 1 = 0
So that the Green’s theorem is verified.
Q12) Define surface integral.
A12)
Definition- An integral which is to be evaluated over a surface is called a surface integral.
Let  be a continuous vector point function. Let
be a continuous vector point function. Let  =
=  (u, v) be a smooth surface such that
(u, v) be a smooth surface such that 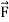 (u, v) possesses continuous first order partial derivatives. Then the normal surface integral of
(u, v) possesses continuous first order partial derivatives. Then the normal surface integral of 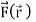 over S is denoted by
over S is denoted by
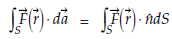
Where d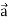 is the vector area of an element dS and
is the vector area of an element dS and 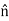 is a unit vector normal to the surface dS.
is a unit vector normal to the surface dS.
Suppose F1, F2, F3 which are the functions of x, y, z be the components of F along the coordinate axes, then surface integral
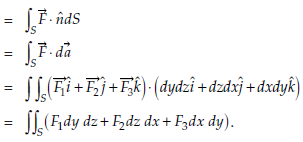
Q13) State and prove stoke’s theorem.
A13)
Statement-
If 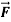 is any continuously differentiable vector point function and S is a surface bounded by a curve C, then-
is any continuously differentiable vector point function and S is a surface bounded by a curve C, then-
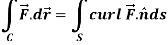
Where  is the unit normal vector at any point of S.
is the unit normal vector at any point of S.
Proof:
Let S is surface such that its projection on the xy, yz and xz planes are regions
Bounded by simple closed curves. Let equation of surface f(x, y, z) = 0, can be written as
z = f1 (x, y)
y = f2 (x, z)
x = f3 (y, z)
Let

Then we need to show that

Suppose an integral 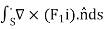 , we have
, we have
 ….(1)
….(1)
 … (2)
… (2)
Also

So that
But  is tangent to the surface S. Hence, it is perpendicular to
is tangent to the surface S. Hence, it is perpendicular to 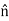
So
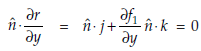
Hence

Hence, (ii) becomes
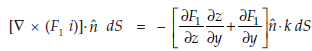 ….(3)
….(3)
But on surface S
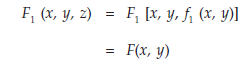 … (4)
… (4)
 … (5)
… (5)
Hence, (iii) with the help of (v) gives
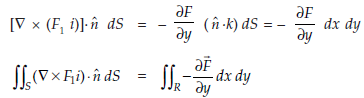 ….(6)
….(6)
Where R is projection of S on xy-plane.
Now, by Green’s theorem in plane, we have
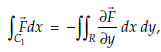
Where C1 is the boundary of R.
As at each point (x, y) of the curve C1 the value of F is same as the value of F1 at each point (x, y, z) on C and dx is same for both curves. Hence, we have
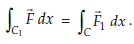
Hence
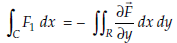 … (7)
… (7)
From eqns. (vi) and (vii), we have
 …(8)
…(8)
Similarly, taking projection on other planes, we have
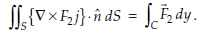 …(9)
…(9)
And
 …. (10)
…. (10)
Adding equations (viii), (ix), (x), we get
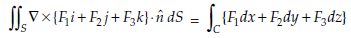
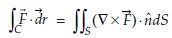
Which is the proof of stoke’s theorem
Q14) If 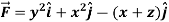 and C is the boundary of the triangle with vertices at (0, 0, 0), (1, 0, 0) and (1, 1, 0), then evaluate
and C is the boundary of the triangle with vertices at (0, 0, 0), (1, 0, 0) and (1, 1, 0), then evaluate 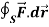 by using Stoke’s theorem.
by using Stoke’s theorem.
A14)
Here we see that z-coordinates of each vertex of the triangle is zero, so that the triangle lies in the xy-plane and 
Now,
Curl 
Curl 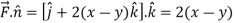
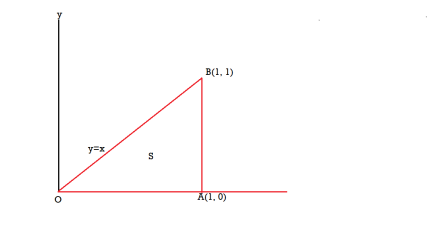
The equation of the line OB is y = x
Now by stoke’s theorem,
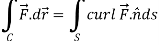
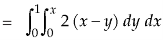

Q15) State and prove the divergence theorem.
A15)
Statement:
The surface integral of the normal component of a vector function F taken around a closed surface S is equal to the integral of the divergence of F taken over the volume V enclosed by the surface S.
Mathematically it can be written as-

Where  unit vector to the surface S.
unit vector to the surface S.
Note- Divergence theorem is the relationship between surface integral and volume integral.
Proof:

Putting the values of  in the statement of the divergence theorem, we have
in the statement of the divergence theorem, we have
 ..(1)
..(1)
We need to prove equation (1),
Let us evaluate, 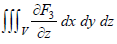
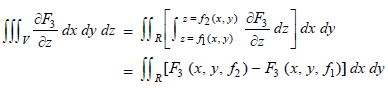 …(2)
…(2)
For the upper part of the surface i.e. S2, we have

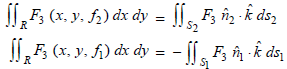
Putting these values in (2), we get
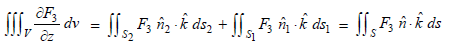 …(3)
…(3)
Similarly, it can be shown that
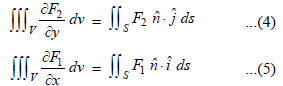
Adding (3), (4) & (5), we have

Hence proved
Q16) Prove the following by using Gauss divergence theorem-
1. 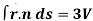
2. 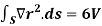
Where S is any closed surface having volume V and 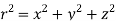
A16)
Here we have by Gauss divergence theorem-

Where V is the volume enclose by the surface S.
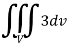
We know that-
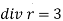
= 3V
2.
Because