Unit - 3
Systems of variable composition
Q1) Define Partial Molar quantities?
A1) The symbol Xi, here X refers to the extensive property present in a homogenous mixture, the principal species of the mixture is denoted by i, and forms the partial molar quantity of species i defined by
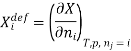
This forms the rate where the extensive property X undergoes a change with the constituent species that is added to the mixture, however the temperature pressure and the amounts of other species is kept constant. As the temperature, the pressure, and the amounts of all other species are kept constant. The partial molar quantity refers to an intensive state function whose values depend on temperature, pressure and composition of the mixture.
Here it should be noted that a charged species like ions, cannot be added directly by itself as it would result in a huge electric charge. Therefore, if the species i is charged, xi as defined by a theoretical concept whose value cannot be determined experimentally.
Among the other thermodynamic variables, the Partial molar quantities is expressed as a function. As temperature and pressure are easily controlled the functions that relate to partial molar quantities are specifically used to show in detail the chemical changes that occur in systems that refer to the assumptions and is introduced in §1. The parameter chemical potential is similar to partial molar Gibbs free energy, it plays a very important role in such equations.
Most often the pressure, temperature and the number of moles for every component are independent variables. And are useful easy to control in typical lab experiments. The Partial derivatives of thermodynamic quantities, taken with respect to the number of moles of a component, at constant pressure, temperature, and θk, are given a special designation; they are called partial molar quantities. That is,
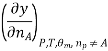
Is the partial molar energy of component A,
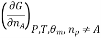
Is the partial molar Gibbs free energy, etc. All partial molar quantities are intensive variables.
The variable partial molar quantities are specifically useful; therefore, they have a distinctive symbol to represent them. We use a horizontal bar over a thermodynamic variable to represent a partial molar quantity. (A horizontal bar is used to imply per mole, when this is used to designate partial molar quantity, it implies per mole of a specific component) Thus, we write
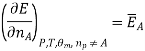
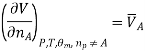
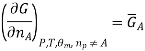
We introduce the chemical potential for substance A, μA, and find that the chemical potential of substance A is equivalently expressed by several partial derivatives. In particular, we have
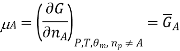
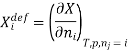
That is, the chemical potential is also the partial molar Gibbs free energy.
Q2) Derive the Gibbs-Duhem equation?
A2) The Gibbs-Duhem equation in thermodynamics, shows the relationship between changes in chemical potential for components in a thermodynamic system.
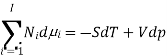
Where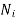 
represents the number of moles of the particular component, 
, 
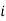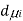the incremental increase in chemical potential for this component, S refers to the entropy, T the absolute temperature, V volume and p the pressure. Thermodynamically the intensive properties are not independent, they are however related forming a statement mathematically of the state postulate. When the variables are temperature and pressure, only 
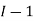of 
components have independent values for chemical potential and Gibb’s phase rule follows. The equation is named after Josiah Gibbs and Pierre Duhem.
Derivation
The derivation of Gibbs -Duhem equation is straight forward as it is obtained from basic thermodynamic state equation. The total differential of the Gibbs free energy G in terms of its Natural variables is
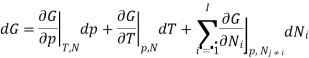
With the substitution of two of the Maxell relations and the definition of chemical potential, this is transformed into:
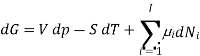
As shown in the Gibbs free energy, the chemical potential is just another name for the partial molar (or just partial, depending on the units of N) Gibbs free energy, thus
The total differential of this expression is
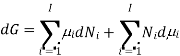
Subtracting the two expressions for the total differential of the Gibbs free energy gives the Gibbs-Duhem relation:
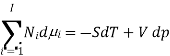
Q3) What does the chemical potential of an ideal gas mean?
A3) The chemical potential is defined in terms of the entropy a
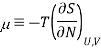
This definition leads to a general thermodynamic identity
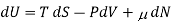
For a mixture of ideal gases, each species i constitutes a molar fraction xi of the total number N total of molecules, so each species has its own chemical potential defined as
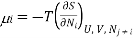
(3) where all Nj with j 6= i are held constant in the derivative. Also, for an ideal gas, each species contributes its own portion of the overall entropy, independently of the other species. We can see this by noting that if we have a mixture of, say, 2 gases, then for each configuration of the gas A molecules there is a multiplicity of ΩB of the gas B molecules and since for an ideal gas, the molecules don’t interact, the total multiplicity of the mixture is Ωtotal = ΩAΩB, so the entropy is the sum of the entropies for the separate species: Stotal = k lnΩtotal = SA +SB. Since ideal gas molecules don’t interact, species i contributes a fraction xi of the total pressure, or in other words, its partial pressure is
We can therefore write the thermodynamic identity for a mixture of ideal gases as
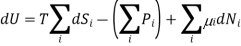
Since dSj6=i = 0 in 3 (because only the number Ni of species i is changing, and no properties of any of the other species are changing), we can write the chemical potential of species i as
But this is the definition of chemical potential in a system containing only species i at partial pressure Pi in volume V . Thus, for a mixture of ideal gases, the chemical potential of each species is independent of the other species. In a mixture of real gases, however, this is probably not the case, since interactions between the species means.
Q4) What changes occur when mixing of ideal gases takes place with respect to Gibbs free energy?
A4) In a single phase, two or more components mix homogenously, forming a solution. A solution is created when two or more components mix homogeneously to form a single phase. And chemical processes, occur in multiple component system, therefore such solution becomes very important, the integral part of any system is to analyse and understand the behaviour of mixtures that involve ideal and non-ideal solutions as they provide valuable information on the molecular properties of the systems.
At standard temperature and pressure, most of the gases behave like ideal gases, this enables us to combine the knowledge about ideal systems and solutions with standard state thermodynamics. In order to derive a set of equations that quantitatively describe the effect that mixing has on a given gas-phase solution’s thermodynamic quantities.
Gibbs Free Energy of Mixing
The one-component system, possess extensive properties that are dependent on the amount of the system present, however the extensive properties of a solution is dependent on the parameters such as pressure, temperature and composition, which is to be understood that the mixture is described in terms of the partial molar quantities and its components. The total Gibbs free energy of a two-component solution is given by the expression
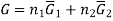
Where
G is the total Gibbs energy of the system,
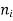is the number of moles of component i, and 
is the partial molar gibbs energy of component i.
The molar Gibbs energy of an ideal gas can be found using the equation
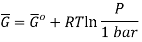
Where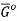 
is the standard molar gibbs energy of shte gas at 1 bar and P is the pressure of shte system. In a mixture of ideal gases, molar Gibbs energy is equivalent ot its chemical potential or that
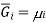
This means that a solution for ideal gases, the equation becomes,
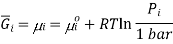
Where
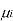is the chemical potential of the ith component
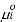is the standard chemical potential of component 
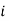at 1 bar, and
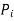is the partial pressure of component 
.
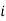Now if we consider the two gases at the same temperature, for example gas1 and gas 2, the Gibbs free energy of the system before the gases are mixed and can be combined with the first and the above equation to form,
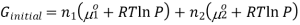
If gas 1 and 2 are mixed together, they will exert a partial pressure on the total system, P1 and P2 therefore P1+P2 = P, thus the final Gibbs free energy can be calculated using the equation,
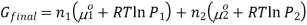
The gibbs energy of mixing, 
can then be found by subtracting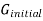 
from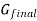 
.
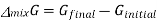
Where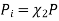
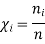
And χi is the mole fraction of gas i. This equation can be simplified further by knowing that the mole fraction of a component is equal to the number of moles of that component over the total moles of the system, o
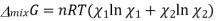
Q5) What changes occur in Entropy occur when mixing of ideal gases take place?
A5) Entropy of mixing
The figure below shows that when two gases mix, it can really be seen as two gases expanding into twice their original volume. This greatly increases the number of available microstates, and therefore we can expect the entropy of the system to increase as well.
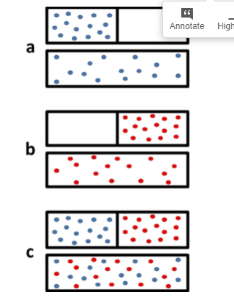
Figure: The mixing of two gases can be seen as two expansions. (a) Expansion of gas 1 alone when the barrier is removed. In the microstate of the open boxes the molecules are present in twice its number. (b) Expansion of gas 2 along. (c) The expansion of gases occur and is equivalent to mixing
In Thermodynamic the dependence of Gibbs free energy with respect to temperature is shown as
This means that differentiating Equation at constant pressure with respect to temperature will give an expression for the effect that mixing has on the entropy of a solution. We see that
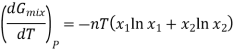
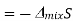
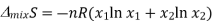
Since the mole fractions again lead to negative values for ln x1 and ln x2, the negative sign in front of the equation makes ΔmixS positive, as expected. This agrees with the idea that mixing is a spontaneous process.
Q6) Explain the criteria of thermodynamic Equilibrium?
A6) Thermodynamic equilibrium is a self-evident and clear concept of thermodynamics, it is a single system and an internal state thermodynamic system.it also refers to the various other thermodynamic systems that are connected more or less to the permeable and impermeable walls. In thermodynamic equilibrium there are no net macroscopic flows of matter or of energy either within a system or between systems.
No macroscopic change occurs when a system is present in its own state of internal thermodynamic equilibrium.
In thermodynamic equilibrium, the systema are mutual in nature they include thermal, mechanical, chemical and radiative equilibria. The system can exist in one kind of mutual equilibrium, in thermodynamic equilibrium, all kinds of equilibrium hold at once and indefinitely, until disturbed by a thermodynamic operation. In a macroscopic equilibrium, perfectly or almost perfectly balanced microscopic exchanges occur; this is the physical explanation of the notion of macroscopic equilibrium.
In the thermodynamic equilibrium when present in a state of internal equilibrium, has a spatial uniform temperature, the intensive properties excluding the temperature may be driven to spatial inhomogeneity by an unchanging long-range force field imposed on it by its surroundings.
In systems that are at a state of non equilibrium there are, by contrast, net flows of matter or energy. If such changes can be triggered to occur in a system in which they are not already occurring, the system is said to be in a meta-stable equilibrium.
Though not a widely named "law," it is an obvious state of thermodynamics that there exist states of thermodynamic equilibrium. The second law of thermodynamics states that when a body of material starts from an equilibrium state, in which, portions of it are held at different states by more or less permeable or impermeable partitions, and a thermodynamic operation removes or makes the partitions more permeable and it is isolated, then it spontaneously reaches its own, new state of internal thermodynamic equilibrium, and this is accompanied by an increase in the sum of the entropies of the portions
Therefore, the concept of equilibrium conveys something that “balances out.” Equilibrium is a state of vanishing driving forces or gradients, where everything is constant, if a system is in equilibrium, it retains its current state because there are no driving forces causing anything to change.
When two materials have similar temperature, they are called thermal equilibrium. There are no thermal gradients and no exchange of heat takes place for example a liquid and a vapor phase are in thermal equilibrium when:
Tl=Tv
Mechanical equilibrium occurs when two substances are present at same pressure, there is absence of bulk movement as there is no pressure gradients. A liquid and a vapor phase are in mechanical equilibrium when:
Pl=Pv
For a system that is thermodynamic, if it has to be in equilibrium then all its intensive (temperature, pressure) and extensive thermodynamic properties (U, G, A, H, S, etc) must be constants. Therefore, the total changes that occur in any of these properties should be zero at equilibrium.
When we consider a heterogeneous vapour-liquid closed system, any change that occurs in the total property of the system, results in the change of that property in the liquid phase and also the changes of that property in the vapour phase.
DI(total)=dI(liquid)+dI(vapor)
In this case, the liquid and the vapour are not closed system themselves, however they can exchange liquid and vapour within themselves but not with the surroundings. To elaborate more upon the concept of equilibrium, let’s look at equation .as it refers to the changes in pressure and temperature, the two measurable laboratory quantities written in both phases
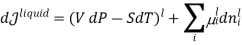
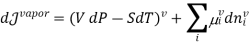
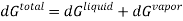
Hence
Since at equilibrium all extensive properties, such as G, must remain constant, dG(total) must be zero.
[Mechanical equilibrium]
DT=0 [Thermal equilibrium]
[
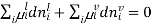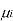criteria for equilibrium]
It can be also proven that, A minimum value of the total free energy of the system (G(total)) is attained at equilibrium, the total free energy of the system must take a minimum value; this reinforces the fact that dG(total)=0 at equilibrium. The minimum Gibbs energy criterion for equilibrium is a restatement of the second law of thermodynamics, from which we know that the entropy of a system in equilibrium must be at its maximum, considering all of the possible states for equilibrium.
Q7) Elaborate the chemical Equilibrium in Ideal gases?
A7) Consider a reaction of the type
Where A, B, C, and D all behave ideally. During the reaction, the partial pressure of each gas changes. The resulting free energy change for each gas can be expressed as
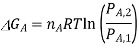
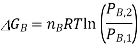
If we assume that the reaction starts with each gas at the standard pressure, denoted as P°, we may write
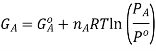
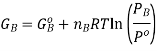
It is important to remember that the free energies in the expressions above are NOT molar quantities, ie we need to keep in mind that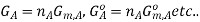 
Now, the free energy change for the reaction may be written as
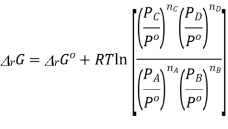
Where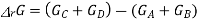 
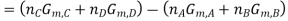
And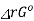 
is similarly defined.
Now at equilibrium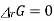 
And so, we get
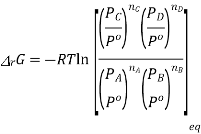
The partial pressures that enter into this expression are the values measured at equilibrium and, therefore, is a constant at a given temperature. Note that, because of the division of each pressure term by the standard pressure, the quantity within the square brackets is dimensionless
In other words,
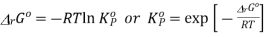
This is the thermodynamically correct definition of the equilibrium constant in relation to pressure. Note that this is a dimensionless quantity due to the division of each pressure term by the standard pressure. For real gases, the partial pressures must be replaced by partial flow capacities. The equilibrium constant can also be expressed in concentrations by inserting PA = nART / V etc. and recognizing that nA / V = [A] etc. where xA is the mole fraction of A and P is the total pressure in equilibrium, we can express the equilibrium constant in terms of mole fractions K
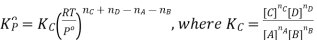
Also, by recalling that PA = xAP, where xA is the mole fraction of A and P is the total pressure at equilibrium, we can express the equilibrium constant in terms of mole-fractions K
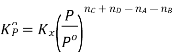
Where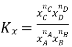 
Q8) Define the Concept of fugacity?
A8) We have seen that, for a closed system, the Gibbs energy is related to pressure and temperature as follows:
DG=VdP−SdT
For a constant temperature process,
DG=VdP @ constant T
For an ideal gas,
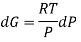
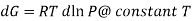
The expression is applied only to ideal gases, but later this concept was extended and the expression was applicable to all substances, this was done by Lewis in 1905 and he defined a new thermodynamic property was defined called the fugacity, f, such that:
DG=RTd In f @ constant T
This definition implies that for ideal gases, ‘f’ must be equal to ‘P’. For mixtures, this expression is written as:
DGi¯¯¯¯=RTd In fi @ constant T
Where Gi¯¯¯¯ and fi are the partial molar Gibbs energy and fugacity of the i-th component, respectively. As Gibbs energy is closely related to chemical potential, Fugacity can be related directly to chemical potential. Therefore, the definition of fugacity in terms of chemical potential becomes:
For a pure substance,
d In f=dμRT@ constant T
LimP→0 f=P (ideal gas limit)
For a component in a mixture,
d In fi= dμiRT@ constant T
LimP→0 fi=yiP = partial pressure (ideal gas limit).
The fugacity coefficient (ϕi is defined as the ratio of fugacity to its value at the ideal state. Hence, for pure substances:
ϕ=fP2
And for a component in a mixture,
ϕi=fiyiP
y coefficient takes a value of unity when the substance behaves like an ideal gas. Therefore, the fugacity coefficient is also regarded as a measure of non-ideality; the closer the value of the fugacity coefficient is to unity, the closer we are to the ideal state.
Fugacity results in a secondary chemical potential, the above definitions allow us to restate the same principle in terms of fugacity. To do this, previous expressions can be integrated for the change of state from liquid to vapor at saturation conditions to obtain:
∫lvd In fi=1RT∫lvdμi
In f(v)i− In f(v)i=1RT(μ(v)i−μ(l)i)
For equilibrium, μ(l)i=μ(v)i
Hence,
In(f(v)if(l)i)=0
Therefore:
f(l)i=f(v); i=1, 2, ... nci
The fugacities must be the same for equilibrium.in other words for the system to be in equilibrium. The chemical potential and fugacity of each component in every phase must be equal. Once one of them is satisfied, the other is satisfied immediately.
Using μ(l)i=μ(v)i or f(l)i=f(v)i
To describe equilibrium is a matter of choice, but generally the fugacity approach is preferred
Q9) Differentiate between Extensive and Intensive properties?
A9) Thermodynamic properties can be divided into 2 (two) general classes such as intensive and extensive properties.
An intensive property, is the system’s physical property that does not depend on the size or the amount of material in the system. In contrast the extensive property of a system is not dependent on the system size or the amount of the material. According to the definitions, density, pressure and temperature are intensive properties and volume, internal energy are extensive properties.
Symbols for representing properties: Extensive properties are symbolized by upper case (capital) letter such as V (volume), KE (kinetic energy), PE (potential energy), etc. Intensive properties are symbolized by lower case letters such as v (specific volume), ke (specific kinetic energy), e, u (specific internal energy), h (specific enthalpy), etc. Mole based properties are symbolized by lower case letters with overbars. For example, kinetic energy and molar specific potential energy respectively.
Q10) Derive the relationship between Gibbs free Energy and Reaction Quotient?
A10) The Van 't Hoff equation relates the change in the equilibrium constant, Keq, of a chemical reaction to the change in temperature T, given the standard enthalpy change, ΔrH⊖, for the process
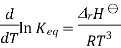
Where ln denotes natural logarithm Keq is the thermodynamic equilibrium constant, and R is the ideal gas constant. This equation is similar and occurs at all pressures and at any one temperature, it is derived from the requirement that the Gibbs free energy of reaction be stationary in a state of chemical equilibrium.
In general, the equation is integrated at two temperatures, assuming the standard reaction enthalpy ΔrH⊖ is constant (and furthermore, this is also often assumed to be equal to its value at standard temperature). Since in reality ΔrH⊖ and the standard reaction entropy ΔrS⊖ do vary with temperature for most processes, the integrated equation is only approximate. Approximations are also made in practice to the activity coefficients within the equilibrium constant.
A new equilibrium constant is established when the equation is integrated at a new absolute temperature, that assumes standard enthalpy change over the temperature range. To obtain the integrated equation, it is convenient to first rewrite the Van 't Hoff equation as
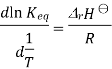
The definite integral between temperatures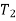 
and 
is then
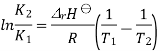
Combining the well-known formula for the Gibbs free energy of reaction
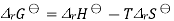
Where S is the entropy of the system, with Gibbs free energy isotherm equation
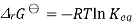
We obtain
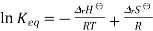
Differentiation of this expression with respect to the variable T while assuming that both ΔrH⊖ and ΔrSH⊖ are independent of T yields the Van 't Hoff equation. These assumptions are expected to break down somewhat for large temperature variations.
Provided that ΔrH⊖ and ΔrSH⊖ a are constant, the preceding equation gives ln K as a linear function of 1/T and hence is known as the linear form of the Van 't Hoff equation. Therefore, a straight line Is formed, when a plot of the natural logarithm of the equilibrium is plotted against reciprocal temperature .as the temperature is quite small and the standard Enthalpy and Entropy is constant. The slope of the line may be multiplied by the gas constant R to obtain the standard enthalpy change of the reaction, and the intercept may be multiplied by R to obtain the standard entropy change.
Q10) Define an Equilibrium Constant?
A11) The equilibrium constant of a chemical reaction (usually denoted by the symbol K) provides insight into the relationship between the products and reactants when a chemical reaction reaches equilibrium. For example, the equilibrium constant of concentration (denoted by Kc) of a chemical reaction at equilibrium can be defined as the ratio of the concentration of products to the concentration of the reactants, each raised to their respective stoichiometric coefficients. It is important to note that there are several different types of equilibrium constants that provide relationships between the products and the reactants of equilibrium reactions in terms of different units.
For a chemical reaction, the equilibrium constant can be defined as the ratio between the amount of reactant and the amount of product which is used to determine chemical behaviour
Q11) Explain the Equilibrium in Homogenous solutions?
A12) In order to simplify the problems and understand the concept, we divide such reactions into different categories, namely, the homogeneous reactions, where the components involved in the reaction are present in the same phase and the heterogeneous reactions, where the components involved are present in different phases. The methods of dealing with both the reactions are different and so is the determination of the equilibrium state. In this section, we will learn about a homogeneous equilibrium and the calculation of equilibrium constant for a homogeneous reaction.
Equilibrium Constant for Homogeneous Reaction
Let us consider a homogeneous system, given by the following reaction
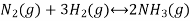
Ammonia is formed when gaseous nitrogen reacts with gaseous hydrogen, the equilibrium constant can now be calculated for the reaction above.
We can write the molar concentration of the reaction components, can be written as
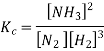
As we know, for reactions involving gases, we express the equilibrium concentration in terms of the partial pressure.
Using the ideal gas equation,
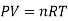
Where P is the pressure of the system, V is the volume of the system, n is the number of moles of the components in the system, R is the universal gas constant, and T is the temperature of the system.
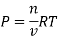
Here, c is the concentration of the system. We can also write it as,
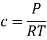
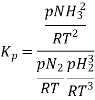
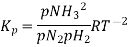
So, equilibrium constant can also be written as,
Here, we note that the general expression for Kc and Kp can be written as,
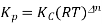
Where Δn refers to the numbers of moles of gaseous products and the number of moles of gaseous reactants.
Q12) What changes occur when mixing of ideal gases takes place with respect to Enthalpy?
A13) We know that in an ideal system ΔG=ΔH−TΔSΔG=ΔH−TΔS, but this equation can also be applied to the thermodynamics of mixing and solved for the enthalpy of mixing so that it reads
Plugging in our expressions for ΔmixG and ΔmixS , we get
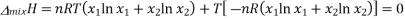
This result makes sense when considering the system. The molecules of ideal gas are spread out enough that they do not interact with one another when mixed, which implies that no heat is absorbed or produced and results in a Δmix of zero. Figure illustrates how TΔmix and Δmix change as a function of the mole fraction so that ΔmixH of a solution will always be equal to zero (this is for the mixing of two ideal gasses).
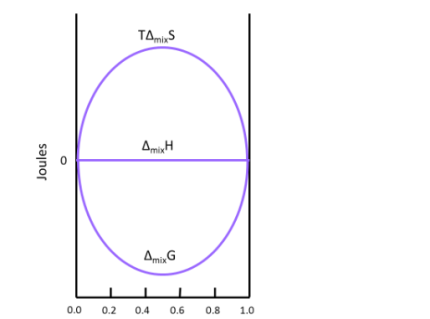
A graph of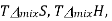 
and 
as a function of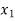 
For the mixing of two ideal gases.
Q13) Explain the relation of Gibbs free energy and Spontaneity?
A14) Gibbs energy is a state function and an Extensive property Since, change in enthalpy too increases or decreases the randomness by affecting the molecular motions, entropy change alone cannot account for the spontaneity of such process. Therefore, we use the Gibbs energy change for explaining the spontaneity of a process
Expression for Gibbs Energy Change
General expression for Gibbs energy change at constant temperature is expressed as:
△Gsys = △Hsys–T△Ssys
Where,
△Gsys = Gibbs energy change of the system
△Hsys = enthalpy change of the system
△Ssys = entropy change of the system
T = Temperature of the system
Above equation is popularly known as Gibbs equation. Gibbs’ equation relates enthalpy and entropy of the system. We know that for a spontaneous process, the total entropy change,
△Stotal is greater than zero.
△Stotal = △Ssys+△Ssurr
Where,
△Stotal = total entropy change for the process
△Ssys = entropy change of the system
△Ssurr = entropy change of the surrounding
In case of thermal equilibrium between system and surrounding, temperature change between system and surrounding, △T = 0. Hence, we can say that enthalpy lost by the system is gained by the surrounding. Hence, the entropy change of the surrounding is given as,
△Ssurr = △HsurrT=–△HsysT
△Stotal = △Ssys+(−△HsysT)
△Hsurr = change in enthalpy of the surrounding
△Hsys = change in enthalpy of the system
As discussed earlier, for the spontaneity of a process,
ΔStotal > 0. Above equation becomes,
T△Ssys–△Hsys>0
△Hsys–T△Ssys<0
Above equation can be related to Gibbs equation as,
△Gsys<0
On the basis of above equation we can infer:
- △Gsys<0, the process is spontaneous
- △Gsys>0,, the process is non-spontaneous
The Spontaneity of a Process
Gibbs equation helps us to predict the spontaneity of reaction on the basis of enthalpy and entropy values directly. When the reaction is exothermic, enthalpy of the system is negative making Gibbs free energy negative. Hence, we can say that all exothermic reactions are spontaneous.
In the case of endothermic reaction when enthalpy of the system is positive, the process is spontaneous under two conditions:
- Temperature is very high to make the Gibbs energy value negative
- Entropy change is very high to make the Gibbs free energy negative.
Spontaneity can only indicate if a reaction can occur not necessarily if a reaction will occur. For example, the conversion of diamond to graphite is a spontaneous process at Standard Temperature and Pressure (STP) but it is a slow process. It will take years for the transformation to occur
Q14) Explain the Relation between the equilibrium constants Kp, Kc and Kx.?
A15) For a balanced reaction of the type,
AA + bB ⇌ cC + dD
According to the law of mass action, the constant value obtained by relating equilibrium concentrations of reactants and products is called the equilibrium constant. For the forward reaction, this is given by
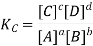
The equilibrium constant for the reverse reaction is the inverse of the forward reaction and is given by:
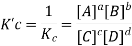
If the coefficients of the chemical equation are multiplied by a factor ‘n’ then the equilibrium constant is raised by the power ‘n’ i.e., the constant becomes Knc.
Equilibrium Constant Representation | Expressed in terms of | Expressed as |
Kc | Concentrations of reactants and products |  |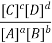
Kp | Partial pressures of reactants and products. (only for the substances which are in gaseous state) |  |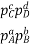
Kx | Mole fractions of reactants and products |  |
Relation between Kc, Kp and Kx
Kp = Kc(RT)Δng
Kx = Kp(RT)Δng
Where,
Δng = moles of gaseous products – moles of gaseous reactants.
Kc is the equilibrium constant expressed in terms of the concentration of the reactants/products. Similarly, Kp is the constant in terms of the partial pressures of the substances and K x is expressed in terms of the mole fraction.
Q15) Explain the Le Chatelier’s Principle?
A16) Le Chatelier’s principles, also known as the equilibrium law, are used to predict the effect of some changes on a system in chemical equilibrium (such as the change in temperature or pressure). The principle is named after the French chemist Henry Louis Le Chatelier.
Le Chatelier said that equilibrium adjusts the forward and backward reactions in such a way to accept the changes affecting the equilibrium conditions.
Le Chatelier's principle can be used to predict the behaviour of a system due to changes in pressure, temperature, or concentration. Le Chatelier's principle implies that the addition of heat to a reaction will favour the endothermic direction of a reaction as this reduces the amount of heat produced in the system.
According to Le Chatelier’s Principles,
- In exothermic equilibrium, an increase in temperature decreases the product formation and decrease in temperature increases product formation.
- In endothermic reactions, an increase in temperature increases the product formation and decrease in temperature decreases the product formation.
Q16) How do different parameters (Temp, pressure, volume and concentration) change with the Equilibrium constant?
A17)
Effect of Concentration Changes on Equilibrium and Product Formation
As per Le Chatelier’s principles, the only way of equilibrium to accept more reactant is to increase product formation. The forward reaction is favoured when the concentration of the reactant is increased. The equilibrium of the reaction shift towards the use of reactants in the reaction, which decreases the concentration of the reactants.
Similarly, the addition of product (concentration/pressure) shall increase the backward reaction to decrease the product concentration. The backward reaction is favoured when the concentration of the reactant decreases and equilibrium of the reaction shift towards the production of reactants and concentration of the reactants will be more.
Effect of Change of Volume, Pressure, or Inert Gas on Equilibrium and Product Formation
Kp = Kc (RT)Δn = Kc (p/v)Δn
Change of volume, pressure, or inert gases has no effect on reactions of liquids and solids. They may have an effect in gaseous reactions and that too only when the difference in the sum of the number of reactant and product molecules (∆n) is not zero.
When ∆n = 0:
As per the Le Chatelier’s principles, there will be no effect on Equilibrium and Product Formation on changing the volume, pressure or inert gas.
When ∆n = +ve:
Increase of pressure or decrease in volume will decrease the formation of the product. Decrease of pressure or increase of volume shall have the opposite effect of increasing the product formation.
Effect of Change of Temperature on Equilibrium and Product Formation
The individual reaction in the equilibrium can be either endothermic or exothermic. Likewise, at equilibrium net energy involved may make the reversible reactions either endothermic or exothermic
Q17) Highlight the Applications of Le Chatelier’s principle?
A18)
- Le Châtelier's principle can be used to encourage formation of a desired product in chemical reactions. In the Haber process for the industrial synthesis of ammonia, nitrogen gas and hydrogen gas react to form ammonia gas in the reaction N2+3H2→2NH3; the process is exothermic, i.e., one that gives off heat.
- The le Chatelier's principle can be applied to understand the effect of change in pressure on the systems at equilibrium as follows. 1) When the partial pressure of any of the gaseous reactants or of the products is increased, the position of equilibrium is shifted so as to decrease its partial pressure.
- Contact process in the manufacture of sulfuric acid and the synthesis of methanol.
- Le-Chatelier’s principle of equilibrium is used in the industrial applications in the scheme of reaction, that involves certain parameters like pressure, temperature, concentration of reaction and species with a single parameter that results in the change of equilibrium leading to undesired product formation.
Q18) Explain the Equilibrium Constant in a heterogenous reaction?
A19) Equilibrium Constant for Heterogeneous Reaction
When the state of equilibrium in a system has components in more than one phase, it is called heterogeneous equilibrium if, for example, we take a container of ice and water at a temperature that allows both phases to exist simultaneously, so that both ice and water. Water is in a state of equilibrium. This state is called heterogeneous equilibrium.
In his equation this can be written as follows:
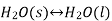
When we look at another example, an aqueous solution of a solid like calcium hydroxide, we observe that the solid calcium hydroxide is in equilibrium with its saturated solution.
Writing the equilibrium constant for heterogeneous reactions differs from that for homogeneous reactions. For example, consider the thermal dissociation of calcium carbonate into calcium oxide and carbon dioxide. Here we see that the equilibrium constant for the dissociation of the reactant into its products depends only on the gaseous component, since solid and liquid reactants are regarded as constant.
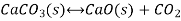
Here, the components CaCO3 and CaO are solids, so their molar concentration remains constant throughout the reaction. Therefore, the equilibrium constant can be written as,
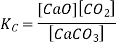
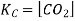
Also, in terms of Kp, we can write
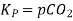
Where p denotes the partial pressure. In other words, we can state that, at a given temperature, there is a constant concentration or partial pressure of CO2 in the equilibrium reaction with CaO and CaCO3.
Q19) How does the reaction advance with respect to the Equilibrium constant?
A20) The product of activities at equilibrium is known as equilibrium constant of the reaction
ΔrGo = - R T I n K
When ΔrGo > 0, K< 1
i.e at equilibrium, the partial pressure of A> partial pressure of B
PA > PB
Which means reactant A is favoured in the reaction or the reaction proceeds in the backward reaction.
When ΔrGo < 0
i.e at equilibrium, the partial pressure of b > partial pressure of A
PA < PB
Product B is favoured in the reaction and the reaction proceeds in the forward reaction.
