Unit - 2

Transition Elements-I

Q1) What are Transition Elements?

A1)

Transition elements are elements with partially filled d orbitals (also known as transition metals). Transition elements are described by IUPAC as elements with a partially filled d subshell or elements that have the ability to form stable cations with an incompletely filled d orbital.

Any element that corresponds to the d-block of the modern periodic table (which includes groups 3-12) is called a transition element in general. Also, the lanthanides and actinides, which make up the f-block, are called transition metals.

The f-block elements are also referred to as inner transition elements or inner transition metals because their f-orbitals are incompletely filled. The role of transition metals on the periodic table, as well as their general electronic configurations, are depicted in the diagram below.

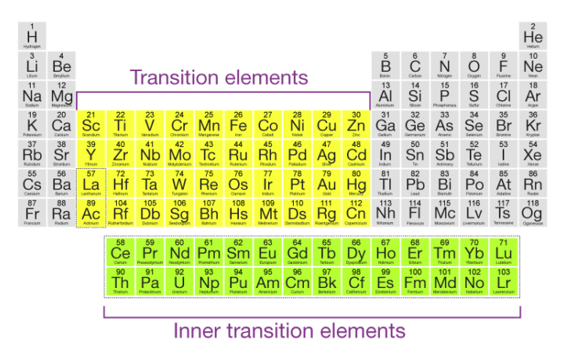
Because of their electronic structures, which correspond to (n-1) d10 ns2, mercury, cadmium, and zinc are not considered transition elements.

In their ground states and also in some of their oxidation states, these elements have fully filled d orbitals. The +2 oxidation state of mercury, which corresponds to an electronic configuration of (n-1) d10, is one such example.

Q2) What are electronic configuration of Transition Elements?

A2)

The first two rows of transition elements are mentioned below, along with the electronic configurations that correspond to them. It should be noted that the electron configuration in some of these elements corresponds to (n-1) d5 ns1 or (n-1) d10 ns1. This is due to the stability offered by electron orbitals that are half-filled or fully filled.

Many transition elements, such as chromium, do not seem to obey the Aufbau theory. The low energy difference between the 3d and 4s orbitals, as well as the 4d and 5s orbitals, is thought to be the explanation for this.

Q3) What are General properties of Transition Elements?

A3)

Zinc, cadmium, and mercury are not called transition elements because their electronic structures vary from that of other transition metals, as previously mentioned. The rest of the d-block elements, on the other hand, have properties that are very similar, and this similarity can be seen along each row of the periodic table. The transition elements' properties are described below.

Colored compounds and ions are formed by these elements. The d-d electron transition is responsible for this colour.

• The energy difference between these elements' potential oxidation states is relatively small. As a result, the transition elements have a wide range of oxidation states.
• Because of the unpaired electrons in the d orbital, these elements form a lot of paramagnetic compounds.
• These elements can be bound by a wide variety of ligands. As a result, transition elements can form a wide range of stable complexes.
• These elements have a very high charge-to-radius ratio.
• As compared to other elements, transition metals are strong and have relatively high densities.
• Owing to the participation of the delocalized d electrons in metallic bonding, these elements have high boiling and melting points.
• The transition elements are strong conductors of electricity due to the metallic bonding of the delocalized d electrons.
• Catalytic properties of some transition metals are very useful in the industrial processing of certain chemicals. Iron, for example, is used as a catalyst in the Haber ammonia production process. In the industrial manufacture of sulfuric acid, vanadium pentoxide is sometimes used as a catalyst.

Q4) Define Atomic Ionic radii.

A4)

Because of the weak shielding provided by the small number of d-electrons, the transition elements' atomic and ionic radii decrease from group 3 to group 6. Those in groups 7 and 10 have atomic radii that are very similar, while those in groups 11 and 12 have larger radii. This is due to the electron-electron repulsions, which balance out the nuclear charge.

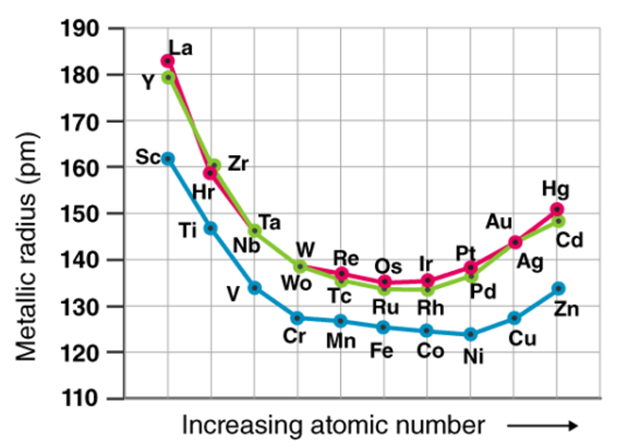
As you progress down the group, you'll notice a rise in the atomic and ionic radii of the elements. The existence of a larger number of subshells may explain the increase in radius.

Q5) Explain Electronic Structure and Reactivity of the Transition Metals.

A5)

The valence electron configurations of the first-row transition metals are given in Table. As we go across the row from left to right, electrons are added to the 3d subshell to neutralize the increase in the positive charge of the nucleus as the atomic number increases. With two important exceptions, the 3d subshell is filled as expected based on the Aufbau principle and Hund’s rule. Unexpectedly, however, chromium has a 4s13d5 electron configuration rather than the 4s23d4 configuration predicted by the Aufbau principle, and copper is 4s13d10 rather than 4s23d9. We attributed these anomalies to the extra stability associated with half-filled subshells. Because the ns and (n − 1) d subshells in these elements are similar in energy, even relatively small effects are enough to produce apparently anomalous electron configurations.

Electron–electron repulsions inside the 4d subshell in second-row transition metals cause additional abnormalities in electron configurations that are difficult to predict. Nb and Tc, with atomic numbers 41 and 43, have a half-filled 5s subshell, with valence electron configurations of 5s14d4 and 5s14d6, respectively. Further problems arise in third-row transition metals, where the energies of the 4f, 5d, and 6s orbitals are extremely similar. While La has a valence electron configuration of 6s25d1, the next part, Ce, has a valence electron configuration of 6s25d04f2. Added electrons join the 4f subshell from this point via element 71, giving rise to the 14 elements known as the lanthanides. Following the filling of the 4f subshell, the 5d subshell is occupied, resulting in the third row of transition metals. The actinides have three subshells (7s, 6d, and 5f) that are so close in energy that their electron configurations are even more unstable in the seventh century.

The size of neutral atoms in the d-block elements gradually decreases from left to right across a row, as seen in the s-block and p-block elements, due to an increase in the effective nuclear charge (Zeff) with increasing atomic number. Furthermore, as with the s and p blocks, the atomic radius increases as one moves down the group. The increase in size between the 3d and 4d metals, however, is much greater than the increase in size between the 4d and 5d metals due to lanthanide contraction (Figure 23.1). Ionic radii show the results of lanthanide contraction, which explains why, for example, there is only a small rise in radius from Mo3+ to W3+.

Q6) Give Catalytic properties of transition metals and its application.

A6)

Catalysts are substances that change the rate of a chemical reaction without changing themselves. As compared to the amount of reactants, the amount of catalyst used for any given reaction is usually very small. Catalysts are divided into two categories: positive catalysts that speed up reactions and negative catalysts that slow down reactions. Transition metals and their oxides are used as catalysts in a variety of chemical reactions. Transition metals and their derivatives, such as iron, cobalt, nickel, platinum, chromium, and manganese, are commonly used catalysts in today's industries. Here is a representation of transition metals in the periodic table:

Q7) Explain behaviour of catalyst.

A7)

Transition metals exhibit catalytic behaviour for a variety of reasons:

1. The existence of unoccupied d orbitals.

2. They have the ability to display a wide range of valency’s.

3. They have a proclivity for forming complicated compounds.

Since transition metals have a propensity to exhibit variable valency and form complexes, they form unstable intermediates with their reactants. The reaction will take a different direction with a lower activation energy because of the unstable intermediate produced during the reaction. The rate of the reaction increases as the activation energy is reduced. Later in the reaction, these unstable intermediates decompose to give the final product, and the catalyst is regenerated. Finely divided catalysts are often used because they have a larger surface area for the reaction to take place. The reactant molecules are absorbed on the surface due to the wide surface area, which provides free valencies. A large surface allows more reactant molecules to travel closer together and combine, increasing the rate of reaction.

Q8) Write applications of catalyst.

A8)

Catalysts are used in almost every reaction that is used in industry for industrial purposes. Catalysts are used in the following reactions:

1. Finely divided nickel is used as a catalyst in hydrogenation reactions. The catalyst is primarily used to hydrogenate oil for the production of vegetable ghee.

2. Finely divided iron is used as a catalyst in Haber's method to synthesise ammonia.

3. V2O5 is used in the Contact phase to produce H2SO4.

4. TiCl4 is used in the production of high-density polyethylene (HDPE).

We've learned about transition elements' catalytic properties, how they're used in the production of different materials, and why they behave this way. Download BYJU'S – The Learning App for any more questions on any subject and have fun learning.

Q9) Explain Magnetic Properties of Coordination Compounds.

A9)

The ability of transition metals to form magnets is an intriguing property. Unpaired electrons in metal complexes make them magnetic. This magnetism may be due to the presence of unpaired d electrons, since the last electrons are in the d orbitals. Unpaired electrons occur in monometallic complexes when the complex has an odd number of electrons or when electron pairing is destabilised.

Monomeric Ti (III) species, for example, have one d electron and must be (para)magnetic, regardless of ligand geometry or existence. Ti (II) forms certain complexes with two unpaired electrons and others with none when it has two d electrons.

Fe, for instance, tends to live as Fe3+ and has a coordination number of six. Since Fe3+ has five d electrons in its structure, we can expect to see five unpaired spins in Fe complexes. This is correct for [FeF6]3-, but [Fe (CN)6]3- has only one unpaired electron, rendering it a weaker magnet. The properties of the ligands can be used to clarify this pattern. Since we expect CN to have a stronger electric field than F, the energy differences in the d orbitals for the cyanide complex should be greater.

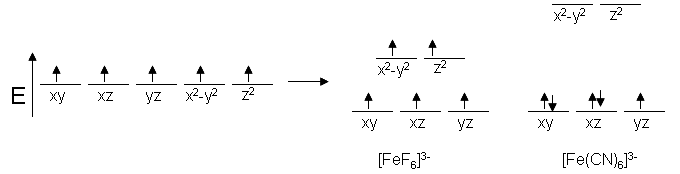
Crystal field theory splitting diagram

Electronic properties of ligands influence d orbital splitting in this example. This diagram illustrates the difference between low-spin and high-spin electrons.

There must be some kind of energy gain of providing paired spins for our cyanide complex in order for this to make sense. That is, the difference in energy levels must be greater than the repulsive energy of electron pairing. Since systems aim for the lowest possible energy, electrons can pair up before progressing to higher orbitals. Low spin refers to an electron moving down before pairing, while high spin refers to an electron moving up before pairing.

Since none of the ligands are in the plane of the orbitals, tetrahedral complexes have inherently weaker splitting. As a result, they have either too many or too few d electrons to be concerned with low or high spin. Transition metals with eight d electrons, on the other hand, are the only source of square planar compounds. [Pt (NH3)3Cl] +, [PtCl4]2-, and [Ni (CN)4]2- are all diamagnetic.

We may infer that square planar compound are always low spin and thus weakly magnetic since this covers the entire range of ligand strength. The condition is more complicated in bi- and polymetallic complexes where the individual centres have an odd number of electrons or the electrons are high-spin.

The electrons may pair, resulting in a weak magnet, or they may reinforce each other if the two (or more) metal centres interact. The two (or more) individual metal centres behave as if they are two different molecules when there is no interaction.

Q10) Define Latimer diagram.

A10)

For substances with multiple oxidation states, Latimer diagrams are the simplest and most compact way to describe electrochemical equilibria. For successive redox reactions (from highest to lowest oxidation state), electrochemical potential values are written under normal conditions in either strong acid ([H+] = 1 M, pH 0) or strong base ([OH-] = 1 M, pH 14). In a Latimer diagram, the oxidation states of successive substances may differ by one or more electrons. Difference determines the oxidation state of the element undergoing redox; we give the oxygen atoms an oxidation state of -2 and the hydrogen atoms an oxidation state of +1.

Example:

Mn in Acid

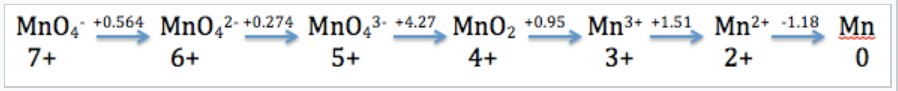
The Latimer diagram for Mn illustrates its standard reduction potentials (in 1 M acid) in oxidation states from +7 to 0.

The Latimer diagram compresses into shorthand notation all the standard potentials for redox reactions of the element Mn. For example, the entry that connects Mn2+ and Mn gives the potential for the half-cell reaction:

Mn2+(aq) +2e−⟶Mn(s) E1/2° = -1.18V

And the entry connecting Mn4+ and Mn3+ represents the reaction:

MnO2(s)+4H+(aq) +e−⟶Mn3+(aq) +2H2O(l)

MnO2(s)+4H(aq)++e−⟶Mn(aq)3++2H2O (l), E1/2° = +0.95V

We can also calculate values for multi-electron reactions by first adding ΔG°(=-nFE°) values and then dividing by the total number of electrons

For example, for the 5-electron reduction of MnO4- to Mn2+, we write

Eo=1(0.564) +1(0.274) +1(4.27) +1(0.95) +1(1.51)5=+1.51V

And for the three-electron reduction of MnO4-(aq) to MnO2(s),

Eo=1(0.564) +1(0.274) +1(4.27)3=+1.70V

Remember to divide by the number of electrons involved in the oxidation number change (5 and 3 for the above equations).

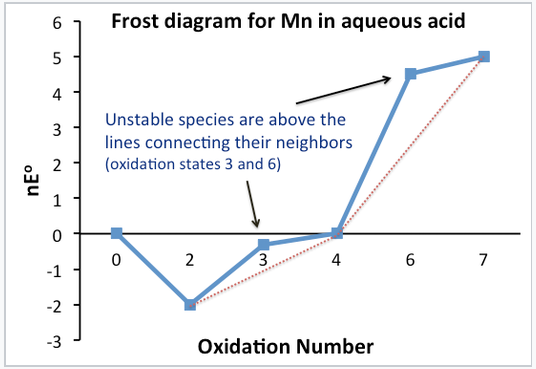
Q11) Define Frost diagram.

A11)

We plot G°F (= nE°) vs. Oxidation number in a Frost diagram. The nE° value for the zero-oxidation state is zero.

The plot clearly distinguishes between stable and unstable oxidation states. The line connecting unstable compounds to their neighbours is higher on the plot than the line connecting stable compounds to their neighbours. This is only a graphical representation of how we used the Latimer diagram to figure out which oxidation states were stable and which were unstable.

The slope of the line connecting the two species on a Frost diagram determines the normal potential for any electrochemical reaction. On the Frost diagram, the line connecting Mn3+ and MnO2 has a slope of +0.95, which is the normal potential of MnO2 reduction to Mn3+. In the Latimer diagram for Mn, this is the number written above the arrow. By connecting the dots in a Frost diagram, multielectron potentials can be easily measured.

Q12) Explain Trends in Transition Metal Oxidation States.

A12)

For several transition metals, the similarity in ionisation energies and the relatively small rise in successive ionisation energies leads to the formation of metal ions of the same charge. This leads to extensive horizontal chemistry similarities, which are most evident in the first-row transition metals, lanthanides, and actinides. As a result, all first-row transition metals except Sc form stable compounds containing the 2+ ion, and all except Zn form stable compounds containing the 3+ ion due to the slight difference between their second and third ionisation energies. Many transition metals have several oxidation states divided by a single electron due to the slight rise in successive ionisation energies. Manganese, for example, forms compounds in all oxidation states from 3 to 7. High oxidation states become increasingly less stable for the elements on the right side of the d block as ionisation potentials increase slowly but steadily over a chain. Many, if not most, transition metal compounds are paramagnetic, with one to five unpaired electrons, due to the existence of several oxidation states separated by a single electron. This behaviour contrasts sharply with that of the p-block elements, where the presence of two oxidation states separated by two electrons is typical, making nearly all p-block element compounds diamagnetic.

Q13) Define Ionization Enthalpy.

A13)

The amount of energy required to remove a valence electron from an element is referred to as ionisation enthalpy. The ionisation potential of an element is proportional to the effective nuclear charge acting on electrons. This is why transition elements' ionisation enthalpies are normally higher than those of s-block elements.

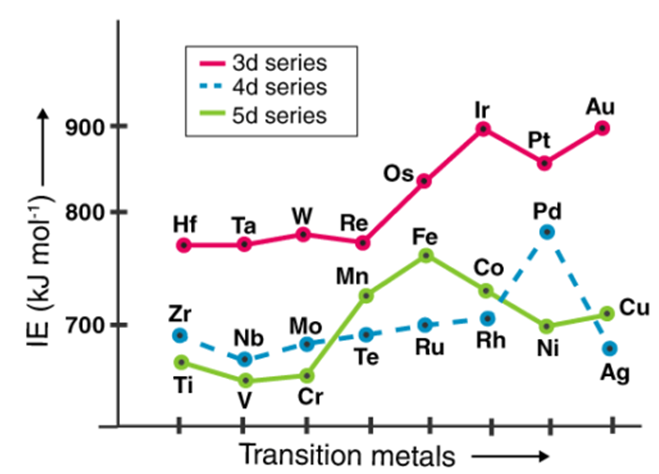
In certain ways, an element's ionisation energy is linked to its atomic radius. Ionization enthalpies are higher in atoms with smaller radii than in atoms with larger radii. The transition metals' ionisation energies increase as they move down the lines (due to the increase in atomic number).