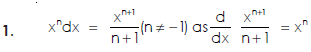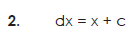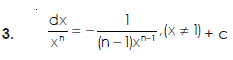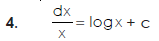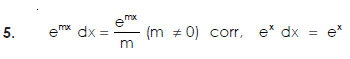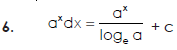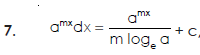Unit 3
Calculus II
Q1) Explain integration.
A1)
Integration is the reverse process of differentiation. It is also called annti-differentiation.
Integration calculus has its own application in economics, Engineering, Physics, Chemistry, business, commerce, etc.
The integral of a function is denoted by the sign 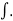
Let the function is y = f(x),
Its derivative is-
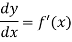
Then
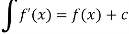
Where c is the arbitrary constant.
For example,
A function,

Then, its derivative-
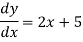
Or
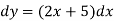
Then-
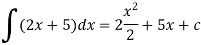
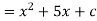
Here c is an arbitrary constant.
Some fundamental integrals-
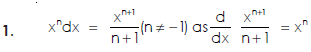
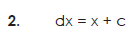
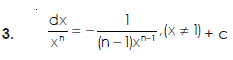
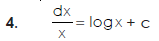
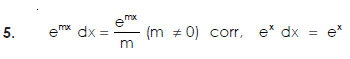
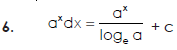
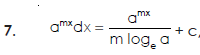
Q2) Find the integral of- 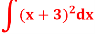
A2) We know that-
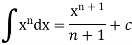
Then
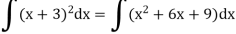

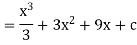
Q3) Find the integral.

A3)
We know that-
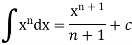
Then
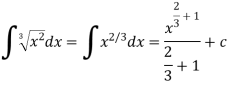
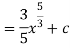
Q4) Evaluate-
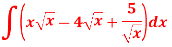
A4)
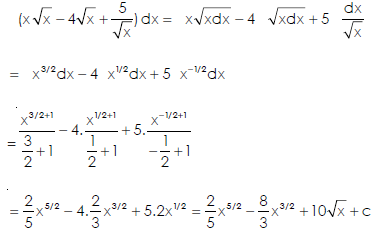
Q5) Evaluate the following integral-
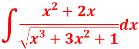
A5)
Let us suppose,
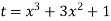
Then-
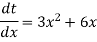
Or
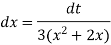
Substituting –
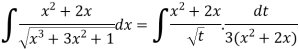
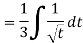
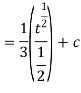
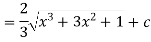
Q6) Evaluate the following integral-
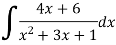
Sol.
Let us suppose-
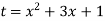
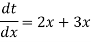
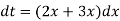
Now
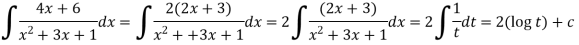
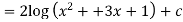
Q7) Evaluate-
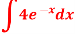
A7)
Let,
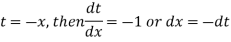
Now substituting-

Q8) Evaluate-

A8)
Here according to ILATE,
First function = log x
Second function = 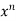
We know that-
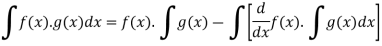
Then-
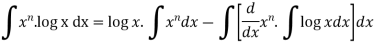
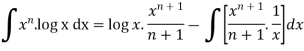
On solving, we get-
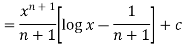
Q9) Explain simple integration.
A9)
Some standard form of simple integration-

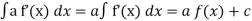
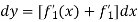
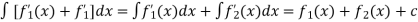
The integration of  will be as follows-
will be as follows-
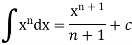
Q10) Write some fundamental integrals.
A10)