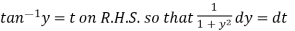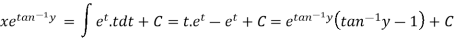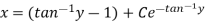Unit - 1

Differential equations and mathematical models

Q1) What are differential equations?

A1)

A differential equation is an equation involving an unknown function and its derivatives.

For example:      y + = 8x and

A differential equation with only single independent variable is known as ordinary differential equation.

Or in other words- A differential equation is an ordinary differential equation if the unknown function depends on only one independent variable

Notation: the expression y’, y’’ , y’’’ …….yⁿ are used to represent derivatives.

Here, y’ = 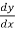 ,         y’’ =  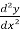    and so on.

1st order linear differential equations-

A 1st order linear differential equations is linear when it is given in the form below-

,

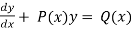Where P(x) and Q(x) are the functions of x

Q2) Define order and degree of differential equations.

A2)

Order: The order is the highest derivative:

For example:  (1) 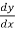   + y³ = 8x ,  here it has only first derivative so its ‘first order’.

(2) 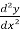 + y³ = 7x ,  here it has a second derivative so its ‘second order’  and so on.

Degree:  Degree is known as the exponent of higher derivative.

For example:  (1)   )² + y³ = 8x, here its highest derivative has an exponent of 2, it is a first order second degree ordinary differential equation.

(2)  ) + y³ = 8x,  here in this example its higher derivative has no exponent, so we can say that this is the third order and first degree ordinary differential equation.

Q3) From the differential equation by eliminating arbitrary constant.

y² = Ax² + Bx² + C

A3)

We will differentiate the given function,  we get

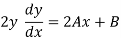
Again differentiate,

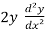+ 2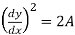

On differentiating again, we get

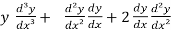= 0 

Q4) Find the general solution of x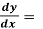2 – 4x³

A4)

Rearranging the given equation:

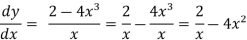
On integrating both sides,

y = 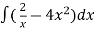= 2 In x - 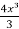+ c

This is the general solution.

Q5) Find the general solution of   = 3 + 2y

A5)

Here,   = 3 + 2y gives,

,

Integrating both sides,

,

By substitution,  u = (3 +2y),

X =  In(3 + 2y) + c.

Q6) Solve the equation 4xy 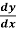= y² - 1

A6)

On separating variables, we get

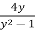() dy  = dx

=  

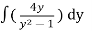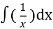
Using substitution, u = y² - 1

2In(y² - 1) = In x + c.

Q7) What are exact differential equations?

A7)

An exact differential equation is formed by differentiating its solution directly without any other process,

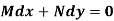
Is called an exact differential equation if it satisfies the following condition-

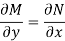
Here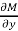
is the differential co-efficient of M with respect to y keeping x constant and
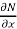is the differential co-efficient of N with respect to x keeping y constant.

Q8) Solve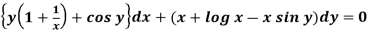

A8)

Here M = 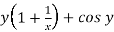and N =

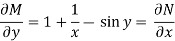
Then the equation is exact and its solution is-

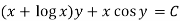
Q9) Determine whether the differential function ydx –xdy = 0 is exact or not.

A9)

Here the equation is the form of  M(x , y)dx + N(x , y)dy = 0

But, we will check for exactness,

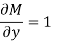
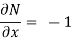
These are not equal results, so we can say that the given diff. Eq. Is not exact.

Q10) Solve-

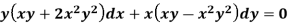
A10)

Here we have-

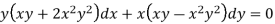
Now divide by xy, we get-

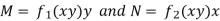
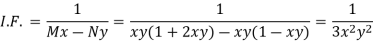
Multiply (1) by , we get-

Which is an exact differential equation-

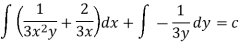
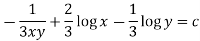
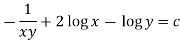
Q11) Solve-

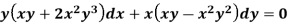
A11)

We can write the equation as below-

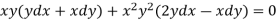
Now comparing with-

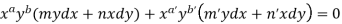
We get-

a = b = 1, m = n = 1, a’ = b’ = 2, m’ = 2, n’ = -1

I.F. =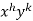

Where-

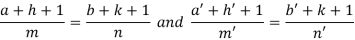
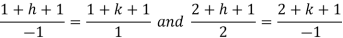
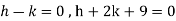
On solving we get-

h = k = -3

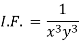
Multiply the equation by , we get-

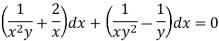
It is an exact equation.

So that the solution is-

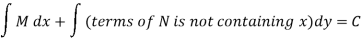
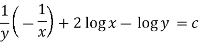
Q12) Solve 

A12)

Separate the variables,

dx

Integrate on both sides,

=  

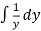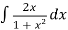
Let, u = 1 + x² ,   so ,  du = 2x dx,

=  

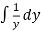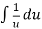
In(y) = In(u) +C

Let, C = In(k)         In(y) = In(u) +In(k)

y = uk

Put, u = 1 + x²

y = k(1 + x²)

Q13) Solve the equation:

A13)

Here the function is,

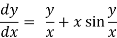
We will put  y =vx as mentioned above , so that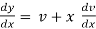 

v +

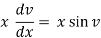
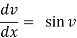
Separate the variables,

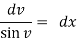
Cosec v dv =  dx

On integrating,

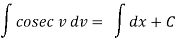
Log tan = x +C

Log tan 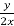= x +C  

Q14) Solve ( x+ 2y) ( dx – dy) =  dx + dy

A14)

On solving the given eq.

Now, here, we see that  

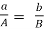     (case-2)

So we put,      x +2y = z

1 +2

Here original eq. Becomes

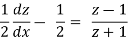
On solving it becomes,

() dz  =  dx

Now integrate,

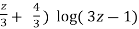(=  x + C

Put the value of z,  it becomes,

3( x+2y) + 4log ( 3x +6y – 1) = 9x + 9C

Q15) Solve-

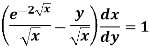
A15)

We can write the given equation as-

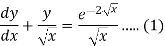
So that-

I.F. =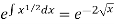

The solution of equation (1) will be-

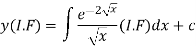
Or

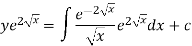
Or

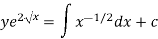
Or

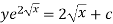
Q16) Solve sin x )

A16)

Here we have,

Sin x )

which is the linear form,

Now,

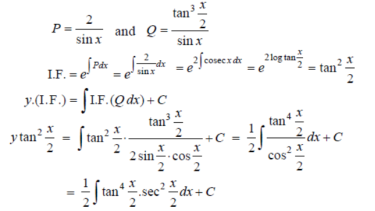
Put tan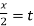   so that  sec² dx =  dt, we get

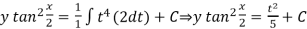
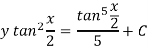
Which is the required solution.

Q17) What is Bernoulli’s equations and how do we solve it?

A17)

The equation

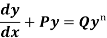
Is reducible to the Leibnitz’s linear equation and is usually called Bernoulli’s equation.

Working procedure to solve the Bernoulli’s linear equation-

Divide both sides of the equation –

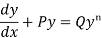
By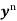, so that

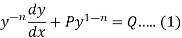
Put 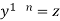so that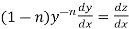

Then equation (1) becomes-

)

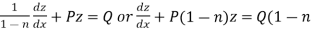Here we see that it is a Leibnitz’s linear equations which can be solved easily.

Q18) Solve

A18)

Here given,

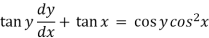
Now let z = sec y, so that dz/dx = sec y tan y dy/dx

Then the equation becomes-

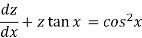
Here,

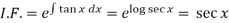
Then the solution will be-

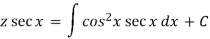
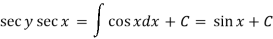
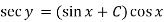
Q19) Solve-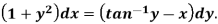

A19)

Here given-

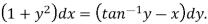
We can re-write this as-

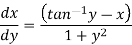
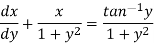
Which is a linear differential equation-

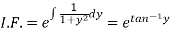
The solution will be-

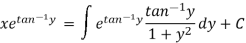
Put