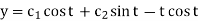Unit - 3

General solution of homogeneous equation of second order

Q1) What are homogeneous equation of second order differential equations?

A1)

A homogeneous linear ordinary differential equation with constant coefficients is an ordinary differential equation in which coefficients are constants, all terms are linear, and the entire differential equation is equal to zero,

The form of second order linear differential equation with constant coefficients is,

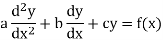
Where a,b,c are the constants.

Let, aD²y+bDy+cy = f(x),    where d² = ,  D =

∅(D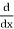)y = f(x), where ∅(D)y = aD²y+bDy+cy

Here first we solve,  ∅(D)y = 0, which is called  complementary function(C.F)

Then we find particular integral (P.I)

P.I. = 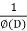f(x)

General solution = C.F. +P.I.

Q2) Define differential operators?

A2)

D stands for operation of differential i.e.

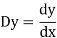
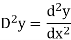
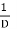stands for the operator of integration.

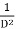stands for operation of integration twice.

Thus,

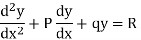
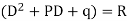
Q3) Solve (4D² +4D -3)y =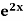 

A3)

Auxiliary equation is 4m² +4m – 3 = 0

We get,             (2m+3)(2m – 1) = 0

m =   ,

Complementary function:  CF is  A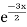+ B

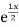
Now we will find particular integral,

P.I. = 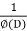f(x)

=   .

=    .

=    .

=   . =   .

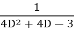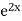
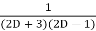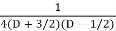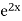
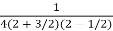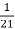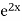
General solution is y =  CF + PI

= A+ B  .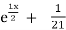

Q4) Solve

A4)

Given,

Here Auxiliary equation is

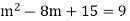
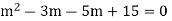
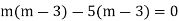
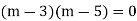
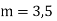
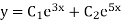
Q5) Solve

A5)

Auxiliary equation is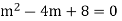

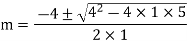
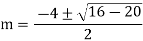
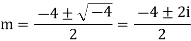
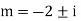
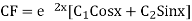
Q6) Solve

A6)

Given,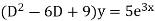

Auxiliary equation is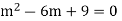

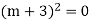
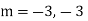
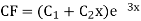
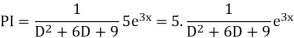
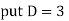
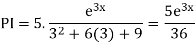
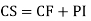
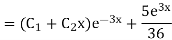
Q7)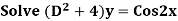

A7)
Auxiliary equation are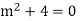

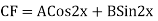
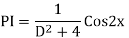
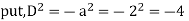
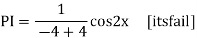
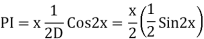
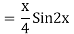
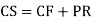
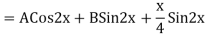
Q8) Solve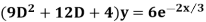

A8)

The AE is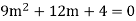

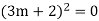
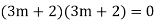
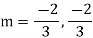
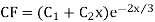
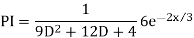
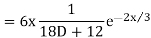
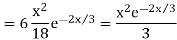
Complete solution y= CF + PI

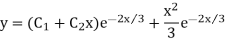
Q9) Solve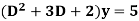

A9)

The AE is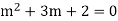

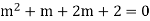
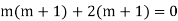
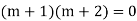
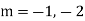
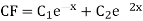
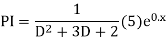
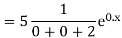
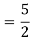
Complete solution = CF + PI

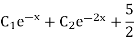
Q10) Solve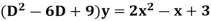

A10)

The AE is

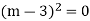
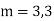
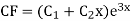
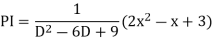
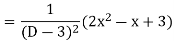
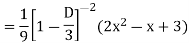
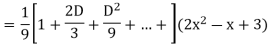
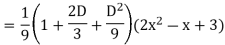
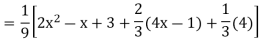
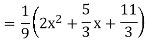
Complete solutio0n is y= CF + PI

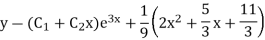
Q11) Solve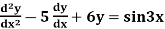

A11)

Given equation in symbolic form is

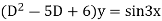
Its Auxiliary equation is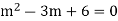

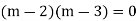
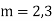
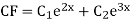
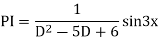
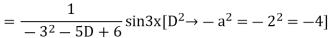
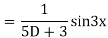
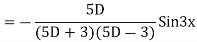
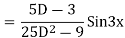
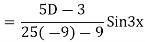
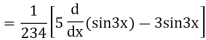
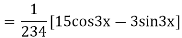
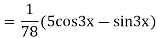
Complete solution is y= CF + PI

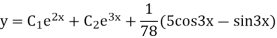
Q12) Find the PI of (D2-4D+3)y=ex cos2x

A12)

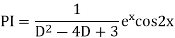
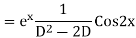
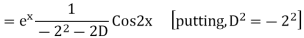
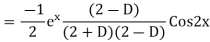
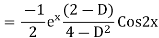
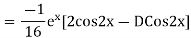
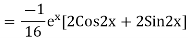
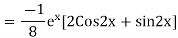
Q13) Determine whether the following two functions are independent or dependent?

f(x) = x,  g(x) = x³

A13)

Here we will make wronskian as follows,

W = 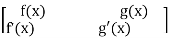= f(x).g’(x) – f’(x).g(x)

W = =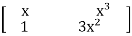   

=  3x³ - x³

Here we can see that W is not equals to zero so that these two functions are independent.

Q14) Solve 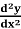– y = 2x² - x – 3

A14)

First we find general solution:

The characteristic function is:   r² - 1 = 0

( r-1)(r+1) = 0

R = 1, -1

General solution is - A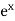 + B,

Now, let        

y = ax² + bx +c

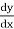  = 2ax + b

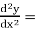2a

Put these value in 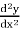 – y = 2x² - x – 3,

2a – (ax² + bx +c) = 2x² - x – 3

2a – ax² - bx - c = 2x² - x – 3

Now compare coeff.

Coeff. Of x² ,    a = -2

Coeff. Of x ,      b = 1

Constant coeff.

2a – c = -3,       c =-1

So the particular solution will be,

y =  -2x² + x – 1

Complete solution is,

y = A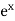 + B- 2x² + x – 1

Q15) Solve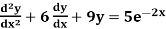

A15)

Here cf is  r² -6r +9 = 0

(r – 3)² = 0

r = 3

So the general solution is - A + B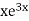

Now we will find particular solution,

Lets, y =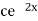

=

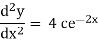
Substitute these values,

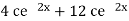+ 9 =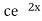

=

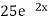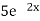
C = 1/5

The particular solution is,

y = 1/5

Complete solution,

y = - A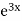 + B 1/5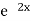

Q16) Solve the following DE by using variation of parameters-

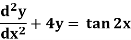
A16)

We can write the given equation in symbolic form as-

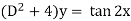
To find CF-

It’s A.E. Is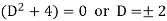

So that CF is-     

To find PI-

Here

Now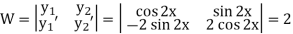

Thus PI = 

= 

= 

= 

= 

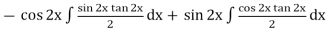
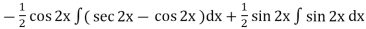
So that the complete solution is-

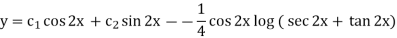
Q17) Find the P.I. Of (D + 2)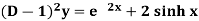

A17)

P.I. =

Now we will evaluate each term separately-

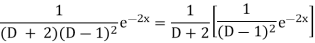
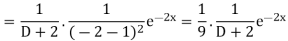
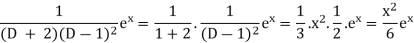
And

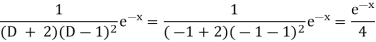
Therefore-

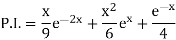
Q18) Find the P.I. Of (D + 1) (D + D’ – 1)z = sin (x + 2y)

A18)

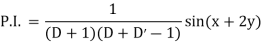
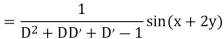
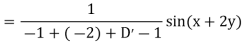
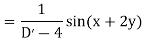
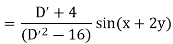
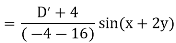
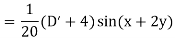
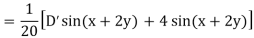
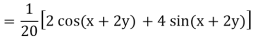
Q19) Solve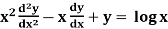

A19)

As it is a Cauchy’s homogeneous linear equation.

Put

Then the equation becomes [D(D-1)-D+1]y = t   or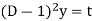

Auxiliary equation-

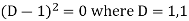
So that-

C.F.=

Hence the solution is- , we get-

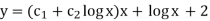
Q20) Solve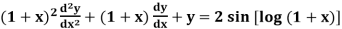

A20)

As we see that this is a Legendre’s linear equation.

Now put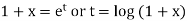

So that-

And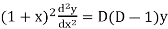

Then the equation becomes- D (D – 1)y+ Dy + y = 2 sin t

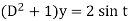
Its auxiliary equation is-

And particular integral-

P.I. =

Note -

Hence the solution is -