Unit - 2

Magnetic Field

Q1) A 100 turn closely wound circular coil of radius 10 cm carries a current of 3.2A

(a) What is the field at the centre of the coil?

(b) What is the magnetic moment of this coil? The coil is placed in a vertical plane and is free to rotate about a horizontal axis which coincides with its diameter. A uniform magnetic field of 2T in the horizontal direction exists such that initially the axis of the coil is in the direction of the field. The coil rotates through an angle of 90° under the influence of the magnetic field.

(c) What are the magnitudes of the torques on the coil in the initial and final position?

(d) What is the angular speed acquired by the coil when it has rotated by 90°? The moment of inertia of the coil is 0.1 kg m2.

A1)

(a)

We know

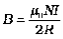
Here, N = 100; I = 3.2 A, and R = 0.1 m. Hence,

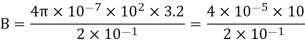
(using π×3.2=10)

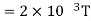
The direction is given by the right-hand thumb rule.

(b) The magnetic moment is given by

m = N I A = NIπr2 = 100 × 3.2 × 3.14 × 10–2 = 10 A m2

The direction is once again given by the right-hand thumb rule.

(c) = m× B = m B sinθ

Initially, θ= 0. Thus, initial torque i = 0.

Finally, θ = π/2 (or 90º).

Thus, final torque f = m B = 10 × 2 = 20 N m.

(d) From Newton’s second law

I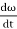 = m B sinθ

Where I is the moment of inertia of the coil. From chain rule

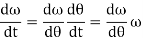
Using this

I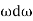 = m B sin dθ

Integrating from θ= 0 to θ = π/2 

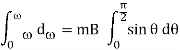
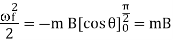
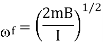
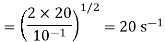
Q2) A long solenoid is fabricated to closely winding wire of radius 0.5mm over a cylindrical frame, so that the successive turns nearly touch each other. The magnetic field at the centre of solenoid, if it carries a current of 5A is?

A2)

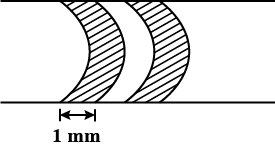
Figure: Long solenoid

The turns of wire on the solenoid are shown in figure.

Number of turns in 1mm = 1,

n = 1000 turns / m

Therefore, B = μ0nI = 4π × 10−7 × 103 × 5 = 2π × 10−3 T

Q3) Derive Bio-Savart law?

A3)

The Biot Savart Law is an equation describing the magnetic field generated by a constant electric current. It relates the magnetic field to the magnitude, direction, length, and proximity of the electric current. Biot–Savart law is consistent with both Ampere’s circuital law and Gauss’s theorem. The Biot-Savart law is fundamental to magnetostatics, playing a role similar to that of Coulomb’s law in electrostatics.

The Biot-Savart law can be stated as:

Hence   or      ……….(1)

Where, k is a constant, depending upon the magnetic properties of the medium and system of the units employed.

In the SI system of unit,

……….(2)

Therefore, the final Biot-Savart law derivation is,

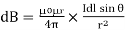        ……….(3)

DERIVATION

Let us consider a long wire carrying a current I and also consider a point p in the space. The wire is presented in the picture below, by red colour.

Let us also consider an infinitely small length of the wire dl at a distance r from the point P as shown. Here, r is a distance-vector which makes an angle θ with the direction of current in the infinitesimal portion of the wire.

If you try to visualize the condition, you can easily understand the magnetic field density at point P due to that infinitesimal length dl of the wire is directly proportional to current carried by this portion of the wire.

As the current through that infinitesimal length of wire is the same as the current carried by the whole wire itself, we can write,

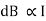        ……….(4)

The magnetic field density at that point P due to that infinitesimal length dl of wire is inversely proportional to the square of the straight distance from point P to the center of dl. Mathematically we can write this as,

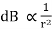         ……….(5)

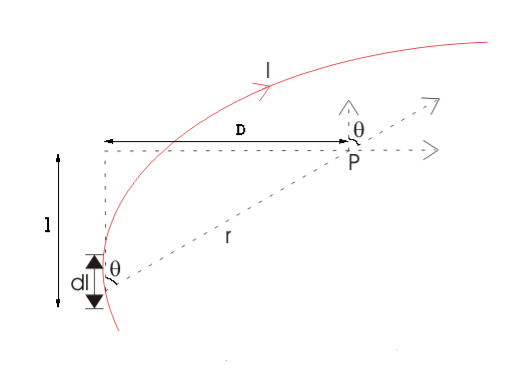
Figure: Biot-Savart law

As θ be the angle between distance vector r and direction of current through this infinitesimal portion of the wire, the component of dl directly facing perpendicular to the point P is dlsinθ,

Hence, 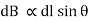……….(6)

Now, combining these three statements, we can write,

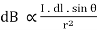   ……….(7)

This is the basic form of Biot Savart’ s Law

Now, putting the value of constant k (which we have already introduced at the beginning of this article) in the above expression, we get

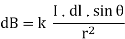
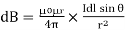      ……….(8)

Here, μ0 used in the expression of constant k is absolute permeability of air or vacuum and its value is 4π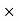10-7 Wb/ A-m in the SI system of units. μr of the expression of constant k is the relative permeability of the medium.

Q4) What do you meant by divergence and curl of magnetic field?

A4)

The static electric field E(x; y; z) such as the field of static charges obeys equations

∇. E =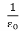ρ         ………..(3.2a)

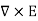= 0         ………..(3.2b)

The static magnetic field B(x; y; z) such as the field of steady currents obeys different equations

=0          ………..(3.2c)

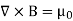J         ………..(3.2d)

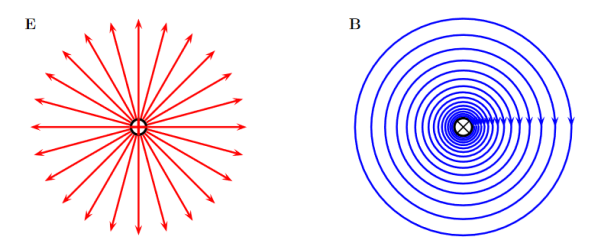
Figure: Electric and Magnetic Fields

Due to this difference, the magnetic field of long straight wire looks quite different from the electric field of a point charge or a linear charge.

Later in these notes we will derive equations (3.2c) and (3.2d) from the Biot Savart Law.

Let us explore some of their consequences. The zero-divergence equation (3.2c) is valid for any magnetic field, even if it is time-dependent rather than static. Physically, it means that there are no magnetic charges, otherwise we would have ∇.B mag instead of ∇ B= 0.

Consequently, the magnetic field lines never begin or end anywhere in space; instead they form closed loops or run from in infinity to in infinity.

The integral form of eq. (3.2c) follows by the Gauss theorem: the magnetic flux through any closed surface is zero

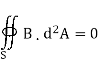
Consequently, any open surfaces S1,S2,……..spanning the same loop L have the same magnetic flux through them, for example

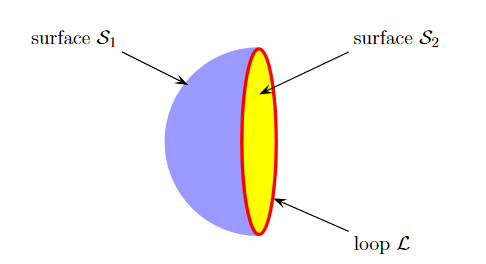
Figure: Open  Surface

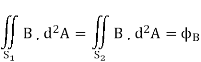
We shall this identity very useful for stating the Faraday's Law of magnetic induction.

Now consider the curl equation

J 

Which is the differential form of the Ampere's Law. The integral form of the Ampere's Law obtains by the Stokes' theorem: For any closed loop L and any surface S spanning that loop,

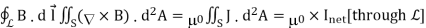………..(3.2e)

Where Inet [through L] is the net electric current flowing through the loop L. The integral form (3.2e) of the Ampere's Law is particularly convenient when the current flows through a wire or several wires; in this case all we need is to check which wire goes through the loop L and which does not, then add up the currents in the wires that do go through L and mind their directions. But it is also convenient for the volume currents flowing through thick conductors or for current sheets flowing on surfaces.

As we know that the Ampere's Law applies only to the magnetic fields of steady currents. Otherwise, we need to use the more general Maxwell {Ampere Law, with an extra term for the time-dependent electric field.

For simplicity we say that the original Ampere's Law is simply mathematically inconsistent unless the electric current has zero divergence,∇J= 0.Indeed, the left hand side of the curl equation 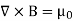J always have zero divergence.

∇. () = 0

So we cannot have () = 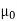J unless the RHS has zero divergence as well,

∇.J= 0. As to the integral form (3.2e) of the Ampere's Law, we need a divergence-less current density J to make sure that the net current through the loop L is the same for any surface S spanning the loop; otherwise we simply cannot de ne the net current through L

Q5) Determining whether a field F(x,y) = x2y, y-xy2 is Magnetic?

A5)

It is possible for F(x,y) = x2y, y-xy2 to be a magnetic field, if its divergence would be zero.

If F were magnetic, then its divergence would be zero. The divergence of F is

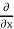(x2y)+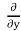(y-xy2) = 2xy +1 -2xy =1

And therefore F cannot model a magnetic field

The divergence of vector field F(x,y) = x2y, y-xy2 is one, so it cannot model a magnetic field.

Q6) Determine if the vector field F =  yz2i + (xz2 + 2) j + (2xyz - 1) k is conservative. 

A6)

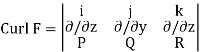
Therefore the given vector field F is conservative.

Q7) Find the magnetic field where vector potential associated with field is given by

A(x, y, z) = 3x2i + 2zj – xk.

A7)

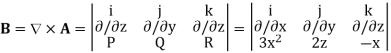
Q8) Find the magnetic field where vector potential associated with field is given by A= (x + y + z, x − y – z, x2 + y2 + z2)?

A8)

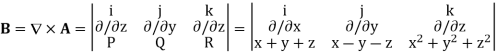
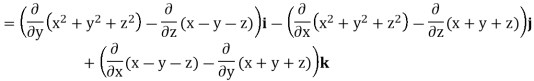
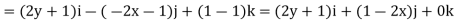
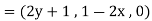
Q9) Derive equation of divergence and curl of magnetic field?

A9)

In general, when dealing with a current density J(x’) instead of a current I so (equation 1 of article 3.1) for the magnetic induction B(x) takes the form

     ……….(1)

Equation (1) can be expressed differently, since

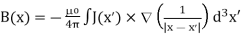    ……….(2)

But since

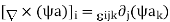
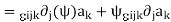
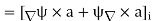    ……….(3)

We get

……….(4)

If we set =1/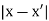 and a=J(x’), with J(x’)=0, since the current density is independent of x. It follows from equation (3) that the magnetic induction is divergence-less

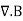=0          ……….(5)

Equation (5) is a mathematical statement on the inexistence of magnetic monopoles.

Taking the curl of the B field,

Using this identity 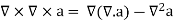we get

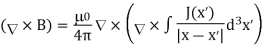
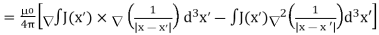……….(5)

Solving this we get

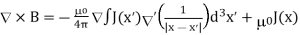………..(6)

This equation can be further simplified by using the divergence theorem

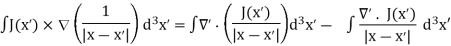
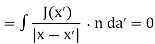
Since 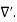J(x’)=0  and the surface of integration extends over all space where the integrand vanishes. We therefore find that

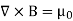J         ………..(7)

If we evaluate the integral on both sides of equation (7) over an open surface S of contour C (see Figure 13), we find

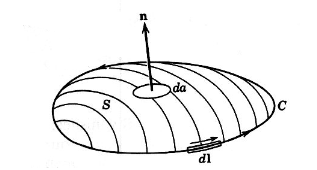
Figure: A magnetic induction is induced around a circuit C by a current density J through the open surface S bounded by C.

And using Stokes’ theorem

………..(8)

c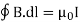         ………..(9) 

Where I is the current passing through the surface. Equations (8) and (9) are mathematical representations of Ampère’s Law.

Q10) What is vector potential?

A10)

Since we know from equation (3.2c) that the divergence of the magnetic induction is zero, it follows that the B field can be expressed as the curl of another vector field. Introducing the potential vector A(x), we can write

B(x) = 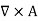(x)         ………..(1) 

Referring to equation (4) of article 3.2, we find that the most general equation for A is

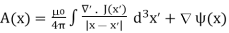    ………..(2) 

Where  is some arbitrary scalar function. Inserting equation (2)  into equation (3.2d)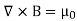J             

We get

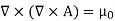J        ………..(3) 

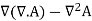= J        ………..(4) 

But because of the freedom brought by the presence of 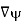in the equation defining the potential vector (i.e., equation (2)), we can choose 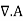to suit our needs. Using the so-called Coulomb gauge, which sets 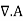we write

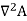= J         ………..(5) 

Just as

………..(6) 

Is the solution to the Poisson equation (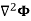 = 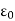/ρ), the following is the solution to equation

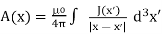………..(7)

Equation (7) is valid in general, as we can set =cste due to the fact that 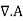=0

Reduces to =0 from equation (2). This is because

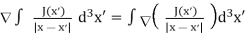………..(8)

Since  J(x’)=0. Equation (8).

Q11) Calculate vector potential for a given magnetic field using stokes’ theorem?

A11)

The magnetic field B(r) determines the vector potential A(r) only up to a gradient of an arbitrary scalar field 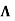(x; y; z). Indeed, the vector potentials A (x; y; z) and

A’(x,y,z) = A(x,y,z) + 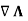(x; y; z)      ………..(9)

Have the same curl everywhere, so they correspond to the same magnetic field,

B’(x,y,z) = 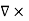A’(x,y,z) +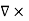 (x; y; z) = B(x,y,z) +0  ………..(10)

The relations (9) between different vector potentials for the same magnetic field are called the gauge transforms.

Despite ambiguity of the vector potential itself, some of its properties are gauge invariant i.e., the same for all potentials related by gauge transforms. For example, for any closed loop L, the integral.

………..(11)

Is gauge invariant; indeed,

………..(12)

Physically, the integral (11) in the magnetic flux through the loop L. Indeed, take any surface S spanning the loop L; by the Stokes' theorem,

………..(13)

We may use eq. (13) to easily find the vector potential for a magnetic field which has some symmetries. For example, consider the uniform magnetic field B=B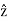 inside a long solenoid. By the rotational and translational symmetries of the solenoid, we expect.

A(s, ϕ, z) = A(s)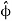        ………..(14)

While the magnitude A(s) follows from eq. (13): Take a circle of radius s < R solenoid, then

     ………..(15)

While the magnetic flux through that circle is

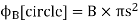      ………..(16)

Hence

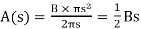………..(17)

In Cartesian coordinates, the vector potential becomes

     ………..(18)

Which makes it easy to verify

………..(19)

Eq. (18) gives the vector potential inside the long solenoid. Outside the solenoid, the magnetic field is negligible, but the flux through a circle of radius s > R solenoid is non-zero due to the flux inside the solenoid. Thus,

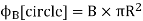………..(20)

And hence

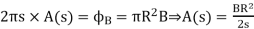 ………..(21)

In vector notations

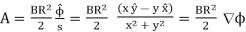………..(22)

Which agrees with the zero magnetic field outside the solenoid,

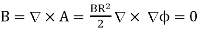   ………..(23)

Q12) Derive Ampere’s Law from biot savart law?

A12)

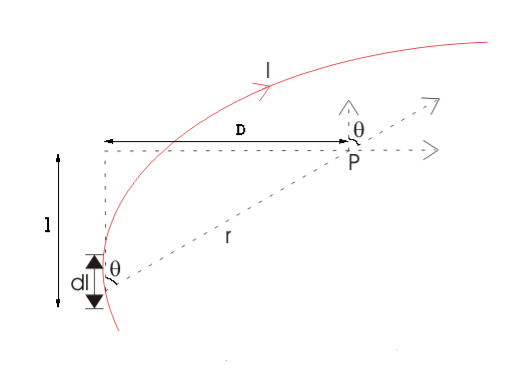
Figure: Biot-Savart law

Biot-Savart law is given by

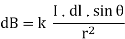
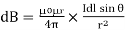      ……….(8)

Here, μ0 used in the expression of constant k is absolute permeability of air or vacuum and its value is 4π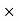10-7 Wb/ A-m in the SI system of units. μr of the expression of constant k is the relative permeability of the medium.

Now, flux density(B) at the point P due to the total length of the current-carrying conductor or wire can be represented as,

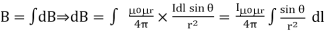 ……….(9)

If D is the perpendicular distance of the point P from the wire, then

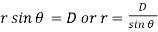               ……….(10)

Now, the expression of flux density B at point P can be rewritten as,

Again              ……….(11)

As per the figure above,

Therefore, 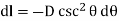              ……….(12)

Finally, the expression of B comes as,

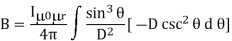
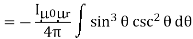
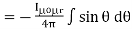……….(13)

This angle θ depends upon the length of the wire and the position of the point P. Say for a certain limited length of the wire, angle θ as indicated in the figure above varies from θ1 to θ2. Hence, magnetic flux density at point P due to the total length of the conductor is,

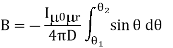
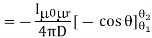
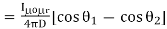      ……….(14)

Let’s imagine the wire is infinitely long, then θ will vary from 0 to π that is θ1 = 0 to θ2 = π. Putting these two values in the above final expression of Biot Savart law, we get,

 ……….(15)

This is nothing but the expression of Ampere’s Law.

Q13) A short wire of length 1.0 cm carries a current of 2.0 A in the vertical direction (as shown in figure ). The rest of the wire is shielded so it does not add to the magnetic field produced by the wire. Calculate the magnetic field at point P, which is 1 meter from the wire in the x-direction.

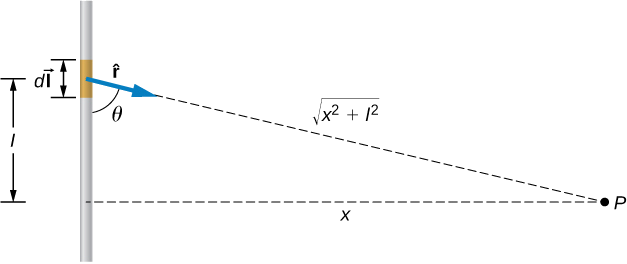
A13)

The angle between Δl⃗ and 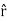is calculated from trigonometry, knowing the distances l and x from the problem:

θ=tan−1(1m/0.01m)=89.4o.

The magnetic field at point P is calculated by the Biot-Savart law

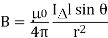
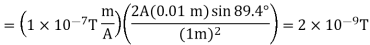
Q14) Derive a relation for magnetic field on the axis of a current carrying circular loop?

A14)

Firstly we shall evaluate the magnetic field due to a circular coil along its axis.

We assume that the current I is steady and that the evaluation is carried out in free space.

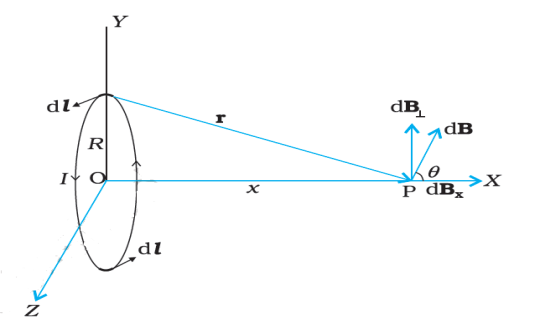
Figure: Magnetic field on the axis of a current carrying circular loop of radius R.

Figure depicts a circular loop carrying a steady current I. The loop is placed in the y-z plane with its centre at the origin O and has a radius R. The x-axis is the axis of the loop. Let x be the distance of P from the centre O of the loop.

Consider a conducting element dl of the loop. This is shown in Figure. The magnitude dB of the magnetic field due to dl is given by the Biot-Savart law

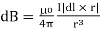  …… (1)

Now r2 = x2 + R2. Further, any element of the loop will be perpendicular to the displacement vector from the element to the axial point. For example, the element dl in is in the y-z plane, whereas, the displacement vector r from dl to the axial point P is in the x-y plane. Hence |dl × r|=r dl. Thus,

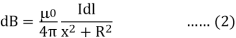
The direction of dB is shown in figure. It is perpendicular to the plane formed by dl and r. It has an x-component dBx and a component perpendicular to x-axis, dB⊥. When the components perpendicular to the x-axis are summed over, they cancel out and we obtain a null result.

For example, the dB⊥ component due to dl is cancelled by the contribution due to the diametrically opposite dl element, shown in figure. Thus, only the x-component survives. The net contribution along x-direction can be obtained by integrating dBx = dBcosθ over the loop.

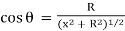 …… (3)

Thus from (2) and (3)

…… (4)

The summation of elements dl over the loop yields 2πR, the circumference of the loop. Thus, the magnetic field at P due to entire circular loop is

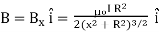…… (5)

As a special case of the above result, we may obtain the field at the centre of the loop. Here x = 0, and we obtain,

…… (6)

The direction of the magnetic field is given by right-hand thumb rule. Curl the palm of your right hand around the circular wire with the fingers pointing in the direction of the current. The right-hand thumb gives the direction of the magnetic field.

Q15) How current loop act as a magnetic dipole and also calculate its dipole moment?

A15)

Consider the elementary magnetic element: the current loop. We know that the magnetic field (at large distances) due to current in a circular current loop is very similar in behaviour to the electric field of an electric dipole.

The magnetic field on the axis of a circular loop, of a radius R, carrying a steady current I. The magnitude of this field is (Using equation (4))

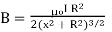…… (1)

And its direction is along the axis and given by the right-hand thumb rule . Here, x is the distance along the axis from the centre of the loop. For x >> R, we may drop the R2 term in the denominator. Thus

…… (2)

The area of the loop A = πR2. Thus

…… (3)

The magnetic moment m to have a magnitude IA,

m = IA. Hence

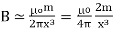…… (4)

The expression of Eq (4) is very similar to an expression obtained for the electric field of a dipole. The similarity may be seen if we substitute,

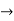μ0 1/Ɛ0

m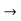pe (electrostatic dipole)

B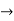E (electrostatic field)

We then obtain,

Which is precisely the field for an electric dipole at a point on its axis.

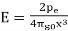…… (5)

It can be shown that the above analogy can be carried further. We had found that the electric field on the perpendicular bisector of the dipole is given by

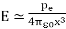…… (6)

Where x is the distance from the dipole. If we replace μ0 1/Ɛ0

And m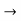pe in the above expression, we obtain the result for B for a point in the

Plane of the loop at a distance x from the centre. For x >>R,

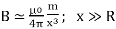…… (7)

The results given by Equations (4) and (7) become exact for a point magnetic dipole.

The results obtained above can be shown to apply to any planar loop: a planar current loop is equivalent to a magnetic dipole of dipole moment m = I A, which is the analogue of electric dipole moment p.

Note, however, a fundamental difference: an electric dipole is built up of two elementary units — the charges (or electric monopoles). In magnetism, a magnetic dipole (or a current loop) is the most elementary element. The equivalent of electric charges, i.e., magnetic monopoles, are not known to exist.

We have shown that a current loop (i) produces a magnetic field and behaves like a magnetic dipole at large distances, and (ii) is subject to torque like a magnetic needle. This led Ampere to suggest that all magnetism is due to circulating currents. This seems to be partly true and no magnetic monopoles have been seen so far. However, elementary particles such as an electron or a proton also carry an intrinsic magnetic moment, not accounted by circulating currents.

Q16) State and explain ACL?

A16)

Ampere’s circuital law (ACL) relates current to the magnetic field associated with the current. The magnetic field in space around an electric current is proportional to the electric current which serves as its source, just as the electric field in space is proportional to the charge which serves as its source.

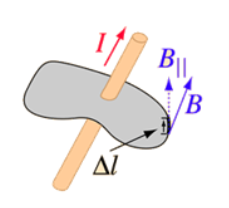
Figure: Ampere’s circuital law

Ampere's Law states that

The integral around a closed path of the component of the magnetic field tangent to the direction of the path equals μ0 times the current intercepted by the area within the path.

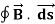= μ0I     ………..(1)

Ampere's Law tells that for any closed loop path, the sum of the length elements times the magnetic field in the direction of the length element is equal to the permeability times the electric current enclosed in the loop.

In order to apply Ampère’s Law

All currents have to be steady (i.e.do not change with time)

Only currents crossing the area inside the path are taken into account and have some contribution to the magnetic field

The total magnetic circulation is zero only in the following cases:-

• The enclosed net current is zero
• The magnetic field is normal to the selected path at any point
• The magnetic field is zero

Finally, be aware that the form of Ampère’s Law addressed here applies to magnetostatics only. In the presence of a time-varying electric field, the right side of ACL includes an additional term known as the displacement current.

Q17) What is Solenoid? Calculate magnetic field for it using ACL?

A17)

Consider a long solenoid. Long solenoid mean that the solenoid’s length is large compared to its radius. It consists of a long wire wound in the form of a helix where the neighbouring turns are closely spaced. So each turn can be regarded as a circular loop. The net magnetic field is the vector sum of the fields due to all the turns. Enamelled wires are used for winding so that turns are insulated from each other.

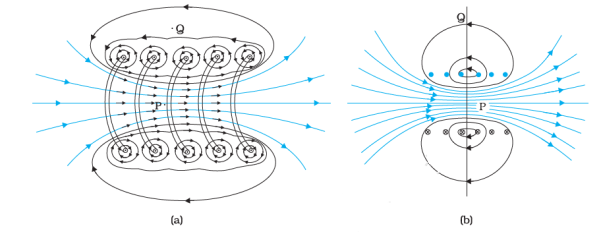
Figure: (a) The magnetic field due to a section of the solenoid which has been stretched out for clarity. Only the exterior semi-circular part is shown. (b) The magnetic field of a finite solenoid.

Figure displays the magnetic field lines for a finite solenoid. It is clear from the circular loops that the field between two neighbouring turns vanishes. We can see that the field at the interior mid-point P is uniform, strong and along the axis of the solenoid.

The field at the exterior mid-point Q is weak and moreover is along the axis of the solenoid with no perpendicular or normal component.  As the solenoid is made longer it appears like a long cylindrical metal sheet. The field outside the solenoid approaches zero. We shall assume that the field outside is zero. The field inside becomes everywhere parallel to the axis.

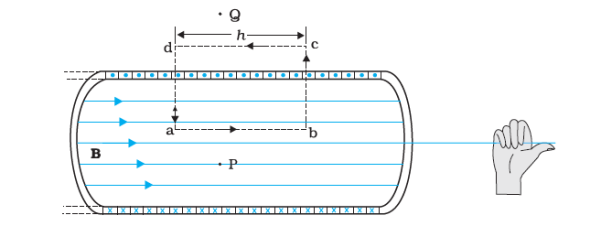
Figure: Magnetic field of a very long solenoid

Consider a rectangular loop abcd. Along cd the field is zero. Along transverse sections bc and ad, the field component is zero. Thus, these two sections make no contribution.

Let the field along ab be B. Thus, the relevant length of the loop is, L = h.

Let n be the number of turns per unit length, then

The total number of turns is = nh

The enclosed current is, Ie = I (nh) ……… (1)

Where I is the current in the solenoid.

From Ampere’s circuital law

BL = μ0Ie  ……… (2)

Bh = μ0I(nh)  ……… (3)

B = μ0nI       ……… (4)

The direction of the field is given by the right-hand rule. The solenoid is commonly used to obtain a uniform magnetic field.

Q18) What is Toroid? Calculate magnetic field for it using ACL? Also compare the results with solenoid.

A18)

The toroid is a hollow circular ring on which a large number of turns of a wire are closely wound. It can be viewed as a solenoid which has been bent into a circular shape to close on itself. It is shown in Figure below.

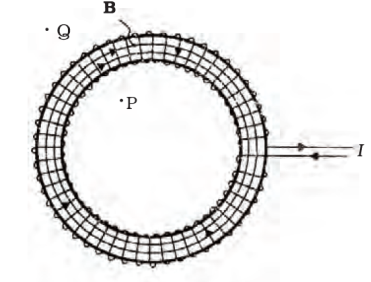
Figure: Toroid carrying  current I.

Current I is flowing through it. We shall see that the magnetic field in the open space inside (point P) and exterior to the toroid (point Q) is zero. The field B inside the toroid is constant in magnitude for the ideal toroid of closely wound turns.

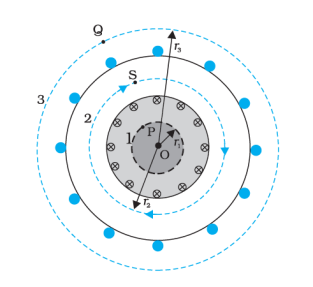
Figure: A sectional view of the toroid.

The direction of the magnetic field inside is clockwise as per the right-hand thumb rule for circular loops. Three circular Amperian loops 1, 2 and 3 are shown by dashed lines. By symmetry, the magnetic field should be tangential to each of them and constant in magnitude for a given loop. The circular areas bounded by loops 2 and 3 both cut the toroid: so that each turn of current carrying wire is cut once by the loop 2 and twice by the loop 3.

Let the magnetic field along loop 1 be B1 in magnitude.

Ampere’s circuital law

L = 2πr1.

However, the loop encloses no current, so Ie = 0. Thus,

B1 (2πr1) = μ0(0)

B1 = 0

Thus, the magnetic field at any point P in the open space inside the toroid is zero.

We shall now show that magnetic field at Q is likewise zero. Let the magnetic field along loop 3 be B3. Once again from Ampere’s law L = 2πr3.  However, from the sectional cut, we see that the current coming out of the plane of the paper is cancelled exactly by the current going into it. Thus,

Ie= 0, and B3 = 0.

Let the magnetic field inside the solenoid be B. We shall now consider the magnetic field at S.

Thus again by Ampere’s law

We find,

L = 2πr

The current enclosed Ie is (for N turns of toroidal coil) = NI

B (2πr) = μ0NI

B  = μ0NI/2πr

Compare the two results: for a toroid and solenoid.

Let r be the average radius of the toroid and n be the number of turns per unit length. Then

N = 2πr n = (average) perimeter of the toroid × number of turns per unit length and thus,

B = μ0 nI 

This is the result for the solenoid.

In an ideal toroid the coils are circular. In reality the turns of the toroidal coil form a helix and there is always a small magnetic field external to the toroid.

Q19) What is Helmholtz coil? Calculate magnetic field for it using ACL?

A19)

A Helmholtz coil is a parallel pair of identical circular coils spaced one radius apart and wound so that the current flows through both coils in the same direction. This winding results in a uniform magnetic field between the coils with the primary component parallel to the axes of the two coils.

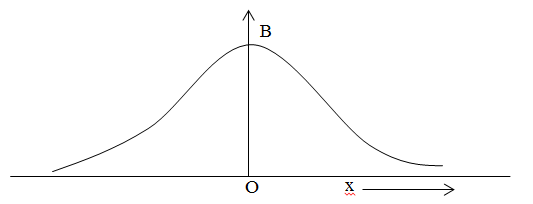
Figure: Graph between B and x

Consider a circular current carrying coil carrying current I. The magnetic field at any axis at a distance x from the centre of the coil is

From equation-(i) it is seen that the magnetic field varies with varying a distance from centre to at any point along the axis. It is seen that from above graph the magnetic field due to single circular current carrying coil cannot be uniform.

Helmholtz found that when two identical co-axial circular current carrying coil carrying current in same direction are placed at a distance which is equal to the radius of the coil, the magnetic field due to coil is uniform. It is due to magnetic field of one coil balanced by the magnetic field of other coil. i.e. if the magnetic field of one coil is decreased then the magnetic field of other coil is increased and the net magnetic field remains constant.

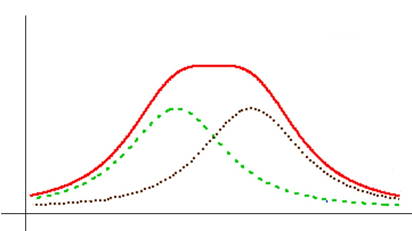
Figure: Net magnetic field remains constant

Magnitude of B:

The magnetic field due to circular current carrying coil having n number of circular turns carrying current I at a distance x from the centre of the coil is

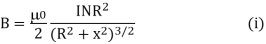
The rate of increasing or decreasing of magnetic field is given by differentiating b with respect to x, we get

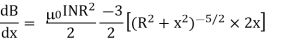
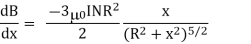
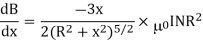
Since the magnetic field is uniform then dB/dx  is constant. Now

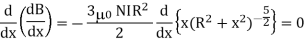
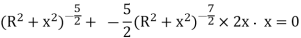
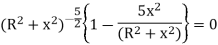
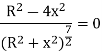
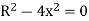
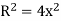
The magnitude of magnetic field is maximum in midway of the space between two current carrying coil.

We know that

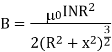
If then

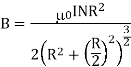
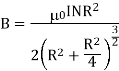
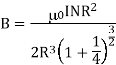
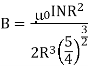
Total magnetic field is

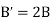
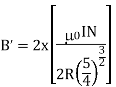
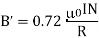
Q20) Explain construction and working of Ballistic Galvanometer?

A20)

BALLISTIC GALVANOMETER

The galvanometer which is used for estimating the quantity of charge flow through it is called the ballistic galvanometer. The working principle of the ballistic galvanometer is very simple. It depends on the deflection of the coil which is directly proportional to the charge passes through it. The galvanometer measures the majority of the charge passes through it in spite of current.

Construction of Ballistic Galvanometer

• The ballistic galvanometer consist coil of copper wire which is wound on the non-conducting frame of the galvanometer.
• The phosphorous bronze suspends the coil between the north and south poles of a magnet.
• For increasing the magnetic flux the iron core places within the coil.
• The lower portion of the coil connects with the spring. This spring provides the restoring torque to the coil.

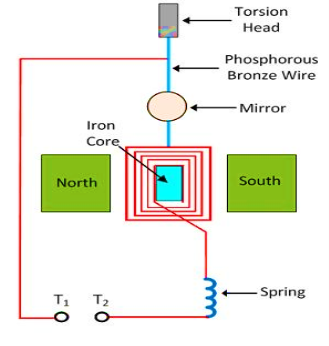
Figure: Ballistic-galvanometer

• When the charge passes through the galvanometer, their coil starts moving and gets an impulse.
• The impulse of the coil is proportional to the charges passes through it.
• The actual reading of the galvanometer achieves by using the coil having a high moment of inertia.
• The moment of inertia means the body oppose the angular movement.
• If the coil has a high moment of inertia, then their oscillations are large. Thus, accurate reading is obtained.

Theory of Ballistic Galvanometer

Consider the rectangular coil having N number of turns placed in a uniform magnetic field.

Let l be the length and b be the breadth of the coil.

The area of the coil is

A =lb   …….. (1)

When the current passes through the coil, the torque acts on it. The magnitude of the torque is

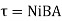   …….. (2)

Let the current flow through the coil for very short duration says dt and it is given by

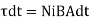   …….. (3)

If the current passing through the coil for t seconds,

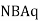=   …….. (4)

The q be the total charge passes through the coil. The moment of inertia of the coil is given by I, and the angular velocity through ω. The angular momentum is

L = Iω    …….. (5)

The angular momentum of the coil is equal to the force acting on the coil. Thus from equation (4) and (5), we get.

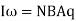  …….. (6)

The Kinetic Energy (K) deflects the coil through an angle θ, and this deflection is restored through the spring.

Restoring torque =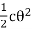

Kinetic energy =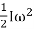

The resorting torque of the coil is equal to their deflection. Thus,

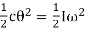
  …….. (7) 

The periodic oscillation of the coil is given as

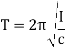
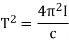
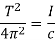
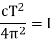
By multiplying the equation (7) from the above equation

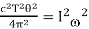
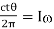…….. (8)

On substituting the value of equation (6) in the equation (8) we get

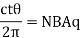
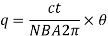
Let

The K is the constant of the ballistic galvanometer and is called the ballistic reduction factor.

Uses of ballistic galvanometers

• To compare capacities of capacitors.
• To compare e m f of cells.
• To find self and mutual inductance of coils.
• To find the magnetic flux or to find the intensity of a magnetic field.
• To find the angle of dip at a place using earth inductor.
• To find a high resistance, by method of leakage through a capacitor.

Q21) Derive an expression for torque on a current loop?

A21)

Let us consider a rectangular loop carrying a steady current I and placed in a uniform magnetic field experiences a torque. It does not experience a net force. This behaviour is analogous to that of electric dipole in a uniform electric field

We first consider the simple case when the rectangular loop is placed such that the uniform magnetic field B is in the plane of the loop. This is illustrated in Figure.

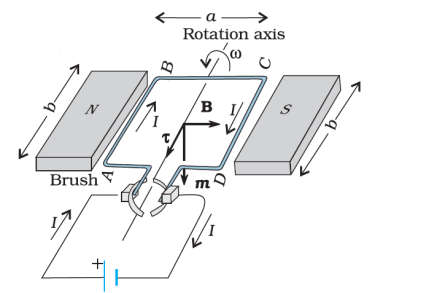
Figure: A rectangular current-carrying coil in uniform magnetic field

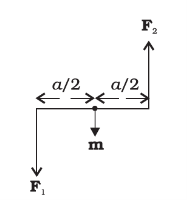
Figure: The couple acting on the coil.

The field exerts no force on the two arms AD and BC of the loop. It is perpendicular to the arm AB of the loop and exerts a force F1 on it which is directed into the plane of the loop. Its magnitude is,

F1 = I b B

Similarly, it exerts a force F2 on the arm CD and F2 is directed out of the plane of the paper.

F2 = I b B = F1

Thus, the net force on the loop is zero. There is a torque on the loop due to the pair of forces F1 and F2.

Figure shows a view of the loop from the AD end. It shows that the torque on the loop tends to rotate it anticlockwise. This torque is (in magnitude),

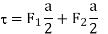
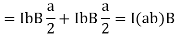
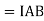
Where A = ab is the area of the rectangle.

We next consider the case when the plane of the loop, is not along the magnetic field, but makes an angle with it. We take the angle between the field and the normal to the coil to be angle θ. (The previous case corresponds to θ = π/2).

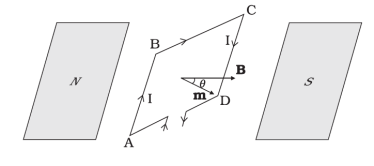
Figure: The area vector of the loop ABCD makes an arbitrary angle θ with the magnetic field

The forces on the arms BC and DA are equal, opposite, and act along the axis of the coil, which connects the centres of mass of BC and DA. Being collinear along the axis they cancel each other, resulting in no net force or torque. The forces on

Arms AB and CD are F1 and F2. They too are equal and opposite, with magnitude

F1 = F2 = IbB

But they are not collinear. This results in a couple as before. The torque is, however, less than the earlier case when plane of loop was along the magnetic field. This is because the perpendicular distance between the forces of the couple has decreased.

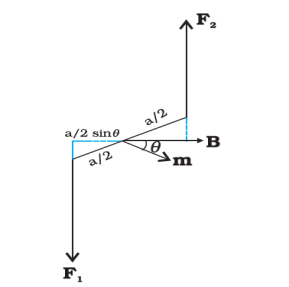
Figure: Top view of the loop. The forces F1 and F2 acting on the arms AB and CD are indicated.

Figure is a view of the arrangement from the AD end and it illustrates these two forces constituting a couple. The magnitude of the torque on the loop is,

F1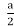 sinθ + F2 sinθ

=IabB sinθ

= IAB sinθ

As θ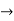0, the perpendicular distance between the forces of the couple also approaches zero. This makes the forces collinear and the net force and torque zero.

The torques in equations can be expressed as vector product of the magnetic moment of the coil and the magnetic field. We define the magnetic moment of the current loop as,

m = I A

Where the direction of the area vector A is given by the right-hand thumb rule and is directed into the plane of the paper. Then as the angle between m and B is θ. Torque can be expressed by

The dimensions of the magnetic moment are [A][L2] and its unit is Am2.

We see that the torque t vanishes when m is either parallel or antiparallel to the magnetic field B. This indicates a state of equilibrium as there is no torque on the coil (this also applies to any object with a magnetic moment m).

When m and B are parallel the equilibrium is a stable one. Any small rotation of the coil produces a torque which brings it back to its original position. When they are antiparallel, the equilibrium is unstable as any rotation produces a torque which increases with the amount of rotation. The presence of this torque is also the reason why a small magnet or any magnetic dipole aligns itself with the external magnetic field.

If the loop has N closely wound turns, the expression for torque become

m = N I A

Q22) What do you mean by current and charge sensitivity?

A22)

Current Sensitivity (Figure of merit): The figure of merit or current sensitivity of a moving coil galvanometer is the current required to produce a deflection of 1 mm on a scale kept at a distance of 1 m from the mirror. It is expressed in μA/mm. Current sensitivity is

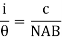
The current sensitivity can be defined as the deflection produced by unit current on a scale kept at a distance 1m from the mirror of the galvanometer. Current sensitivity = θ/i

With this definition the figure of merit is the reciprocal of the current sensitivity.

Charge sensitivity: The charge sensitivity (the ballistic reduction factor) of a moving coil galvanometer is the charge (transient current) required to produce a deflection (throw or kick) of 1 mm on a scale kept at a distance of 1 m from the mirror. Charge sensitivity is

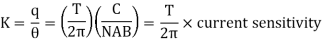
Q23) Explain electromagnetic damping?

A23)

A moving coil galvanometer consists of a rectangular current carrying coil suspended in a uniform radial magnetic field. The current through the coil produces a torque which tries to rotate the coil. A restoring torque, provided by the stiffness of the suspension, is set up in the system and it counterbalances the electromagnetic torque.

Damping factors

1. Viscous drag of air and mechanical friction. The damping couple due to this is

Proportional to the angular velocity of the system. Usually it is very small and is

Neglected.

2. Induced currents in the neighbouring conductors. These currents produce two types of damping couple, the open circuit damping couple and the closed circuit damping couple.

The former one, according to the law of electromagnetic induction, is proportional to the angular velocity and is represented by -b 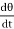where, b is the damping coefficient. The latter one is directly proportional to the angular velocity and inversely proportional to the resistance of the circuit. It is given by

Where,  involves all the coil constants such as area, magnetic flux and so on.

If C is the restoring couple per unit twist of the suspension and I is the moment of inertia of the oscillating system, the equation of motion is given by,

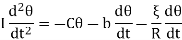
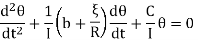
On substituting

and

Then

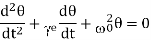
This equation is similar to the equation for a damped harmonic oscillator given by

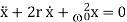
Comparing these, we get

and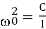

For damped oscillations, we have

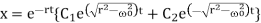
Then

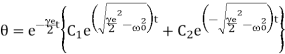
Where, C1 and C2 are undetermined constants to be evaluated from the initial conditions

Case 1: Non-oscillatory, aperiodic or dead beat motion

If the damping is high such that

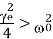
The exponents of equation are real and the motion of the system is non-oscillatory. The angular displacement decays. This is the case of dead beat motion. The requirements of a dead beat galvanometer are,

1. The moment of inertia I of the system must be small.
2. The electromagnetic rotational resistance ‘b’ should be large and the suspension is not fine.
3. The term , which involves all coil constants, should be large and the coil should be wound on a conducting frame.
4. The resistance R must be small.

Case 2: Critical damping

When

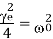
The galvanometer is said to be critically damped. The motion of the coil is non-oscillatory and it comes to rest in a minimum time after deflection.

Case 3: Light damping: Ballistic motion

When

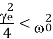
The exponents of the bracketed terms of equation are imaginary. Hence the motion of the coil is oscillatory in this case. The solution is given by

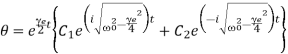
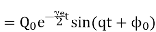
Where,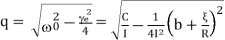

The motion of the galvanometer is said to be ballistic when the damping factor

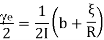
Is small. The requirements of a ballistic galvanometer are reverse of those for a dead beat galvanometer and are,

1. The moment of inertia I of the system must be large.
2. The electromagnetic rotational resistance ‘b’ is small and the suspension is fine.
3. The term , which involves all coil constants, should be small and the coil should be wound on a non-conducting frame like wood or paper.
4. The resistance R must be large.

Q24) What is Logarithmic damping?

A24)

We have assumed that the whole kinetic energy imparted to the coil is completely used to twist the suspension wire. This is not exactly true. In

Actual practice the motion of the coil is damped by air resistance and induced current in the coil.

The first throw of the galvanometer is, therefore, smaller than it would have been in the absence of damping. So it is necessary to apply a correction for damping to the first throw of the galvanometer.

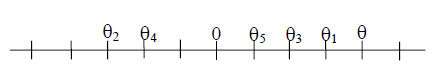
Figure: Logarithmic damping

Let θ1, θ2, θ3, …… be the successive maximum deflections from the zero position to the right and left as shown in the figure. Then it is found that,

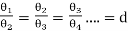……………. (1)

Where, d is a constant called the decrement per half vibration. Let d = eλ. Then, λ = loged. Here λ is called as the logarithmic decrement. Thus for a complete oscillation

……………. (2)

For one fourth of an oscillation the RHS of eqn.2 is eλ/2. Let θ be the true first throw in the absence of damping. The actual first throw in presence of damping is θ1, which is observed after the coil completes a quarter of vibration. Thus the decrement for θ is given by,

……………. (3)

Taking fourth root of eqn.2, we get,

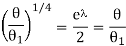
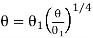……………. (4)

Or approximately,

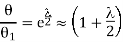
……………. (5)

We calculate λ by observing the first 11 consecutive throws. Then

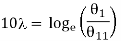
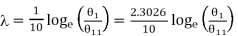……………. (5)

Then damping is given by

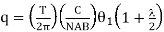……………. (6)