Unit - 3

EM Wave in Bounded Media

Q1) Describe the Boundary conditions.

A1)

It's important to distinguish between boundary and interface conditions. The space in which the electromagnetic field is calculated must be constrained by some boundaries for numerical calculations. This is accomplished by assuming physically correct and numerically solvable in finite time boundary conditions. The boundary conditions may revert to a simple interface condition in some cases. A fully reflecting (electric wall) boundary is the most common and straightforward example, in which the outer medium is treated as a perfect conductor. It gets more complicated in some cases: for instance, reflection-less (i.e., open) boundaries are simulated as a perfectly matched layer or magnetic wall that does not resume to a single interface.

Intersection of dielectric media Consider a planar dielectric interface between two media. A plane wave strikes medium 1 at an oblique angle. The interface plane is the plane that separates the two media. The propagation vector is contained in the plane of incidence, which is perpendicular to both the interface plane and the wave's phase planes.

Q2) Explain the Elementary Orientations of the Electromagnetic Fields.

A2)

There are two elementary orientations (polarizations) for the electromagnetic fields:

Polarization in the Perpendicular Direction: The magnetic field is parallel to the plane of incidence, while the electric field is perpendicular to it. Transverse Electric (TE) modes are used to configure the fields.

Polarization in the same direction: The electric field is parallel to the plane of incidence, while the magnetic field is perpendicular to it. Transverse Magnetic (TM) modes are used to configure the fields. By superimposing two waves with perpendicular and parallel polarization, any plane wave with general field orientation can be created.

Q3) Write about Reflection and refraction at a dielectric boundary.

A3)

An electromagnetic wave of real (positive) frequency  can be written

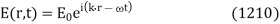
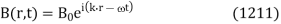
The wave-vector, k, indicates the direction of propagation of the wave, and also its phase-velocity, 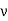, via

Since the wave is transverse in nature, we must have 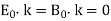. Finally, the familiar Maxwell equation

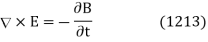
Leads us to the following relation between the constant vectors 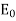 and :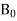

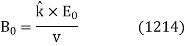
Here, 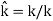  is a unit vector pointing in the direction of wave propagation.

Suppose that the plane 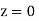forms the boundary between two different dielectric media. Let medium 1, of refractive index 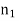, occupy the region , whilst medium 2, of refractive index 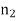, occupies the region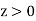. Let us investigate what happens when an electromagnetic wave is incident on this boundary from medium 1.

Q4) What happens when an electromagnetic wave is incident on this boundary from medium 1?

A4)

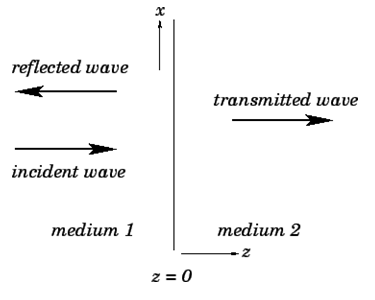
Consider, first of all, the simple case of incidence normal to the boundary. In this case,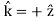 for the incident and transmitted waves, and  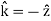for the reflected wave. Without loss of generality, we can assume that the incident wave is polarized in the x-direction. Hence, using Eq. (1214), the incident wave can be written

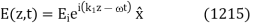
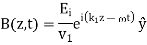
Where 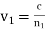is the phase-velocity in medium 1, and 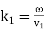. Likewise, the reflected wave takes the form

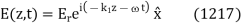
Finally, the transmitted wave can be written

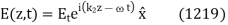
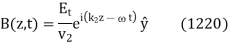
Where 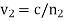 is the phase-velocity in medium 2, and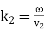 

For the case of normal incidence, the electric and magnetic components of all three waves are parallel to the boundary between the two dielectric media. Hence, the appropriate boundary conditions to apply at 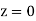 are

The latter condition derives from the general boundary condition 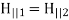, and the fact that 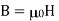in both media (which are assumed to be non-magnetic).

Application of the boundary condition (1221) yields

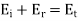  (1223)

Likewise, application of the boundary condition (1222) gives

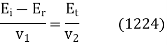
Or

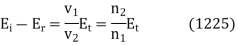
Since 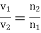 Equations (1223) and (1225) can be solved to give

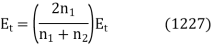
As a result, we've calculated the amplitudes of the reflected and transmitted waves in terms of the incident wave's amplitude.

Q5) Write about the shift on reflection from a boundary with a lower refractive index medium.

A5)

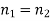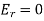It can be seen, first of all, that if then  and 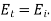 In other words, if the indices of refraction of the two media are the same, there is no reflection at the boundary between them, and the transmitted wave has the same amplitude as the incident wave. If there is some reflection at the boundary, on the other hand. In fact, the reflected wave's amplitude is roughly proportional to the difference between  and 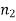. This has significant practical implications. Because of the different refractive indices of air and glass, some of the light incident at an air/glass boundary is reflected, we can only see a clean pane of glass in a window. When glass is submerged in water, as is well known, it becomes much more difficult to see. Because the refractive indices of glass and water are nearly identical, light incident on a water/glass boundary is reflected very little.

According to Eq. (1226), 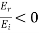 when 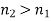. The negative sign indicates a  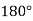phase-shift of the reflected wave, with respect to the incident wave. We conclude that there is a  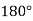On reflection from a boundary with a medium with a higher refractive index, the reflected wave shifts in phase relative to the incident wave. On the other hand, there is no phase shift on reflection from a boundary with a lower refractive index medium.

Q6) Derive an expression on the laws of reflection and refraction.

A6)

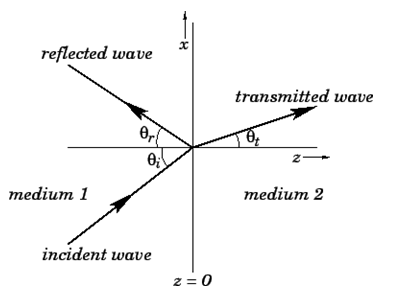
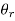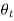Consider the case of oblique incidence to the boundary (see above Fig.). Assume that the incident wave forms an angle with the normal to the boundary, while the reflected and transmitted waves form angles with the normal to the boundary and , respectively.

The incident wave can be written

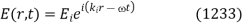
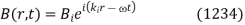
The reflected and transmitted waves have analogous expressions. Because the electric and magnetic components of the wave fields are no longer necessarily parallel to the boundary in the case of oblique incidence, the boundary conditions (1221) and (1222) at 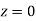 must be supplemented by the additional boundary conditions

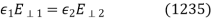
Equation (1235) derives from the general boundary condition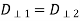 

Both components of the magnetic field are continuous at the boundary, as shown by Eqs. (1222) and (1236). As a result, we can write

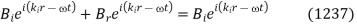
At 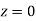. Given that 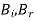and 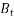 are constant vectors, the only way in which the above equation can be satisfied for all values of  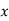and is if

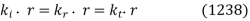
Throughout the 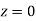 plane. This, in turn, implies that

And

It immediately follows that if 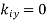 then 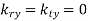. In other words, if the incident wave lies in the x-z plane then the reflected and transmitted waves also lie in the x-z plane. Another way of putting this is that the incident, reflected, and transmitted waves all lie in the same plane, known as the plane of incidence. This, of course, is one of the laws of geometric optics. From now on, we shall assume that the plane of incidence is the  plane.

Now, 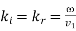 and 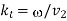. Moreover,

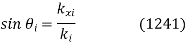
With similar expressions for  and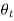. Hence, according to Eq. (1239),

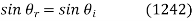
Which implies that 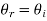. Moreover,

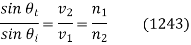
The above expressions, of course, correspond to the laws of reflection and refraction, respectively.

Q7) Write about the Unit vectors in the propagation directions of incident, reflected, and transmitted waves.

A7)

Let's take a look at the first wave polarization. Unit vectors in the propagation directions of incident, reflected, and transmitted waves can be written as follows:

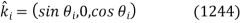
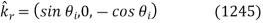
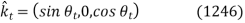
The constant vectors associated with the incident wave are written

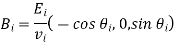
Eq. Has been used in the following cases: (1214). The constant vectors associated with reflected and transmitted waves are also constant.

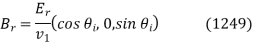
And

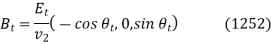
Respectively.

Now, the boundary condition (1221) yields 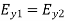 or

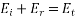 (1253)

Likewise, the boundary condition (1236) gives 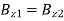, or

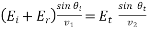 (1254)

However, using Snell's law, (1243), the above expression reduces to Eq. (1253). Finally, the boundary condition (1222) yields 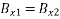, or

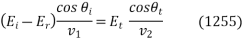
It is convenient to define the parameters

And

Equations (1253) and (1255) can be solved in terms of these parameters to give

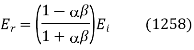
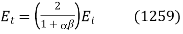
These relations are known as Fresnel equations.

The wave intensity in the z-direction is given by

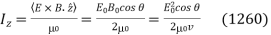
Hence, the coefficient of reflection is written

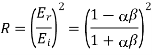
Whereas the coefficient of transmission takes the form

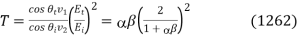
Note that it is again the case that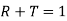 

Let's take a look at the second wave of polarization. Constant vectors associated with incident, reflected, and transmitted waves are written in this case.

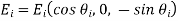   (1267)

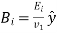     (1268)

And

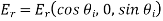   (1265)

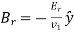     (1266)

And

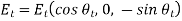   (1267)

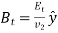     (1268)

Respectively. The boundary condition (1222) yields 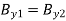, or

Likewise, the boundary condition (1221) gives , or

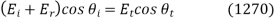
Finally, the boundary condition (1235) yields 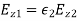, or

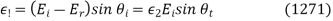
Making use of Snell's law, and the fact that 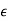 =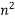, the above expression reduces to Eq. (1269).

Solving Eqs. (1239) and (1270), we obtain

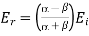   (1272)

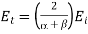   (1273)

Q8) What is the Brewster angle?

A8)

The wave polarisation with the electric field parallel to the boundary is shown on the left, while the wave polarisation with the magnetic field parallel to the boundary is shown on the right. In general, as the angle of incidence increases, the coefficient of reflection increases and the coefficient of transmission decreases. However, there is a specific angle of incidence for the second wave polarization, known as the Brewster angle, at which the reflected intensity is zero. For the first wave polarization, there is no similar behavior.

Q9) Derive an equation according to Brewster angle of the given:

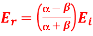
A9)

The Brewster angle corresponds to the condition, according to Eq. (1272).

Or

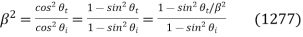
Where use has been made of Snell's law. The above expression reduces to

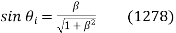
or 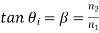. Hence, the Brewster angle satisfies

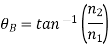
When unpolarized light is incident at the Brewster angle on an air/glass (say) boundary, the reflected beam is 100% plane polarized.

Q10) What is Law of Reflection?

A10)

Definition:

The angle of the reflected ray is equal to the angle of the incident ray when reflected from a smooth surface with respect to the normal to the surface, which is a line perpendicular to the surface at the point of contact, according to the law of reflection.

At the point of contact of the incident ray, the reflected ray is always in the plane defined by the incident ray and the normal to the surface.

The law of reflection can be used to explain the images produced by plane and curved mirrors.

Q11) State the Law of Refraction.

A11)

The law of refraction, also known as Snell's law, governs how light rays behave as they pass through a sharp interface between two transparent dielectric media.

As shown in Fig, a light ray is incident on a plane interface between two transparent dielectric media, labelled 1 and 2. The incident ray, the refracted ray, and the normal to the interface all lie in the same plane, according to the law of refraction. In addition,

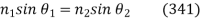
Where 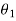 is the angle subtended between the incident ray and the normal to the interface, and  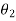is the angle subtended between the refracted ray and the normal to the interface. The quantities  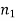and  The refractive indices of media 1 and 2 are referred to as such. As a result of the law of refraction, a light ray always deviates more towards the normal in the optically denser medium: that is, the medium with a higher refractive index. Note that 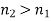  in the figure. The law of refraction also applies to non-planar interfaces, as long as the normal to the interface at any given point is understood to be the normal to the interface's local tangent plane at that point.

Q12) In a vacuum, what is the speed of light?

A12)

Consider two parallel light rays, and, which are incident at an angle to the normal at the interface between two dielectric media, 1 and 2. Let and be the refractive indices of the two media, with and being the refractive indices of the two media, respectively. From Fig., it is clear that ray must move from point to point in medium 1 in the same time interval as ray moves between points and in medium 2. Now, the speed of light in medium 1 is, while it is in medium 2. As a result, the length is determined by, whereas the length is determined by. By means of trigonometry,

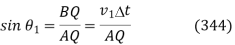
And

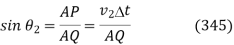
Hence,

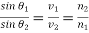                       (346)

Which can be rearranged to give Snell's law. Note that the lines 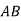 and 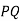 represent wave-fronts in media 1 and 2, respectively, and, therefore, cross rays  and  at right-angles.

Q13) What is Brewster’s Law?

A13)

When unpolarized light of a known wavelength is incident on a transparent substance surface, the angle of incidence whose tangent is the refractive index of the substance for the wavelength experiences maximum plan polarization.

Brewster's law describes the relationship between light waves at their maximum polarization angle. Sir David Brewster, a Scottish physicist, proposed the law in 1811, and it is named after him. The law states that at a certain angle, p-polarized rays vanish completely on different glasses.

Brewster's angle is another name for the polarization angle. It is the angle of incidence at which a ray of light with p-polarization passes through a transparent dielectric surface without reflection. While unpolarized light is transmitted at this angle, light is reflected from the surface.

Brewster discovered that the medium's refractive index is numerically equal to the tangent angle of polarization. To learn more about Brewster's Law Formula.

μ=tani

Where,

μ = Refractive index of the medium.

i = Polarization angle.

From Snell’s Law:

μ=sinisinr……..1

From Brewster’s Law:

μ=tani=sinicosi……..2

Comparing both formulas: 1 and 2

Cosi=sinr=cos(π2−r)

i=π2–r, or i+r=π2

As, i+r=π2<ABC is also equal to the π2. Therefore, the reflected and the refracted rays are at right angles to each other.

Q14) Derive the Relation Between Brewster Angle and Critical Angle.

A14)

Brewster’s angle is given as:

Ө b = arc tan (n2/n1)

TanӨ b = n2/n1

The critical angle is given as:

Ө c = arc sin (n1/n2)

SinӨ c = n1/n2

SinӨ c = 1/(n2/n1)

But we know that,

TanӨ p = n2/n1

SinӨ c = 1/tanӨ b

Therefore, 

Өc = arc sin (1/tanӨ b)

Q15) What is Reflection and Transmission Coefficients?

A15)

Consider the following two half spaces (deep ocean on top of earth, for example). When a wave of unit amplitude strikes the boundary, a transmitted wave of amplitude t and a reflected wave of amplitude c are produced, as shown in Figure below.

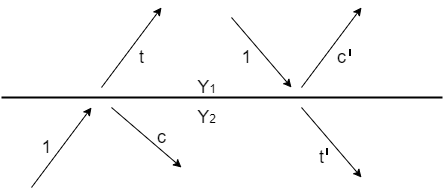
Figure: Waves incident, reflected c, and transmitted t at an interface. 

T and c have a very straightforward relationship. Pressure, material displacement, traction, or tangential electric or magnetic fields are all physical properties of wave amplitudes. On both sides of the boundary, these physical variables must have the same value. This means that the transmitted wave must be equal to the sum of incident and reflected waves.

The transmission coefficient t can be greater than unity if the reflection coefficient c is positive or negative. It might surprise you to learn that t can be greater than unity. There are no physical laws that are broken in this process. At the beach, we see waves approaching the shore, which grow in size as they get closer. The energy of a wave is not solely determined by its height. Depending on the medium in which the wave is measured, energy equals the squared wave amplitude multiplied by a proportionality factor Y. The statement that the energy before incidence equals the energy after incidence is true if the top medium's factor is Y1 and the bottom medium's factor is Y2.

               (3)

Solving for c leads us to

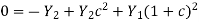
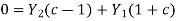
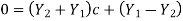
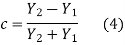
The upgoing and down going wave variables in acoustics can be normalized to either pressure or velocity. The impedance I is the scale factor multiplied by velocity squared when measuring velocity. The admittance Y is the scale factor used to measure pressure.

If Y1 and Y2 are swapped, the wave c' that reflects when energy is incident from the other side is obtained from (4). Thus  

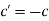
A perfectly reflecting interface is one that allows no energy to pass through it. This happens when t = 0 or c = -1, as well as when t = 2 or c = +1. To see this, look at Figure on the left.

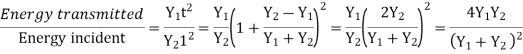
When Y1 = Y2, 100 percent of the incident energy is transmitted, but when Y1 and Y2 are very different, the percentage of transmission is very small.

Waves are typically described using two types of variables, both of which can be continuous at a material discontinuity. Pressure, tension, voltage, potential, stress, and temperature are examples of scalars. The other is a vector, of which the vertical component is used. Velocity, stretch, electric current, displacement, and heat flow are examples of the latter. A tensor is occasionally used as a wave variable. When one of the motion components vanishes as a boundary condition, the boundary is often described as rigid. When the pressure or potential disappears, the boundary is often referred to as "free." Waves with unit magnitude reflection coefficients are reflected by rigid and free boundaries.

Q16) Define Total Internal Reflection.

A16)

A high-quality mirror can reflect more than 90% of the light that strikes it while absorbing the remainder. However, having a mirror that reflects all of the light that falls on it would be beneficial. Surprisingly, we can achieve total reflection by manipulating a refraction aspect.

Consider what happens when a ray of light collides with the surface of two materials, as in below Figure a. The light is refracted in part and reflected in part as it passes through the boundary. The ray bends away from the perpendicular if the index of refraction of the second medium is less than that of the first, as shown in the figure below. (Because n1 > n2, the refraction angle is greater than the incidence angle—that is, 1 > 2.) Consider what happens as the incident angle gets wider. This causes θ2 to increase also. As shown in Figure b, the angle of refraction 2 can be as large as 90o. The incident angle 1 that produces a 90o angle of refraction is defined as the critical angle c for a combination of materials. To put it another way, c is the incident angle for which 2 = 90o. Figure c shows that if the incident angle 1 is greater than the critical angle, all of the light is reflected back into medium 1, a condition known as total internal reflection.

The incident angle θ1 that produces an angle of refraction of 90º is called the critical angle, θc.

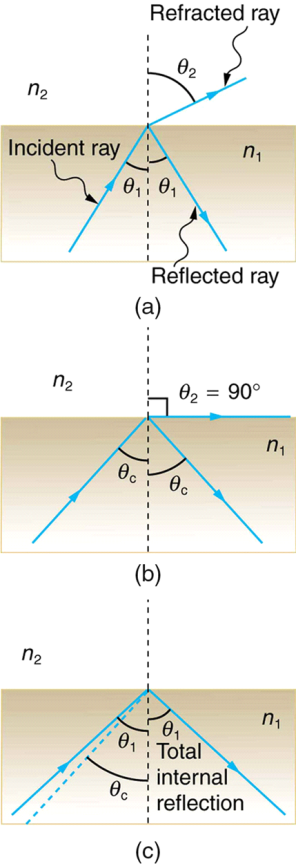
Figure: Aluminum (black line), silver (red line), gold (blue line), and copper reflectance spectra (green line).

Q17) What are the factors that leads to acoustic wave (Z).

A17)

The pressure normally disappears at the sea surface, but if we want to cause an impulsive disturbance, we can temporarily change the pressure to 1, for example. At t=0, this 1 denotes a constant frequency function that is an impulsive function of time. Figure shows the subsequent waves, with the upcoming wave -R(Z) being a result of both the downgoing 1 and the downgoing +R. (Z). D - U = 1 + 2R is the vertical component of the sea surface velocity W due to the source and the resulting acoustic wave (Z). 

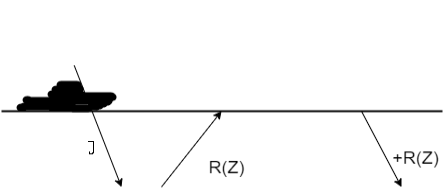
Figure: A down going disturbance 1 is followed by an upgoing reflected wave -R(Z), which reflects back down as R. (Z). D + U = 1 + R - R = 1 is the pressure at the surface.

Q18) Define Law of Reflection and Law of Refraction respectively.

A18)

Law of Reflection.

The angle of the reflected ray is equal to the angle of the incident ray when reflected from a smooth surface with respect to the normal to the surface, which is a line perpendicular to the surface at the point of contact, according to the law of reflection.

At the point of contact of the incident ray, the reflected ray is always in the plane defined by the incident ray and the normal to the surface.

The law of reflection can be used to explain the images produced by plane and curved mirrors.

Law of Refraction

The law of refraction, also known as Snell's law, governs how light rays behave as they pass through a sharp interface between two transparent dielectric media.

As shown in Fig. Below, a light ray is incident on a plane interface between two transparent dielectric media, labelled 1 and 2. The incident ray, the refracted ray, and the normal to the interface all lie in the same plane, according to the law of refraction. In addition,

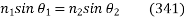
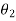Where  is the angle subtended between the incident ray and the normal to the interface, and  is the angle subtended between the refracted ray and the normal to the interface. The quantities  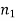and 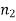 The refractive indices of media 1 and 2 are referred to as such. As a result of the law of refraction, a light ray always deviates more towards the normal in the optically denser medium: that is, the medium with a higher refractive index. Note that   in the figure. The law of refraction also applies to non-planar interfaces, as long as the normal to the interface at any given point is understood to be the normal to the interface's local tangent plane at that point.

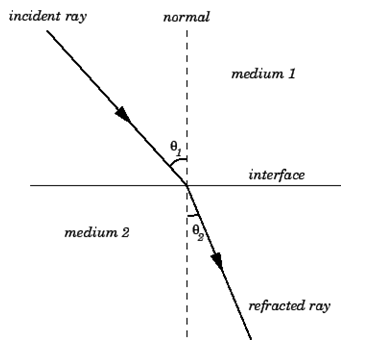
Figure: The law of refraction.