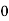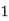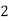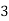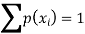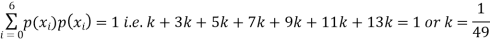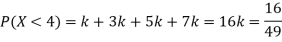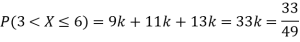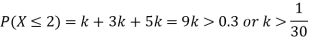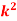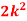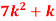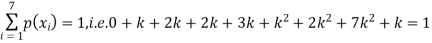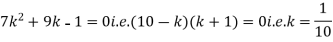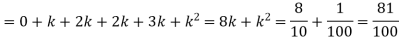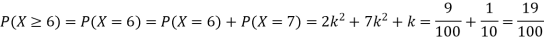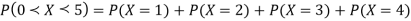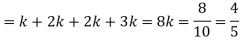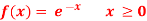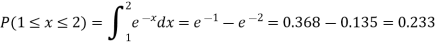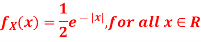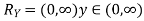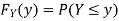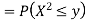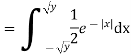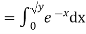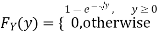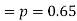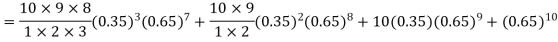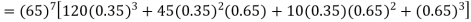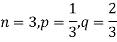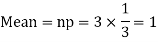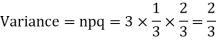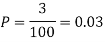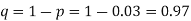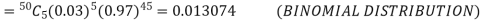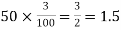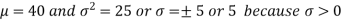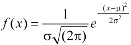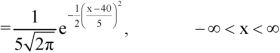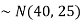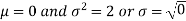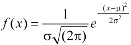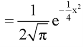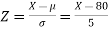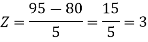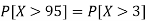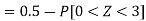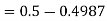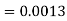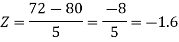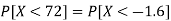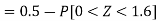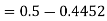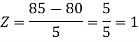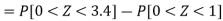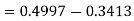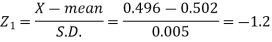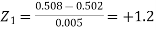The sample space will be- {HHH, HHT, HTH, THH, HTT, THT, TTH, TTT} Here variable X can take the values 0, 1, 2, 3 with the following probabilities- P[X= 0] = P[TTT] = 1/8 P[X = 1] = P [HTT, THH, TTH] = 3/8 P[X = 2] = P[HHT, HTH, THH] = 3/8 P[X = 3] = P[HHH] = 1/8 |
X |
|
|
|
|
P(x) |
|
|
|
|
|
1. We know that-
So that- 0 + c + c + 2c + 3c + c = 1 8c = 1 Then c = 1/8 Now, 2. P[1<x<4] = P[X = 2] + P[X = 3] = c + 2c = 3c = 3× 1/8 = 3/8 |
X | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
P(X) | k | 3k | 5k | 7k | 9k | 11k | 13k |
 (ii) What will be e minimum value of k so that
(ii) What will be e minimum value of k so that  A3)
A3)(i) If X is a random variable then
(i) Thus minimum value of k=1/30. |
x | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
P (x) | 0 | k | 2k | 2k | 3k |
|
|
|
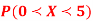 A4)
A4)(i) If X is a random variable then
|
 .
.
|
here
So that, the function can be defined as a density function. Now.
|
|
 , find the CDF of Y.A6)
, find the CDF of Y.A6)First we note that
Thus, |
The probability that a man aged 60 will live to be 70
Number of men= n = 10 Probability that at least 7 men will live to 70 = (7 or 8 or 9 or 10) = P (7)+ P(8)+ P(9) + P(10) =
|
|
(a) Hence the probability for 5 defectives bolts in a lot of 50.
(b) To get Poisson approximation m = np = Required Poisson approximation= |
 then find the probability density function of X.2. If X
then find the probability density function of X.2. If X  then find the probability density function of X.A10)
then find the probability density function of X.A10)1. We are given X Here We know that-
Then the p.d.f. will be-
2. . We are given X Here We know that-
Then the p.d.f. will be-
|
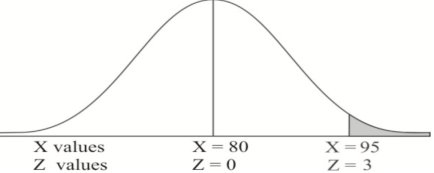
The standard normal variate is – Now- 1. X = 95,
So that-
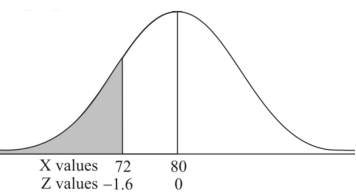
2. X = 72,
So that-
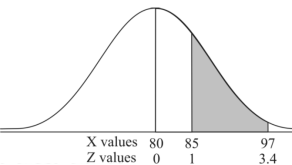
3. X = 85,
X = 97,
So that-
|
Here-
And Area for non-defective washers = area between z = -1.2 to +1.2 = 2 area between z = 0 and z = 1.2 = 2 × 0.3849 = 0.7698 = 76.98% Then percent of defective washers = 100 – 76.98 = 23.02 % |