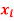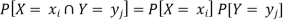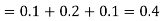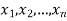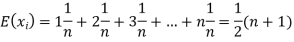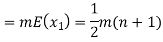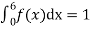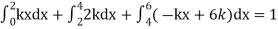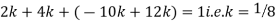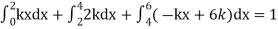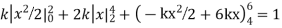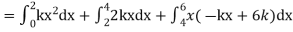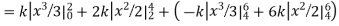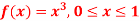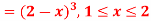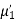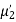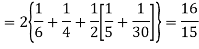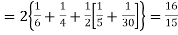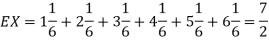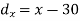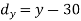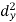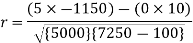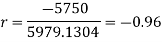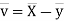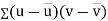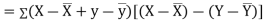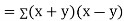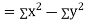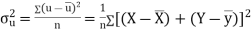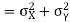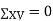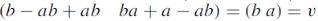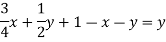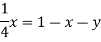| 1 | 2 |
P [ X = | 0.7 | 0.3 |
| -2 | 5 | 8 |
P [ Y = | 0.3 | 0.5 | 0.2 |
Since X and Y are independent random variables,
Thus the entries of the joint distribution are the products of the marginal entries
|
X/Y | 1 | 2 |
1 | 0.1 | 0.2 |
2 | 0.1 | 0.3 |
3 | 0.2 | 0.1 |
X/Y | 1 | 2 | p(x) [totals] |
1 | 0.1 | 0.2 | 0.3 |
2 | 0.1 | 0.3 | 0.4 |
3 | 0.2 | 0.1 | 0.3 |
p(y) [ totals] | 0.4 | 0.6 | 1 |
X | 1 | 2 | 3 |
p(x) | 0.3 | 0.4 | 0.3 |
Y | 1 | 2 |
p(x) | 0.4 | 0.6 |
|
X | 1 | 2 | 3 |
| ¼ | 1/4 | ½ |
Which gives-
|
Let
Therefore expected value of the sum of the numbers on the tickets drawn
|
|
Since the total probability is unity.
Mean of X =
|
|
Total frequency N =
Hence, i.e., standard deviation Mean derivation about the mean
|
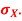 A6)
A6)We have
Thus,
|
X | 10 | 20 | 30 | 40 | 50 |
Y | 90 | 85 | 80 | 60 | 45 |
X | Y |
|
|
|
|
|
10 | 90 | -20 | 400 | 20 | 400 | -400 |
20 | 85 | -10 | 100 | 15 | 225 | -150 |
30 | 80 | 0 | 0 | 10 | 100 | 0 |
40 | 60 | 10 | 100 | -10 | 100 | -100 |
50 | 45 | 20 | 400 | -25 | 625 | -500 |
Sum = 150 |
360 |
0 |
1000 |
10 |
1450 |
-1150 |
|
 the
the  of correlation between
of correlation between 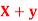 and
and 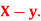 A8)
A8)Let Then Now Similarly, Now
Also
Similarly,
|
 = (b a) is a fixed point of the stochastic matrix-
= (b a) is a fixed point of the stochastic matrix-
|
|
|
Suppose t = (x, y, z) be the fixed probability vector. By definition x + y + z = 1. So t = (x, y, 1 − x − y), t is said to be fixed vector, if t P = t
On solving, we get-
Required fixed probability vector is-
|