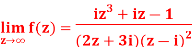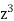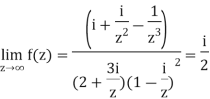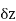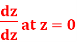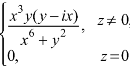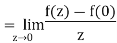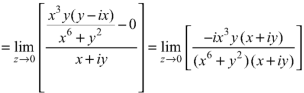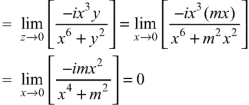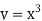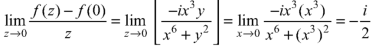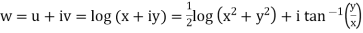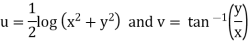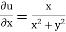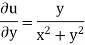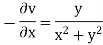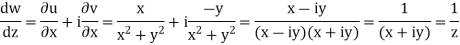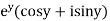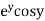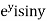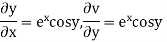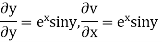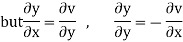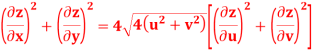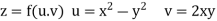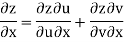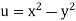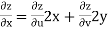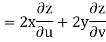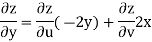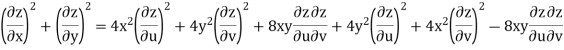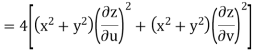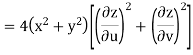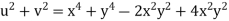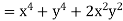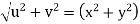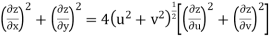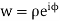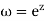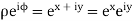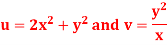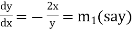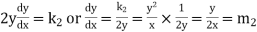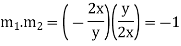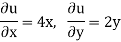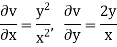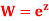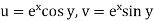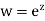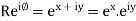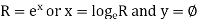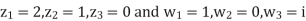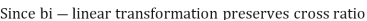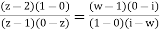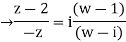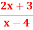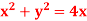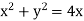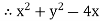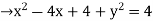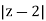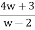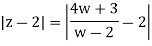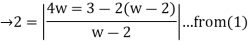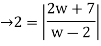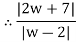|
. Here we have-
Divide numerator and denominator by
Q2: Let f(z) be a single valued function of the variable z, then
Provided that the limit exists and has the same value for all the different ways in which |
|
If z→0 along radius vector y = mx
But along
In different paths we get different value of |
 . Also determine where w is non-analytic.A3)
. Also determine where w is non-analytic.A3)Here we have Therefore-
Again-
Hence the C-R conditions are satisfied also the partial derivatives are continuous except at (0 , 0). So that w is analytic everywhere but not at z = 0
|
 is an analytical function.A4)
is an analytical function.A4)Let Let
Hence C-R-Equation satisfied. |
|
Given that
Since
V=2xy
Now
But
Hence |
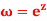 is conformal in the whole of the z plane.A6)
is conformal in the whole of the z plane.A6)Let z=x+iy
Then
|
 which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into
which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into 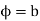 which is a radius vector in the w plane. The angle between the line x=a and y=b in the z plane is a right angle. The corresponding angle in the w plane between the circle e = constant and the radius vector
which is a radius vector in the w plane. The angle between the line x=a and y=b in the z plane is a right angle. The corresponding angle in the w plane between the circle e = constant and the radius vector 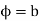 is also a right angle which establishes that the mapping
is also a right angle which establishes that the mapping 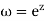 is conformal. Q7) Show that the curve u = constant and v = constant cut orthogonally at all intersections but the transformation w = u + iv is not conformal. Where-
is conformal. Q7) Show that the curve u = constant and v = constant cut orthogonally at all intersections but the transformation w = u + iv is not conformal. Where-
|
Let Differentiate (1), we get-
Now-
Differentiate (3), we get-
As we know that for the condition for orthogonallity, from (2) and (4)
So that these two curves cut orthogonally. Here,
And
Here the C-R equation is not satisfied so that the function u + iv is not analytic. Hence the transformation is not conformal. |

|
Now equating real and imaginary parts-
Again
Hence
|
Let
Thus we have
=
|
w= |
Consider
Thus, centre of the circle is (h,k) Thus in z-plane it is given as Consider w= W(z-4) = 2z+3 Wz-4w=2z+3 Wz-2z=4w+3 Z(w-2) = (4w+3)
z-2 =
|